Distributed hydrological models for addressing effects of spatial variability of roughness on overland flow

*,Yin Liu,Mio-mio LiBo Ling

aCollege of Earth Science and Engineering,Shandong University of Science and Technology,Qingdao 266590,China

bCollege of Mining and Safety Engineering,Shandong University of Science and Technology,Qingdao 266590,China

Distributed hydrological models for addressing effects of spatial variability of roughness on overland flow

Sheng-tang Zhanga,*,Yin Liub,Miao-miao Lia,Bo Lianga

aCollege of Earth Science and Engineering,Shandong University of Science and Technology,Qingdao 266590,China

bCollege of Mining and Safety Engineering,Shandong University of Science and Technology,Qingdao 266590,China

Available online 9 July 2016

In this study,we investigated the origin of the overland flow roughness problem and divided the current overland flow roughness research into three types,as follows:the first type of research takes into account the effects of roughness on the volume and velocity of surface runoff,f l ood peaks,and the scouring capability of flows,but has not addressed the spatial variability of roughness in detail;the second type of research considers that surface roughness varies spatially with different land usage types,land-cover conditions,and different tillage forms,but lacks a quantitative study of the spatial variability;and the third type of research simply deals with the spatial variability of roughness in each grid cell or land type.We present three shortcomings of the current overland flow roughness research,including(1)the neglect of roughness in distributed hydrological models when simulating the overland flow direction and distribution,(2)the lack of consideration of spatial variability of roughness in hydrological models,and(3)the failure to distinguish the roughness formulas in different overland flow regimes.To solve these problems, distributed hydrological model research should focus on four aspects in regard to overland flow:velocity field observations,flow regime mechanisms,a basic roughness theory,and scale problems.

Distributed hydrological model;Overland flow;Roughness;Spatial variability

1.Introduction

Overland flow,also known as sheet flow or overflow,is gravity-driven flow that occurs on channelized surfaces.It is formed when rainfall or snowmelt does not inf i ltrate the soil or collect in surface depressions(i.e.,channels or surface water bodies).Overland flow generally occurs near watershed divides on the upper part of a slope,and is considered to be shallow water flow that covers the slope.It forms sheet-like flows when there is a large flow rate,but can become a brooklet intertwined through separate mesh trickles.It is easily affected by changes in resistance and micro-terrain,and also has non-unique flow directions.

Overland flow forms the major part of rivers,streams, lakes,etc.,and its movement towards water bodies is accompanied by pollutant transport and soil leaching.Therefore,studying overland flow is important to understanding slope hydrologic processes and soil erosion mechanisms. Theoretically,distributed hydrological models can accurately simulate the overland flow process using precise discrete grid cells(Wang and Hjelmfelt,1998).However,in reality, compared to lumped hydrological models,distributed models sometimes produce unsatisfactory or even incorrect simulations of the velocity fields among the grid cells,even though they can simulate the runoff at the watershed exit by various means(e.g.,parameter adjustment)(Mu¨gler et al.,2011; McDonnell and Beven,2014).The main cause of theproblem stated above is the simple processing of the complex slope roughness effects in distributed hydrological models. There have been numerous achievements in the study of roughness.However,previous research has differed in levels and has arrived at different conclusions because roughness varies not only with boundary characteristics but also with flow velocity,water depth,and other hydraulic factors.Even a tiny change in roughness can have a marked impact on the flow characteristics(Darboux et al.,2002;Candela et al., 2006;Sahoo et al.,2006).Actual conditions of basins are varied.When water channel networks develop in humid areas, overland flow will soon reach a river system,and slope characteristic factors have little effect in these areas.In contrast,in arid areas,overland flow has a longer pathway to the river system,and the main mechanism of flow generation is excess inf i ltration.When water flow is impeded by surface roughness, it will affect the f i nal runoff volume because the inf i ltration rate is high and the inf i ltration will continue along the pathway.In this sense,different climatic regions have different surface roughness effects on overland flow.Different hydrological models can be applied to different climatic regions due to the diverse flow generation patterns(Liu et al.,2009).The numerous factors and their interactions with roughness further complicate the effect.

In this study,we investigated the origin of the overland flow roughness problem,classified the methods used to address it, and pointed out the inappropriate aspects of surface roughness research in theory.Moreover,we present possible solutions to the roughness problem based on the current research status.

2.Origin of roughness problem

Surface roughness was first investigated by de Chezy,who presented a formula of resistance including the Chezy coeff icient.Later,Ganguillet and Kutter introduced the concept of roughness when they studied the Chezy coeff i cient.Subsequently,Manning established the Manning resistance formula, which was translated later to another formula with the roughness coeff i cient,and this coeff i cient is the so-called roughness at present(Smith et al.,2007).Through these studies,roughness has developed a definite meaning:a comprehensive coeff i cient that represents the blocking effect of the solid coarse surface on flow.In regard to open channel flow,roughness is an integrated hydraulic resistance coef ficient.It varies not only with the surface characteristics but also with the flow volume,water depth,and other hydraulic factors. In the investigation of open channel flow,an increasing number of researchers have begun to consider the roughness change in different flow regimes.The interactions between flow quantity,water depth,and velocity in different regimes make roughness a complicated problem.Wu and Christensen (2007)used a wind tunnel test to study the effects of surface roughness on velocity distribution,shear stress,and other hydraulic factors of turbulence.

Unlike open channel flow,overland flow is usually shallow flow on a slope,which can be solved with the Saint-Venant equation and the Manning formula as follows:

where h represents the average depth of overland flow,t is time,q represents the fl ux per unit width,r is the net input fl ux,l is the slope length,sfrepresents the friction gradient that approximately equals the directional gradient,v is the overland flow velocity,and n is the Manning roughness coef fi cient of the slope.

Currently,researchers use the available knowledge of pipe and open channel flow to address the overland flow roughness coeff i cient in Eq.(1).However,because the overland flow is formed by precipitation and moves along the slope,it has various flow directions,as opposed to open channel flow.

Overland flow changes speed and flow direction frequently because of the effects of water depth,micro-terrain,resistance, and other factors.The pathway of overland flow may differ because of rainfall volume,humidity,or other climatic conditions.A distributed hydrological model used to simulate overland flow is generally affected by the overland flow characteristics,which change easily according to earth surface,hydrological,and meteorological conditions.

In general,shallow flow is signif i cantly affected by the friction of the solid surface,and is simultaneously affected by the surface geological types,land usage types,cultivation,and other spatial variation factors.Consequently,slope roughness has spatial variability,which complicates the overland flow roughness problem.Because of the unique features stated above,overland flow roughness has received considerable attention.Overland flow is easily affected by the change of resistance and micro-terrain because of thin-layer flow.Therefore,flow direction and distribution become complex.Macroscopic slope topography determines the large-scale direction of convergence,but at the grid scale,the variations of the microterrain and resistance in different directions within the inner grid will affect the pathway of overland flow in the grid cell. Inaccurate simulations of these effects will result in a loss of information about the real flow path and may lead to biases of flow length(Liu et al.,2012).Thus,the simulation results of distributed hydrological models will be highly variable.

3.Current research on overland flow roughness

Overland flow roughness has become the most active research area in hydrology,and substantial efforts have been made by researchers with different research objectives.After analyzing their achievements,we divided all of the methods for handling roughness into three types.

3.1.First type

In the first type of methods,it is assumed that roughness affects the volume and velocity of surface runoff,f l ood peaks, and the scouring capability of flow(Lumbroso and Gaume,2012).However,these methods have not addressed the spatial variability of roughness in detail.Smith et al.(2007)classi fi ed flow into pipe flow,open channel flow,and overland flow. Then,they demonstrated that roughness plays an important role in overland flow and that its in fl uence changes when it affects other types of flow.Moreover,a roughness classi fi cation method was presented.In the research of Cea et al. (2014),the micro-roughness characteristics of the terrain were taken into account to correctly reproduce the flow hydrodynamics.Shit and Maiti(2012)used tests to reveal that flow velocity is not directly controlled by the rill gradient; rather,it is in fl uenced by the hydraulic roughness coef fi cient.

3.2.Second type

In the second type of methods,it is demonstrated that surface roughness varies spatially with different land usage types,land-cover conditions,and even different tillage forms. However,the spatial variability of roughness has not been studied quantitatively.Helmers and Eisenhauer(2006)showed that roughness is a spatial variable,and its spatial variability can change the runoff by 14%—18%.By using a onedimensional unsteady flow model to study plain f l oods and by calibrating the Manning coeff i cient for different slope types,Remo and Pinter(2007)concluded that the roughness varies with slope type.Medeiros et al.(2012)discussed the possible runoff prediction errors that occur when the surface roughness distribution is automatically determined by land usage type and land-cover conditions.Jin et al.(2000)suggested that the roughness coeff i cient is highly related to the shear stress and drag force.The resistance due to vegetation was related to vegetation density and flow depth.Stoof et al. (2015)conf i rmed that the decrease in vegetation coverage decreases the average slope roughness,and then increases runoff and erosion risk in these areas.

3.3.Third type

In the third type of methods,the spatial variability of roughness is considered and simply handled in each grid cell or land type.Saxena and Perumal(2014)characterized land strips with a set of uniform Manning's roughness coeff i cients. They also determined the roughness coeff i cients according to the land-cover type classif i cation method.Based on the spatial variation of surface frictional resistance,Schumann et al. (2007)classified the surface roughness distribution based on data collected by satellite remote sensing.The signi fi cant infl uences of gradient,coherence of saturated surface soil,and particle size on roughness and the considerable variation of channel roughness caused by surface soil characteristic variations were demonstrated by Torri et al.(2012).The Manning roughness coef fi cient was tested under various flow conditions and with different vegetation parameters by Noarayanan et al. (2012),who regarded this coef fi cient as a proper tool to calculate and describe the resistance to shallow flow.

Realistically,it is dif fi cult to take into account the spatial variability of roughness.Due to the complexity of the factors in modern catchments and the lack of a foundational theory in overland flow roughness studies,distributed hydrological models do not always provide satisfactory results in watershed hydrological process simulations when the effect of roughness is considered.In conclusion,of the three types of roughness handling methods mentioned above,it is diff i cult to determine which one is better.Currently,researchers choose their methods according to different objectives in research and applications.

4.Shortages of distributed hydrological models for roughness effect simulation

Most of the studies on overland flow roughness have aimed to meet the requirements of accurate overland runoff process simulation.When distributed hydrological models are used to simulate overland flow roughness,several problems arise.

4.1.Neglect of roughness

Numerous studies show that the roughness factor has a signif i cant effect on the overland flow concentration(Lane and Woolhiser,1977;Podmore and Huggins,1980;Shen et al., 1994;Straatsma and Baptist,2008;Laloy and Bielders, 2008),and it has been concluded that this factor also affects flow directions and distributions among grid cells divided by the distributed overland flow concentration model(Zhang and Kang,2005).The single flow direction arithmetic method, multiple flow direction arithmetic method,and topographic index method in TOPMODEL are the most popular methods currently used in distributed hydrological models to simulate the overland flow concentration(Tarboton,1997).The mathematical principles of these methods are explained below.

The D8 flow direction algorithm is a typical example of the single flow direction arithmetic method.The following formulas are used to calculate the flow direction in grid cell i:

where Ziis the elevation of grid cell i,Zjis the elevation of the adjacent cell j,Dijis the distance between the centrums of grid cells i and j,βijstands for the gradient from cells i to j,and βiacts as the gradient of real flow direction(the runoff path is the steepest).

The multiple flow direction arithmetic method has been developed on a solid physical foundation.The process of the method is demonstrated below.The f l ux per unit width passing from grid cell i to the adjacent cell j,qij,is expressed as

where nijis the surface Manning roughness coeff i cient in the direction from cells i to j,and vij,hij,and Sijare the flowvelocity,average depth of overland flow,and directional gradient from cells i to j,respectively.Sijis def i ned as

Then,the total f l ux per unit width from grid cell i to the downstream cells can be obtained:

The proportion of the flow from grid cell i to the adjacent downstream cell j accounting for the total f l ux is calculated as

The overland flow depth in grid cell i is considered a constant at a certain moment.Thus,Eq.(7)is simplified as

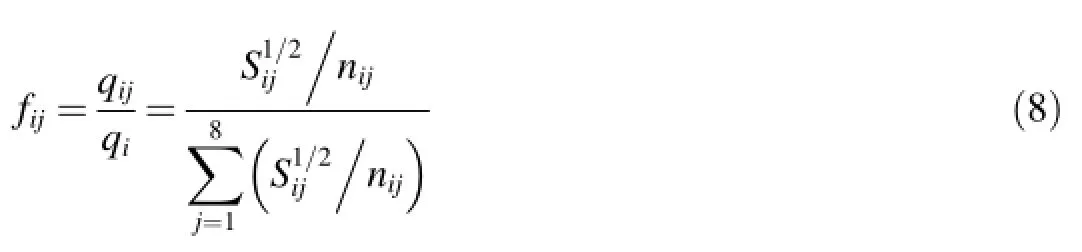
Through homogenization of the Manning roughing coeff icient,the following equation is obtained from Eq.(8):

where P is a constant without hydrological dimensions,whose variation inf l uences the flow distribution model directly.In Eq.(9),a value of 0.5 is assigned to P.With the increase of P, the flow distribution simulation result of the multiple flow direction arithmetic method tends to be similar to that of the single flow direction arithmetic method.In a sense,the flow distribution among grid cells simulated by the single flow direction arithmetic method can be regarded as an extreme case of the multiple direction arithmetic method(in the extreme case,P→+∞).Relevant studies have shown that the multiple flow direction arithmetic method,which has more precise results than the single direction arithmetic method,has replaced the previous arithmetic methods based on DEM raster data to become the primary method for calculating the flow direction and distribution among grid cells.

From the discussion above,we can see that the multiple flow direction arithmetic method fi rst homogenizes and then neglects the roughness,which leads to an inaccurate result of the distributed hydrological simulation of the flow direction and distribution among the grid cells.Due to this neglect, theoretically,distributed hydrological models are not always superior to lumped hydrological models,even with their physical foundations and precise discrete grid cells.

In TOPMODEL,the topographic index,ln（α/tan β),is used to represent the accumulation trend and downward flow trend of the runoff from a grid cell with the elevation of Z (referred to as the original grid cell below)toward a downstream grid cell with the elevation of Zˊ.α and tan β are calculated as below:

where A represents the total upstream area of the original grid cell;L is the effective contour length,vertical to the flow direction;D is the distance between the centrums of grid cells; and tan β represents the slope angle,ref l ecting the downward tendency of the runoff under gravity.

As discussed above,in the simulation of overland flow concentration using distributed hydrological models,the gradient is adopted as the only factor to calculate the flow direction and distribution in all three arithmetic methods, ignoring the effect of roughness.A slope factor is used to partially represent the effect of terrain on overland flow.With discrete grid cells,the flow direction algorithms in the highresolution grid cells of distributed hydrological models simulate the effect of variation of the micro-terrain performance by determining overland flow direction at the grid scale.If the variation of the micro-terrain is so tiny that a slope factor at a grid scale cannot ref l ect the changes,its effect on overland flow will probably be ignored by the flow direction algorithms.However,if the variation of micro-terrain is a bit larger,it will change the value of roughness of the underlying surface of the grid cell.In this case,it will indirectly affect the flow distribution in different directions by in fl uencing the value of roughness.Thus,it is necessary to consider the infl uence of roughness in the simulation of overland flow direction and distribution with the flow direction algorithms.

4.2.Lack of consideration of spatial variability of roughness

In theory,roughness is a coeff i cient that ref l ects the effect of the solid coarse surface on flow.However,in application, this coeff i cient cannot be measured directly because of a lot of factors,including water depth and velocity,roughness of the earth's surface,section form,surface cover type,and land usage type.

The method for calibration of the roughness in a single surface type with a certain kind of vegetation and surface geological conditions has been discussed widely.For example, by analyzing field f l ood data with an iterative curve f i tting method,Engman(1986)obtained the Manning roughness coeff i cients in different surface situations,such as eroded bare clay ground(n=0.02),natural pasture(n=0.13),cut grazing land(n=0.10),and bluegrass meadow(n=0.45).There are also many similar studies in which the slope roughness is assigned as only one definite value without consideration of the roughness spatial variability around the slope.

The neglect or simple processing of roughness causes deviations in research results.It is obvious that watersheds with only one surface type are rare and most catchments are covered with different forms of underlying surfaces of various proportions.In reality,the modern watershed surface,consisting of arti fi cial vegetation,large-scale farming,various engineering construction projects,and numerous hardened pavements,has been divided into broken patches,which destroy the spatial continuity of the watershed state parameters (vegetation,slope continuity,soil characteristics,etc.),leading to signi fi cant spatial variability of roughness along the catchment surface and affecting the overland flow depth and velocity distribution.Thus,simple processing of the spatial variability of roughness will lead to a substantial difference between reality and simulation.

Compared to open channel flow,it is clearer in overland flow that the boundary non-uniformity will inevitably cause the spatial variability of roughness.However,consideration of this variability in current hydrological models does not always lead to more accurate simulation results,and therefore,an effective method to express the spatial variability of roughness has not been found in theory or experimentally(Deng and Li, 2013).Thus,a breakthrough is required in the theoretical basis of roughness to improve future models.

4.3.Controversial opinions on roughness in different flow regimes

In the research of overland flow roughness,opinions vary with regard to the flow regime and its evaluation index.Some researchers assert that the slope is steep,so the overland flow with a high velocity is turbulent(Zhang,2002).However, others regard overland flow as laminar flow because it is a type of shallow flow and is distinctly affected by surface soil and vegetation.A group of investigators classify overland flow as cross-regime flow(Roels,1984;Chen et al.,2012).Haque (2002)supported the viewpoint that overland flow is laminar in general after applying the Darcy-Weisbach resistance formula,the Manning roughness coeff i cient formula,and the Chezy resistance formula to an elementary watershed paved by cement in Los Angeles.Roels(1984)studied the coarse surface resistance against overland flow.He considered overland flow to have three regimes,where a Reynolds number less than 100 corresponds to laminar flow,and an increase in the Reynolds number corresponds to turbulent or cross-regime flow.In the study of the relationships between hydraulic elements(surface runoff regime,head loss,water velocity, Froude number,Reynolds number,etc.),the results suggest that overland flow is laminar and the inertia effect is the main cause of head loss(Roche et al.,2007).Using field experiments,Chen et al.(2012)demonstrated that overland flow is laminar in natural woodlands,but changes to a transitional state between laminar and turbulent flows on slopes under conditions of accelerated erosion.Using water tanks with changing slopes,Zhang(2002)studied the transformation law of the flow regime,velocity,depth,and resistance coef fi cient of overland flow with the variations of water quantity and gradient.His experimental results showed that overland flow was mainly cross-regime or turbulent flow.

Because opinions vary on the issue of flow regime,no unanimous theoretical foundation has been agreed upon for the research of hydraulic elements( flow resistance,velocity, quantity,etc.)regarding the effect of roughness on overland flow.In this area,many studies have focused on open channel flow in water tanks with only one flow direction.However,the applicability of the conclusions obtained from these studies is dubious for multiple-direction flow.

5.Suggested solutions

5.1.Observing overland flow velocity field

Since the effect of roughness is homogenized in the process of flow concentration in a large-scale watershed,it is advisable to construct an outside experimental watershed or an indoor small catchment model in order to observe the flow velocity field.Small experimental catchments can be precisely divided into grid cells,with their discrete boundaries and joints of water channel systems located by GPS.To obtain all of the critical flow distribution data of grid cells and the joints synchronously and to clearly observe the velocity field distribution,water depth,and flow assignment in different runoff regimes,we can use several surface runoff measurement instruments,which can provide the flow volume at more than ten weir outlet channels.Then,the roughness values for different underlying surfaces and flow conditions can be calibrated,and thus,the roughness effect on overland flow can be analyzed. Using distributed hydrological models,we can obtain accurate flow simulations at watershed exits by adjusting parameters. However,the simulations can be biased or even incorrect in terms of the flow velocity field because of the inexact understanding of the roughness effect.In other words,distributed hydrological models have acceptable f i nal results,but the initial and middle flow concentrations are poorly simulated (Mu¨gler et al.,2011).Thus,it is of great signif i cance to observe the velocity field of overland flow.

5.2.Analyzing overland flow regime mechanism

In the process of overland flow concentration along the slope,the flow is distributed downstream and the water depth increases in the lower reaches.Simultaneously,the corresponding flow regime changes with the varying flow velocity and quantity.There is a close relationship between roughness and the flow regime because the roughness acts as a resistance mechanism and its measurement method changes under different regime conditions.Disagreement still exists over the def i nition and classif i cation of the flow regime.Because of this confusion,no unanimous theoretical basis has been determined to study the roughness effect,and the estimates resulting from various empirical formulas are quite different from one another(Dunkerley,2002).In many studies,the application of test results regarding open channel flow to overland flow is unreliable(Li et al.,2013).Therefore,it isnecessary to conduct overland flow simulation experiments, aiming to analyze the flow regime mechanism and its controlling factors based on the overland flow velocity field.In this way,we can explore the index system for flow regime classif i cation,discuss the hydrodynamic characteristics in different regimes,and analyze the surface roughness mechanisms in each flow regime.

5.3.Developing a basic roughness theory

The study of the roughness coeff i cient in overland flow originates from research on the pipe and open channel flow, where it is used to describe the flow resistance of a solid surface.However,in real overland flow,there is more than one flow direction,as proven by the application of the multiple flow direction arithmetic method in distributed hydrological models,which divide the watershed surface into multiple grid cells.When the flow is distributed from the original cell to adjacent downstream cells through different paths,the spatial variabilities of land usage type and vegetation distribution lead to different flow resistances,and the roughness values vary as a result.Even within the same cell,the roughness in different directions may vary,as opposed to that in pipe or open channel flow.Thus,surface roughness has unique features in overland flow,as it has spatial variability as well as directivity. Considering the large difference in the roughness mechanism between overland flow and other flows,it is essential to develop an independent basic theory for roughness in overland flow to consider its multiple directions.This development has been attempted,such as through the vector roughness theory (Zhang and Kang,2005),the variable roughness theory(Rai et al.,2010),and the effective roughness theory(Barros and Colello,2001).

5.4.Considering scale problems

Surface roughness theories are the results of field observations.Meanwhile,hydrological simulation using distributed hydrological models usually regards the whole catchment as the study area.The performances of the surface roughness theories at a catchment scale are uncertain,since a catchment of the size of 1 km2may be considered highly heterogeneous, whereas a basin of several thousand square kilometers may be considered homogeneous by an operational hydrological forecaster(Bergstrom and Graham,1998).Scale problems will occur when the micro-scale surface roughness studies are applied to catchment modeling.The flow distribution module of a catchment hydrological model can be modified by considering the surface roughness factor.The simulation results of the modified model may be eff i ciently improved by addressing specif i c weaknesses.The performance of the modified model can vary greatly depending on the hydrological conditions(f l oods or droughts).To take into account the roughnessvariability atthecatchmentscale willadd complexity to the model,but does not necessarily lead to improved performance of a hydrological model.Therefore, researchers should carefully examine the simulation results by comparing them to actual(i.e.,observed)responses of the catchment hydrological processes,and adjust the catchment hydrological model in order to reduce the impact of scale problems if necessary.

6.Conclusions

Overland flow is a type of shallow flow on slopes,signif icantly affected by surface soil types,vegetation,microtopography,etc.Thus,it is affected by more complex roughness factors compared with open channel flow.The current distributed hydrological models cannot always produce better simulation results than lumped hydrological models,because of their simple processing of roughness.In this paper,we divide current overland flow roughness study into three types:the first type of study considers the effects of roughness on the volume and velocity of surface runoff,f l ood peaks,and the scouring capability of flows,but has not addressed the spatial variability of roughness in detail;the second type of study concludes that surface roughness varies spatially with different land usage types,land-cover conditions,and different tillage forms,but the spatial variability has not been demonstrated quantitatively in these studies;and the third type of study simply deals with the spatial variability of roughness in each grid cell or land type. Three problems related to roughness in flow simulation processes of distributed hydrological models are discussed, including the neglect of roughness in distributed hydrological models,the lack of consideration of spatial variability of roughness in hydrological models,and the failure to distinguish the roughness formulas in different overland flow regimes. Moreover,we suggest four possible solutions to the roughness problem for studies of overland flow:observing the overland flow velocity field,analyzing the overland flow regime mechanism,developing a basic roughness theory,and considering scale problems.To increase the accuracy of distributed hydrological models in overland flow concentration simulation,we must encourage and support exploration of these four aspects.

Barros,A.P.,Colello,J.D.,2001.Surface roughness for shallow overland flow on crushed stone surface.J.Hydraul.Eng.127(1),38—52.http:// dx.doi.org/10.1061/(ASCE)0733-9429(2001)127:1(38).

Bergstrom,S.,Graham,L.P.,1998.On the scale problem in hydrological modelling.J.Hydrol.211(1),253—265.http://dx.doi.org/10.1016/S0022-1694(98)00248-0.

Candela,A.,Noto,L.V.,Aronica,G.,2006.Inf l uence of surface roughness in hydrological response of semiarid catchments.J.Hydrol.313(3—4), 119—131.http://dx.doi.org/10.1016/j.jhydrol.2005.01.023.

Cea,L.,Legout,C.,Darboux,F.,Esteves,M.,Nord,G.,2014.Experimental validation of a 2D overland flow model using high resolution water depth and velocity data.J.Hydrol.513,142—153.http://dx.doi.org/10.1016/ j.jhydrol.2014.03.052.

Chen,L.,Li,Z.B.,Li,P.,Xu,G.C.,Song,W.,2012.Hydraulic characteristics and flow energy consumption of accelerated erosion on steep slope woodland.Bull.Soil Water Conserv.32(4),5—9(in Chinese).

Darboux,F.,Davy,P.,Gascuel-Odoux,C.,Huang,C.,2002.Evolution of soil surface roughness and flowpath connectivity in overland flow experiments. CATENA 46(2—3),125—139.http://dx.doi.org/10.1016/S0341-8162(01) 00162-X.

Deng,P.,Li,Z.J.,2013.Comparison of three hydrological models in flood simulation for Xixian Basin of Huaihe River.J.Hohai Univ.(Nat.Sci.) 41(5),377—382.http://dx.doi.org/10.3876/j.issn.1000-1980.2013.05.001 (in Chinese).

Dunkerley,D.,2002.Volumetric displacement of flow depth by obstacles,and the determination of friction factors in shallow overland flows.Earth Surf. Process.Landf.27(2),165—175.http://dx.doi.org/10.1002/esp.309.

Engman,E.T.,1986.Roughness coef fi cients for routing surface runoff.J.Irrig. Drain.Eng.112(1),39—53.http://dx.doi.org/10.1061/(ASCE)0733-9437 (1986)112:1(39).

Haque,M.A.,2002.Study of surface runoff using physical models.Environ. Geol.41(7),797—805.http://dx.doi.org/10.1007/s00254-001-0455-1.

Helmers,M.J.,Eisenhauer,D.E.,2006.Overland flow modeling in a vegetative fi lter considering non-planar topography and spatial variability of soil hydraulic propertiesand vegetation density.J.Hydrol.328(1—2), 267—282.http://dx.doi.org/10.1016/j.jhydrol.2005.12.026.

Jin,C.X.,Romkens,M.J.M.,Grif fi oen,F.,2000.Estimating manning's roughness coef fi cient for shallow overland flow in non-submerged vegetative fi lter strips.Trans.ASAE 43(6),1459—1466.http://dx.doi.org/ 10.13031/2013.3044.

Laloy,E.,Bielders,C.L.,2008.Plot scale continuous modelling of runoff in a maize cropping system with dynamic soil surface properties.J.Hydrol. 349(3—4),455—469.http://dx.doi.org/10.1016/j.jhydrol.2007.11.033.

Lane,L.J.,Woolhiser,D.A.,1977.Simpli fi cations of watershed geometry affecting simulation of surface runoff.J.Hydrol.35(1—2),173—190. http://dx.doi.org/10.1016/0022-1694(77)90085-3.

Li,G.,Wang,X.,Zhao,X.,Huang,E.,Liu,X.,Cao,S.,2013.Flexible and rigid vegetation in overland flow resistance.Trans.ASABE 56(3), 919—926.http://dx.doi.org/10.13031/trans.56.9559.

Liu,J.T.,Chen,X.,Zhang,J.B.,Flury,M.,2009.Coupling the Xinanjiang model to a kinematic flow model based on digital drainage networks for flood forecasting.Hydrol.Process.23(9),1337—1348.http://dx.doi.org/ 10.1016/j.iswcr.2015.03.004.

Liu,J.T.,Chen,X.,Zhang,X.N.,Hoagland,K.D.,2012.Grid digital elevation model based algorithms for determination of hillslope width functions through flow distance transforms.Water Resour.Res.48(4),W04532. http://dx.doi.org/10.1029/2011WR011395.

Lumbroso,D.,Gaume,E.,2012.Reducing the uncertainty in indirect estimates of extreme fl ash flood discharges.J.Hydrol.414—415,16—30. http://dx.doi.org/10.1016/j.jhydrol.2011.08.048.

McDonnell,J.J.,Beven,K.,2014.Debates—The future of hydrological sciences:A(common)path forward?A call to action aimed at understanding velocities,celerities,and residence time distributions of the headwater hydrograph.Water Resour.Res.50,5342—5350.http://dx.doi.org/10.1002/ 2013WR015141.

Medeiros,S.C.,Hagen,S.C.,Weishampel,J.F.,2012.Comparison of floodplain surface roughness parameters derived from land cover data and field measurements.J.Hydrol.452—453(7),139—149.http://dx.doi.org/ 10.1016/j.jhydrol.2012.05.043.

Mu¨gler,C.,Planchon,O.,Patin,J.,Weill,S.,Silvera,N.,Richard,P.,Mouche,E., 2011.Comparison of roughness models to simulate overland flow and tracer transport experiments under simulated rainfall at plot scale.J.Hydrol. 402(1—2),25—40.http://dx.doi.org/10.1016/j.jhydrol.2011.02.032.

Noarayanan,L.,Murali,K.,Sundar,V.,2012.Manning's‘n’co-ef fi cient for fl exible emergent vegetation in tandem con fi guration.J.Hydro-Environ. Res.6(1),51—62.http://dx.doi.org/10.1016/j.jher.2011.05.002.

Podmore,T.H.,Huggins,L.F.,1980.Surface roughness effects on overland flow.Trans.ASAE 23(6),1434—1439.http://dx.doi.org/10.13031/2013. 34794.

Rai,R.K.,Upadhyay,A.,Singh,V.P.,2010.Effect of variable roughness on runoff.J.Hydrol.382(1—2),115—127.http://dx.doi.org/10.1016/j.jhydrol. 2009.12.022.

Remo,J.W.F.,Pinter,N.,2007.Retro-modeling the middle Mississippi River. J.Hydrol.337(3—4),421—435.http://dx.doi.org/10.1016/j.jhydrol.2007. 02.008.

Roche,N.,Daïan,J.F.,Lawrence,D.S.L.,2007.Hydraulic modeling of runoff over a rough surface under partial inundation.Water Resour.Res.43(8), W08410.http://dx.doi.org/10.1029/2006wr005484.

Roels,J.M.,1984.Flow resistance in concentrated overland flow on rough slope surfaces.Earth Surf.Process.Landf.9(6),541—551.http:// dx.doi.org/10.1002/esp.3290090608.

Sahoo,G.B.,Ray,C.,De Carlo,E.H.,2006.Calibration and validation of a physically distributed hydrological model,MIKE SHE,to predict streamflow at high frequency in a fl ashy mountainous Hawaii stream.J.Hydrol. 327(1—2),94—109.http://dx.doi.org/10.1016/j.jhydrol.2005.11.012.

Saxena,M.,Perumal,M.,2014.Appraisal of overland flow modeling using HEC-HMS and a variable parameter Muskingum method.ISH J.Hydraul. Eng.20(1),102—110.http://dx.doi.org/10.1080/09715010.2013.848607.

Schumann,G.,Matgen,P.,Hoffmann,L.,Hostache,R.,Pappenberger,F., Pf i ster,L.,2007.Deriving distributed roughness values from satellite radar data for f l ood inundation modeling.J.Hydrol.344(1—2),96—111.http:// dx.doi.org/10.1016/j.jhydrol.2007.06.024.

Shen,B.,Li,H.E.,Shen,J.,1994.Experimental studies of effective roughness in rainfall-overland flow processes.J.Hydraul.Eng.25(10),61—68(in Chinese).

Shit,P.K.,Maiti,R.,2012.Rill hydraulics:An experimental study on Gully Basin in lateritic upland of Paschim Medinipur,West Bengal,India.J. Geogr.Geol.4(4),1—11.http://dx.doi.org/10.5539/jgg.v4n4p1.

Smith,M.W.,Cox,N.J.,Bracken,L.J.,2007.Applying flow resistance equations to overland flows.Prog.Phys.Geogr.31(4),363—387.http:// dx.doi.org/10.1177/0309133307081289.

Stoof,C.R.,Ferreira,A.J.D.,Mol,W.,Van den Berg,J.,De Kort,A., Drooger,S.,Slingerland,E.,Mansholt,A.U.,Ferreira,C.S.S., Ritsema,C.J.,2015.Soil surface changes increase runoff and erosion risk after a low-moderate severity f i re.Geoderma 239—240,58—67.http:// dx.doi.org/10.1016/j.geoderma.2014.09.020.

Straatsma,M.W.,Baptist,M.J.,2008.Floodplain roughness parameterization using airborne laser scanning and spectral remote sensing.Remote Sens. Environ.112(3),1062—1080.http://dx.doi.org/10.1016/j.rse.2007.07.012.

Tarboton,D.G.,1997.A new method for the determination of flow directions and upslope areas in grid digital elevation models.Water Resour.Res. 33(2),309—319.http://dx.doi.org/10.1029/96WR03137.

Torri,D.,Poesen,J.,Borselli,L.,Bryan,R.,Rossi,M.,2012.Spatial variation of bed roughness in eroding rills and gullies.CATENA 90(3),76—86. http://dx.doi.org/10.1016/j.catena.2011.10.004.

Wang,M.H.,Hjelmfelt,A.T.,1998.DEM based overland flow routing model. J.Hydraul.Eng.3(1),1—8.http://dx.doi.org/10.1061/(ASCE)1084-0699(1998)3:1(1).

Wu,Y.,Christensen,K.T.,2007.Turbulence modif i cations in the roughness sublayer of flow over a highly-irregular surface topology.In:Collection of Technical Papers of 37th AIAA Fluid Dynamics Conference,American Institute of Aeronautics and Astronautics Inc.,Reston,pp.630—648.

Zhang,G.H.,2002.Study on hydraulic properties of shallow flow.Adv.Water Sci.13(2),159—165(in Chinese).

Zhang,S.T.,Kang,S.Z.,2005.Grid cell runoff distribution model based on vector roughness.J.Hydraul.Eng.36(11),1326—1330(in Chinese).

Received 10 May 2015;accepted 2 December 2015

This work was supported by the National Natural Science Foundation of China(Grants No.41471025 and 40971021)and the Natural Science Foundation of Shandong Province(Grant No.ZR2014DM004).

*Corresponding author.

E-mail address:zst0077@163.com(Sheng-tang Zhang).

Peer review under responsibility of Hohai University.

http://dx.doi.org/10.1016/j.wse.2016.07.001

1674-2370/©2016 Hohai University.Production and hosting by Elsevier B.V.This is an open access article under the CC BY-NC-ND license(http:// creativecommons.org/licenses/by-nc-nd/4.0/).

©2016 Hohai University.Production and hosting by Elsevier B.V.This is an open access article under the CC BY-NC-ND license(http:// creativecommons.org/licenses/by-nc-nd/4.0/).