参数约束系统的度量次正则性
张彬彬,王传坚,靳巧花
(1.昆明理工大学理学院,云南 昆明 650500;2.云南大学旅游文化学院信息科学与技术系,云南 丽江 674100.)
参数约束系统的度量次正则性
张彬彬1,王传坚1,靳巧花2
(1.昆明理工大学理学院,云南 昆明 650500;2.云南大学旅游文化学院信息科学与技术系,云南 丽江 674100.)
运用变分法和Clarke法锥,得到非凸参数约束系统具有度量次正则性的一个充分条件,并给出其在非线性参数约束系统中的一个应用.
严格可微;Clarke 法锥;度量次正则性;参数约束系统
在数学规划和向量优化问题的误差分析中,许多问题可归结为形如F(x)∶={y∈Y|ɡ(x,y)∈Θ},x∈X的集值映射的度量正则性或次正则性,其中ɡ:X×Y→Z是单值映射,Θ⊂Z为闭子集.我们称此集值映射为参数约束系统[1-4].集值映射的度量次正则性在数学规划、误差界的分析、弱极化最优解等问题中起着非常重要的作用,近年来受到许多学者的广泛关注.[5-9]目前得到的许多结论是在闭凸多值映射的假设下完成的,但事实上,诸多应用问题和理论问题需要我们考虑非凸多值映射,最近许多学者在这方面也做了深入的研究.[3-4,8,10]
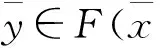
1 预备知识
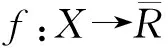
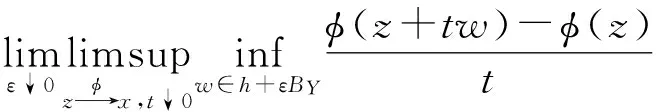
∂cφ(x)∶={x*∈X*|〈x*,h〉≤φ↑(x,h),∀h∈X}.
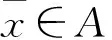
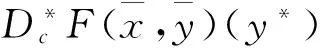
2 主要结论及证明

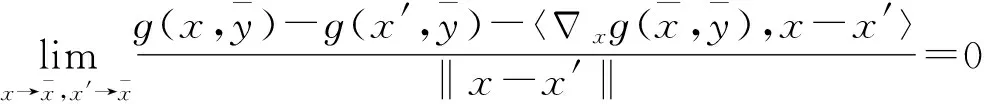
F(x)∶={y∈Y|ɡ(x,y)∈Θ},∀x∈X.
(1)
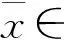
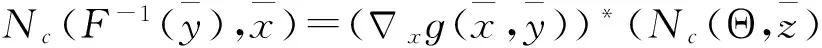
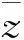
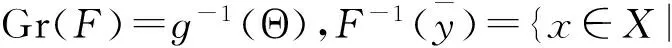

‖ɡ(x,y)-ɡ(u,v)‖≤M(‖x-u‖+‖y-v‖),
(2)
(3)
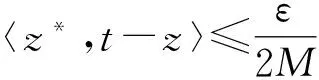
(4)
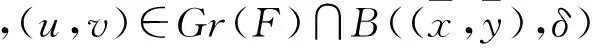

(5)
根据(3)及(5)式
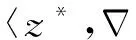
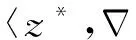

(ε/2)(‖x-u‖+‖y-v‖)+(ε/2)(‖x-u‖+‖y-v‖)=ε(‖x-u‖+‖y-v‖).
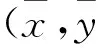
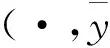
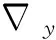
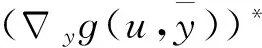
任取(u′,v′)∈X×Y,可得
〈(x*,y*),(u′,v′)〉=〈x*,u′〉+〈y*,v′〉=
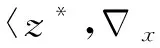
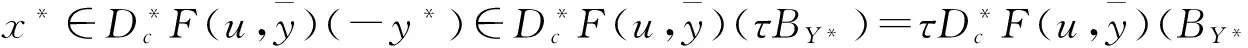
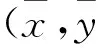
在优化中许多问题可归结为非线性参数约束系统的度量次正则性,其中非线性参数约束系统形如
F(x)={y∈Y|φi(x,y)≤0,ψj(x,y)=0,i=1,…,m;j=1,…,r}.
(6)
其中φi,ψj:X×Y→R为实值函数[2].我们给出该系统具有度量次正则性的一个充分条件.
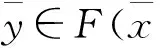
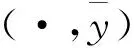
Θ∶={(α1,…,αm,β1,…,βr)|αi≤0,βj=0,i=1,…,m;j=1,…,r},
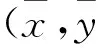
[1] HENRION R,OUTRATA J.Calmness of constraint systems with applications [J].J Math Program,2005,104: 437-464.
[2] MORDUKHOVICH B S.Variational analysis and generalized differentiation Ⅰ,Ⅱ: basic theory [M].New York: Springer-verlag,2006: 23-168.
[3] HELMUT G.First order and second order characterization of metric subregularity and calmness of constraint set mappings [J].SIAM J Optim,2011,4: 1439-1474.
[4] HE Q H,YANG J,ZHANG B B.Metric subregularity for subsmooth generalized constraint equations in Banach spaces [J/OL].J Applied Mathematics,2012[2014-04-02].http://www.hindawi.com/journals/jam/2012/185249.
[5] CLARKE F H.Optimization and nonsmooth analysis [M].New York: Wiley,1983: 46-102.
[6] ZHENG X Y,YANG X Q.Weak sharp minima for semi-infinite optimization problems with applications [J].SIAM J Optim,2007,18: 573-588.
[7] ZHENG X Y,NG K F. Linear regularity for a collection of subsmooth sets in banach spaces [J].SIAM J Optim,2008,19:62-76.
[8] ZHENG X Y,NG K F.Calmness for L-subsmooth multifunctions in banach spaces [J].SIAM J Optim,2009,4: 1648-1673.
[9] 程亚焕,段丽芬,左明霞.赋广义 Orlicz 范数 Orlicz 函数空间的完全k-凸性 [J].东北师大学报(自然科学版),2015,47(2):5-8.
[10] 王慧勤,雷 刚.预条件下含参数的 JOR 迭代法敛散性分析 [J].东北师大学报(自然科学版),2015,47(1):43-47.
(责任编辑:李亚军)
The metric subregularity of parametric constraint systems
ZHANG Bin-bin1,WANG Chuan-jian1,JIN Qiao-hua2
(1.School of Science,Kunming University of Science and Technology,Kunming 650500,China;2.Department of Information Science and Technology,Tourism and Culture College of Yunnan University,Lijiang 674100,China)
Using variational technique,a sufficient condition via Clarke normal cones for metric subregularity of parametric constraint systems is provided.Consequently,a sufficient condition is given for parametric constraint systems of nonlinear programming.
strict differentiability; Clarke normal cone; metrically subregular; parametric constraint systems
1000-1832(2015)04-0046-03
10.16163/j.cnki.22-1123/n.2015.04.010
2014-04-02
国家自然科学基金资助项目(11261024).
张彬彬(1987—),男,硕士,讲师,主要从事非光滑优化理论及应用研究.
O 177.92 [学科代码] 110·57
A

