角动量算符与轨道波函数

王建伟　齐海啸

(喀什大学 物理与电气工程学院， 新疆 喀什　844008)



角动量算符与轨道波函数

王建伟齐海啸

(喀什大学 物理与电气工程学院， 新疆 喀什844008)

摘要稀土元素、锕系元素的价电子都处于f态,研究价电子轨道角动量问题是量子力学中重要的课题.在量子力学教科书中只给出了氢原子span算符共同本征矢的递推公式，没有计算出span的本征矢;只给出了氢原子的s、p和d电子span算符共同本征矢，没有给出f电子span算符共同本征矢.本文运用表象理论和氢原子span本征矢的递推公式不仅求出了氢原子f电子span算符的共同本征矢，而且还计算出氢原子f电子span的本征矢，并讨论了它们的有关性质.这对于全面了解氢原子电子轨道运动的细节是有好处的，完善了教科书中的不足.

关键词轨道角动量；本征矢；本征值；表象理论

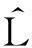
1理论与方法

1.1　表象理论及Q表象

1) 表象理论

态和力学量的具体表示称为表象.在表象理论中，主要解决态和力学量算符的矩阵表示以及不同表象间的相互转换[5].

2)Q表象

1.2　波函数的矩阵表示

(1)

1.3　力学量算符的矩阵表示

(2)

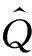
由此得到一个结论： 算符在其自身表象中是一个对角矩阵，对角元素为其本征值.

1.4　氢原子轨道角动量算符及本征函数的递推公式

(3)

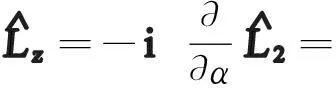
(4)

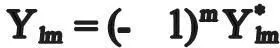
(5)

2.1　氢原子f电子轨道角动量算符的本征值及其本征函数

Y3,3;Y3,2;Y3,1;Y3,0;Y3,-1;Y3,-2;Y3,-3.

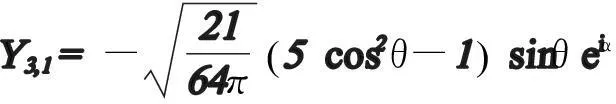
2.2　氢原子f电子轨道角动量算符的本征矢在Lz表象中的表示

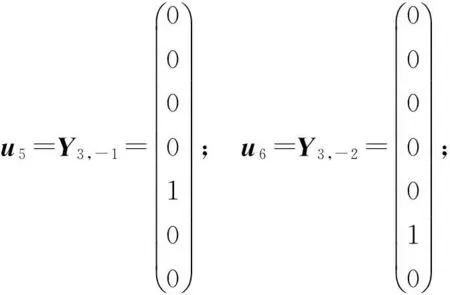
2.3　氢原子f电子轨道角动量算符在Lz表象中的矩阵表示

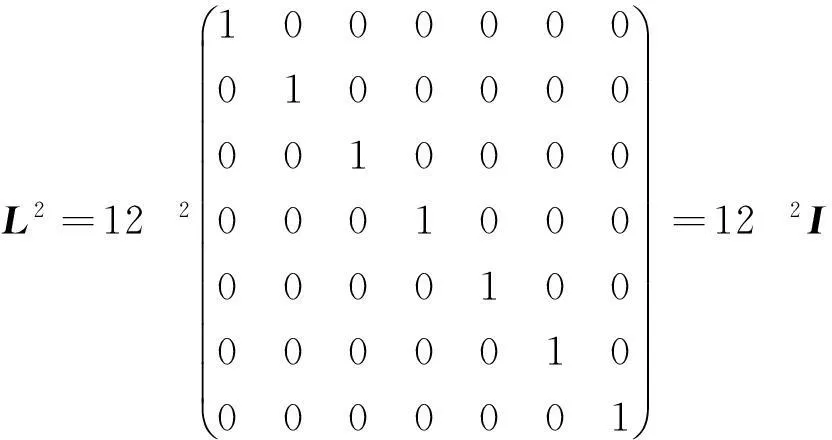
(I是一个7阶单位矩阵)

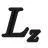
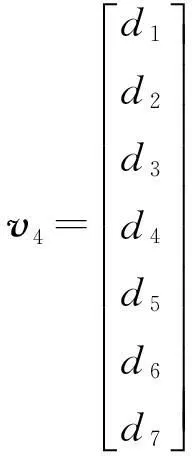
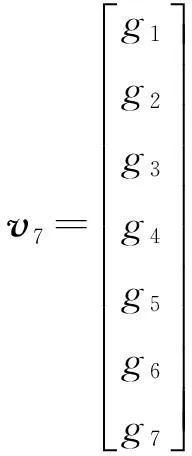
可以得到：

Ly=

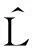
可以得到

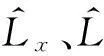
在V1态测量Lx，可以得到Lx=3，相应的概率w=1.

其相应的概率为

4结语

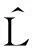
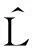
参考文献

[1]周世勋.量子力学教程[M].2版.北京：高等教育出版社，2009.52-58.

[2]曾谨言.量子力学： 卷I[M].北京： 科学出版社，2000:167-169.

[3]赵凯华，罗蔚茵.量子物理[M].北京： 高等教育出版社，2001： 33-38.

[4]钱伯初. 量子力学[M].北京： 高等教育出版社.2006：157-162.

[5]周世勋.量子力学教程[M].2版.北京： 高等教育出版社,2009:93-106.

[6]倪光炯，陈苏卿.高等量子力学[M].上海： 复旦大学出版社,2004:11-13.

[7]苏汝铿.量子力学[M].2版. 上海： 复旦大学出版社,2002:104-109.

■

物理实验

THE ORBITAL ANGULAR MOMENTUM OPERATOR AND ORBITAL WAVE FUNCTION

Wang JianweiQi Haixiao

(Institute of Physics and Electrical Engineering, University of Kashgar, Kashgar, Xinjiang 844008)

AbstractValence electrons of both rare earth elements and actinides are in the f state. So study of orbital angular momentum problem of valence electron is an important topic in quantum mechanics. In quantum mechanics textbooks, only the recursive figure of common eigenvectors for2andzoperators of hydrogen atom is given, without the calculation of eigenvectors forxandy. The given common eigenvectors for2andzoperators only corresponds to the electrons on s, p and d orbitals of the hydrogen atom, not including the f orbital. In this paper, using representation theory and the recursive figure of common eigenvectors for2andzoperators, we not only calculated the common eigenvectors for2andzoperators of f electrons in hydrogen atom, but also gave the eigenvectors forxandyoperators of f electrons. Relevant properties of these eigenvectors are discussed. This is good for a comprehensive understanding of the details of the atomic orbital motion of the hydrogen atom, and an improvement of the deficiencies in the textbook.

Key wordsorbit angle momentum; eigenvector; eigenvalue; theory of representation

作者简介:2015-05-11 王建伟，男，教授，主要从事量子力学与物理课程教学论的教学与研究工作.Wangjianwei-ks@163.com