Empirical model of correction for zenith tropospheric delay

V F Kravchenko, V I Lutsenko, I V Lutsenko, D O Popov, A G Laush, V N Gudkov

(1.Kotel’nikov Institute of Radio Engineering and Electronics, Russian Academy of Sciences, Moscow 125009, Russia; 2.Usikov Institute of Radiophysics and Electronics of National Academy of Sciences of Ukraine, Kharkov 61085, Ukraine; 3.Co.Ltd.Navis-Ukraine, Smela 20708, Ukraine)

Empiricalmodelofcorrectionforzenithtroposphericdelay

V F Kravchenko1, V I Lutsenko2, I V Lutsenko2, D O Popov2, A G Laush3, V N Gudkov3

(1.Kotel’nikovInstituteofRadioEngineeringandElectronics,RussianAcademyofSciences,Moscow125009,Russia; 2.UsikovInstituteofRadiophysicsandElectronicsofNationalAcademyofSciencesofUkraine,Kharkov61085,Ukraine; 3.Co.Ltd.Navis-Ukraine,Smela20708,Ukraine)

Abstract：The paper considers the possibility of correction of zenith tropospheric delays, and calculates it with the standard model, which takes into account the values of the refractive index of the troposphere at the time of measurement.Based on the experimental research, this empirical model of correction for zenith tropospheric delays can reduce the measurement error of coordinates to about 30% and altitude to about 40%.

Key words：zenith tropospheric delays; satellite navigation signal; global positioning system (GPS); global navigation satellite system (GLONASS)

CLDnumber： TN911.7Documentcode： A

0 Introduction

Now there are a large number of high-precision receivers of satellite navigation signals of different systems that are able to make measurements with millimeter precision.However, for the dominant factor which makes significant errors in the positioning of objects with high accuracy, there are spatio-temporal unevenness distribution of electromagnetic waves in the atmosphere, particularly in the troposphere.

Tropospheric delay value depends on temperature, humidity, pressure and position of the satellite relative to the receiving point, but does not depend on frequency of the received signal.Such errors can be ruled out by modeling or by incorporating it into unknown parameters when solving the navigation problem.It is of interest to develop techniques and algorithms to reduce influence of the spread conditions for most of the abundant and cheap single-frequency receivers.There are several mathematical models that describe parameters of the troposphere and according to which are performed calculations of the amendments, for example Saastamoinen model[1], Hopfield[2], Black, minimum operational performance standards (MOPS)[3-4], etc.The main drawback of these models is that they are based on the global distribution of meteorological parameters and does not take into account their regional variations.Aim of this work is to improve accuracy of coordinate determination by taking into account the influence of meteorological parameters of the troposphere in regional area of the object.This is performed by introducing an empirical correction coefficient to the existing and used navigation solution evaluation model of zenith tropospheric delay.It is necessary to establish regression relationship between this coefficient and refractive index value of the troposphere and conduct impact assessment of these corrections on the positioning accuracy.

1 Experimental results and discussion

The experimental data are obtained with specially deployed measurement systems receiving global positioning system (GPS) and global navigation satellite system (GLONASS) signals in four cities at distances from 140 km to 400 km.Navigation receiver CH-4719 based on the complex is developed and produced by Co.Ltd.Navis-Ukraine (Smela).The antenna system of receiver allows receiving signals from navigation satellites, starting with zero or even small negative angles of satellite's elevation.Equipment for the reception of GPS and GLONASS signals, as well as the software interface used for measuring points are shown in Figs.1 and 2.

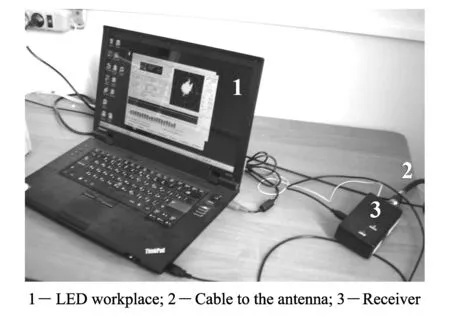
Fig.1 Exterior view of the complex for receiving GPS and GLONASS signals

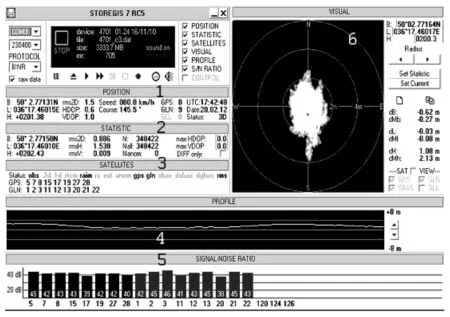
1-Live results of coordinate measuring; 2-True coordinates; 3-Visible satellites; 4-Altitude; 5-Signal-noise ratio for satellites; 6-Changes in latitude and longitude for the experimentFig.2 Example of the measurement system interface

The software provides registration of “raw” data from the signals received from GNSS and GLONASS, thus special software for this purpose was developed.By the introduction of coefficient in dialog mode, the calculated zenith tropospheric delaysRTis corrected by

(1)

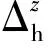
(2)

whereg=9.806 65 m/s2;His the receiver height above mean sea level (m);Tis the average temperature at sea level (K);kis the vertical temperature gradient (K/m);Rd=287.054 (J/(kg·K));λis the vertical gradient of water vapor density (dimensionless);zhis the zenith hydrostatic component of the delay at mean sea level andzwis the zenith “wet” component of the delay at mean sea level, wherezhandzware calculated by

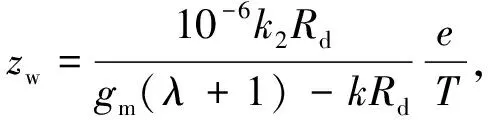
(3)

wherek1=77.604 K/mbar;k2=382 000 K2/mbar;Pis the pressure at mean sea level (mbar);gm=9.784 m/s2;eis the water vapor pressure at mean sea level (mbar).

Meteorological parameters used in the model (atmospheric pressure, temperature and partial vapor pressure at sea level and their vertical gradients) are determined based on table data[6](see Table 1) by

whereφis the receiver latitude;Dis the day of the year (from 1 January);Dmin=28 for northern latitudes,Dmin=211 for southern latitudes;ξ0and Δξare the mean value and seasonal variations in weather parameters, respectively.

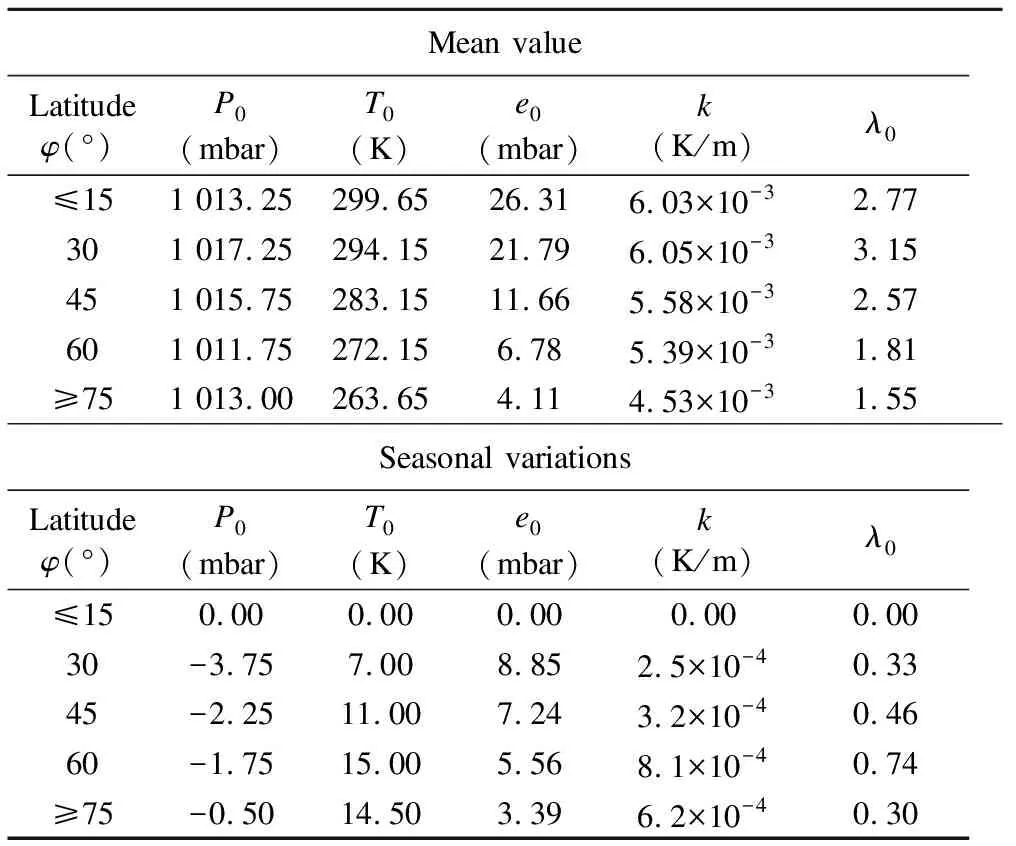
Table 1 Model parameters[6]

The measured data have been selected in the processing for several days in winter, when the refractive index change during the day.In most cases they are insignificant in days of spring and summer when the refractive index differences reach 55 N units per day.

Fig.3 provides an example which shows how to change coordinates of the target center when we enter correction coefficients for zenith tropospheric delay in the morning (see Figs.3(a),(c) and (e)), and for the evening (see Figs.3(b),(d) and (f)) in winter.Statistical processing results of program Storegis[6-7], developed by Co.Ltd.Navis-Ukraine, are shown on the right side of the target.It determines average value of the coordinates (latitude-B, longitude-L, altitude-H), their deviations from true value deltaMb, deltaMl and deltaMh (deviations of mathematical expectation from the sampling coordinates of reference point of latitude, longitude and altitude) and standard deviations rms2D, rmsH and rmsV (coordinates, altitude and velocity) obtained byNobservations.

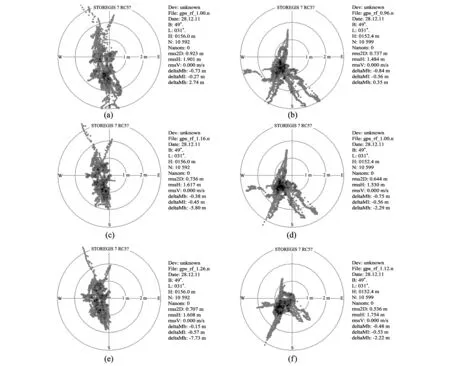
Fig.3 Results using the algorithm of introducing amendments to zenith tropospheric refraction for Smela: 28.12.2011 from 07:44:36 to 10:53:38, where a-α=1, c-α=1.16, e-α=1.26; from 14:03:49 to 17:13:47, where b-α=0.84, d-α=1.0, f-α=1.12

It can be seen that the mioimum spread calculates coordinates of the target relative to the center occurs when the values of input amendment to zenith tropospheric delay exceeds the theosetically calculated values, that is whenKres=α≥1.At the same time, a reduction of the mean square error in determining the coordinates in some cases may cause the systematic error of their determination.The dependence of error in determining coordinates of the input correction coefficient was investigated.

Fig.4 shows the root mean square (rms) error in determining the altitude (see Fig.4(a)) and coordinates (see Fig.4(b)).They are obtained by introducing different values of the coefficients (corrective zenith tropospheric delayKres=α).It is seen that, for both the altitude and the coordinates, as well as the optimum correction coefficients for zenith tropospheric delay, the minimum rms error differs from the theoretical assessmentKres=α=1.In most cases, they exceed it, that isKres=α≥1.

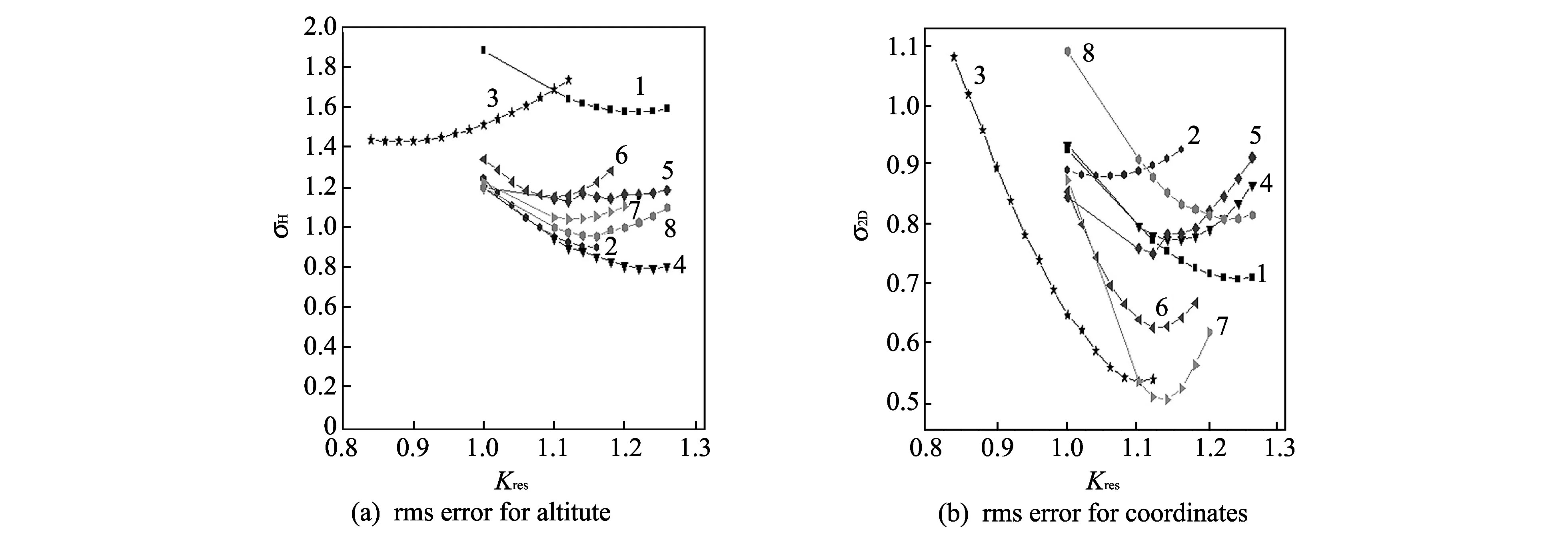
1-28.12.2011 from 07:44:36 to 10:53:38; 2-28.12.2011 from 10:53:38 to 14:03:48; 3-28.12.2011 from 14:03:49 to 17:13:47; 4-28.12.2011 from 17:13:48 to 20:23:52;5-28.12.2011 from 20:23:53 to 23:34:09; 6-28.12.2011 from 23:34:10 to 02:44:32; 7-29.12.2011 from 02:44:33 to 05:54:56; 8-29.12.2011 from 05:54:57 to 09:04:57Fig.4 Dependence of the rms error in determining the altitude (a) and the coordinates (b) from value of the corrective zenith tropospheric delay coefficient Kres

Table 2 shows the optimum values of correction coefficients for different measurement days, depending on the refractive index, during the measurement.For each of the measurement intervals about 10 000 observations are used.

In some cases, as seen from Table 2, values of the optimum correction coefficients which minimize the error in determining coordinates and altitude are mostly the same, and approximately the same for them as the averages values.

Using these data is performed by rejecting emission of the correction coefficients, which can be identified as a blunder.Thus dependence of the coordinate correction coefficients ane the altitude to tropospheric refraction coefficient are obtained and shown in Fig.5.In the second step, those coefficients are discarded and they are obtained for days during which the value of the refractive index and behavior are not characteristic for the season, for example, in the rejected days of winter, for which there is a strong diurnal variation in the refractive index due to the strong warming in the summer-the days when behavior of the refractive index is typical for the winter, especially that of a strong cold snap.

The obtained dependence of optimal correction coefficients from the refractive index is shown in Fig.5(c) for altitude, and Fig.5(d) for coordinates.The regression line is also plotted.Analysis shows that the optimal values of winter zenith tropospheric delay are substantially identical with the theoretical values in most cases.Offset from the optimal theoretical values does not exceed 5%-10% (αopt≈1.05-1.1).At the same time, the summer optimal delay value for the coordinates in the plane and the altitude are about 10%-25% greater than the theoretical values (αopt≈1.1-1.25).

Table2DependenceofoptimalvaluesofratiosKresforcorrectivetroposphericzenithdelayonrefractiveindexoftroposphereforSmela

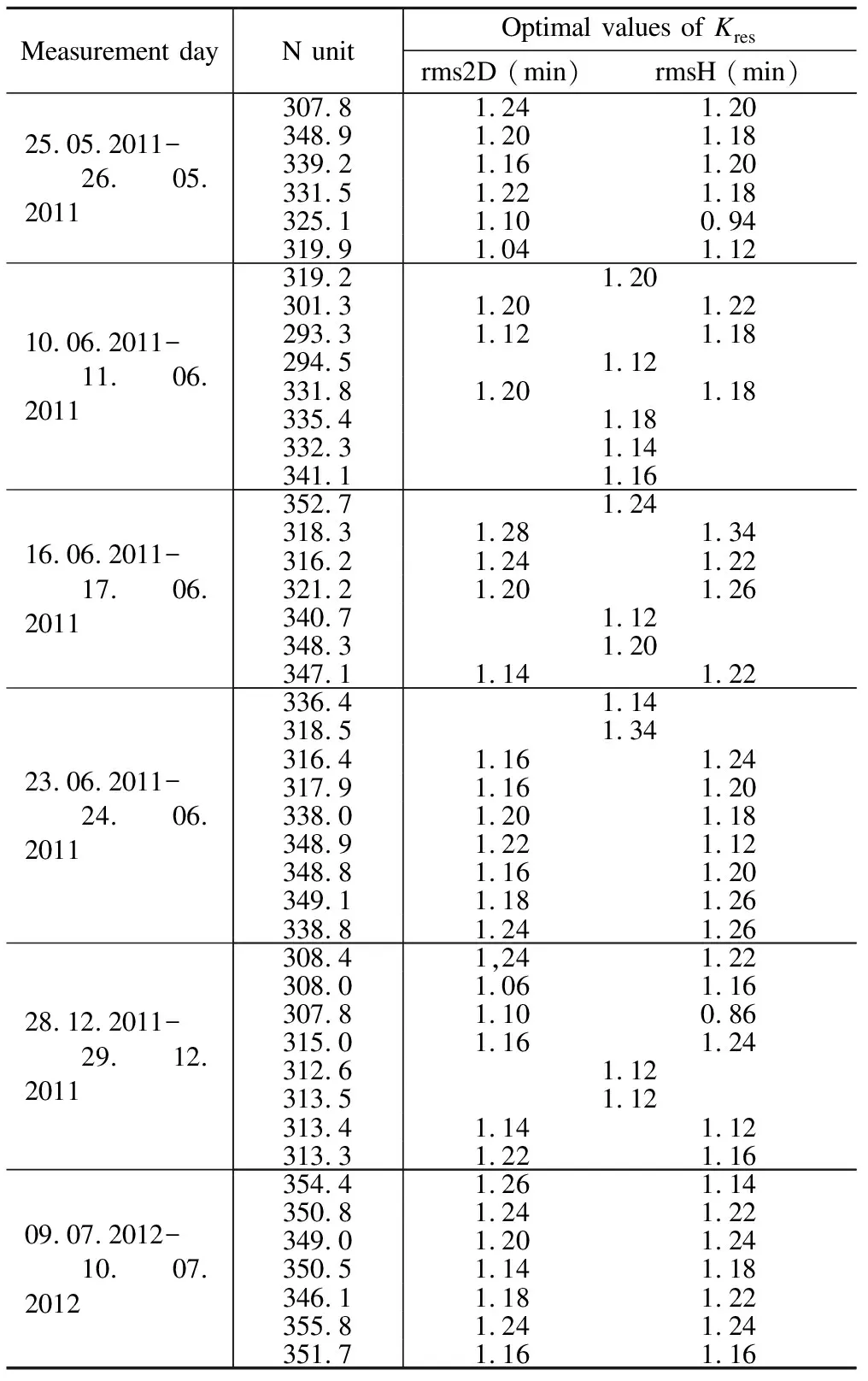
Measurement dayN unitOptimal values of Kresrms2D (min)rmsH (min)25.05.2011-26.05.2011307.81.241.20348.91.201.18339.21.161.20331.51.221.18325.11.100.94319.91.041.1210.06.2011-11.06.2011319.21.20301.31.201.22293.31.121.18294.51.12331.81.201.18335.41.18332.31.14341.11.1616.06.2011-17.06.2011352.71.24318.31.281.34316.21.241.22321.21.201.26340.71.12348.31.20347.11.141.2223.06.2011-24.06.2011336.41.14318.51.34316.41.161.24317.91.161.20338.01.201.18348.91.221.12348.81.161.20349.11.181.26338.81.241.2628.12.2011-29.12.2011308.41,241.22308.01.061.16307.81.100.86315.01.161.24312.61.12313.51.12313.41.141.12313.31.221.1609.07.2012-10.07.2012354.41.261.14350.81.241.22349.01.201.24350.51.141.18346.11.181.22355.81.241.24351.71.161.16

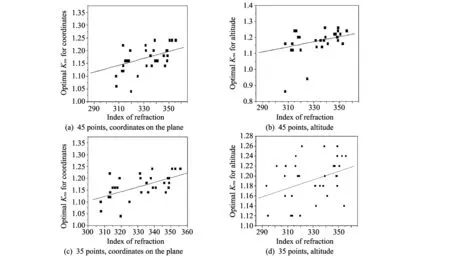
Fig.5 Dependence of corrective coefficients for zenith tropospheric delay on the refractive index of the troposphere

The meanings of regression coefficients in Eq.(5) for different sample sizesmare obtained from each of the processing steps shown in Table 3.The correlation coefficients of the positioning errors are also given with values of refractive indices of the troposphere.Regression expression for optimal estimate of the correction coefficient on the value of refractive indexNhas the form

α=Kres=A+B·N.

(5)

Practical calculations can be taken asBh≈1.4×10-3-2.0×10-3for coordinates and 2.0×10-3-2.5×10-3for altitude.The optimal correction can to reduce the rms measurement error of coordinates by 4%-5%, and altitude by 8%-9%.For the calculations of zenith tropospheric delay in the summer, it is necessary to increase the value, compared with the theoretical estimate of this parameter obtained by the MOPS model 15%, which will reduce the measurement error of the altitude as well as the coordinates.The obtained empirical Eq.(5) can be used.With the introduction of corrections to the zenith tropospheric delay, its daily dependence is taken into account through the daily changes in the refractive index.The proposed approach can give a more accurate positioning than introduction of the same during the day correction of zenith tropospheric delay.

Table3CoefficientsoftheequationforthelinearregressionoftheoptimaladjustmentfactorKreswitharefractiveindexofthetroposphere

CoordinatesAltitudeABRmABRm0.922 817.791 11×10-40.238 78450.964 976.408 11×10-40.139 21450.727 020.001 340.474 82350.651 840.001 580.358 67350.5122 90.001 970.594 68310.312 150.002 580.474 8431

2 Modeling of correction algorithm for zenith tropospheric delays

The resulting empirical Eq.(5) is used for the correction of zenith tropospheric delays, depending on refractive index values of the troposphere which takes place in the measurements.Processing method consists of decomposition of the daily measured data arrays at 3 h intervals.Each of the intervals from meteorological data (temperature, pressure and humidity) is estimated with refractive index valueNof the troposphere, and used in Eq.(5) to calculate the correction coefficientαfor the calculated value of zenith tropospheric delay.The resulting corrected values are used in solving the navigation task in determining coordinates.Each 3 h data segment is estimated rms error in determining the coordinates in the correction of the theoretical values of tropospheric zenith delay.It is compared with the rms error of the positioning when the correction is not introduced and the calculations uses value of the tropospheric zenith delay, calculated from the standard model.

The obtained values for the rms error of determining the coordinates and altitude are shown in Tables 4 and 5.Also the average values of errors resulting from the use of zenith tropospheric delay correction coefficient are given when they are used in the calculation of its theoretical value.

Table4Fordifferentweatherconditionsrmserrorindeterminingthecoordinatesusingaregressionmodelofcorrection,thezenithtroposphericdelayandthevaluesobtainedwiththeMOPSmodel

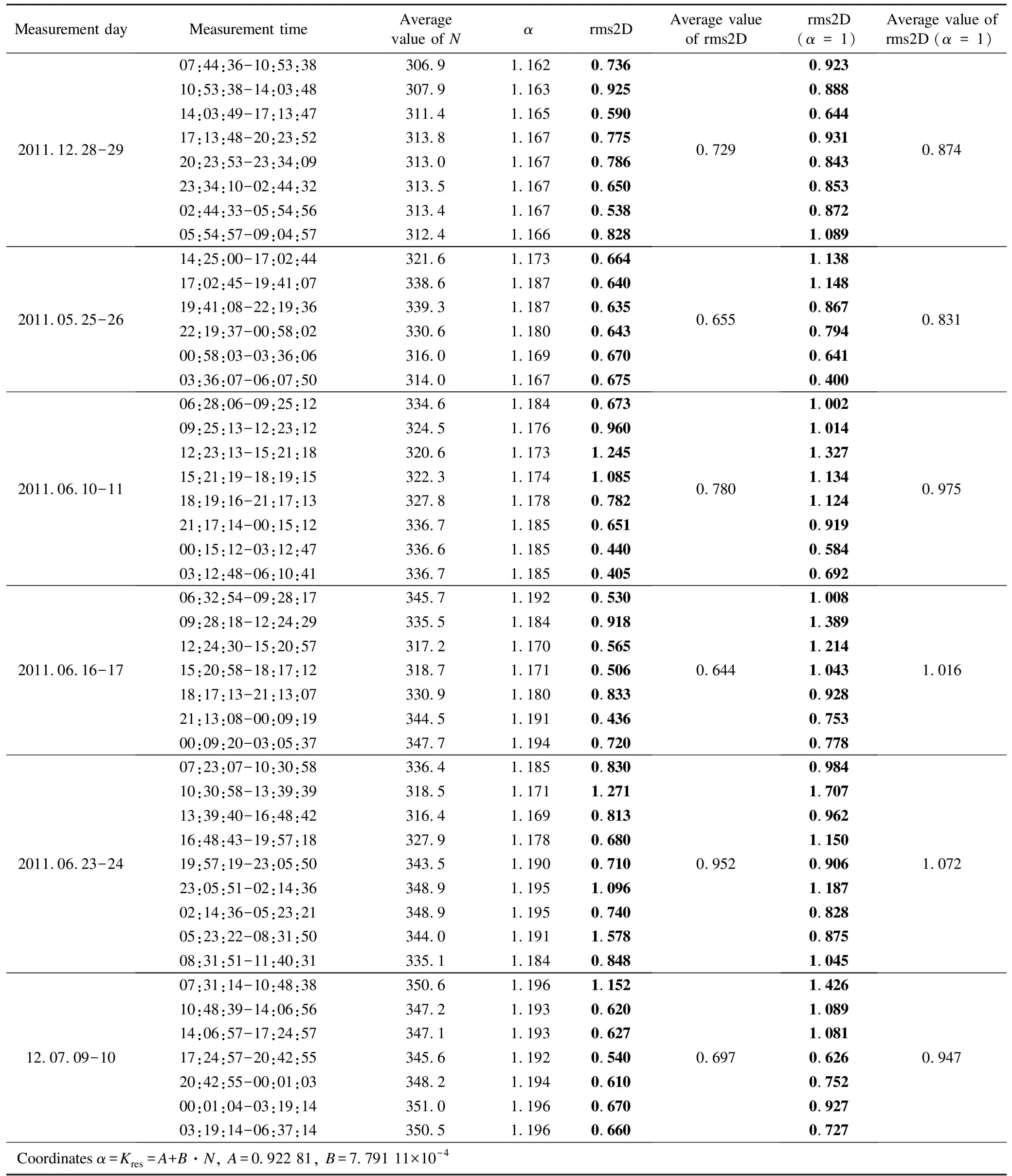
Measurement dayMeasurement timeAverage value of Nαrms2DAverage valueof rms2Drms2D(α=1)Average value ofrms2D (α=1)2011.12.28-2907:44:36-10:53:38306.91.1620.73610:53:38-14:03:48307.91.1630.92514:03:49-17:13:47311.41.1650.59017:13:48-20:23:52313.81.1670.77520:23:53-23:34:09313.01.1670.78623:34:10-02:44:32313.51.1670.65002:44:33-05:54:56313.41.1670.53805:54:57-09:04:57312.41.1660.8280.7290.9230.8880.6440.9310.8430.8530.8721.0890.8742011.05.25-2614:25:00-17:02:44321.61.1730.66417:02:45-19:41:07338.61.1870.64019:41:08-22:19:36339.31.1870.63522:19:37-00:58:02330.61.1800.64300:58:03-03:36:06316.01.1690.67003:36:07-06:07:50314.01.1670.6750.6551.1381.1480.8670.7940.6410.4000.8312011.06.10-1106:28:06-09:25:12334.61.1840.67309:25:13-12:23:12324.51.1760.96012:23:13-15:21:18320.61.1731.24515:21:19-18:19:15322.31.1741.08518:19:16-21:17:13327.81.1780.78221:17:14-00:15:12336.71.1850.65100:15:12-03:12:47336.61.1850.44003:12:48-06:10:41336.71.1850.4050.7801.0021.0141.3271.1341.1240.9190.5840.6920.9752011.06.16-1706:32:54-09:28:17345.71.1920.53009:28:18-12:24:29335.51.1840.91812:24:30-15:20:57317.21.1700.56515:20:58-18:17:12318.71.1710.50618:17:13-21:13:07330.91.1800.83321:13:08-00:09:19344.51.1910.43600:09:20-03:05:37347.71.1940.7200.6441.0081.3891.2141.0430.9280.7530.7781.0162011.06.23-2407:23:07-10:30:58336.41.1850.83010:30:58-13:39:39318.51.1711.27113:39:40-16:48:42316.41.1690.81316:48:43-19:57:18327.91.1780.68019:57:19-23:05:50343.51.1900.71023:05:51-02:14:36348.91.1951.09602:14:36-05:23:21348.91.1950.74005:23:22-08:31:50344.01.1911.57808:31:51-11:40:31335.11.1840.8480.9520.9841.7070.9621.1500.9061.1870.8280.8751.0451.07212.07.09-1007:31:14-10:48:38350.61.1961.15210:48:39-14:06:56347.21.1930.62014:06:57-17:24:57347.11.1930.62717:24:57-20:42:55345.61.1920.54020:42:55-00:01:03348.21.1940.61000:01:04-03:19:14351.01.1960.67003:19:14-06:37:14350.51.1960.6600.6971.4261.0891.0810.6260.7520.9270.7270.947Coordinates α=Kres=A+B·N, A=0.922 81, B=7.791 11×10-4

Table5Fordifferentweatherconditionsrmserrorindeterminingthealtitudeusingtheregressionmodelofcorrection,thezenithtroposphericdelayandthevaluesobtainedwiththeMOPSmodel

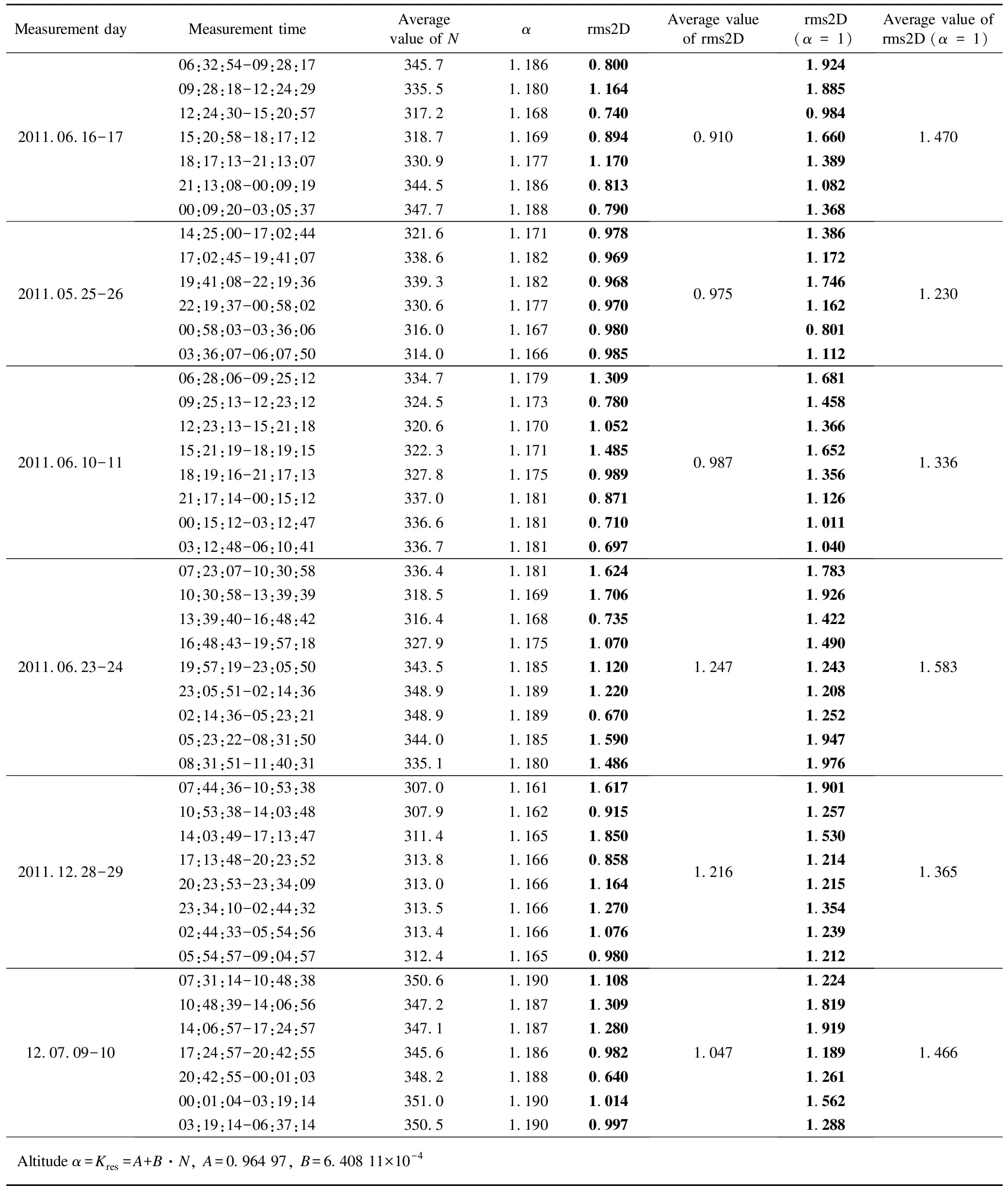
Measurement dayMeasurement timeAverage value of Nαrms2DAverage valueof rms2Drms2D(α=1)Average value ofrms2D (α=1)2011.06.16-1706:32:54-09:28:17345.71.1860.80009:28:18-12:24:29335.51.1801.16412:24:30-15:20:57317.21.1680.74015:20:58-18:17:12318.71.1690.89418:17:13-21:13:07330.91.1771.17021:13:08-00:09:19344.51.1860.81300:09:20-03:05:37347.71.1880.7900.9101.9241.8850.9841.6601.3891.0821.3681.4702011.05.25-2614:25:00-17:02:44321.61.1710.97817:02:45-19:41:07338.61.1820.96919:41:08-22:19:36339.31.1820.96822:19:37-00:58:02330.61.1770.97000:58:03-03:36:06316.01.1670.98003:36:07-06:07:50314.01.1660.9850.9751.3861.1721.7461.1620.8011.1121.2302011.06.10-1106:28:06-09:25:12334.71.1791.30909:25:13-12:23:12324.51.1730.78012:23:13-15:21:18320.61.1701.05215:21:19-18:19:15322.31.1711.48518:19:16-21:17:13327.81.1750.98921:17:14-00:15:12337.01.1810.87100:15:12-03:12:47336.61.1810.71003:12:48-06:10:41336.71.1810.6970.9871.6811.4581.3661.6521.3561.1261.0111.0401.3362011.06.23-2407:23:07-10:30:58336.41.1811.62410:30:58-13:39:39318.51.1691.70613:39:40-16:48:42316.41.1680.73516:48:43-19:57:18327.91.1751.07019:57:19-23:05:50343.51.1851.12023:05:51-02:14:36348.91.1891.22002:14:36-05:23:21348.91.1890.67005:23:22-08:31:50344.01.1851.59008:31:51-11:40:31335.11.1801.4861.2471.7831.9261.4221.4901.2431.2081.2521.9471.9761.5832011.12.28-2907:44:36-10:53:38307.01.1611.61710:53:38-14:03:48307.91.1620.91514:03:49-17:13:47311.41.1651.85017:13:48-20:23:52313.81.1660.85820:23:53-23:34:09313.01.1661.16423:34:10-02:44:32313.51.1661.27002:44:33-05:54:56313.41.1661.07605:54:57-09:04:57312.41.1650.9801.2161.9011.2571.5301.2141.2151.3541.2391.2121.36512.07.09-1007:31:14-10:48:38350.61.1901.10810:48:39-14:06:56347.21.1871.30914:06:57-17:24:57347.11.1871.28017:24:57-20:42:55345.61.1860.98220:42:55-00:01:03348.21.1880.64000:01:04-03:19:14351.01.1901.01403:19:14-06:37:14350.51.1900.9971.0471.2241.8191.9191.1891.2611.5621.2881.466Altitude α=Kres=A+B·N, A=0.964 97, B=6.408 11×10-4

It should be noted that the calculation of correction coefficient is taken from regression equation coefficients derived for the entire set of data, without rejecting gross errors.It is understood that the results obtained are rated below.Actually somewhat greater gains will be obtained in reducing measurement errors.It can be seen that, using correction factors for the calculated values of zenith tropospheric delay calculated from data on the refractive indices of the troposphere, reducing the rms error in determining the coordinates are about 20%-30%, and altitude is to 30%-40%.

Fig.6 shows the dependence of average (per day) rms error in determining the coordinates (see Fig.6(a)) and altitude (see Fig.6(b)).It can be seen that the introduction of correction calculated by the zenith tropospheric delay models that take into account the refractive index value at the time of measurement, allows to reduce the rms error of the coordinates and altitude.

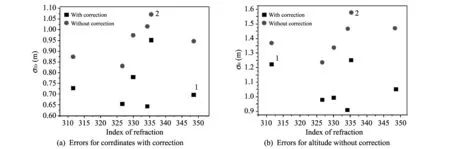
Fig.6 Errors in determining coordinates and altitude with correction of tropospheric zenith delay nd without correction

The parameters of linear regression dependence of mean errors of coordinates and altitude of the mean value per day of the refractive index are shown in Table 6.

Table6Parametersofregressiondependenciesoferrorsinthedeterminationofcoordinatesfromthemeanvalueforthedayofrefractiveindex

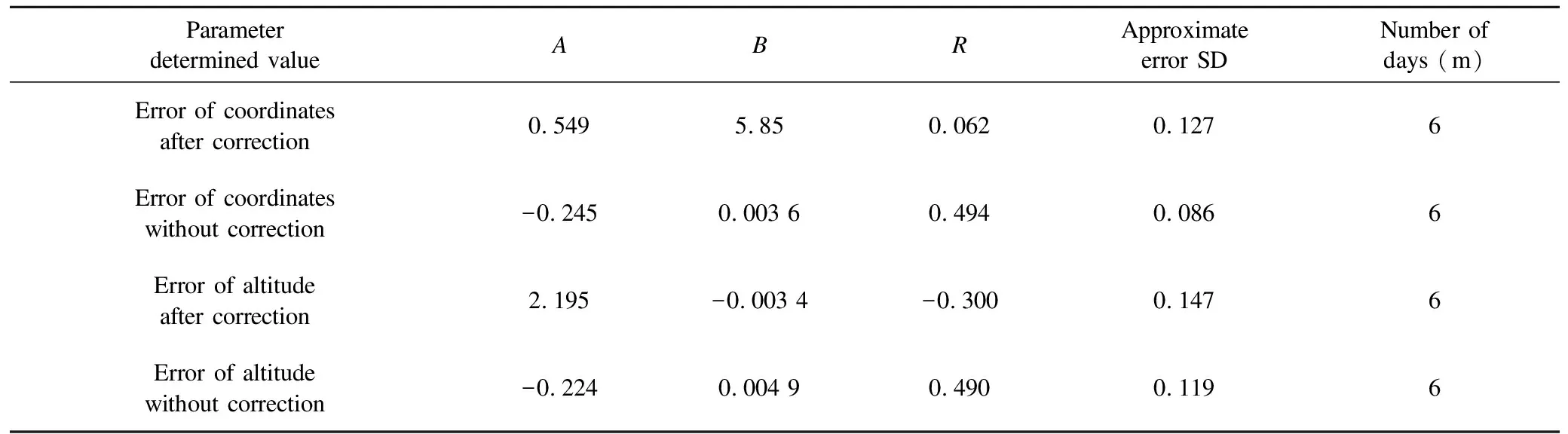
Parameterdetermined valueABRApproximate error SDNumber of days (m)Error of coordinates after correction0.5495.850.0620.1276Error of coordinates without correction-0.2450.003 60.4940.0866Error of altitude after correction2.195-0.003 4-0.3000.1476Error of altitude without correction-0.2240.004 90.4900.1196

The uncorrected values of the rms error in the determination of the coordinates and altitude are correlated with the refractive index values of the troposphere.Introducing correction allows reducing it.By eliminating correlation with state of the troposphere error component, determination of coordinates and altitude can improve the accuracy of their determination.

3 Conclusion

It is shown that the use of standard accounting models for zenith tropospheric delay gives higher values of rms errors in determination of coordinates and altitude.Correction of these data with respect to the refractive index of the troposphere at the time of measurement can improve the accuracy of their determination.Reduction in the rms error in determination of coordinates is about 20%-30%, and altitude is to 30%-40%.

[1] Saastamoinen J.Atmospheric correction for troposphere and stratosphere in radio ranging of satellites In: Proceedings of the 3rd International Symposium on the Use of Artificial Satellites for Geodesy, American Geophysical Union, 2013, 15: 461.

[2] Hopfield H S.Tropospheric range error at the zenith.Space Research XII, Akademie-Verlag, Berlin, 1972: 581-594.

[3] Minimum operational performance standards for global positioning system, wide area augmentation system airborne equipment.RTCA/DO-229C, 2001.

[4] Black H D, Eisner A.Correcting satellite Doppler data for tropospheric effects.Journal of Geophysical Research, 1984, 89(D2): 2616-2626.

[5] Combined performances for SBAS receivers using WAAS and EGNOS.EU-US Cooperation on Satellite Navigation, 2010.

[6] Lutsenko V I, Lutsenko I V, Sitnik.O V, et al.Predicting of the refractive index of the troposphere from measurements of meteorological parameters in the reference points.Radio Physics and Electronics, 2012, 3(4):54-63.

[7] Lutsenko V I, Lutsenko I V, Popov I V, et al.Diagnostics of refraction coefficient on results of meteorological parameters measurement in arbitrary points of area.In: Proceedings of 2010 International Kharkov Symposium on Physics and Engineering of Microwaves, Millimeter and Submillimeter Waves, Kharkov, Ukraine, June 21-26, 2010[2013-06-12].http:∥www.ire.kharkov.ua/MSMW10/index.htm.

对流层延迟修正的实证模型

V F Kravchenko1, V I Lutsenko2, I V Lutsenko2, D O Popov2, A G Laush3, V N Gudkov3

(1.Kotel’nikov Institute of Radio Engineering and Electronics, Russian Academy of Sciences, Moscow 125009, Russia; 2.Usikov Institute of Radiophysics and Electronics of National Academy of Sciences of Ukraine, Kharkov 61085, Ukraine; 3.Co Ltd.Navis-Ukraine, Smela 20708, Ukraine)

摘 要:针对对流层延迟问题， 考虑测量时对流层的折射率， 采用标准模型计算对流层修正概率。 经大量实验研究得到对流层延迟修正模型， 它可以减少坐标测量误差约30%， 海拔测量误差约40%。

关键词:对流层延迟； 卫星导航信号； 全球定位系统(GPS); 全球导航卫星系统(GLONASS)

引用格式：Kravchenko V F, Lutsenko V I, Lutsenko I V, et al.Empirical model of correction for zenith tropospheric delay.Journal of Measurement Science and Instrumentation, 2014, 5(4): 20-28.[doi: 10.3969/j.issn.1674-8042.2014.04.005]

Article ID：1674-8042(2014)04-0020-09

10.3969/j.issn.1674-8042.2014.04.005

Receiveddate： 2014-08-10

Foundation item：Task Complex Program of National Academy of Sciences of Ukraine on Space Research for 2012-2016

Corresponding author：V F Kravchenko (kvf-ok@mail.ru)