Measurement of spatiotemporal characteristics of femtosecond laser pulses by a modified single-shot autocorrelation
Deng Yangbao Deng Shuguang Xiong Cuixiu Zhang Guangfu Tian Ye Shen Lianfeng
(1National Mobile Communications Research Laboratory, Southeast University, Nanjing 210096, China)(2College of Communication and Electronic Engineering, Hunan City University, Yiyang 413000, China)
Measurement of spatiotemporal characteristics of femtosecond laser pulses by a modified single-shot autocorrelation
Deng Yangbao1,2Deng Shuguang2Xiong Cuixiu2Zhang Guangfu2Tian Ye2Shen Lianfeng1
(1National Mobile Communications Research Laboratory, Southeast University, Nanjing 210096, China)(2College of Communication and Electronic Engineering, Hunan City University, Yiyang 413000, China)
To overcome the shortcomings of the single-shot autocorrelation (SSA) where only one pulse width is obtained when the SSA is applied to measure the pulse width of ultrashort laser pulses, a modified SSA for measuring the spatiotemporal characteristics of ultrashort laser pulses at different spatial positions is proposed. The spatiotemporal characteristics of femtosecond laser pulses output from the Ti:sapphire regenerative amplifier system are experimentally measured by the proposed method. It was found that the complex spatial characteristics are measured accurately. The pulse widths at different spatial positions are various, which obey the Gaussian distribution. The pulse width at the same spatial position becomes narrow with the increase in input average power when femtosecond laser pulses pass through a carbon disulfide (CS2) nonlinear medium. The experimental results verify that the proposed method is valid for measuring the spatiotemporal characteristics of ultrashort laser pulses at different spatial positions.
spatiotemporal characteristics; modified single-shot autocorrelation; femtosecond laser pulses; ultrafast laser technology
With the rapid development of the ultrafast laser technology, the pulse width of ultrashort laser pulses has entered the attosecond field[1-2]. Ultrafast laser technology has broad application prospects in many fields, such as optical communication, laser-plasma interaction, and pump-probe spectroscopy[3-5]. The ultrashort laser pulse is used as a very short time probe, which provides an important tool for investigation in the microscopic world. Before ultrashort laser pulses are used for practical applications, it is necessary to properly characterize their main parameters, including energy, power, spectrum, spatial beam, temporal shape, pulse width and phase. It is easy to measure the energy, power, spectrum and spatial shape directly, but it is more difficult to measure the temporal shape, pulse width and phase directly due to the limitations of the instruments.
There are two types of methods for measuring the temporal shape and pulse width. One is a direct electronic technique, consisting of fast photodiodes and high-bandwidth oscilloscopes[6], which are limited to the several picoseconds. Therefore, fast photodiodes and high-bandwidth oscilloscopes are not suitable for recording the temporal profile of an ultrashort (femtosecond or attosecond) laser pulse. The only detector that reaches a time resolution below one picosecond is the streak camera[7], which is also not suitable for measuring the temporal profile of the ultrashort laser pulse. The other is the optical correlation technique, mainly including intensity autocorrelation[8-10], intensity cross-correlation[11-12], frequency resolved optical gating (FROG)[13-14]and spectral phase interferometry for direct electric-field reconstruction (SPIDER)[15-16]. The intensity autocorrelation technique uses a model to retrieve the pulse shape according to the experimental data. The intensity cross-correlation technique characterizes the temporal shape of a complex pulse directly by measuring the sum-frequency signal light, whose resolution primarily depends on the pulse width of a probe pulse[11]. Unfortunately, the autocorrelation and cross-correlation only measure the pulse shape and pulse width, and do not measure the phase characteristics. FROG retrieves the electric field of ultrashort laser pulses based on a complex iterative algorithm. SPIDER does not give the pulse width information directly, but reconstructs the pulse shape and pulse width by the Fourier transform. FROG and SPIDER are very useful for measuring the amplitude and phase of the ultrashort laser pulse, but they are more complex in experiment operations.
In all the above methods, the SSA’s operation is simple and convenient, and it is also suitable for measuring the ultrashort laser pulse with a pulse width of hundreds of femtoseconds. However, only one pulse width of the whole beam is obtained when the SSA is used to measure the pulse width of ultrashort laser pulses. In fact, pulse stretching and compression are asymmetric, causing the pulse widths at different spatial positions to be different due to the effect of the residual spatial chirp. For the shortcomings of the SSA and the aforementioned reasons, a modified SSA is proposed for measuring the spatiotemporal characteristics of the femtosecond laser pulse. The proposed method not only measures the spatiotemporal characteristics of a laser pulse at different spatial positions, but also characterizes the temporal evolution of a pulse at the same spatial position after nonlinear propagation.
1 Measurement Principle Analysis
SSA is used to measure the pulse width of ultrashort laser pulses, which mainly measures the cross distribution of the second harmonic (SH) beam. If the pulse has the Gaussian time shape, the cross sizeDof the SH beam depending on the pulse widthTof base frequency laser pulse is written as[7]
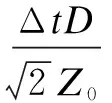
(1)
where Δtis time delay, andZ0is the center of the SH beam cross distribution. It should be noted that Eq.(1) is correct under the condition of
(2)
wherenis the refraction index of the nonlinear crystal;uis the light velocity of the base frequency laser pulse in the crystal;Tis the pulse width of base frequency laser pulse at FWHM;dis the beam diameter of the base frequency beam at the FWHM of intensity;Ψis the angle between the two beams outside the crystal.
It is clear that direct measurement of ΔtandZ0is rather difficult. With the help of optical delay line, it is possible to change the value of Δt. Simultaneously, the centerZ0of the SH beam is changed. If two centersZ01andZ02are corresponding to the micrometric head positionsL1andL2, the time delay differential can be written as
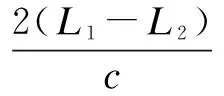
(3)
wherecis the light velocity of the base frequency beam in vacuum. On the other hand, the time delay differential can be obtained from Eq.(1),
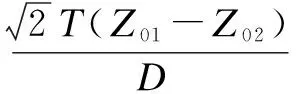
(4)
By Eqs.(3) and (4), we can obtain
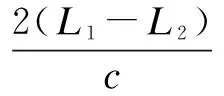
(5)
By Eq.(5), the pulse widthTcan be written as

(6)
which is the basic expression for measuring the pulse width based on the SSA.
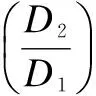
(a)
(b)
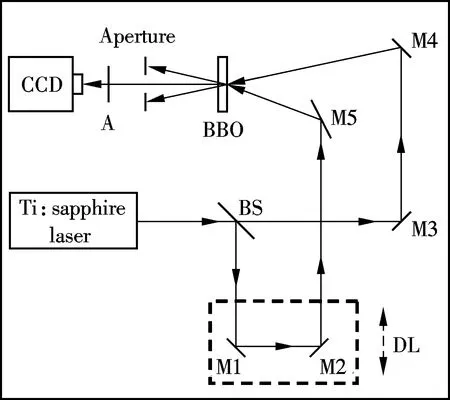
2 Experimental Setup of Modified SSA
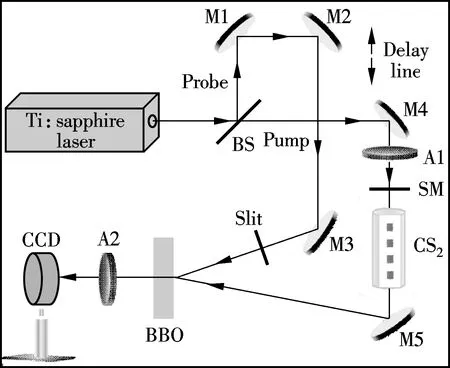
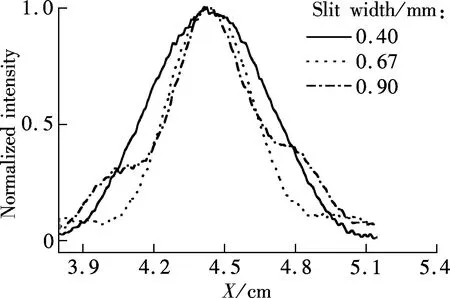
The experimental setup of the modified SSA and spatial intensities distributions of the probe laser pulse after a slit are shown in Fig.2. In Fig.2(a), A1 and A2 represent the adjustable neutral density attenuators and SM is the spatial modulation. The femtosecond laser pulse is output from the Ti:sapphire regenerative amplifier system (Coherent Libra S), whose main parameters are as follows: the pulse width is 100 fs; central wavelength is 800 nm and then repetition frequency is 1 kHz. A collimated laser pulse is separated into two laser pulses by a beam splitter, where one is used as a pump laser pulse and the other is used as a probe laser pulse that is controlled by a slit. The reflectivity of the beam splitter is about 20%. The sum-frequency light with the 400 nm wavelength is generated in the BBO crystal (7 mm×7 mm×0.7 mm) by a small angle nonlinear sum-frequency interaction between the pump laser pulse and the probe laser pulse. The charge-coupled device CCD (Coherent Laser Cam-HRTM, 1 280×1 024 pixel, resolution: 6.7 μm×6.7 μm) has two functions. One is used to measure the spatial characteristics of the pump laser pulse directly, and the other is used to measure the pulse width evolution of the pump laser pulse at different spatial positions indirectly based on the principle in Section 1. As the probe laser pulse is controlled by a silt, the transverse spatial profile of the probe laser pulse after passing through a slit is approximately a sinc function distribution[17], as shown in Fig.2(b). The main peak becomes narrow and the side-lobes are very weak with the increment of the slit width. However, the intensities of the side-lobes increase gradually when the slit width is wider than 0.67 mm. In order to achieve the narrowest spatial distributions of the main peak and ensure that the side-lobes have little effect on the measurement results, the slit width is adjusted to 0.67 mm (see Fig.2(b)).
(a)
(b)
3 Experimental Results and Discussion
3.1 Measurement of the spatial characteristics
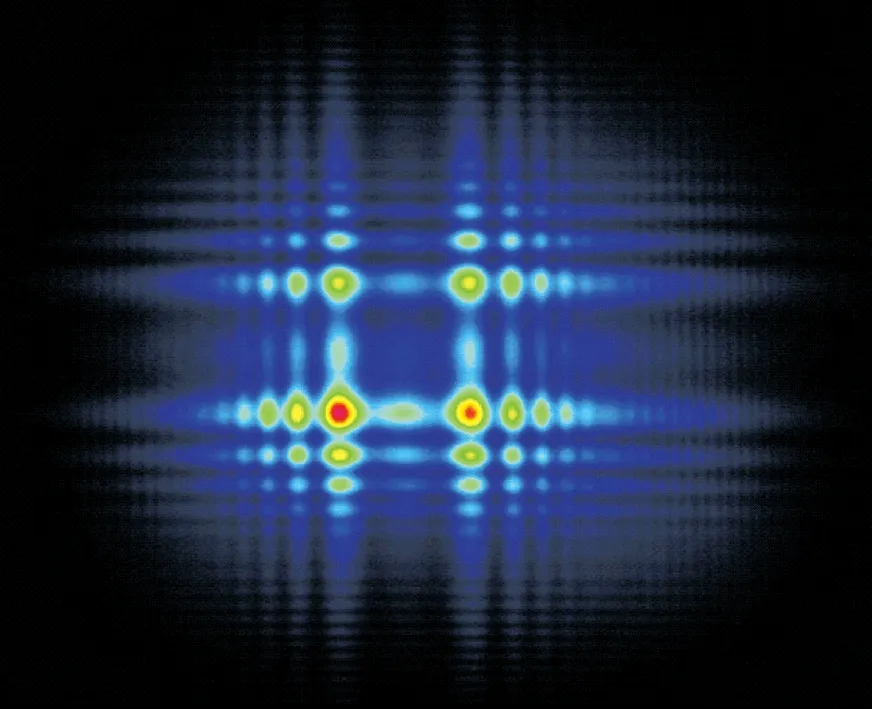
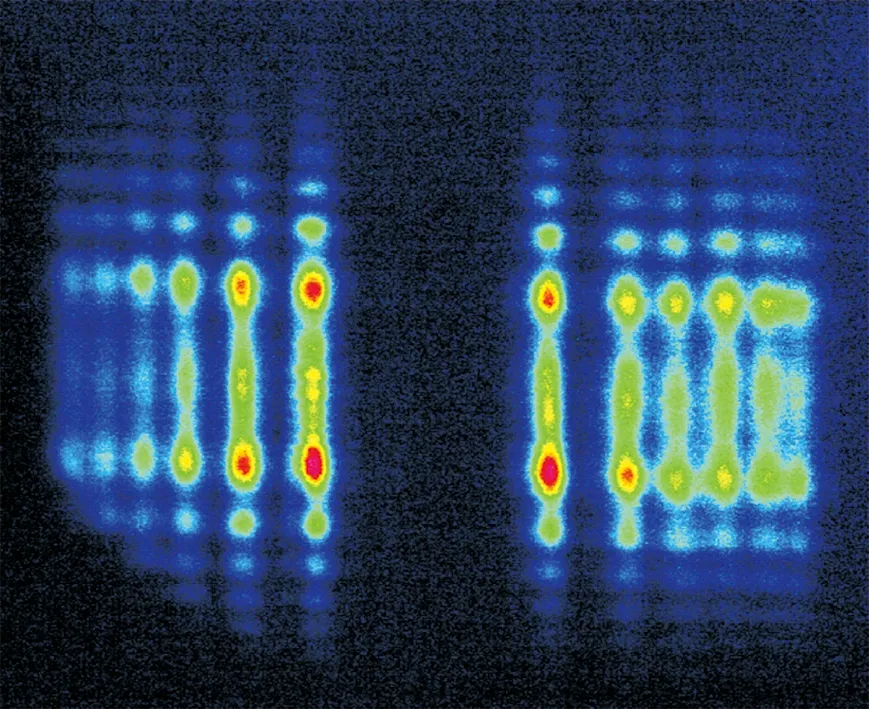
The spatial intensity distribution of the pump laser pulse with a cross-silk modulation and the measured spatial characteristics of the pump laser pulse are shown in Fig.3. In order to measure the complex transverse spatial characteristics of the pump laser pulse, the slit is vertically placed. The experimental measured transverse spatial distributions of the modulation peaks of the pump laser pulse are shown in Fig.3(b), which is almost the same as that in Fig.3(a). The BBO crystal is not sufficiently large for our experiment and the edge spatial intensity of the pump laser pulse is very weak, so Fig.3(b) does not characterize the edge spatial characteristics of the pump laser pulse. The longitudinal spatial intensity distributions of the pump laser pulse with a cross-silk modulation are also measured when the slit is horizontally placed. Therefore, all the spatial intensity distributions of the ultrashort laser pulse are measured accurately based on the modified SSA.
(a)
(b)
Fig.3 Experimental results of spatial measurement. (a) Spatial intensity distribution of the pump laser pulse with a cross-silk modulation; (b) Measured transverse spatial intensity distribution of pump laser pulse
3.2 Measurement of the temporal characteristics
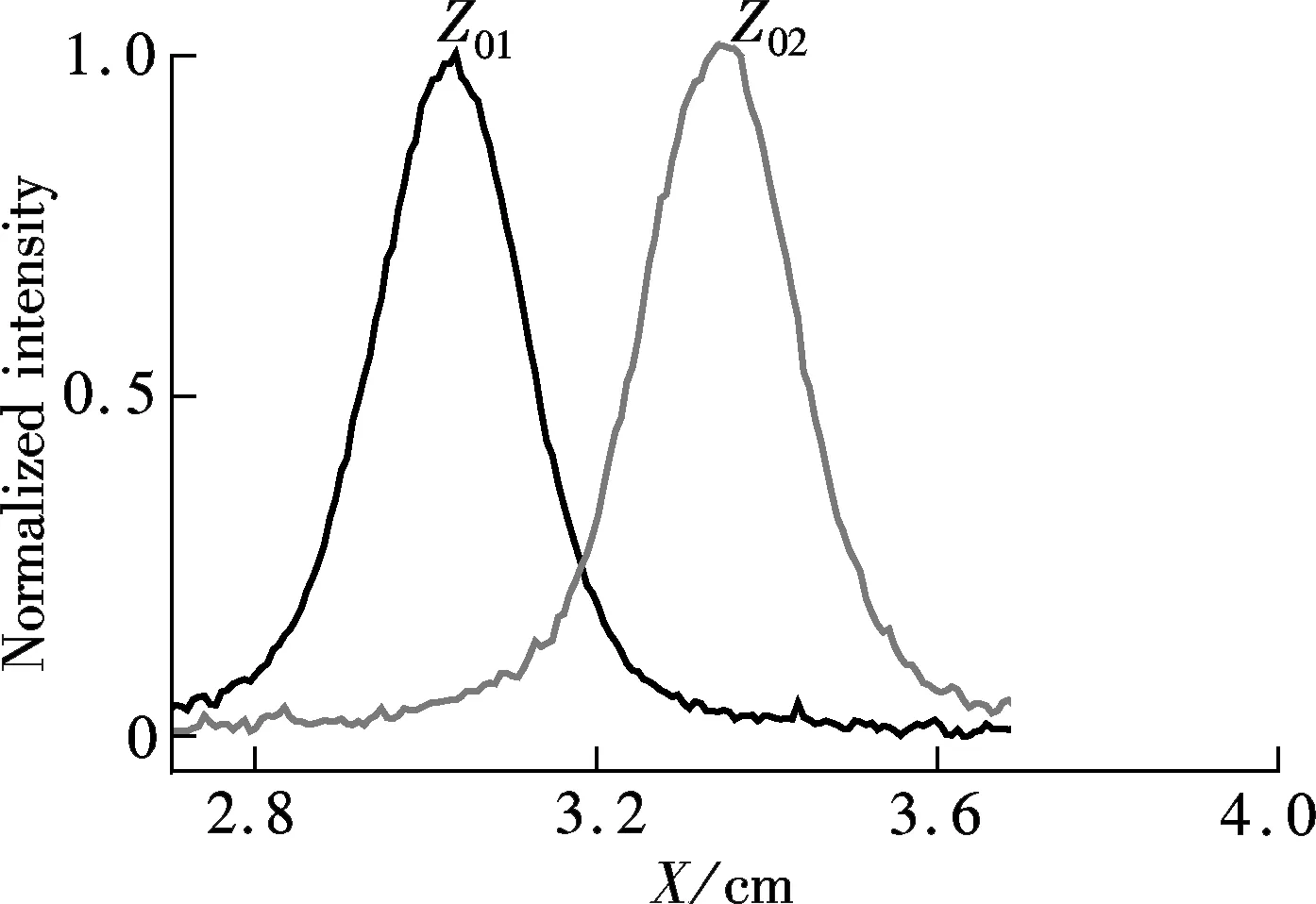
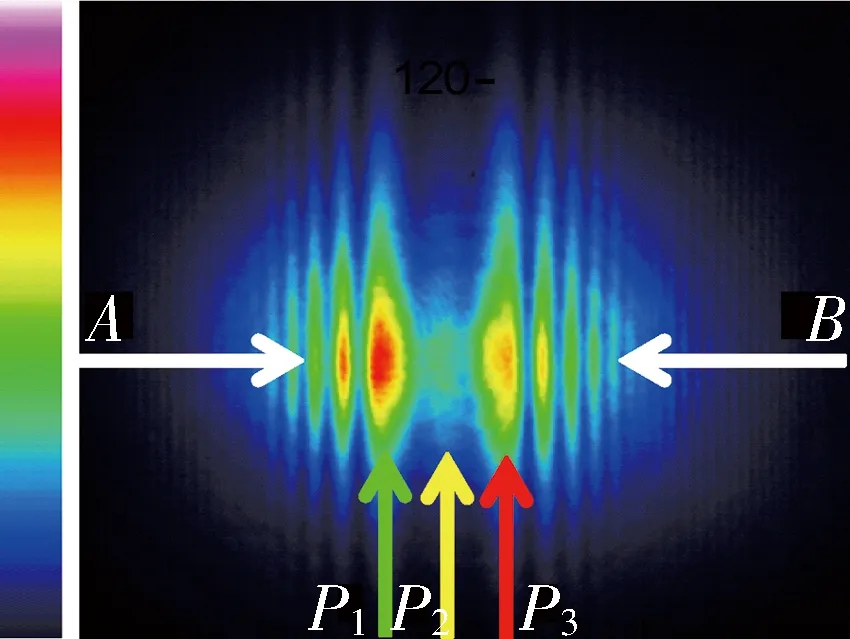
The experimental measured temporal characteristics are shown in Fig.4. As the initial spatial modulation shape of the pump laser pulse has little effect on the validity of the pulse width measurement, for convenience of spatial orientation, the pump laser pulse is added to a single silk modulation during measurement, whose spatial intensity distribution is shown in Fig.4(a). When the slit is vertically placed, the pulse widths at different transverse spatial positions between pointsAandBare measured as shown in Fig.4(b), where the solid square boxes are the experimental results and the solid curve is the Gaussian fitting results. The pulse widths at different transverse spatial positions obey the Gaussian distribution. The pulse width of the pump laser pulse at the strongest spatial modulation positionP1is measured and then the probe laser pulse is moved in the transverse spatial direction step by step. So the pulse width at different transverse spatial positions between pointsAandBare measured. The pulse widths at transverse spatial positions are represented byP1,P2andP3(see Fig.4(a)) are 95, 106 and 100 fs (see Fig.4(b)), respectively. The pulse width atP3position is the same as that measured by the SSA. Pulse broadening and compression are asymmetric, which causes the best compression in the central positions, thus the pulse widths at edge positions are wider than those of central positions due to the effect of the residual spatial chirp (see Fig.4(b)). The pulse widths at different longitudinal spatial positions are also measured when the slit is horizontally placed. Therefore, the pulse widths of the ultrashort laser pulse at all spatial positions are measured based on the modified SSA.
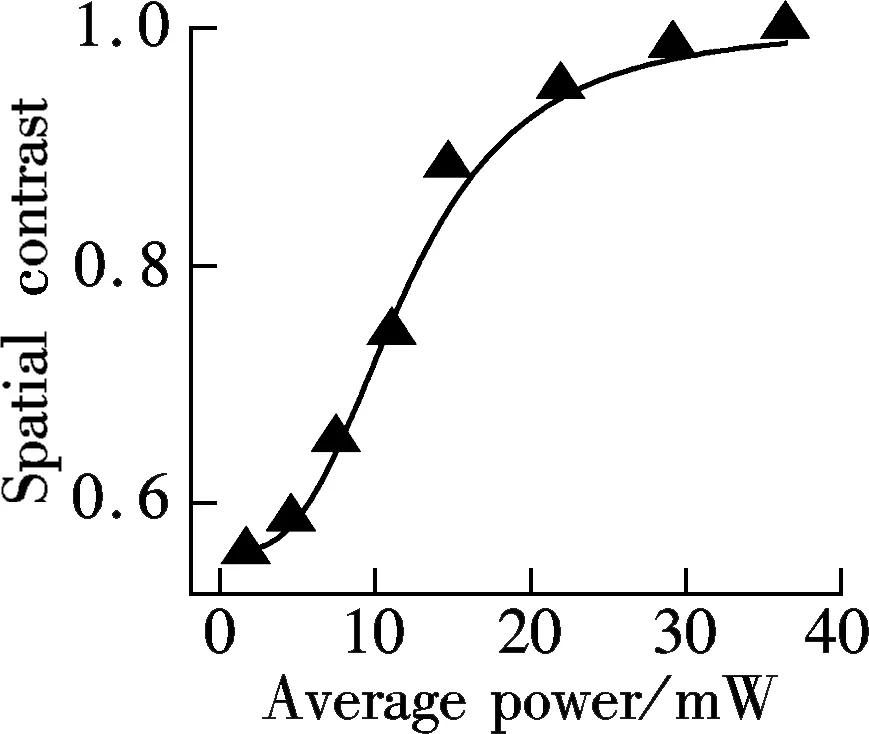
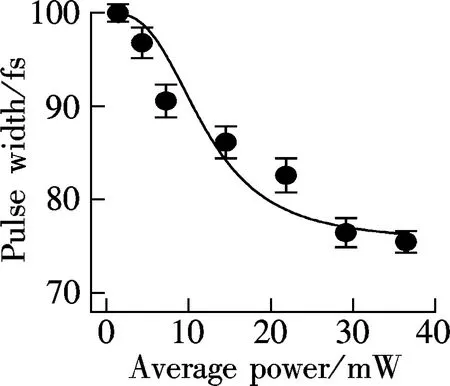
Furthermore, the temporal evolution of the pump laser pulse atP3position after propagating in the CS2nonlinear medium is measured. The variation of the measured spatial contrast with input average power is shown in Fig.4(c),where the solid triangles are the experimental results and the solid curve is the nonlinear fitting results. It is clearly seen that the spatial contrast is increased with the increment of rising power, so the pump laser pulse generates a small-scale self-focusing effect (see Fig.4(c)). The small-scale self-focusing effect enhances the spatial intensity atP3position, which in turn affects the temporal evolution at this position. The measured evolution of pulse width atP3position is represented in Fig.4(d), where the solid circle boxes are the experimental results and the solid curve is the nonlinear fitting results. The pulse width becomes narrower with the increase in the input average power due to the spatiotemporal coupling effect[18].
(a)

(b)
(c)
(d)
Fig.4 Experimental results of temporal measurement. (a) Spatial intensity distribution of the pump laser pulse with a single silk modulation; (b) The measured pulse widths of pump laser pulse at different spatial positions between pointsAandB; (c) Variation of spatial contrast with input average power; (d) Variation of the measured temporal evolutions of pump laser pulse atP3position with input average power
4 Conclusion
In this paper, a modified SSA is proposed for measuring the spatiotemporal characteristics of a femtosecond laser pulse at different spatial positions. After theoretical analyses on the measurement principle, the spatiotemporal characteristics of femtosecond laser pulses output from the Ti:sapphire regenerative amplifier system are measured by the proposed method. The experimental results show that the complex spatial characteristics are measured accurately when the femtosecond laser is added to a diffraction modulation. The initial pulse widths of the whole beam at different spatial positions are different due to the effect of the residual spatial chirp, which obey the Gaussian distribution. When the femtosecond laser pulse propagates in the CS2nonlinear medium, the pulse width at the same spatial position becomes narrow with the increase in the input average power due to the spatiotemporal coupling effect. Therefore, the experimental results verify that the proposed method is valid for measuring the spatiotemporal characteristics of ultrashort laser pulses at different spatial positions.
[1]Ferrari F, Calegari F, Lucchini M, et al. High-energy isolated attosecond pulses generated by above-saturation few-cycle fields[J].NaturePhotonics, 2010, 4(12): 875-879.
[2]Baker S, Walmsley I A, Tisch J W G, et al. Femtosecond to attosecond light pulses from a molecular modulator[J].NaturePhotonics, 2011, 5(11): 664-671.
[3]Sun X L, Skillman D R, Hoffman E D, et al. Free space laser communication experiments from Earth to the Lunar Reconnaissance orbiter in lunar orbit[J].OpticsExpress, 2013, 21(2): 1865-1871.
[4]Cairns R A, Bingham R, Norreys P, et al. Laminar shocks in high power laser plasma interactions[J].PhysicsofPlasmas, 2014, 21(2): 022112.
[5]Shang J Z, Ma L, Li J W, et al. Femtosecond pump-probe spectroscopy of graphene oxide in water[J].JournalofPhysicsD:AppliedPhysics, 2014, 47(9): 094008.
[6]Träger F.Handbookoflasersandoptics[M]. Berlin: Springer, 2007:962-978.
[7]Tsuchiya Y. Advances in streak camera instrumentation for the study of biological and physical processes[J].IEEEJournalofQuantumElectronics, 1984, 20(12): 1516-1528.
[8]Kolliopoulos G, Tzallas P, Bergues B, et al. Single-shot autocorrelator for extreme-ultraviolet radiation[J].JournalofOpticalSocietyofAmericaB, 2014, 31(5): 926-938.
[9]Moshammer R, Pfeifer T, Rudenko A, et al. Second-order autocorrelation of XUV FEL pulses via time resolved two-photon single ionization of He[J].OpticsExpress, 2011, 19(22): 21698-21706.
[10]Wei Y Z, Howard S, Straub A, et al. High sensitivity third-order autocorrelation measurement by intensity modulation and third harmonic detection[J].OpticsLetters, 2011, 36(12): 2372-2374.
[11]Deng Y B, Yang H, Tang M, et al. Experimental research on measuring the fine structure of long pulse in time domain by synchronized ultrashort pulse[J].OpticsCommunications, 2011, 284(3): 847-851.
[12]Ma J, Yuan P, Wang Y Z, et al. Single-shot cross-correlator using a long-wavelength sampling pulse[J].OpticsLetters, 2011, 36(6): 978-980.
[13]Wilcox D E, Fuller D F, Ogilvie J P. Fast second-harmonic generation frequency-resolved optical gating using only a pulse shaper[J].OpticsLetters, 2013, 38(16): 2980-2983.
[14]Calò C, Schmeckebier H, Merghem K, et al. Frequency resolved optical gating characterization of sub-ps pulses from single-section InAs/InP quantum dash based mode-locked lasers[J].OpticsExpress, 2014, 22(2): 1742-1748.
[15]Pasquazi A, Peccianti M, Azaa J, et al. FLEA: Fresnel-limited extraction algorithm applied to spectral phase interferometry for direct field reconstruction (SPIDER)[J].OpticsExpress, 2013, 21(5): 5743-5758.
[16]Tsermaa B, Yang B K, Kim J S, et al. Crystal-dithering method applied to spectral phase interferometry for direct electric-field reconstruction (SPIDER) for sensitivity enhancement of the pulse phase measurement[J].OpticsCommunications, 2011, 284(7): 1955-1958.
[17]Goodman J W.IntroductiontoFourieroptics[M]. 3rd ed. Englewood: Roberts & Company, 2005: 28-55.
[18]Deng Y B, Fu X Q, Tan C, et al. Experimental investigation of spatiotemporal evolution of femtosecond laser pulses during small-scale self-focusing[J].AppliedPhysicsB, 2014, 114(3): 449-454.
基于改进的单次自相关测量飞秒激光脉冲的时空特性
邓杨保1, 2邓曙光2熊翠秀2张光富2田 野2沈连丰1
(1东南大学移动通信国家重点实验室, 南京 210096)(2湖南城市学院通信与电子工程学院, 益阳 413000)
针对单次自相关方法只能测量一个脉宽的缺点,提出一种改进的单次相关方法测量超短激光脉冲全空域中不同空间位置的时空特性.通过实验测量了钛宝石激光器输出飞秒激光脉冲的时空特性,结果表明飞秒激光脉冲全空域中不同位置的复杂空间特性得以精密测量;不同空间位置的时间脉宽不同,它们服从高斯分布;当飞秒激光脉冲经过二硫化碳非线性介质传输后,随着输入平均功率的增加,同一空间位置的时间脉宽呈现慢慢变窄的趋势.实验结果验证了所提方法可以有效地测量超短激光脉冲全空域中不同空间位置的时空特性.
时空特性; 改进的单次自相关; 飞秒激光脉冲; 超快激光技术
TN2
Received 2014-05-07.
Biographies:Deng Yangbao (1983—), male, doctor, lecturer; Shen Lianfeng (corresponding author), male, professor, lfshen@seu.edu.cn.
s:The National Natural Science Foundation of China (No.61171081, No.61471164), the Natural Science Foundation of Hunan Province (No.14JJ6043).
:Deng Yangbao, Deng Shuguang, Xiong Cuixiu,et al.Measurement of spatiotemporal characteristics of femtosecond laser pulses by a modified single-shot autocorrelation[J].Journal of Southeast University (English Edition),2014,30(4):411-415.
10.3969/j.issn.1003-7985.2014.04.002
10.3969/j.issn.1003-7985.2014.04.002
 Journal of Southeast University(English Edition)2014年4期
Journal of Southeast University(English Edition)2014年4期
- Journal of Southeast University(English Edition)的其它文章
- Improved design of reconfigurable frequency response masking filters based on second-order cone programming
- Experimental study on thermal characteristicsof a double skin façade building
- Gene expression profile in H4IIE rat hepatoma cells exposed to an antifouling booster biocide Irgarol-1051 degradation product
- Synthesis and application of an environmentallyfriendly antiscalant in industrial cooling systems
- L(1,2)-edge-labeling for necklaces
- Design and implementation of multi-hop video transmission experiment system in VANET
