多维函数在广义三角范数下的收敛定理

雷国梁，岳田

（湖北汽车工业学院理学院，湖北十堰442002）

多维函数在广义三角范数下的收敛定理

雷国梁，岳田

（湖北汽车工业学院理学院，湖北十堰442002）

给出了广义T模糊测度和多维函数(T)模糊积分的定义，采用类比方法证明了多维可测函数在一维测度下对应的EropoB定理和Riesz定理，得到了多维(T)可积函数在广义三角范数下的2个收敛定理。

广义T测度；多维(T)模糊积分；依模糊测度收敛；收敛定理

模糊数学相关理论及应用研究不断丰富与发展，已形成了一个有着广泛应用的数学分支。近年来在模糊集上建立测度与积分理论，是模糊数学走向成熟的重要标志之一。如文献［1］研究了模糊积分运算的特点并提出了广义模糊积分的概念；文献［2］中给出了广义模糊积分的收敛定理；文献［3-5］通过增加一些适当条件改善了广义模糊积分的收敛定理，而且推广了文献［6］中的结果；文献［7-8］通过改进广义三角范数的定义，建立了广义TFuzzy测度空间上的T-Fuzzy积分，并讨论了相应积分的若干性质，同时给出了T-Fuzzy积分的收敛性定理；文献［9-10］将T-Fuzzy积分的被积函数由一维情形推广到了多维情形，并给出了多维函数在一维测度和多维测度下的定义和相关性质。本文中将在上述文献基础上，给出广义T模糊测度和多维函数T模糊积分的概念，并对多维(T)可积函数在广义三角范数下的收敛性定理进行研究。

1 定义及相关引理

定义1 设(Ω ,℘ )是可测空间，若集合函数μ:℘→R+满足：

1）μ(∅)=0；若A,B∈℘,A⊂B，则

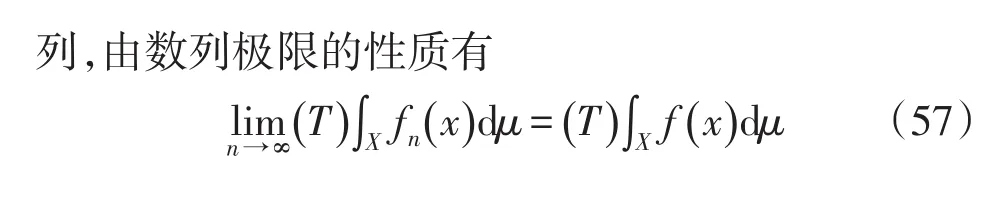
［1］吴从炘，马明.模糊分析学基础［M］.北京：国防工业出版社，1991．

［2］Zhang D L，Guo C M.On the convergence of sequences of fuzzy measures and generalized convergence theorems of fuzzy integrals［J］.Fuzzy Sets and Systems，1995（72）：349-356．

［3］Fang J X.On the convergence theorems of generalized fuzzy integral sequence［J］.Fuzzy Sets and Systems，2001（124）：117-123．

［4］Fang J X.A note on the convergence theorem of general⁃ized fuzzy integrals［J］.Fuzzy Sets and Systems，2002（127）：377-381．

［5］Fang J X.Some properties of sequences of generalized fuzzy integrable functions［J］.Fuzzy Sets and Systems，2007（158）：1832-1842．

［6］Wang Z Y，Klir G J.Generalized Measure Theory［M］．New York：Springer，2008．

［7］Song X Q，Pan Z.Fuzzy algebra in triangular norm system［J］.Fuzzy Sets and Systems，1998（93）：331-335．

［8］宋晓秋.模糊数学原理与方法［M］.徐州：中国矿业大学出版社，2004．

［9］Liu W L，Song X Q，Zhang Q Z.（T）fuzzy integral of multi-dimensional function［C］∕∕2010 7thInternational Conference on Fuzzy systems and Knowledge Discovery．IEEE Circuits and Systems Society，2010：1-5．

［10］Liu W L，Song X Q，Zhang Q Z，et al.（T）fuzzy integral of multi-dimensional function with respect to multi-val⁃ued measure［J］.Iranian Journal of Fuzzy Systems，2012（9）：111-126．

Convergence Theorem of Multi-dimensional Function Based on Generalized Triangular Norm

Lei Guoliang，Yue Tian
（School of Science,Hubei University of Automotive Technology,Shiyan 442002,China）

The concept of generalized(T)fuzzy measure and generalized(T)fuzzy integral of multidimensional function were introduced.EropoB theorem and Riesz theorem based on one dimensional fuzzy measure were given by comparison and inference.The two convergence theorems of multi-dimen⁃sional function(T)fuzzy integral were obtained.

generalized(T)measure;multi-dimensional(T)fuzzy integral;convergence in fuzzy measure;convergence theorem

O159

A

1008-5483（2014）04-0053-05

2014-09-26

国家自然科学基金（51374199）；中央高校基本科研业务费专项资金（2012LWB53）

雷国梁（1971－），男，湖北十堰人，硕士，从事应用数学研究。

10.3969∕j.issn.1008-5483.2014.04.013