Unloading-induced permeability recovery in rock fractures

To Lin,Wen Meng,Yueu Chen,c,Zhihong Zho,**,Bing Liu,Jintong Zhng,Sicong Chen,Xinggung Zho

a Department of Civil Engineering,Tsinghua University,Beijing,China

b Key Laboratory of Neotectonic Movement and Geohazard,Institute of Geomechanics,Chinese Academy of Geological Sciences,Beijing,China

c College of Mining Engineering,Taiyuan University of Technology,Taiyuan,China

d CNNC Key Laboratory on Geological Disposal of High-level Radioactive Waste,Beijing Research Institute of Uranium Geology,Beijing,China

Keywords:Unloading Permeability Rock fracture Temperature Empirical model

ABSTRACT Underground space creation and energy extraction,which induce unloading on rock fractures,commonly occur in various rock engineering projects,and rock engineering projects are subjected to high temperatures with increasing depth.Fluid flow behavior of rock fractures is a critical issue in many subsurface rock engineering projects.Previous studies have extensively considered permeability evolution in rock fractures under loading phase,whereas changes in fracture permeability under unloading phase have not been fully understood.To examine the unloading-induced changes in fracture permeability under different temperatures,we performed water flow-through tests on fractured rock samples subjected to decreasing confining pressures and different temperatures.The experimental results show that the permeability of fracture increases with unloading of confining pressure but decreases with loadingunloading cycles.Temperature may affect fracture permeability when it is higher than a certain threshold.An empirical model of fracture hydraulic aperture including two material parameters of initial normal stiffness and maximum normal closure can well describe the permeability changes in rough rock fracture subjected to loading-unloading cycles and heating.A coupled thermo-mechanical model considering asperity damage is finally used to understand the influences of stress paths and temperatures on fracture permeability.

1.Introduction

Either underground space creation or energy extraction can lead the surrounding rock mass to deform and fracture,during which the permeability of the surrounding rock mass may significantly increase (Qiu et al.,2014;Xing and Zhao,2023).Therefore,a well understanding of changes in rock fracture permeability during unloading is crucially important for seepage control and disaster mitigation in subsurface rock engineering projects.The relationships between normal or confining stresses and fracture permeability have been experimentally studied in the previous studies,as listed in Table 1 (Selvadurai,2015;Vogler et al.,2016;Selvadurai et al.,2018;Song et al.,2018;Jiang et al.,2020;Zhao et al.,2020).It is found that fracture permeability nonlinearly decreases with increasing effective normal or confining stresses,whereas there is only a partial recovery of fracture permeability during unloading phase due to irreversible damage at contacting asperities,i.e.hysteresis effect(Barton et al.,1985;Selvadurai,2015;Selvadurai et al.,2018;Zhao et al.,2020).Many empirical or theoretical models were proposed to describe the stress-dependent permeability of fractured rock samples considering the hysteresis effect (Gangi,1978;Scholz and Hickmann,1983;Gutierrez et al.,2000;Lavrov,2017;Huang et al.,2021;Wang et al.,2021a).Therefore,the evolutions of fracture permeability under loading and unloading processes should be quantified separately using different model parameters.

Table 1Experimental studies on stress-dependent permeability of rock fractures.

A number of empirical,analytical and numerical models have been proposed to predict the stress-dependent permeability of rock fractures(Table 2).In the empirical models,the constitutive models(relationships between normal or shear stresses and mechanical aperture) and the correlation between mechanical and hydraulic apertures are combined (Barton et al.,1985).In the analytical models,the realistic contacts of rock fractures composed of the two rough surfaces are first transformed into the ideal contacts between a smooth surface and a rough surface,and then the asperity deformation of the rough surfaces is calculated based on the elastoplastic contact deformation principle,e.g.the Hertz solution(Greenwood and Williamson,1966;Walsh and Grosenbaugh,1979;Brown and Scholz,1985,1986;Tang et al.,2014,2016,2017;Tang and Jiao,2017;Zhao et al.,2020;Tang and Zhang,2021).Microscopic numerical models that simulate the asperities as standing cylinders of varying heights are successfully applied to build up the scaling relationship between fracture stiffness and permeability(Hopkins,1990;Pyrak-Nolte and Morris,2000;Marache et al.,2018;Petrovitch et al.,2013,2014;Pyrak-Nolte and Nolte,2016;Wang and Cardenas,2016;Peng et al.,2020).Based on the variational principle,another type of half-space model that uses the Boussinesq’s solution to relate contact stress with surface displacement is developed and widely used to simulate deformation of rock fractures (Li et al.,2015;Lang et al.,2015,2016;Kang et al.,2016;Wu and Sharma,2017;Kling et al.,2018;Zou et al.,2020).Combining finite element method with complex and realistic fracture surface geometry can also reproduce the nonlinear stress-permeability relationships in rock fractures (Walsh et al.,2008).Because the above empirical,analytical or numerical models are mostly validated using the experimental data of fracture closure during the loading phase,whether they can be extended to describe the evolution of fracture permeability during the unloading phase still eludes investigation.

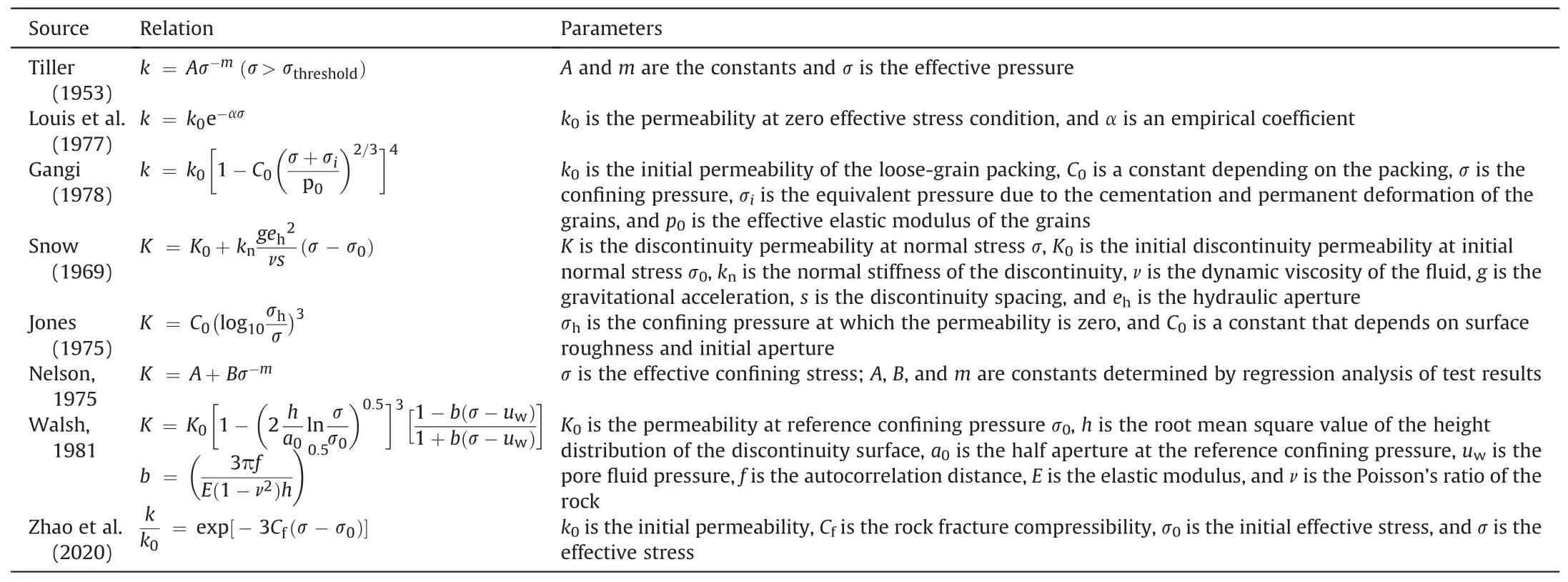
Table 2Theoretical models of stress-dependent permeability of rock fractures.

The main aim of this study is to provide a better understanding of the changes in the permeability of rock fractures during the unloading process under different temperatures.A number of tunnels are being or will be constructed in extremely warm regions,e.g.the highest temperature of the surrounding rocks in the diversion tunnel of the Blunkou-Gongger Hydropower Station in Xinjiang,China,is 82°C,and the air temperature in the diversion tunnel of Qirehataer Hydropower Station in Xinjiang,China,is up to 98°C (Zeng et al.,2020;Zhao et al.,2021b).In this study,we first conducted the permeability tests on the artificial rock fractures under the unloading phase,during which the high temperatures up to 90°C were kept.Second,based on the Barton-Bandis constitutive model and the effective stress coefficient of rock fractures,a theoretical model was developed to describe the evolution of fracture permeability during unloading process.Compared with the empirical exponential or polynomial models,the developed theoretical model has clear physical meaning.Finally,a coupled thermomechanical model for fracture deformation was used to understand the underlying mechanisms of unloading-induced permeability enhancement of rock fractures under different temperatures.

2.Methodology

2.1.Sample preparation

It is known that deep tunnels are subject to three-dimensional(3D) in situ stresses,and the stresses applied on the core are released after coring.The rock’s physical parameters such as porosity and permeability also change,but it is very hard and expensive to conduct in situ test for a tunnel buried in hundreds of meters.Therefore,exploring the changing law of permeability of fractured rock sample in laboratory is an alternative approach.

The fractured core samples were collected from the boreholes(depth of about 620-622 m) at the Layue tunnel construction site in Nyingchi,Tibet,China (Fig.1).The granite is composed of 31% quartz,28%albite,33%microcline,and 8%biotite by volume.Using the Brazilian disc tensile testing apparatus,three cylindrical granite samples (diameter of 50 mm and length of 100 mm) were axially split to produce an artificial fracture between the two semicircular halves(Fig.2).There was one intact granite sample that serves as a reference to compare the difference between the fractured and intact samples.A 3D blue light scanner(OKIO-5M,TENYOUN)was used to obtain the fracture morphology before and after the tests.The dimensionless parameterZ2was calculated as a statistical measure of the fracture surface roughness.The averageZ2value of each fracture surface was calculated from 49 surface profiles at a spacing of 1 mm along the fracture axis.The averageZ2values of three fracture samples(622-1,620 and 622-2)are 0.281,0.347 and 0.305,respectively (Table 3).The surface morphologies of fracture samples obtained before and after the tests were transformed to the same coordinate system by linear transformation,and the changes in asperity heights on fracture surfaces were calculated.

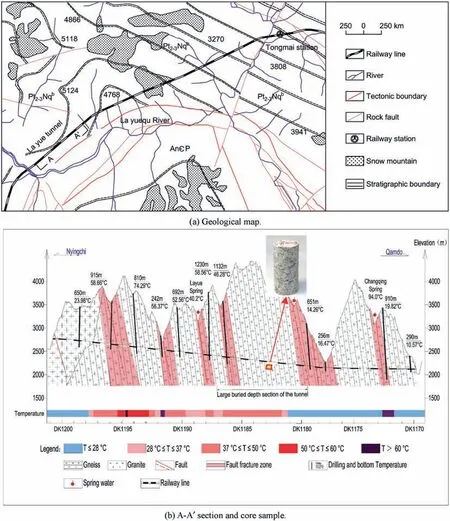
Fig.1.The core samples collected from the boreholes of the Layue tunnel in Nyingchi,Tibet,China.

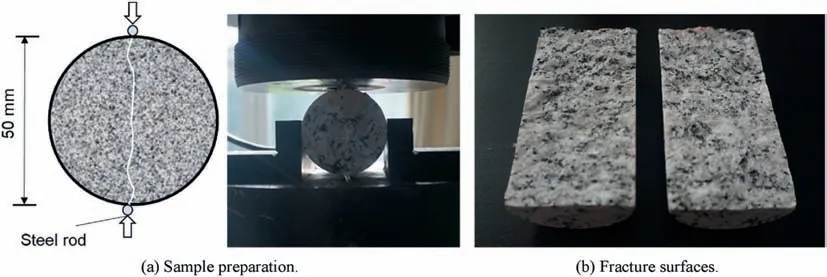
Fig.2.Preparation procedure of fractured rock sample.

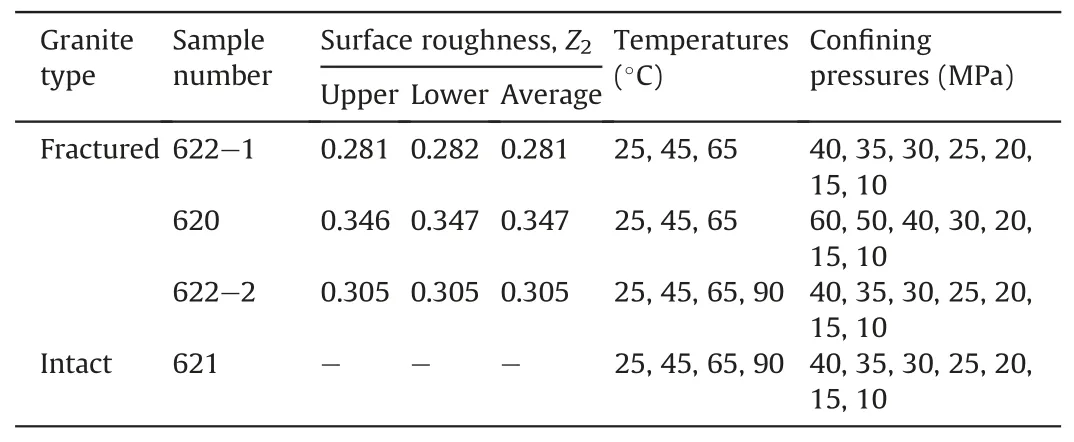
Table 3Rock samples and experimental design.

2.2.Experimental procedure

The high-temperature triaxial rheological test system ROCK 600-50 VHT was used to perform permeability test subjected to confining pressures and temperatures (Fig.3).The test system includes five main units: triaxial cell,loading unit,deformation and pressure monitoring unit,water supply unit,and computerized data-acquisition unit.It has the maximum axial loading capacity of 1500 kN,the maximum confining pressure of 60 MPa,the maximum pore water pressure of 60 MPa and the maximum temperature of 200°C.In the permeability tests,the maximum axial stresses for fractured samples and intact sample were 60 MPa and 100 MPa,and the maximum confining stresses were set as 60 MPa or 40 MPa to simulate the conditions of depth in 2000 m or 1500 m,which are potential risky sections of the Layue tunnel.The problem of high ground temperature is common in the study area,and the maximum measured temperature is 93.5°C.Therefore,the applied temperature in this study was 25°C-90°C.

Fig.3.High-temperature triaxial rheological test system ROCK 600-50 VHT.

The steady-state method was used to measure the sample permeability,and the main experimental procedure is as follows:(1)Assemble the samples into the confining cell and place it in the cylindrical chamber,check the pipeline and sensor connection,and fill the chamber with hydraulic oil;(2) Apply the target temperature and maintain for 2 h to ensure that the whole sample is evenly heated,and apply confining stress at a rate of 0.5 MPa/min to the target stress and maintain for 2 h to ensure that the sample reaches a stable state;(3)Perform quasi-stationary water flow permeability test;(4)Unload the confining pressure and keep the temperature at a constant value;and (5) Repeat the second and third steps.A thermocouple immersed in the hydraulic oil inside the confining cell was used to detect the temperature for the heating or cooling step.The permeability of an intact granite is too low to conduct the steady flow test,but it is essential to measure the permeability in the rock matrix including microcracks.The permeability test usually took several minutes to more than an hour to measure,depending on the sample permeability.

To keep the two halves of fractured sample together during permeability tests,the samples were covered by a heat-shrinkable sleeve.In the permeability tests,water injection pressure was lower than the confining pressure to avoid the possible leakage or fluid flow from the gap between the rock sample and the heatshrinkable sleeve.Therefore,the initial water pressure was set to 0.5 MPa.After applying the initial water pressure,a steady-state pressure of 2 MPa was applied to the injection pipe,which make sure the water flowed from the bottom to the top through the fracture.During testing,the axial stress,confining pressure,pump pressure,radial displacement and longitudinal displacement were recorded.

The widely used parallel plates was adopted to measure the permeability of the fractured and intact samples.Based on the Poiseuille equation,linear flow through parallel plates can be formulated by the cubic law (Witherspoon et al.,1980),and the volumetric water flow rate(q)through the fracture is described as

wherepis the water pressure,xis the fracture axis,μis the dynamic viscosity of the fluid,qis the volumetric flow rate,ehis the fracture hydraulic aperture,andwis the width of the fracture(equal to the diameter of the core sample).The water viscosity is 8.89 × 10-4,5.94×10-4,4.31×10-4and 3.09×10-4Pa s under the temperatures of 25°C,45°C,65°C and 90°C,respectively.

2.3.Experimental results

Fig.4 shows the evolution of permeability of the four samples during unloading under different temperatures.The fracture exerts a significant influence on the permeability in the studied granite samples.The permeabilities of the fractured rock samples are generally higher than that of the intact rock sample under the same confining pressure,but the fracture sample 620 under the higher confining pressure (60 MPa) has a lower permeability than the intact sample 621 under the relatively lower confining pressure(40 MPa).The permeability of sample 622-2 is one magnitude larger than the other fractured samples (e.g.621-1),which is explained by the stress-induced asperity damage based on the calculation results from the coupled thermo-mechanical model in Section 4.

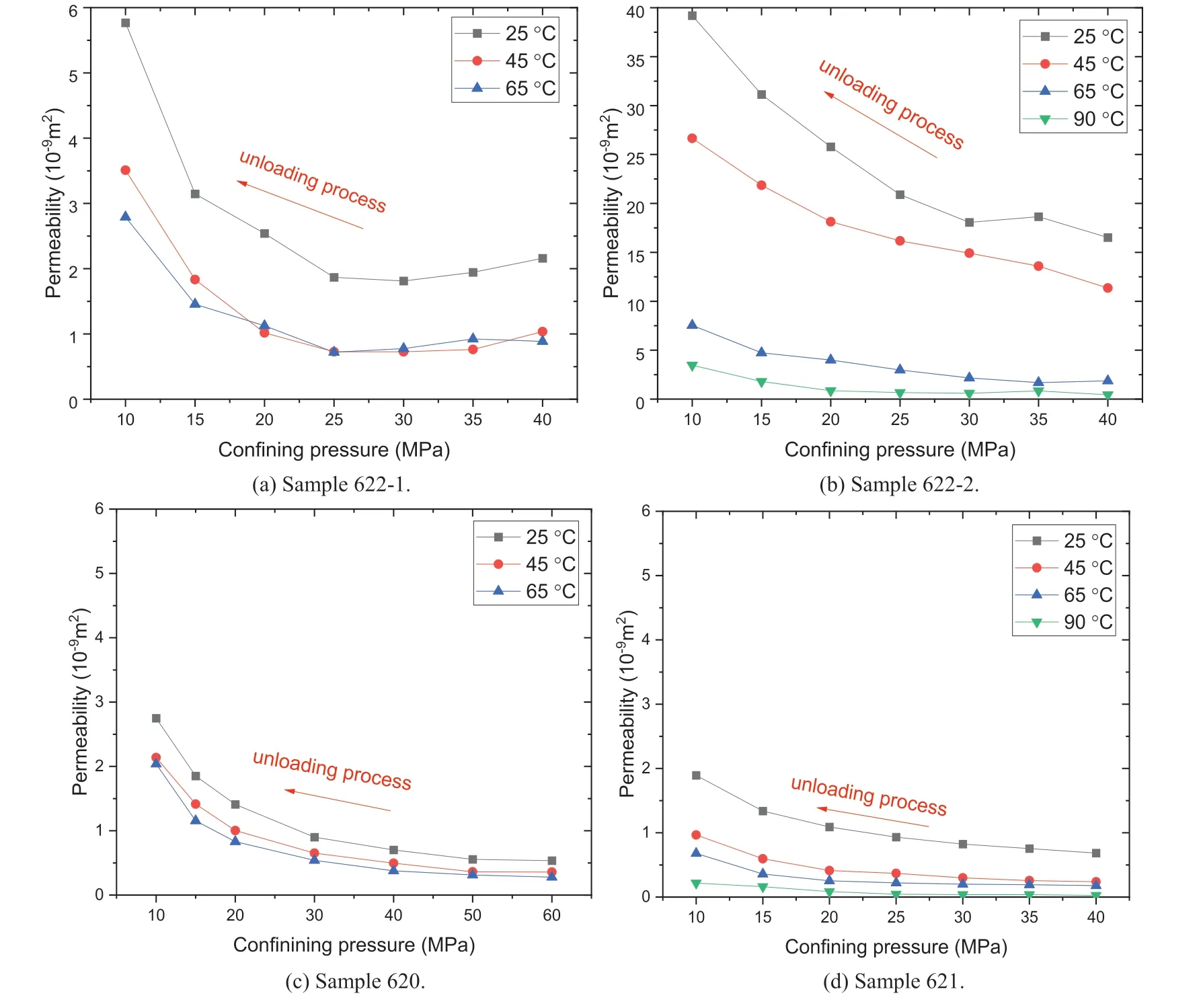
Fig.4.Evolution of permeability of fractured granite samples during unloading under different temperatures.

A similar increasing tendency of permeability is observed for the four samples,i.e.permeability increases with decreasing confining pressures.At room temperature of 25°C,the permeabilities of the four samples 622-1,622-2,620 and 621 are 2.16 × 10-9m2,16.5×10-9m2,0.53×10-9m2and 0.68×10-9m2at the maximum confining pressure,and become 5.77 × 10-9m2,39.2 × 10-9m2,2.75 × 10-9m2and 1.89 × 10-9m2at the confining pressure of 10 MPa,i.e.increase by 2.66,2.37,5.17 and 2.77 times.This indicates that permeability has a strong dependency on confining pressure.Relaxation of confining pressure induces recovery of elastic deformation and then gives rise to fracture opening.

Loading-unloading cycles and temperature also affect the permeability of the four tested rock samples.Under the same confining pressure,the permeabilities of most rock samples gradually decrease with increasing loading-unloading cycles and temperatures.For example,the permeability of the sample 622-2 decreases from 39.2×10-9m2to 3.46×10-9m2after four loadingunloading cycles.Not that the first loading-unloading cycle was performed under the temperature of 25°C,while the fourth loading-unloading cycle was performed under the temperature of 90°C.For the three fractured rock samples,the averageZ2values for the samples 622-1,622-2 and 620 are 0.247,0.28 and 0.304 after compression test,respectively,which are lower than that before the test.This indicates that the asperities on the fracture surface have been damaged and destroyed under the action of temperature and mechanics,making the fracture surface flatter.

It is known that thermally induced volumetric expansion of the fracture sample and the growth of the contact area and high temperature may cause closure of microcracks in the rock matrix and then blocks the existing fluid pathways.However,loadingunloading cycle was performed under different temperatures,and it is difficult to separate the effects of loading-unloading cycles and temperatures on fracture permeability.The relationship between fracture aperture changes caused by mechanical and temperature actions will be discussed in Section 5.1.

3.Empirical model for fracture aperture during unloading phase

Since the 1970s,a number of empirical relationships between normal stress and normal displacement of rock fractures have been proposed based on experimental data (e.g.Goodman,1976;Tsang and Witherspoon,1981;Bandis et al.,1983;Barton et al.,1985),and these empirical models are convenient and widely used in rock engineering.The classic effective stress law for saturated poroelastic media is used to describe the mechanical water-rock interaction in single rock fractures (Rutqvist et al.,2003;Zhao et al.,2018):

whereis the effective stress,andσnis the total normal stress applied on the fracture.Because Eq.(2) deviates from the actual state under high water pressure,an effective stress coefficient was added into Eq.(2) (Biot,1941):

Zhao et al.(2021a) proposed a new effective stress coefficient model for single rough water-bearing fractures in terms of two mechanical parameters,initial normal stiffness and maximum normal closure,and then incorporated the new effective stress coefficient model into the Barton-Bandis constitutive model and built a hydromechanical coupling model,which can be expressed as

wherekn0is the initial fracture normal stiffness;andandunmaxare the normal displacement of the water-bearing rock fracture and maximum normal closure of the rock fracture,respectively.In this study,we can combine Eqs.(4)and(5)with the conditionσn≥p,and expressas

The relationship between the fracture hydraulic aperture (eh)and normal displacement()is described by(Barton et al.,1985):

whereJRCis the joint roughness coefficient,andJRCcan be calculated fromZ2value (Tse,1979):

A theoretical model of hydraulic aperture for rough rock fracture can therefore be expressed as

Once the fracture hydraulic apertureehandJRCare obtained from Eqs.(7) and (8),respectively,kn0andunmaxcan be produced from the fitting results of theeh-σncurve based on Eq.(9).Note that Eq.(9) is suitable for both loading and unloading processes.The hysteresis in the fracture permeability can be considered by Eq.(9),but the model parameters may be different for the loading and unloading processes.

The experiment results were transformed into the variation of the fracture hydraulic apertureehwith the confining stressσn.A comparison of the experiment results and evaluation of the hydraulic aperture predicted by the new model is shown in Fig.5.The best fitting parameters are given in Table 4.With the increasing of temperature and cycles of unloading,unmaxdeceases whilekn0increases.This is because the asperity of the fracture may be damaged during the process of heating and loading,which results in difficulty for further deformation of the fracture.The proposed model of Eq.(9)is proved to be fairly good with unloading-induced permeability enhancement of rock fractures.

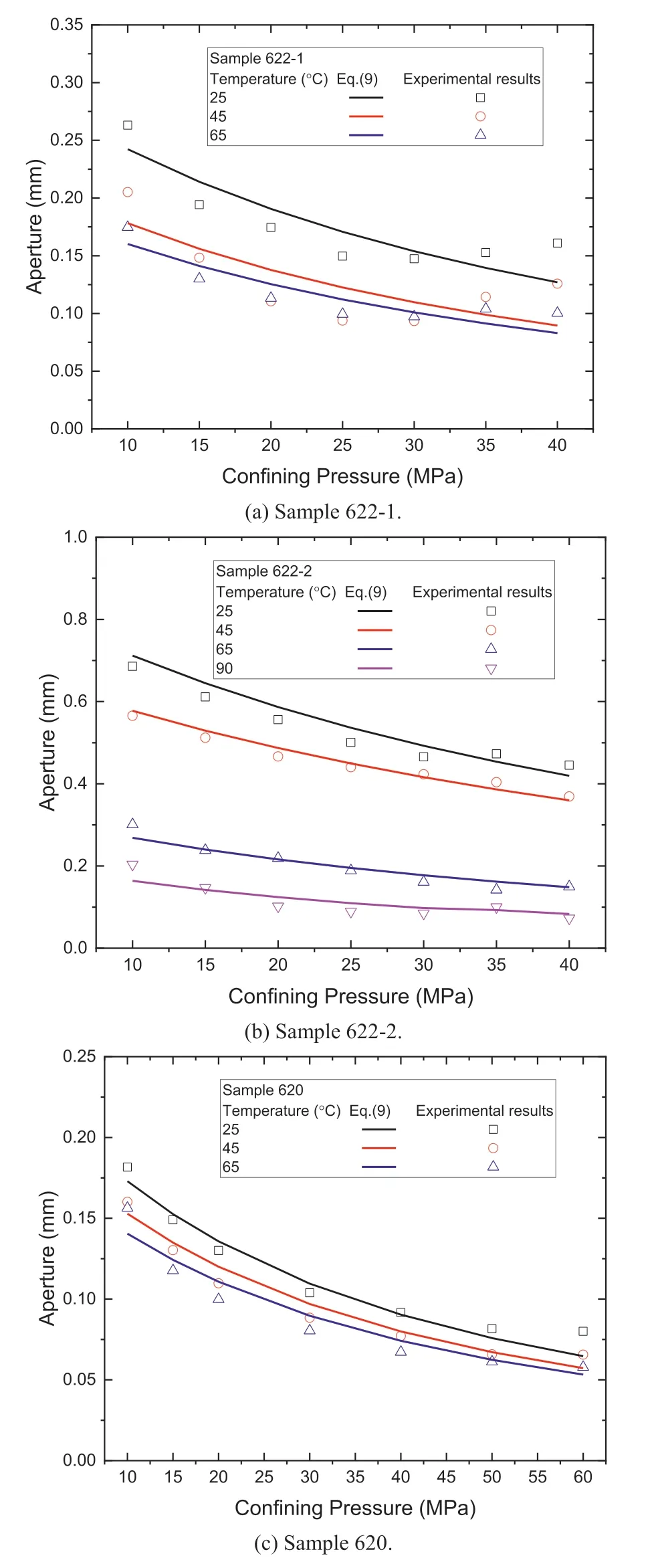
Fig.5.Evolution of fracture aperture during unloading under different temperatures and model prediction by Eq.(9).

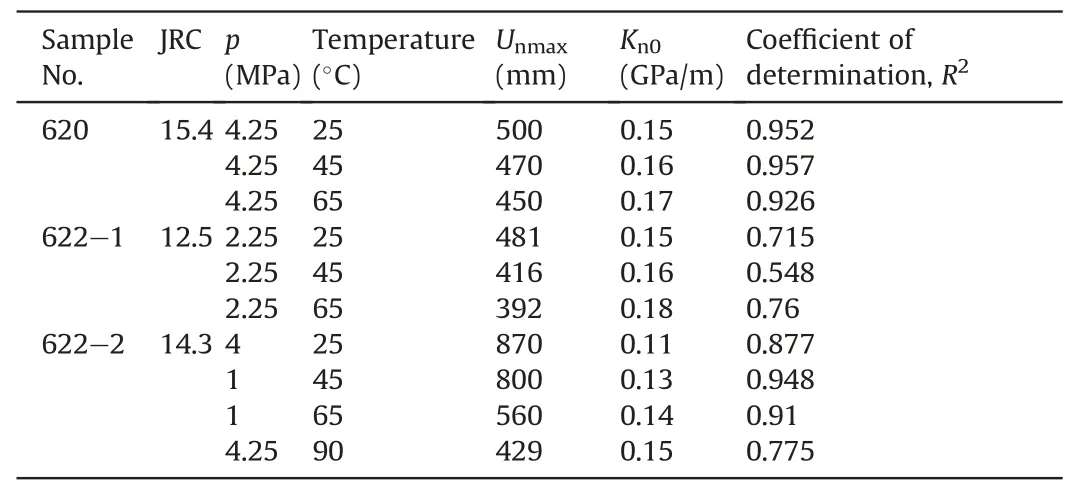
Table 4Parameters used in the empirical model.

4.Coupled thermo-mechanical model for unloading deformation

Based on the fracture deformation model established by Hopkins (1990),Pyrak-Nolte and Morris (2000) and Peng et al.(2020) developed a coupled thermo-mechanical model to take the thermally induced asperity deformation and damage into consideration during constant normal loading,which is used to understand the underlying mechanisms of unloading-induced permeability enhancement of rock fractures under different temperatures in this study.Rock fracture is regarded as two half-spaces separated by a large number of cylindrical asperities with the same radius but varying height,which can be built up based on the scanned data of surface topography (Fig.6).The asperity heights change with the varying stress and temperature.The average displacements(wij)of the half-spaces at asperityicaused by asperityjand asperityiitself are as follows (Hopkins,1990):

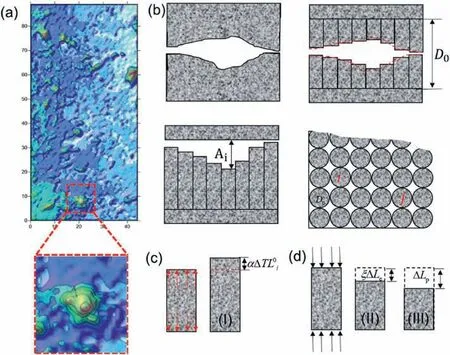
Fig.6.Schematic diagrams of fracture deformation model: (a) Fracture;(b) Simplified fracture in the model;(c) Residual strain in a thermal loading process (I: thermal loading);and (d) Residual elastic and plastic deformation (II: residual elastic deformation;III: residual plastic deformation) (after Pyrak-Nolte and Morris,2000).

whereSiis the area of asperityi,Eis the Young’s modulus,νis the Poisson’s ratio,ais the asperity radius,andrandθare the polar coordinates.

The total displacement at asperityifrom all of the asperities that are in contact is (Pyrak-Nolte and Morris,2000):

wherecrepresents the set of asperities in contact.Elastic-perfectly plastic material was considered as the asperities to include both elastic and plastic parts.

The change of temperature will induce the variation of asperity height and temperature-dependent mechanical parameters,which can cause the deformation and damage of the asperity.In this study,the change in asperity height and Young’s modulus induced by temperature in the absence of stress are (Peng et al.,2020):

whereαis the coefficient of thermal expansion;βis the coefficient of Young’s modulus variation with temperature;E0is the Young’s modulus at the room temperature;ΔTis the change in temperature;andis the original unstressed length of asperityi,which is defined as (Peng et al.,2020):

whereD0is the original distance between the two half-spaces,andAiis the aperture between two half-spaces which can be calculated by the scanned data.During the unloading stage,elastic-perfectly plastic materials were considered for asperities with irrecoverable residual deformation.The residual deformation (ΔLe，m) is proportional to the original deformation (ΔLe) during unloading (Peng et al.,2020):

whereξis a proportionality factor.The residual plastic deformation will not recover when the asperity deformation is in the plastic state or damaged.

Combining Eqs.(10)-(14),the asperity heights and apertures during unloading under constant temperatures are calculated in the following three steps: (1) Thermal loading step: asperity heights and Young’s modulus are calculated at the specified temperature by Eq.(12);(2) Mechanical step: confining stress is increased or decreased,and the displacements of the half-spaces at the individual asperities are calculated by Eqs.(10) and (11);(3)Asperity damage step: the asperity deformation during unloading is calculated by Eqs.(13) and (14),and the asperity heights and apertures are updated.Note that the calculated aperture is mechanical aperture with this method,which also can apply to loading process by changing the mechanical unloading step and final deformation is cumulative.

The coupled thermo-mechanical model is used to calculate the fracture mechanical aperture of the fracture samples during the unloading and heating treatment.A central area of the sample with a size of 45 mm×90 mm is selected to avoid boundary effect.The asperity radius(a)is set at 0.5 mm to balance calculation accuracy and efficiency,thus there are 4050 cylindrical asperities in the model.The parameters in the coupled thermo-mechanical model are presented in Table 5,and most are from the axial compression experiments with the same kinds of sample drilled from the project.Creep effect is also considered following the method developed in Peng et al.(2020).

Table 5Parameters used in the thermo-mechanical model.

Fig.7 compares the experimentally measured and numerically calculated aperture distributions before and after the tests.Whether before or after the test,the aperture distributions are quite similar,indicating that the numerical model can reasonably calculate the changes in fracture apertures under the condition of unloading and heating.Note that some discrepancies exist between the measured and calculated aperture distributions of the sample 622-2,and the reason is the possible leakage between the sample surface and heat-shrinkable sleeve during the experiments.The possibility is also reflected by the fact that the permeability of sample 622-2 is one magnitude larger than other fractured samples.

To compare model prediction and the experimental data,the fracture aperture is normalized with respect to the initial aperture after the confining pressure of 10 MPa is applied.Fig.8 gives the comparison about the fracture aperture during the experiments,and a fairly good agreement is observed between the numerical and experimental curves.Therefore,the thermo-mechanical model can provide a deep and microscopic view about mechanical and thermal damage and properly predict the experimental results,and it is used to explain why the permeability of sample 622-2 is one magnitude larger than the other fractured samples(e.g.621-1).After applying confining stress of 10 MPa,asperity damage occurred on fracture surface,and the more significant asperity damage was observed on the sample 622-1 than 622-2 (Fig.9a),and thus the higher facture closure occurred in the sample 622-1.We divided the fracture into 90 sections along the axis and calculate the average aperture of each section (Fig.9b),and the much higher apertures were observed in the sample 622-2.Therefore,stress-induced asperity damage may play an important role in determining fracture permeability.With parameters changing,some difficult experiment conditions like high temperatures of 150°C or 300°C could be investigated in Section 5.1.

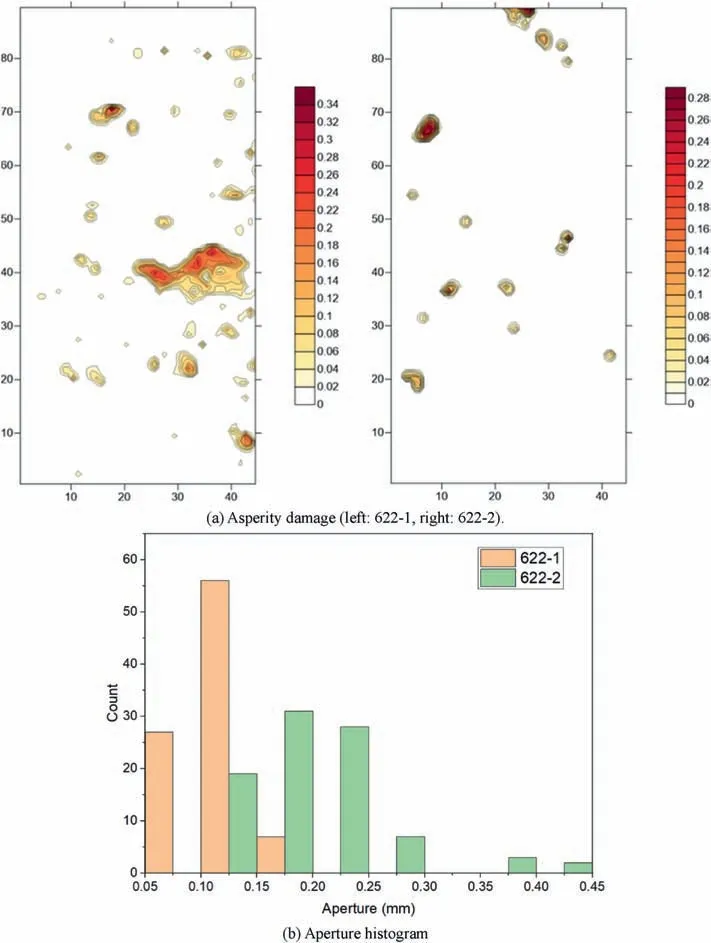
Fig.9.Stress-induced asperity damage on fracture surfaces and aperture distribution.

5.Discussion

5.1.Effect of temperature on rock fracture aperture

In this study,the fractured rock samples experienced a couple of loading-unloading cycles,i.e.the confining pressures increased to the specified value and then decreased to 10 MPa.Each loadingunloading cycle was performed under different temperatures.It is known that fracture morphology changes with increasing loadingunloading cycles,and thus it is difficult to identify the effects of loading-unloading cycles and temperatures on fracture permeability.In addition,much higher temperatures up to 300°C occur in other subsurface projects like geothermal development.Therefore,the coupled thermo-mechanical model in Section 4 is used to investigate the pure thermal effect on rock fracture aperture under unloading.Two scenarios before unloading are considered: (1)fractured samples are first heated and then loaded,and (2) fractured samples are first loaded and then heated.The unloading process is the same for these two scenarios.

Fig.10 shows unloading-induced variation of fracture aperture under different temperatures from 25°C to 300°C.The case of 25°C can be regarded as the pure mechanical effect on fracture aperture,and the fracture aperture further decreases with increasing temperatures.When the temperature is below 90°C,the effect of temperature on fracture aperture is not significant.In other words,the observed reductions in fracture permeability under different temperatures during experiments are mainly due to increasing loading-unloading cycles.When the temperatures are 150°C and 300°C,the effect of temperature on fracture aperture becomes significant.The proposed empirical model Eq.(9) is also used to fit the calculated apertures during unloading under different temperatures,and good fit is obtained (Table 6).This indicates that the empirical model can consider both mechanical and thermal effects on fracture apertures if the model parameters are known or measured.

Table 6Parameters used for fitting the experimental results induced by temperature.

Comparing the two scenarios,similar results are calculated when the temperature is below 90°C,but the second scenario exhibits lower permeability for the temperatures of 150°C and 300°C than the first scenario.This indicates that fracture permeability is dependent on both stress and temperature paths.Peng et al.(2020) showed that fracture permeability decreases during heating,and then slight changes in permeability occur during cooling.Therefore,for tunneling,the unloading-induced fracture permeability recovery should be carefully considered,while the temperature-induced changes in fracture permeability may not be considered necessarily.In contrast,for deep geo-energy projects(e.g.enhanced geothermal system),both stress-and temperatureinduced fracture permeability changes should be considered.

5.2.Implications for water flow into tunnels

There are a number of regional active fault zones at the Layue tunnel construction site,and groundwater infiltrates down along the faults to the deep crust.After heating,the water rises up to form a series of geothermal reservoirs and springs (Wang et al.,2021b).Water inflow is one of the difficult engineering problems during construction of deep tunnels,and a model of predicting water inflow is urgently needed (Zhang et al.,2022).The present experiment and numerical results shed some lights on the evaluation of tunnel water inflow in fractured rock mass.Moon and Fernandez(2010) proposed water inflow for a circular tunnel in fractured rock mass under groundwater level drawdown:

whereKeqis the equivalent hydraulic conductivity of the fractured rock mass,Rzis the vertical influence distance of groundwater level drawdown from the initial groundwater level,Rxis the horizontal influence distance of groundwater level drawdown from the center of tunnel,His the water table above the tunnel,andris the tunnel radius.Farhadian and Katibeh(2017)proposed another equation to describe the relation between conductivity with water inflows on the condition of constant water table for fractured rock:

whereZis the overburden thickness.This empirical model was developed based on the monitoring data in real tunnel projects,which also demonstrates the rapid change of water inflow into tunnels with the increase of permeability.

According to Eqs.(15)-(16),the water inflow linearly increases with increasing permeability of surrounding rocks under constant geometric and hydraulic parameters.Considering the fact that fracture permeability is much higher than rock mass,it is assumed that the equivalent hydraulic conductivity of the fractured rock mass is equal to rock fractures in this analysis.Based on the experimental results,fracture permeability increased by about 2-5 times when confining pressures decreased from 40 MPa or 60 MPa to 10 MPa,and thus the water inflow may increase by the same factors.Therefore,an accurate determination of the permeability variation during unloading is critical to predict the water inflow into tunnels.Of course,discrete element method or other numerical methods can provide more accurate prediction of water inflow during excavation.

5.3.Limitations of this study

In this study,steady-state water flow test was carried out on the intact granite sample,but the inlet and outlet flow rates did not reach the stable stage after one day.The intact granite sample was then loaded to produce microcracks,and thus the steady-state flow test can be completed in a reasonable test time.Therefore,the results for the sample 621 obtained in this way may not represents the permeability of intact granite.Because the permeability of intact granite is very low,it is recommended to use transient-state gas flow method to measure the permeability of intact granite in the future.

Due to the temperature capacity (＜100°C) of the hightemperature triaxial rheological test system ROCK 600-50 VHT,the temperature up to 90°C was considered in this study,while the much higher temperatures of 150°C and 300°C are considered in numerical simulation.In the present experimental design,three fractured rock samples are tested,and the conditions of loadingunloading cycles and temperatures are coupled together.Both high temperatures and loading-unloading cycles can damage the fractured rock samples,and fracture morphology changes after each test.One cannot have the identical fracture morphology before each temperature test,and this is the technical difficult to separate the effect of load-unload cycles and temperature.The effects of loading-unloading cycles and temperatures may be separated in future experimental design,and more experiments for repeatability may also be attempted.

During the permeability test,the water is heated by a long pipe in the chamber before entering the rock sample.Even though the water temperature may be lower than the rock sample,the water can also be quickly heated to the rock temperature considering the low velocity in this study (Zhao and Tso,1993;Zhao,2014).However,the water can be pre-heated to the rock temperature before entering the rock sample in future studies.In addition,the possible leakage between the sample surface and heat-shrinkable sleeve exists in the experiments,especially under low temperatures,which should be avoided as much as possible.

Barton(2020)addressed that rough joints can over-close due to a prior higher stress,or due to temperature increase alone.The results in this study also show that the permeabilities of fractured granite samples decreased with increasing loading-unloading cycles,and recovery of permeabilities during unloading also decreased with increasing loading-unloading cycles.Incorporating the mechanism of over-close into the empirical model will be attempted in future studies.In addition,to quantitatively assess the hysteresis effect of fracture permeability after cyclic loadingunloading or heating-cooling treatments,the recovery rate may be calculated (Wang et al.,2021a,b;Ju et al.,2022).However,the initial permeability before loading is not measured in this study,which should be considered in future experimental design.

6.Conclusions

This study investigates the unloading-induce enhancement of rock facture permeability.A number of permeability tests were performed on the four cylindrical granite samples,three of them with a single artificial fracture,under decreasing confining pressures and different temperatures.A theoretical model with clear physical meaning for fracture hydraulic aperture was developed and validated,and a coupled thermo-mechanical model for fracture deformation was used to understand the influences of stress paths and temperatures on fracture aperture.The following conclusions can be drawn:

(1) The permeability of fracture increases with the unloading of confining pressure but decreases with loading-unloading cycles,which means that permeability of fracture is dependent on stress path.Temperature may affect fracture permeability when it is higher than a certain threshold.

(2) Based on the effective stress law,the theoretical model of fracture hydraulic aperture can well describe the permeability changes in rough rock fracture subjected to loadingunloading cycles and heating.Loading-unloading cycles and heating lead to increase inKnobut decrease inUnmax.

(3) The coupled thermo-mechanical model can consider mechanical and thermal damage and properly predict the experimental results.When the temperature is below 90°C,the changes in fracture apertures caused by mechanical loading or unloading are more significant than temperature.In contrast,thermally induced reductions in fracture apertures become significant when the temperature is above 150°C.Therefore,the thermal and mechanical processes may be decoupled in the temperature range (＜90°C) for tunneling application.

Declaration of competing interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgments

This work is supported by the National Natural Science Foundation of China(Grant Nos.U2067203 and 42277140)and Tsinghua University Initiative Scientific Research Program (Grant No.2022Z11QYJ006).