Influence of pump brightness,self-Kerr and cross-Kerr effects on the entanglement of partially degenerate triple-photon state

Da Zhang and Zhihong Ren

School of Physics and Information Engineering,Shanxi Normal University,Taiyuan 030031,China

Abstract Recently,partially degenerate triple-photon states (TPS) generated by the third-order spontaneous parametric down-conversion have been observed in a superconducting cavity(2020,Phys.Rev.X 10,011011).Their non-Gaussian entanglement properties,characterized by a series of high-order covariance matrices,have also been theoretically revealed.Here,we use the non-Gaussian entanglement criterion proposed in(2021,Phy.Rev.Lett.127,150502)and the logarithmic negativity to study the effect of pump brightness,self-Kerr and cross-Kerr interactions on the entanglement of partially degenerate TPS(PDTPS).We find that the brighter the pump,the easier the entanglement of PDTPS leap to higher-order covariance matrices.Although both self-Kerr and cross-Kerr interactions induce nonlinear phase shifts and weaken the entanglement of PDTPS,cross-Kerr interactions can effectively raise the threshold of entanglement loaded on the third-order covariance matrix.These results can contribute to our understanding of the mechanism of the generation of unconditional non-Gaussian entanglement.

Keywords: non-Gaussian entanglement,triple-photon states,high-order covariance matrices,cross-Kerr effect

The preparation of non-Gaussian entangled states has attracted considerable interest over the past two decades,as they are critical components in realizing continuous-variable universal quantum computation[1–3]and have demonstrated exceptional performance in a variety of quantum information protocols,including quantum teleportation [4,5],quantum cloning [6],quantum key distribution [7] and quantum metrology [8].One of the standard approaches for creating non-Gaussian entangled states is to apply non-Gaussian operations such as photon addition or subtraction to Gaussian states [9–13],and the resultant non-Gaussian entanglement can be witnessed by the negativity of the Wigner function[14].Another common method for generating non-Gaussian entanglement is higher-order spontaneous parametric downconversion (SPDC),for instance,the 3rd-order SPDC,in which a pump photon is converted into triplet photons with phase matching condition.The main advantage of the latter over the former is the certainty of the preparation process.

According to whether the triplet photons are degenerate or not,they can be divided into three categories: fully degenerate [15],partially degenerate [16] and fully nondegenerate [17] triple-photon states (TPS).For the fully nondegenerate case,if one or more bright coherent states are seeded into TPS,the resulting state will degenerate into a Gaussian state [18].It has been shown that the spontaneously generated fully nondegenerate TPS is a Greenberger–Horne–Zeilinger state with super-Gaussian statistics[19],and its entanglement structure has been systematically analyzed by employing different classes of inseparability criteria[20–22].The concept of nonlinear entanglement was developed in[16]based on the nonlinear correlation features of two modes in partially degenerate TPS (PDTPS).By applying the positive-partial-transposition criterion to the high-order covariance matrices [23],we revealed the mechanism implied by nonlinear entanglement,that is,PDTPS is entangled on a series of 3nth-order covariance matrices and there are competition and coexistence between them.

Recently,three kinds of TPS have been observed in superconducting cavity with ultra-strong nonlinearity [24].Other potential implementation platforms for these processes include optical fibers[25]and optical waveguides[26].When light interacts with materials,the high-order nonlinearity in the materials unavoidably induces other nonlinear interactions,such as self-Kerr and cross-Kerr effects[15,24].The impact of these effects on the entanglement of PDTPS,however,has not been studied.In this work,we employ the positive-partialtransposition criterion proposed in [23] and the logarithmic negativity to study the influence of these nonlinear effects and the pump brightness on the entanglement of PDTPS.We find that the brighter the pump,the easier it is for the entanglement of PDTPS to transition to higher order covariance matrices.Self-Kerr interactions suppress the partially degenerate 3rdorder SPDC and reduce the threshold of entanglement loaded on the 3rd-and 6th-order covariance matrices.The influence of the cross-Kerr interactions on the entanglement of PDTPS is similar,but it will increase the threshold of entanglement loaded on the 3rd-order covariance matrix.

Let us start our analysis by considering the physical process described by the Hamiltonian [24]

Different from the 2nd-order SPDC,the amplitude of the pump beam has a non-negligible effect on the 3rd-order SPDC The photon number distribution of the two-mode squeezed vacuum is convergent whether the parametric approximation is used or not.However,for the 3rd-order SPDC,the parametric approximation holds only when ξ is less than a certain threshold [27].Otherwise,non-physical conclusions,such as photon number divergence,are obtained.

Fig. 2.Evolution of(a)and(b)versus ξ for different pump amplitudes.

Fig. 3.Evolution of(a),(b) and EN (c) versus ξ for different κ1.

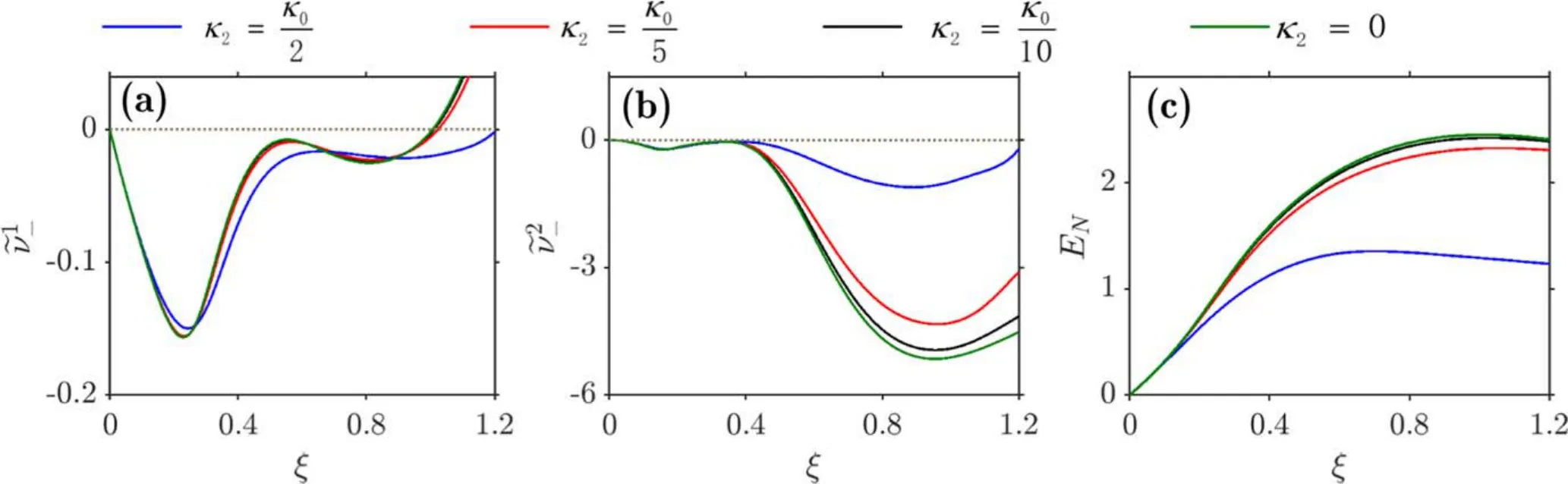
Fig. 4.(a),(b) and EN (c) evolved with ξ for different κ2.

Fig. 5.Evolution of(a),(b) and EN (c) with ξ for different κ1 and κ2.

In summary,we introduced some basic properties of PDTPS and then studied the influences of the pump brightness,self-Kerr and cross-Kerr interactions on their entanglement.We found that the brighter the pump beam,the easier it is for the partially degenerate triple-photon state entanglement to jump to higher-order covariance matrices.The presence of the self-Kerr and cross-Kerr effects alone has exactly opposite effects on the non-Gaussian entanglement loaded on the 3rd-order covariance matrix,with the former lowering the threshold for the presence of entanglement while the latter raising it.The existence of these nonlinear effects does not induce other types of high-order correlations,but reduces the entanglement of PDTPS.These results can further improve our understanding of the process of experimental preparation of PDTPS [24,28].

Acknowledgments

This work was supported by the National Natural Science Foundation of China(12204293)and Applied Basic Research Program in Shanxi Province (No.202203021212387).