Derivative-extremum analysis of current-potential curves showing electrochemical kinetics in the full reversibility range

Fengjun Yin,Hong Liu

a Chongqing Institute of Green and Intelligent Technology,Chinese Academy of Sciences,Chongqing 400714,China

b Key Laboratory of Reservoir Aquatic Environment,Chinese Academy of Sciences,Chongqing 400714,China

c China University of Chinese Academy of Sciences,Beijing 100049,China

Keywords:Reversibility Electrochemical kinetics Half-wave potential Derivative-extremum analysis Parameter determinations

ABSTRACT Derivative-extremum analysis (DEA) of j-E curves is a newly proposed method of half wave potential(E1/2) and activation feature extraction from steady-state voltammetry.Here,the DEA is demonstrated to be valid in the full range of reversibility using numerical simulations with a derived universal electrode equation,providing a novel perspective of electrochemical kinetics in the reversibility domain.The results reveal that E1/2 is a better choice of the reference potential instead of equilibrium potential (Eeq) in electrode equations,especially since Eeq is meaningless in an irreversible case.The equations referenced with standard potential, E1/2 and Eeq,are summarized in three tables,and their applications in parameter determinations are specified.Finally,reversibility is proved to be a relative measure between kinetic slowness and mass transport of electroactive species,and the reversibility classifications are proposed according to the DEA feature in the reversibility domain.This work,based on the DEA principle,refines the electrode equation forms and generalizes their applicability in the full range of reversibility.

Steady-state current (j)-potential (E) voltammograms provide an easy-to-follow technique for extracting the kinetic information of an electrode reaction because of the simplicity of steady-state kinetic theory.In mathematics,steady-state voltammetry avoids charging current interference [1] and possesses time-independent mass transport processes between the electrode surface and the bulk solution [2].

Reversibility is a basic scale in the recognition of the reactivity of a reaction,and the electrode equations are often divided into reversible,irreversible andquasi-reversible types [3].The electrode equations and their applicability are dependent on the reference potential against which the potential is quantified,e.g.,standard(or formal) potential,equilibrium potential (Eeq) and half wave potential (E1/2).The electrode equations are multifarious in terms of reversibility and reference potential.If possible,it is strongly desired to unify all the electrode equations and elaborately differentiate their applicability in steady-state voltammetry.

“Reversible” and “irreversible” are two extreme cases; the former is only dependent on thermodynamics,while the latter is fully governed by kinetics.The variations in the kinetic features embodied in the steady-state voltammograms between the two cases have long been absent from a quantitative measure.E1/2is an important indicator of reaction occurrence,whereas its physical significance in the full range of reversibility has yet to be clarified.

Recently,we found that the peak point of the derivative of aj-Ecurve in irreversible cases corresponded to a special kinetic state,where the peak potential was demonstrated to beE1/2and the peak value designated the activation feature of a reaction driven by potential [4].Additionally,this derivative extremum analysis (DEA)can be easily demonstrated to be applicable in reversible cases; although,the peak potential and peak value have different expressions.In this work,DEA was further proven to be valid in the full range of reversibility through numerical simulations using a derived universal electrode equation,and it provided a new perspective in understanding the kinetic feature evolution in the reversibility domain.We reorganized the equations referenced with formal potential,EeqandE1/2in the full reversibility scale and elucidated their applicability.Reversibility classifications were proposed according to the variation trends of DEA in the reversibility domain.

To investigate the DEA applicability,we first derived a universal electrode equation in the full range of reversibility with three premises as follows: the uniformly accessible electrode treating the mass transport process,a large excess of supporting electrolyte to eliminate the migration effect,and a Nernst diffusion layer with stirring maintaining the bulk concentration constant.The cathodic current is defined as positive,and the anodic current is negative.

The most basic equation describing an elementary reaction O+ne-=R is an original Butler-Volmer form (Eq.1) [5]:

wherek0is the standard rate constant,E0is the standard potential and can be replaced by formal potential in practice,c*Oandc*Rare the oxidant and reductant concentrations on the electrode surface,respectively,αandβare the electron transfer coefficients (ETCs),α+β=1,Fis the Faraday constant,andf=F/RT,where R is the ideal gas constant,andTis the temperature in Kelvin.All the symbols are defined in Table S1 (Supporting information).

Eq.1 is difficult to use sincec*Oandc*Rcan hardly be defined and measured.However,c*Oandc*Rcan be replaced by introducing the mass transport relationships of reactants (O and R).In the steady state,the reaction rate equals the mass transport fluxes of O and R between the electrode surface and bulk solution (Eqs.2)and 3:

wherecbOandcbRare the concentrations in bulk solution,mis the mass transport coefficient,Dis the diffusion coefficient,andδis the diffusion layer thickness.The maximum transport fluxes of O and R are expressed as the cathodic and anodic limiting current densities (jl,candjl,a),which are obtained atc*Oandc*Rreaching zero (Eq.4):

Introducing Eq.4 into Eqs.2 and 3 obtains (Eq.5):

Finally,introducing Eq.5 into Eq.1 derives the following equation with the potential referenced againstE0(Eq.6):

This equation is a universal form in consideration of the concentration polarization in the full range of reversibility,which excludes any simplification of kinetic factors.The electrode geometric size information can be introduced to the equation by using the analytical expressions of the diffusion layer thickness [6].All other equation forms,such as reversible and irreversible,in the presence of only O or R,and with equal mass transport coefficients,can be simplified from this equation.For example,in the presence of only O,jl,a=0,Eq.6 can be simplified to the following well-known equation proposed by Mirkin and Bard (Eq.7) [2]:

Under the premise of equal mass transport coefficients(mO=mR),Eq.6 can be transformed to the following form as reported by Molinaet al.(Eq.8) [6]:

whereη=nf(E-E0),=k0/mOandμ=cbR/cbO.

If specifying the electrode geometric size inmOandmR,more detailed electrode equations hold for hemispherical and disc microelectrodes [3,7].Here,the electrode equations referenced withE0in the full range of reversibility are summarized in Table S2(Supporting information).

The DEA derivation in reversible case is shown as follows.To obtain the reversible equations,Eq.6 is transformed to the following form (Eq.9):

Under reversible conditions,mO/k0→0 andmR/k0→0.We proved thatmo/k0exp(αnf(E-E0))in the first term andmR/k0exp(-βnf(E-E0))in the second term are negligible; the detailed derivation is shown in Supporting information.Thus,a reversible equation form can be simplified from the above equation:

By solving for the extremal solution of the derivative of Eq.10,we derived the expressions of the peak potential (EP) and peak vale(PV) in the dj/dEcurve and demonstrated thatEPwas identical toE1/2.The detailed derivation process is shown in the Supporting information.

The DEA derivation in irreversible case is shown here.To date,the reported irreversible equations have basically applied the forms usingEeqas the reference potential.Eq.6 can be transformed to aEeq-referenced form.The exchange current density(j0) is an equilibrium current at the initial state wherec*O=cbOandc*R=cbRand has the following expressions (Eq.13):

In combination with the expressions ofj0,jl,candjl,a,the cathodic and anodic terms in Eq.6 can be rearranged as follows (Eqs.14 and 15):

Please note that the above transformations are only valid atcbOandcbRare not zero.Based on the above relationships,Eq.6 can be transformed to the following form referenced withEeq(Eq.16):

In the irreversible case,j0is so small that a very high overpotential is required to drive the reaction occurrence.Thus,the term exp(βnf(E-Eeq))for the cathode reaction is negligible,and exp(-αnf(E-Eeq))for the anode reaction is negligible.Eq.16 can be simplified to the following cathodic and anodic irreversible equations (Eqs.17 and 18):

Cathode:

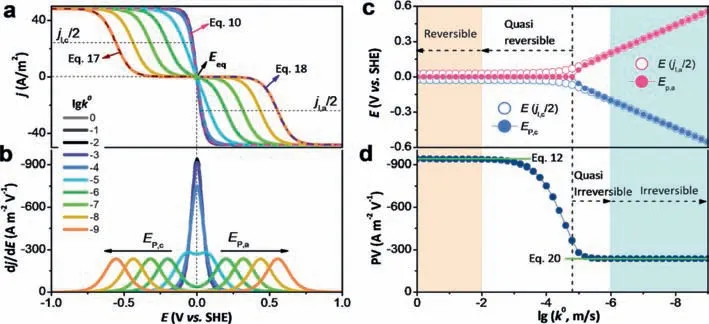
Fig.1.Calculation results of Eq.6 with lgk0 varying from 0 to–9, cbO=cbR=10 mol/m3,and mO=mR=5×10–5 m/s, n=1, E0=0 V, α=0.5 and T = 25 °C: (a) j-E curves,the dashed lines were calculated from the reversible equation (Eq.10) with lgk0=0 and irreversible equations (Eqs.17 and 18) with lgk0=–9,(b) associated dj/dE curves,(c)peak potential (EP) and potentials at jl,c/2 and jl,a/2 plotted vs. lgk0,and (d) PV plotted vs. lgk0,the lines were calculated from the reversible and irreversible expressions of PV.

Anode:

These equations have similar forms to those derived from the multielectron and multistep irreversible process with one sole rate-limiting step [4].The DEA applicability in irreversible cases has been demonstrated by solving for the extremal solution of the derivatives of Eqs.17 and 18 in a previous work [4].The expressions ofE1/2and PV in irreversible cathodic reactions are shown as follows (Eqs.19 and 20):

In both reversible and irreversible cases,Epis identical toE1/2,and PV has a unit of A m-2V-1and can serve to characterize the kinetic slowness of a reaction driven by potential.However,E1/2and PV have different expressions in the two cases.

Furthermore,the DEA simulations in the full range of reversibility using Eq.6 are calculated to show the DEA in the transition range between reversible and irreversible cases,because mathematically solving for the derivatives of Eq.6 is too difficult.In a given scenario,a set ofj-Ecurves withk0values varying from 0 m/s to 10-10m/s are calculated first,then the dj/dEcurves are calculated,and theEpand PV values are extracted from the peak points of dj/dEcurves.Finally,the plots ofEpand PVvs.lgk0are obtained.For all the calculations,n=1,α=β=0.5,E0=0 Vvs.SHE andT= 25 °C.The other parameter values (mO,mR,cbOandcbR) are presented in the figure titles.The calculations were performed using MATLAB 2012a.

The calculation results in the presence of both O and R are shown in Fig.1.Figs.1a clearly presents the variation trend of thej-Ecurves from the reversible to irreversible case: in the condition ofk0＞10-4m/s,the curves approached the calculation result of the reversible equation (Eq.10) withk0=1 m/s; oncek0was small enough,namely,k0=10-9m/s,the curves were consistent with the results of irreversible equations (Eqs.17 and 18).In Fig.1b,the associated dj/dEcurves show that the peak point gradually shifts from one point in the reversible case to two peak points.The isolation degree between the cathode and anode reactions can be characterized by the distance between the two points and is intensified ask0decreases.

To further investigate the relationship betweenEpandE1/2,theEpand the potentials ofjl,c/2 andjl,a/2 were plotted in Fig.1c.Epchanged suddenly from the reversibleE1/2feature,namely,the potential of (jl,c+jl,a)/2,to the irreversibleE1/2feature,namely,the potentials ofjl,c/2 andjl,a/2.In Fig.1d,the PV gradually shifts from the reversible case,as designated by the line calculated from Eq.12 to the irreversible case designated by the line calculated from Eq.20.These results confirmed that DEA is applicable in most cases in the presence of both O and R,except for a narrow transition range between reversible and irreversible cases where the exactE1/2can hardly be defined.In light of the variation features ofEpand PV,the exact reversible and irreversible ranges can be defined as shown by the colored regions.

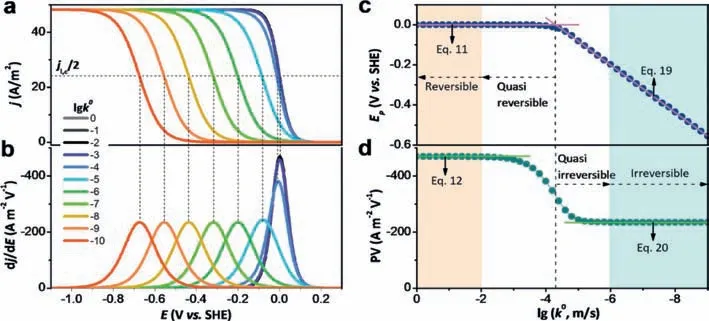
Fig.2.Calculation results of Eq.6 with lgk0 varying from 0 to–10, cbO = 10 mol/m3, cbR = 0, mO = mR=5×10–5 m/s, n=1, E0=0 V, α=0.5 and T = 25 °C: (a) j-E curves,(b) associated dj/dE curves,(c) Ep vs. lgk0 and (d) PV vs. lgk0,the lines were calculated from the reversible and irreversible expressions of Ep and PV.

Table 1 Electrode equation forms referenced with E1/2 in the full range of reversibility.

Table 2 Electrode equation forms referenced with Eeq in the full range of reversibility.

The DEA simulation results in the presence of only O are shown in Fig.2.Figs.2a and b demonstrate that theEpin the dj/dEcurves corresponded to the potential ofjl,c/2 in thej-Ecurves in the full range of reversibility.Thus,Epis invariably identical toE1/2in this case.TheEpvs.lgk0plot in Fig.2c also presented a sudden change from the reversibleE1/2feature to the irreversibleE1/2feature.In Fig.2d,PV gradually shifts from the reversible case to the irreversible case.

Finally,the above DEA simulation results confirmed thatE1/2is a kinetically special potential corresponding to the positions of the half limiting current in thej-Ecurves and the peak point in the dj/dEcurve.DEA is a general method to extractE1/2as well as PV in the full range of reversibility.The variation trends ofE1/2and PV along thek0axis can give a classification criterion ofquasi-reversible andquasi-irreversible regions,namely,(i)quasi-reversible:E1/2is approximate to the reversibleE1/2value;(ii)quasi-irreversible:E1/2is approximate to the irreversibleE1/2value.

The above calculations indicate thatE1/2is a good choice of reference potential because it can be steadily determined in the full range of reversibility.Here,the electrode equations referenced withE1/2in the full range of reversibility are summarized in Table 1.The reversible equation form (Eq.21) can be obtained by combining Eqs.10 and 11:

TheE1/2-referenced equations of irreversible cathode and anode reactions can be derived by introducing the irreversible expression ofE1/2(Eq.19) into Eqs.17 and 18,respectively (Eqs.22 and 23):

Cathode:

Anode:

In irreversible cases,it is not necessary to distinguish the equations in the presence of both O and R and in the presence of only O because the anode and cathode reactions are evidently separated from each other.

Inquasi-reversible/irreversible cases,E1/2-referenced equations are not available because the exact expression ofE1/2and PV can hardly be derived.Nonetheless,DEA is still applicable.

Furthermore,the electrode equations referenced withEeqin the full range of reversibility are summarized in Table 2.The reversible equation is derived as follows.Simultaneously,dividing byj0in the numerator and denominator of Eq.16,it becomes (Eq.24):

In the reversible case,j0is so large that 1/j0is negligible.The above equation can be transformed to the following reversible form (Eq.25):

Although irreversibleEeq-referenced equations are derived and are as given in Table 2,users should be highly cautious about their applications.The applications ofEeqandj0are introduced in the following part.TheEeqused as a reference potential in kinetics is the open circuit potential of an electrode reaction,which satisfies the Nernst relationship (Eq.26):

At this potential,the cathodic and anodic rates reach balance and are expressed as exchange current density (j0) (Eq.27):

It is obvious thatEeqandj0are relevant only in the presence of both O and R.OncecbOorcbRapproaches zero,Eeqandj0in Eqs.26 and 27 become an infinite problem without explicit value.Moreover,Eeqis experimentally indeterminable in irreversible cases because the zero current is not a point but a wide gap between the cathode and anode reactions (Fig.1).Thus,Eeqandj0are applicable for the kinetically fast reactions in the presence of both O and R.

Despite this,Eeqandj0are still frequently applied in irreversible cases.Here,we demonstrate thatEeqandj0are an interdependent pair in irreversible cases.Choosing any potential in the zero current stage as a formal equilibrium potential (E*eq),the deviation ofE*eqfrom the theoreticalEeqcan be described byEeq=E*eq+ΔE.Introducing it to the irreversible equation (Eq.17)obtains Eq.28:

It revealed that the application ofE*eqonly results in the variation ofj0.In irreversible cases,Eeqis indeterminable and is usually determined as the onset potential,the lowest potential where the faradaic current is observed [8].Therefore,thej0value has little physical significance in irreversible cases.If selecting the sameEeqvalue,j0is still useful to compare the activity of a reaction between different conditions or catalysts.

In addition,the activation overpotential (ηact) in the irreversible case is also meaningless because it is dependent on thej0andEeqvalues.Nonetheless,the overpotential analysis of the mass transport effect is feasible,viz.,the concentration overpotential assigned to reactant mass transport [9] and the pH overpotential assigned to H+/OH-transport [10],because the overpotential partE-Eeq-ηacthas excluded theEeqvalue.

Currently,theEeq-referenced equations are typically used to fit thej-Ecurves for parameter determinations,such as the Tafel fitting method and a recently reported nonlinear fitting method [11].The Tafel method either directly ignores the mass transport effect by fitting a narrowj-Ecurve range [12,13] or corrects the mass transport current part to improve the accuracy of parameter values [14–16].The nonlinear fitting method is performed based on Eqs.17 or 18 to fit the entirej-Ecurve range,which overcomes the disadvantages of the Tafel method to obtain reliable ETC,j0andjlvalues.

TheE1/2-referenced equations are more significant for quantifying the activity of irreversible reactions.The information ofEeqandj0is involved inE1/2(see Eq.19) to reveal the priority of reaction occurrence.The nonlinear fitting method can also be performed using the irreversibleE1/2-referenced equations and obtain the values of ETC,E1/2andjl.In Fig.S1 (Supporting information),the nonlinear fitting results of the two types of equations (Eqs.17 and 22)showed that the fitting lines were totally identical and could well fit the entirej-Ecurve.All the parameter values (Table S3 in Supporting information) determined from Eq.22 have a narrow 95%confidence interval,and the intervals of ETC,E1/2andjl,aare less than 1.10%,0.06% and 0.25%,respectively.This confirms that the nonlinear fits based on the irreversibleE1/2-referenced equations are a reliable parameter determination method of irreversible reactions.

Moreover,the irreversibleE1/2-referenced equations are also applicable in conditions deviating from the irreversible case,wherein the ETC reveals reversibility variations.The reversibleE1/2-referenced equations are the special form of irreversibleE1/2-referenced equations with ETC equal to 1.With the aid of micro- and nanoscale electrode techniques to measure steady-state voltammograms [17],the practical application scope of irreversibleE1/2-referenced equations can be greatly extended.

Importantly,the variation trends ofE1/2and PV in DEA in the reversibility domain provide classification criteria of reversible,irreversible,quasi-reversible andquasi-irreversible cases.Here,the influences of transport coefficients (mOandmR) and concentrations (cbOandcbR) on the reversibility classifications are investigated.In the presence of only O,the effect ofmOwith ak0scale is shown in Figs.3a–c.The reversible and irreversible ranges presented by the colored regions gradually shift toward the decreasing direction of lgk0asmOdecreases from 10-4m/s to 10-6m/s.The transition region can be divided intoquasi-reversible andquasi-irreversible parts bounded by lgmOas indicated by the dashed lines in Figs.3a–c.

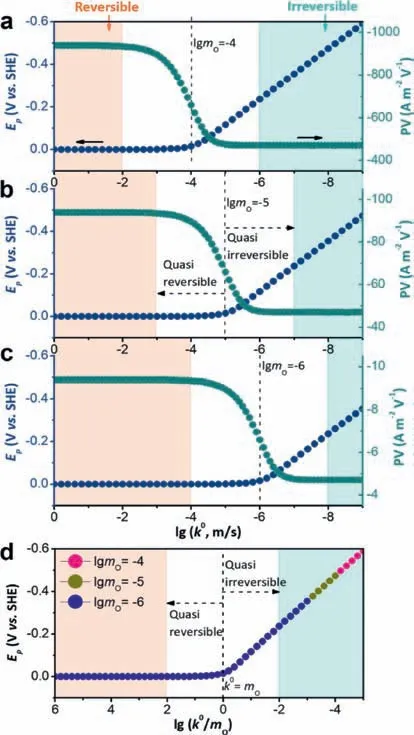
Fig.3. Ep and PV vs. lgk0 plots in the presence of only O calculated from Eq.6 with cbO=10 mol/m3, n=1, α=0.5, E0=0 V and T = 25 °C: (a) lgmO=–4,(b) lgmO=–5,and (c) lgmO=–6; (d) plots of Ep in Fig.(a–c) vs. lg(k0/mO).

TheEpvs.lgk0plots in Figs.3a–c were redrawn using a logarithmick0/mOscale as shown in Fig.3d,and the results at differentmOconditions were the same.It revealed thatk0/mOwas a dimensionless parameter and the most basic scale for reversibility classification.Hence,reversibility was a relative measure between reaction kinetics and mass transport of electroactive species.Roughly,

k0/mO≫1: reversible

k0/mO≪1: irreversible

In theory,the full “reversible” and “irreversible” are two extreme cases that can hardly be reached,and all the reactions may be regarded asquasi-states [3].Here,a detailed classification of the effectivelyquasi-reversible andquasi-irreversible cases was proposed,as listed in Table 3,namely,

100＞k0/mO＞1:quasi-reversible,whereE1/2approaches the reversibleE1/2value

0.01＜k0/mO＜1:quasi-irreversible,whereE1/2approaches the irreversibleE1/2value

Thek0scale for the reversibility classification is dependent on themOand/ormRvalues.In practice,the diffusion coefficient ormass transport coefficient values of most solutes rarely differ by more than one order of magnitude.The diffusion coefficient usually has a magnitude of 10-9m2/s [18,19],and the mass transport coefficient has a magnitude of 10-5m/s [20].The reversibility classification in terms of thek0scale withmO=10-5m/s (see Fig.3b)is listed in Table 3.

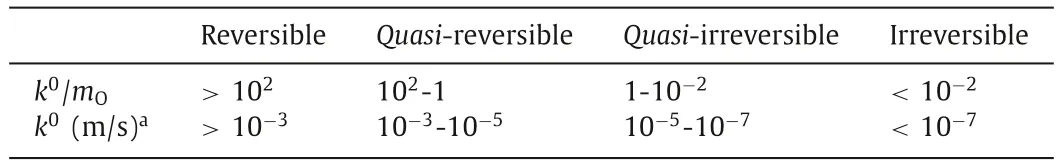
Table 3 Reversibility classifications in terms of k0/mO and k0 in the presence of only O.

In addition,j0,in comparison to the transport limiting current(jl,corjl,a),is also frequently applied to the quantification of reversibility in electrochemistry [21].The ratio betweenj0andjl,ccan be derived from Eqs.4 and 27 as follows (Eq.29):

This indicates thatj0/jl,cis an extended form ofk0/mOthat involves an extra concentration term.Obviously,j0/jl,cis applicable in the presence of both O and R and is identical tok0/mOin the condition ofcbO=cbR.The DEA calculation result under the condition ofcbO=cbRis shown in Fig.S2 (Supporting information),confirming that the reversibility scale is independent of the concentration extent.Under the condition ofcbO/=cbR(Fig.S3 in Supporting information),the reversible and irreversible regions can be well identified but thequasi-state regions for anode and cathode reactions become very complicated.

In this work,numerical simulations of DEA using a derived universal electrode equation successfully verified the validity of DEA in the full range of reversibility and gained panoramic insight into electrochemical kinetics.The equations referenced withE0,E1/2andEeqin conjunction with their applicability were reorganized in three tables in terms of the reversibility scale.Reversibility classifications in terms of the variation features of DEA in the reversibility domain were proposed.

The outcomes of this work are especially important for reactions suffering from mass transport limitations.One can apply the DEA method to extractE1/2and PV from the steady statej-Ecurves.E1/2is a potential feature parameter pronouncing the priority of reaction occurrence on a potential scale.PV is an activation feature parameter revealing the kinetic slowness driven by potential.Alternatively,the nonlinear fitting method ofj-Ecurves using the irreversibleE1/2-referenced equations can obtain reliable ETC,E1/2andjlvalues.In summary,theE1/2-referenced equations have wide application scope in the presence of only O,while theEeq-referenced equations are primarily useful for the kinetically fast reactions in the presence of both O and R.Users should be highly cautious about the applications ofEeqandj0in irreversible cases because they are an interdependent pair and have little physical significance.

Declaration of competing interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgment

This work was financially supported by the National Natural Science Foundation of China (Nos.52131003,52170059,51808526,51727812).

Supplementary materials

Supplementary material associated with this article can be found,in the online version,at doi:10.1016/j.cclet.2022.01.078.