扩散段结构对非对称文丘里施肥器旋涡特征及吸肥性能的影响

王振华，胡贵荣，刘宁宁，刘娉楠，曹 缘，张栋楠

扩散段结构对非对称文丘里施肥器旋涡特征及吸肥性能的影响

王振华，胡贵荣，刘宁宁，刘娉楠，曹 缘，张栋楠

（1. 石河子大学水利建筑工程学院，石河子 832000；2. 现代节水灌溉兵团重点实验室，石河子 832000；3. 兵团工业技术研究院，石河子 832000）

为减小非对称文丘里施肥器扩散段旋涡区的能量耗散，提升非对称文丘里施肥器在低压灌溉系统中的吸肥性能，该研究通过物理试验与数值仿真，对比分析了直线扩散段与弧形扩散段的非对称文丘里施肥器的工作性能差异。结果表明：将非对称文丘里施肥器扩散段设置成弧形结构有利于减小旋涡区面积及强度，提升非对称文丘里施肥器吸肥性能。与直线扩散段结构相比，弧形扩散段的旋涡区面积减小28.41%～42.37%，旋涡强度减小6.64%～35.65%，吸肥效率提升48.15%～98.25%，提升幅度随进出口压差的增大逐渐减小。旋涡对非对称文丘里施肥器吸肥性能具有显著影响（≤0.05），减小旋涡区面积或强度、增大旋涡区边界到喉部的距离等均能提升非对称文丘里施肥器吸肥性能。研究结果可为非对称文丘里施肥器的结构设计提供参考。

CFD；数值分析；文丘里施肥器；扩散段结构；吸肥性能；旋涡

0 引 言

低压灌溉大幅度降低了灌溉系统运行成本，在国家“低功耗农业”发展趋势下，是未来滴灌发展的重要手段[1-2]。施肥设备是滴灌系统中重要的组成部分，其性能好坏直接关系到灌溉质量[3-4]。目前常用的施肥设备有压差施肥罐、文丘里施肥器、水肥一体机等，其中文丘里施肥器因结构简单，价格便宜，无需外加动力等优点被广泛应用在农业灌溉中[5-6]。现有文丘里施肥器存在扩散段旋涡较大，局部水头损失明显，吸肥临界压力高等问题[7]。基于此，研究文丘里施肥器内部旋涡特征及其吸肥性能对结构形式的响应关系对开发适用于低压滴灌的文丘里施肥器具有重要意义。

国内外对文丘里施肥器的研究主要聚焦于对称文丘里施肥器，研究的主要技术手段包括吸肥性能测试试验[8-10]、计算流体动力学（Calculate Fluid Dynamics，CFD）数值仿真[11-14]，高速摄像技术[15-16]等。研究涵盖了文丘里施肥器性能测试、结构参数优化、样件工程应用等方面，形成了较为完善的结构设计及优化方法[17]。针对现有文丘里施肥器的不足，诸多学者对其进行结构参数优化或结构创新：李欢等[18-19]分别设计了螺纹式文丘里施肥器及四通道并联文丘里施肥器，使得文丘里施肥器的吸肥流量得到了明显提升，但仍存在喉部负压利用率较低、吸肥临界压差较高的问题。范兴科等[20]设计了一种偏心向上的非对称文丘里施肥器，并进行了物理试验，研究表明非对称文丘里施肥器水头损失更小、吸肥临界压差明显降低。王海涛等[21]通过CFD数值仿真技术对比分析了对称文丘里施肥器与非对称文丘里施肥器的流场分布差异，指出非对称文丘里施肥器喉部多方向速度碰撞少是其水头损失较小的主要原因。

目前针对非对称文丘里施肥器的研究还停留在与对称结构的对比分析。非对称文丘里施肥器扩散段中存在较大的旋涡区，局部水头损失显著，制约了其吸肥性能的进一步提升，且非对称文丘里施肥器结构形式与内部旋涡特征的关系尚不明晰。基于此，本文采用物理试验与数值仿真相结合的方法，研究扩散段结构形式对非对称文丘里施肥器旋涡特征及吸肥性能的影响，以期进一步提升非对称文丘里施肥器在灌溉系统中的工作性能。

1 材料与方法

1.1 结构模型

本文以贺向丽等[22]设计的弧形扩散段结构的非对称文丘里施肥器（F2）为研究对象（图1a），对比分析直线扩散段（F1，图1b）和弧形扩散段（F2，图1c）的非对称文丘里施肥器旋涡特征和吸肥性能差异。同时为了与市面上常见的对称文丘里施肥器进行比较，建立与F1相同结构尺寸的对称文丘里施肥器（DC，图1d）进行对比分析。文丘里施肥器结构参数依据王海涛等[21]研究确定（表1）。

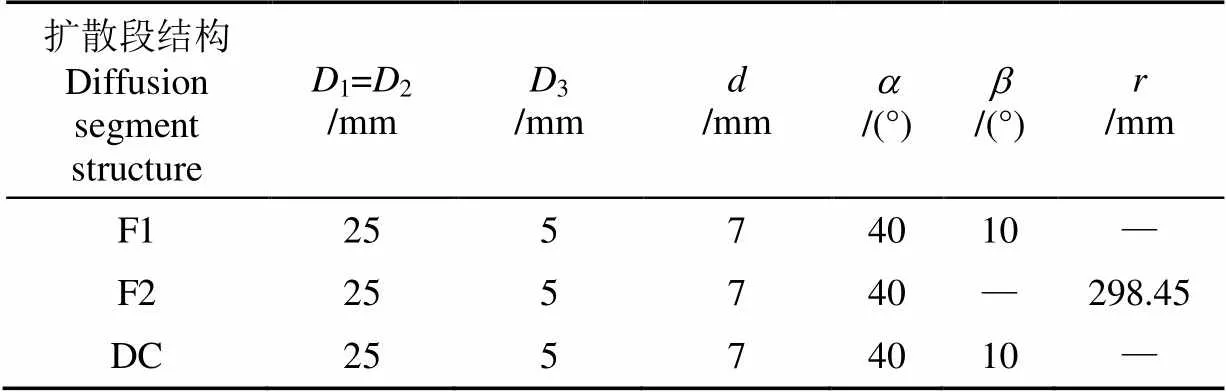
表1 文丘里施肥器结构参数

1.2 数值仿真

采用CFD技术进行流场可视化展示。采用UG12.0建立三维模，利用ANSYS Mesh 19.0进行网格划分。为提高计算精度并尽可能减少计算量，需进行网格无关性验证，以得到最佳网格尺寸。Zhang等[23]研究表明，计算精度主要受网格尺寸的影响，与计算工况相关性较小，因此本文在进水口为0.11 MPa、出口为0.04 MPa、进肥口为0 MPa的工况下进行网格无关性验证，检验指标为吸肥流量变化率，得到吸肥流量变化率与网格数之间的关系（图2）。当计算结果差异小于0.5%时，可认为网格密度对计算结果的精度影响不大，可用作计算网格[24]。综合考虑计算精度和计算成本，确定模型网格计算单元总数95.94万，此时最佳网格划分策略是全局最大尺寸为1.05 mm，针对进水口、水肥出口、进肥口、喉部进行局部网格加密。

图2 网格数量与吸肥流量变化率曲线关系

计算模型的选择取决于水流通过计算域的流态。前人研究指出，文丘里施肥器内部液体流经收缩段进入喉部时，流向发生变化，喉部流态紊乱，且有较大的压力梯度[25-27]，因此本文数值仿真基于压力基求解器，选取Standard-湍流模型，在模型的近壁面采用非平衡壁面函数（Non-Equilibrium Wall Functions）。充分考虑空化效应，采用Mixture多相流模型，将Water-Liquid设置为Phase-1，Water-Vapor设置为Phase-2，激活空化模型中的Zwart-Gerber-Belamri模型。计算过程中，压力速度耦合方程采用SIMPLE算法，设置进水口、进肥口均为压力进口，出口为压力出口。设置进水口压力0.07～0.25 MPa，压力梯度0.02 MPa；进肥口压力设置为0 MPa，出口压力为0.04 MPa（表2），工作环境为1个大气压，计算精度设置为10-5。

1.3 物理试验

为验证数值仿真可靠性，使用PollyPolymer公司提供的精度为0.5 mm的3D打印机，利用HD-50光敏树脂打印试验样件，搭建吸肥性能测试平台（图3）进行试验测试。试验平台采用潜水泵供水，管路安装3个调压阀、2个压力表。试验时吸肥管外接肥液桶供肥，同时通过调节调压阀1、调压阀2、调压阀3控制流经非对称文丘里施肥器的水压。试验过程中设置进水口压力为0.07～0.25 MPa，压力梯度0.02 MPa，出口压力固定为0.04 MPa，吸肥口压力为标准大气压，试验工况如表2。每次试验3 min，用量筒容积法测定不同压力水平下肥液桶试验前后质量差和出口流量，并采用精度为0.001 g的电子天平进行校核。

表2 文丘里施肥器工作压力方案

注：1表示进水口压力；2表示进肥口压力；3表示出口压力；D表示进出口压力差。

Note:1is the water inlet pressure;2is the fertilizer inlet pressure;3is the outlet pressure;Dis the difference between inlet and outlet pressure.

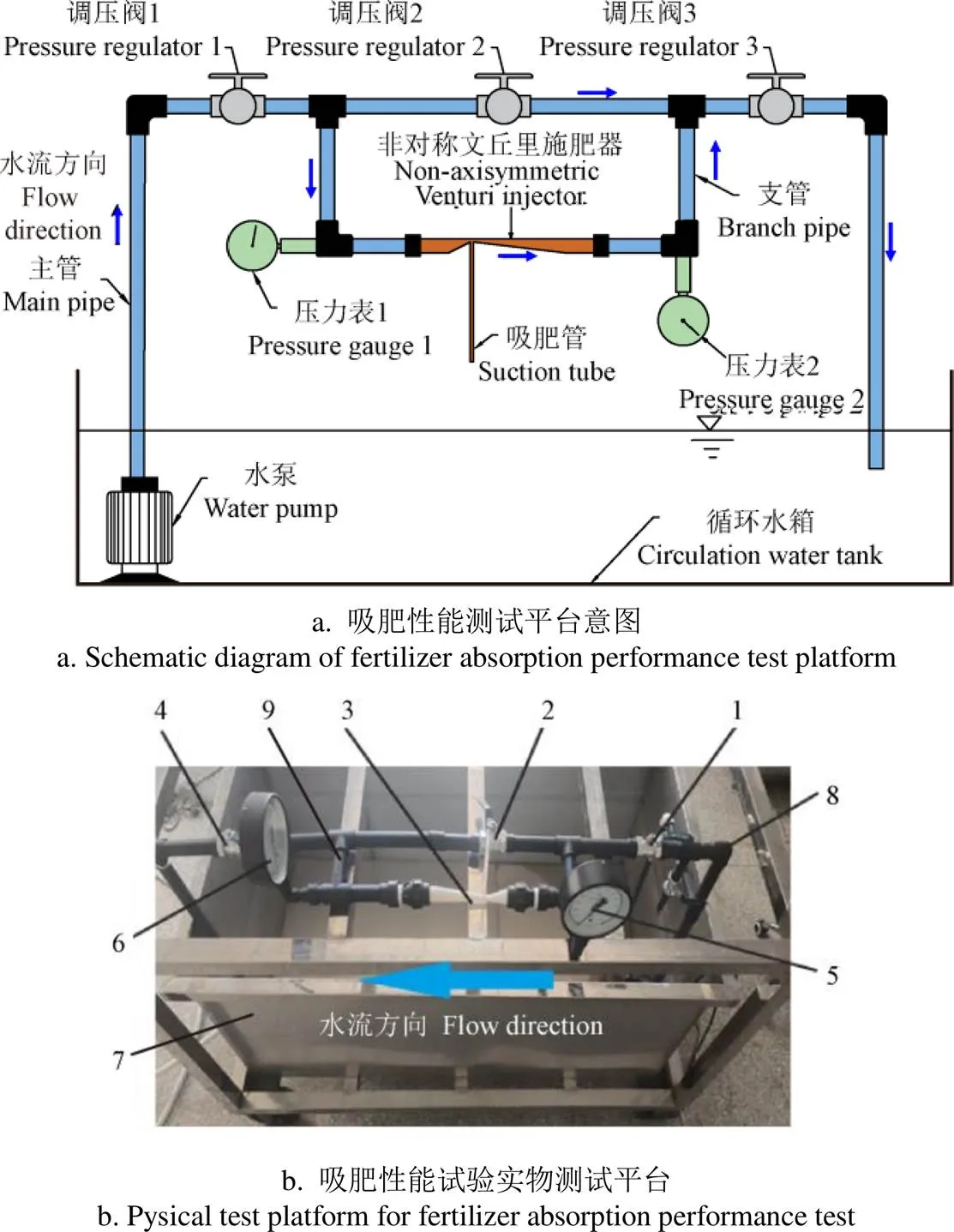
1.调压阀1 2.调压阀2 3.非对称文丘里施肥器 4调压阀3 5.压力表1 6.压力表2 7.循环水箱 8.主管 9.支管

物理试验在石河子大学干旱区滴灌节水兵团科技创新团队滴灌设备试验室进行。为保证试验可持续性，试验时以清水代替肥液。通过试验得到不同压力水平下非对称文丘里施肥器进出口压差D与吸肥流量之间的变化关系，并与模拟值进行对比（图4）。从图4可知，相同进口压力下，实测值与模拟值变化趋势基本相同，二者误差为1.36%～5.18%，小于10%，说明计算模型选取合理[28]。

图4 数值仿真与物理试验对比

1.4 评价指标

文丘里施肥器综合性能通常采用吸肥流量、肥液浓度、吸肥效率这3个指标进行评价[29]。

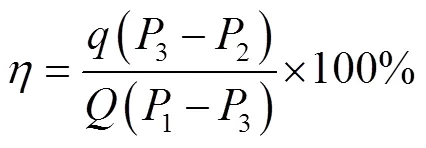
式中表示进水口流量，m3/h。

由公式（1）～（2）可知，和均是、的函数，通过简单变换，可表示为的函数。因此试验以、为主要吸肥性能指标。

漩涡特征包括漩涡面积、漩涡强度及旋涡区边界到喉部末端最小距离，其中旋涡强度[30]计算公式为

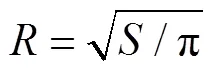
式中表示旋涡区面积，m2；1max表示旋涡区最大速度，m/s；表示旋涡区等效半径，m；表示旋涡强度，s-1。

将旋涡特征数据及吸肥性能数据导入origin 2021b（学习版）中，采用Correlation Plot小程序进行分析，其中相关性计算采用Spearman相关系数，数据缺失采用Listwise补缺方式。

1.5 云图处理

将Fluent计算结果导入Tecplot 360中进行后处理。提取文丘里施肥器中轴面上的速度流线分布、湍流动能分布，分别研究其旋涡特征和水力性能。

对于旋涡处理，以旋涡的最外侧流线作为旋涡区边界，采用样条曲线对边界进行拟合，绘制出旋涡区的形状。沿旋涡边界向旋涡内部延伸，找到旋涡中除边界层部分的最低流速所在的流线，以该流线起点作为旋涡区中心点。其中旋涡边界及中心采用Tecplot软件提取，主要操作步骤包括导入文件、提取中轴面、计算流速、绘制流线、提取出边界层之外的流速最低点坐标，最低流速所在的流线起点即为旋涡中心。样条曲线包含区域大小即为旋涡区面积[31]，旋涡强度由公式（3）～（4）进行计算。为确定漩涡区中心点坐标，以进水口远离吸肥管一侧为坐标轴原点（），沿水流方向为轴，竖直向上为轴建立坐标轴（图5）。

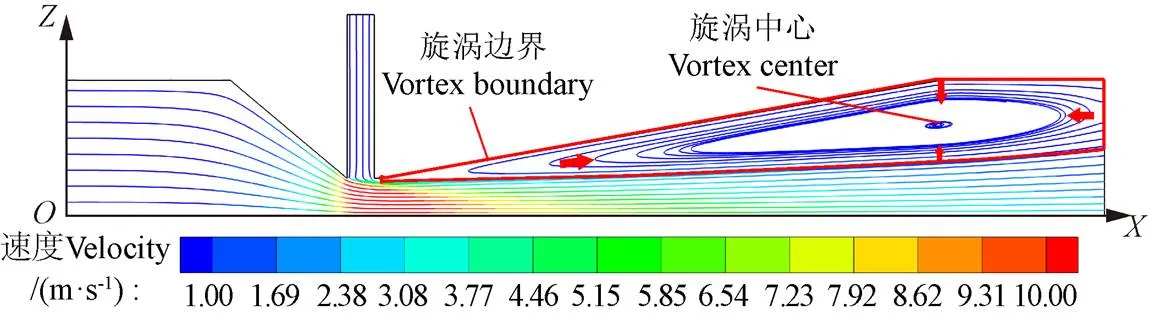
图5 旋涡边界及中心确定方法

2 结果与分析

2.1 旋涡特征分析

图6为不同D下的文丘里施肥器的速度及流线分布。文丘里施肥器的能量转换过程主要是压力与流速之间的转换，在相同的工作环境下，喉部流速越大，能量转换效率越高[13]。随着D的增大，沿水流方向流速先增大后减小，在喉部流速及流速梯度达到最大。喉管下部的流速比喉管上部的流速高，这是因为喉管上部的水流与吸肥管中的肥液相互掺混，流体相互碰撞导致了较大的局部能量损失，进而使得流速降低。在D相同的情况下，最大流速及最大流速区面积从大到小为F2、F1、DC，D0.07 MPa时，F2、F1、DC喉部的最大流速分别为16.76、15.90、15.01 m/s，平均速度分别为9.21、8.91、8.68 m/s，F2的能量转换效率最高，DC的能量转换效率最低。

表3为不同D下的涡旋特征，随着D从0.03 MPa增加到0.19 MPa，3类文丘里施肥器旋涡中心均向后和向下移动。在F1扩散段及出水直管段内，旋涡面积增加了518.77 mm2，增幅达39.27%，主流面积减少48.83%；在F2的扩散段及出水直管段内，旋涡面积增加了427.00 mm2，增幅达47.97%，主流区减小41.85%；在DC扩散段及出水直管段内，旋涡面积增加了531.25 mm2，增幅达38.51%，主流区减小55.02%；随着D的增加，F1与DC的旋涡面积增加幅度减小，F2的旋涡面积增加幅度增大。在相同工况下，与DC相比，F1旋涡区面积减小3.72%～4.25%，旋涡强度减小1.57%～10.54%；与F1相比，F2的旋涡区面积减小28.41%～42.37%，旋涡强度减小6.64%～35.65%。

图6 不同DP下文丘里施肥器的流线及流速对比

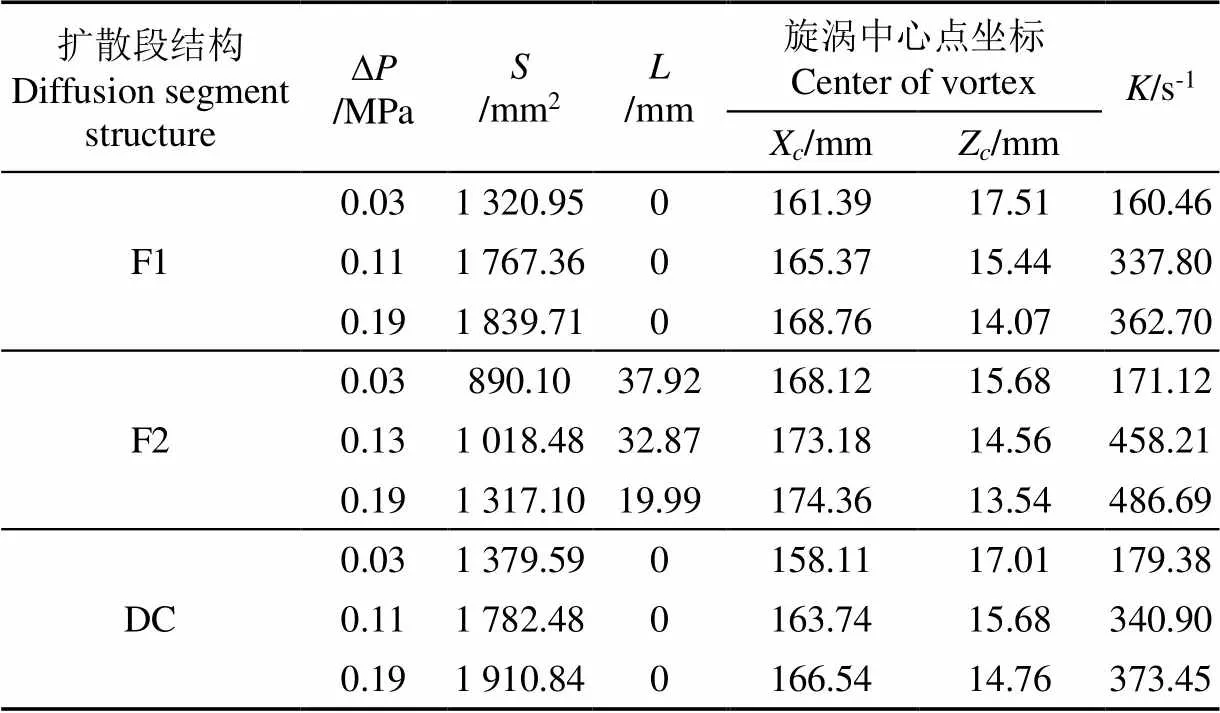
表3 不同DP时的文丘里施肥器旋涡特征

注：表示旋涡区面积，表示旋涡区边界到喉部末端最小距离，表示漩涡中心点的轴坐标，表示漩涡中心点的轴坐标，表示旋涡强度。

Note:represents the of vortex area,is the minimum distance from the vortex area boundary to the throat end,represents theaxis coordinates of the vortex center point,represents theaxis coordinates of the vortex center point, andrepresents the vortex intensity.

当D=0.03 MPa时，DC与F1旋涡区边界与喉部末端的距离为0，说明旋涡已经对喉部造成了影响，而F2的旋涡区较小，且远离喉部，对喉部的影响较小；随着D的增大，F2的扩散段末端形成较大的旋涡，且旋涡区逐步向喉部发展，旋涡对喉部的影响逐渐增大。其原因是与F1相比，F2的扩散段前端与喉部平滑过度，扩散角度较小，不利于旋涡发展；F2扩散段末端的扩散角度较大，导致偏斜流动和回流现象明显，从而产生较大的旋涡，同时随着D的增大，喉部负压增加，在出口压力不变时，逆压梯度增大，导致旋涡区逐渐向喉部发展。与F1、F2相比，DC的扩散段是向两边扩散，其偏斜流动更加明显，主流区主要集中在扩散段远离吸肥管的一侧，从而在其扩散段中形成了更大的旋涡。

2.2 湍流动能分布特征

图7是不同D下文丘里施肥器的湍流动能分布云图。湍流动能反映了流场的湍流程度，湍流动能越大，流体流动状态越复杂，流层之间的碰撞及掺混越强烈，局部水头损失越大[6]。DC、F1的湍流动能在收缩段末端开始迅速增长，在喉管末端和扩散段前端达到最大值，这是因为水流通过收缩段进入喉部时流向发生变化，多方向速度碰撞强烈，同时吸肥管中的肥液与喉部的水流相互掺混碰撞，流态紊乱，导致湍流强度增加。

a. DP=0.03 MPab. DP=0.15 MPac. DP=0.19 MPa

随着D的增大，F2的最大湍流动能逐渐向扩散段中部移动，其原因是随着D的增大，旋涡区逐步向喉部发展，旋涡区回水与主流区中的水发生碰撞，同时该部分产生边界层分离现象，增加了流动阻力损失，导致湍流强度增加。当D<0.15 MPa时，相同工况下，F2的最大湍流动能及其分布区域均小于F1，表明在较低的工作压差下，F2的能量损失较小。当D=0.19 MPa时，DC的最大湍流动能也向着扩散段中部移动，这是因为DC的扩散段向两侧扩散，使得主流区更加远离吸肥管一侧，产生了边界层分离现象，同时远离吸肥管一侧的流向发生转变，多方向速度碰撞增加，湍流强度增加。

2.3 吸肥性能

当D=0.09 MPa时，F2达到最高吸肥流量，其后吸肥流量逐渐下降（图8a），这是因为随着D的增大，F2的扩散段旋涡逐渐向喉部发育，对喉部的影响逐渐增加，且旋涡区域增大趋势较为明显，形成较大旋涡，旋涡区能量耗散显著增加，降低了吸肥性能。当D=0.13 MPa，DC的吸肥流量达到最大值0.143 m3/h，D=0.15 MPa,，F1达到最大吸肥流量0.156 m3/h，其后F1和DC的吸肥流量趋于稳定，这是因为F1和DC的扩散段的旋涡对喉部负压的影响不随着进出口压差的变化而存在显著性差异，但随着进出口压差的增大，管内逐步出现空蚀空化，抑制了喉部负压的进一步增大，影响了吸肥流量的持续增加。相同工况下，F2的吸肥流量比F1提升50.64%～103.22%，增加幅度随着D的增大逐渐降低；DC的吸肥流量比F1的吸肥流量小16.56%～49.15%，减小幅度随着D的增大逐渐降低，这说明相同结构尺寸下，非对称结构文丘里施肥器比对称结构文丘里施肥器吸肥性能更好。

吸肥效率随着进出口压差的增大逐渐降低，并最终趋于平稳（图8b）。在相同工况下，与DC相比，F1的吸肥效率提升31.23%～56.21%。与F1相比，F2的吸肥效率提升48.15%～98.25%，随着D增大，提升幅度逐渐降低。D>0.09 MPa时，F2的吸肥流量下降明显，而F1达到最大吸肥量之后基本保持不变，导致F2的吸肥效率下降速度比F1更快，表明F1的吸肥量随着压强的变化稳定性较强，且工作范围较大，F2在低压差的工作环境下具有较强的吸肥性能，更加适用于低压滴灌系统。

2.4 旋涡对吸肥性能的影响分析

图9是旋涡区面积、强度以及与喉部末端的距离对吸肥流量、吸肥流量变化率及吸肥效率的影响分析。在本试验中，随着D的增大，旋涡区面积及强度与吸肥流量均呈现增长趋势。但随着旋涡区域的增大，吸肥流量增加率及吸肥效率呈现下降趋势，这说明随着旋涡区面积及强度的增加，能量损耗增加，能量转换效率降低。

在95%的置信区间中，F1旋涡区面积及强度对吸肥流量和吸肥效率均存在显著性影响（≤0.05，图9a）。将扩散段改为弧形结构后，旋涡区面积及强度对吸肥流量的影响减弱，仅对吸肥效率有显著影响（图9b），其原因可能是与直线型扩散段相比，弧形扩散段中的旋涡区离喉部较远，旋涡中的回水对喉部最大负压的影响较小，从而对吸肥流量影响较小。在弧形扩散段文丘里施肥器中，旋涡区边界与喉部末端的距离（）对吸肥效率存在显著性关系，随着的减小，吸肥效率逐渐减小。旋涡区面积及强度均与吸肥量流量变化率呈现负相关关系，且仅有DC的旋涡区面积及强度对吸肥流量变化率有显著性影响，说明对称结构文丘里施肥器中旋涡特征对吸肥量的变化有显著影响。

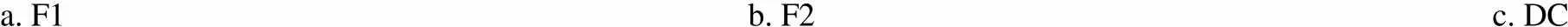
a. F1b. F2c. DC

注：*表示影响显著，≤0.05；为吸肥流量，m3·h-1；表示吸肥流量变化率，=[(q+1-q)/q]´100%，=1～9，%；表示吸肥效率，%；图中数据表示相关性系数，大于0表示正相关，反之表示负相关。

Note: * represents significant effect,≤0.05;is the fertilizer absorption flow rate, m3·h-1;represents the Change rate of fertilizer absorption flow,=(q+1-q)/q´100%,=1-9, %;represents fertilizer absorption efficiency, %; the data in the figure is the correlation coefficient, and if it is greater than 0, it means positive correlation, otherwise it means negative correlation.

图9 旋涡特征对吸肥性能的影响

Fig.9 Effects of vortex characteristics on fertilizer absorption performance

3 讨 论

与对称文丘里施肥器相比，非对称文丘里施肥器的能量转换效率更高，吸肥性能更加优良，在相同结构尺寸下，非对称文丘里施肥器的吸肥流量和吸肥效率分别提升16.56%～49.15%和31.23%～56.21%，本文试验结果与王海涛等[21]的研究结果一致，其原因可能是对称文丘里施肥器中水流流经收缩段、喉部、扩散段时，流向发生多次转变，流体多方向速度碰撞强烈，产生了较大的水头损失；而非对称文丘里施肥器只有一侧进行了渐缩渐阔，另一端平滑过度，湍流程度较小，水头损失明显降低。另外，对称文丘里施肥器喉部负压呈现环状分布，非对称文丘里施肥器喉部负压主要是集中在靠近吸肥管的一侧，其负压区更加集中，负压利用效率更高；此外，在水肥混合的过程中，喉部多余的负压极易由于外界的扰动或自身内部的局部低压区产生空化核，进一步抑制了对称文丘里施肥器吸肥性能的提升[32]。

本文的旋涡特征是基于Fluent数值仿真结果进行分析的，受限于试验条件，并未在实际试验中针对旋涡进行观测，仅用进出口流量、吸肥流量与实测值进行了对比，结果显示数值模拟与实测值误差为1.36%～5.18%，满足分析要求[28]。数值仿真与物理试验相结合的方法是目前研究文丘里施肥器的主要方法，且到目前为止，已经形成了较为完善的试验及结构优化设计体系[33]。数值仿真结果显示，3类文丘里施肥器内部均存在旋涡（图6），多数学者针对文丘里施肥器或者文丘里管进行数值仿真均得到了相似的结果[11,34]。旋涡具有较强的消能作用，旋涡对文丘里施肥器的影响研究较少，但在灌水器的研究中，部分学者将旋涡引入灌水器中进行灌水器消能稳流[35]。可见文丘里施肥器中旋涡的存在增大了局部水头损失，降低了能量转换效率[6]。旋涡主要是依靠流层之间的摩擦进行消能，旋涡强度越大，消能效果越强[31,35]，因此减小旋涡区面积及强度有利于减小局部水头损失，提升文丘里施肥器的能量转换效率。

对于文丘里施肥器内部旋涡产生的原因，部分学者也进行了研究。Kozak等[36]认为偏斜流动是文丘里施肥器内部产生旋涡的主要原因，当喉部直径与进水口直径之比小于0.25或者扩散角大于8°时，偏斜流动最为明显，这是因为当喉部直径与进水口直径之比过小时，在较高的压强下，极易产生射流现象，流速较高，当水流进入扩散段时，流速逐渐降低，水流逐渐扩散，产生回流现象，形成旋涡，且随着进出口压差的增大，旋涡现象更加明显。因此在进行文丘里施肥器结构设计时，应当注意其结构尺寸范围，尽量避免产生较大旋涡，影响文丘里施肥器工作稳定性。王秋良[11,37]等认为吸肥管与喉部交叉，肥液流向改变且与主流发生碰撞，致使整体流动紊乱，产生旋涡。同时水流流经喉部时存在较大的水力梯度，水流的剪切作用及横向环流产生了较大的水头损失，水流流速减小，扩散及回流趋势明显增加。而Li等[6]认为旋涡的产生与喉部结构有关，且喉部吸入室结构形式还会影响旋涡分布的区域，因此在设计文丘里施肥器时也可采用对称型喉部吸入室，以减小偏斜流动。

在相同工况下，旋涡面积及强度从大到小为DC、F1、F2，吸肥流量从大到小为F2、F1、DC。其中F2较F1的吸肥性能明显提升。在产品生产方面，现阶段大都采用压模的生产工艺，虽然弧形结构相较于直线结构相对复杂，但弧形结构内部无倒钩结构，因此生产工艺与直线结构相同，同时弧形扩散段与直线型扩散段的长度和厚度相同，生产成本相当。本文只针对3类不同结构文丘里施肥器进行了研究，其他结构参数对旋涡特征的影响还需进一步研究。

4 结 论

本文以非对称文丘里施肥器扩散段结构形式为研究对象，采用物理试验和CFD数值仿真相结合的方式对比分析了直线扩散段和弧形扩散段的旋涡特征和吸肥性能，同时与市面上常用的对称文丘里施肥器进行了比较，结果显示：

1）随着进出口压差的增大，文丘里施肥器扩散段中的旋涡区面积及旋涡强度增大，旋涡区逐渐向后、向下移动。相同工况下，弧形扩散段的非对称文丘里施肥器旋涡区面积减小28.41%～42.37%，旋涡强度减小6.64%～35.65%。随着进出口压差的增大，弧形扩散段中旋涡区边界到喉部的距离由37.92 mm减小到19.99 mm，对喉部的影响程度逐渐增加。

2）与对称文丘里施肥器相比，相同结构尺寸下的非对称文丘里施肥器吸肥性能更优异。与直线扩散段相比，弧形扩散段的吸肥流量和吸肥效率分别提升50.64%～103.22%和48.15%～98.25%，随着进出口压差的增大，增大的幅度逐渐降低，将扩散段设置成弧形结构更适用于低压灌溉。

3）旋涡特征对非对称文丘里施肥器吸肥性能有显著影响（≤0.05），减小旋涡面积及强度、或增大旋涡区边界到喉部的距离均有利于提升非对称文丘里施肥器吸肥性能。

[1] 张建阔，李加念. 低压灌溉系统中文丘里施肥器吸肥性能试验分析[J]. 农机化研究，2019，41(2)：183-186.

Zhang Jiankuo, Li Jianain. Experimental and analysis of venturi injector fertilizer performance in low pressure irrigation system[J]. Journal of Agricultural Mechanization Research, 2019, 41(2): 183-186. (in Chinese with English abstract)

[2] Duan X N, He W Q, Wang Y B, et al. Optimization design method of a large-scale multilevel gravity drip irrigation pipe network system based on atom search optimization[J]. Journal of Irrigation and Drainage Engineering, 2022, 148(7): 04022023

[3] 汪小珊，严海军，周凌九，等. SSQ系列射流施肥器水力性能试验研究[J]. 农业工程学报，2020，36(21)：31-38.

Wang Xiaoshan, Yan Haijun, Zhou Lingjiu, et al. Experimental research of hydraulic performance on jet fertilizer applicator of SSQ series[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2020, 36(21): 31-38. (in Chinese with English abstract)

[4] Shi Y Y, Hu Z C, Wang X C, et al. Fertilization strategy and application model using a centrifugal variable-rate fertilizer spreader[J]. International Journal of Agricultural and Biological Engineering, 2018, 11(6): 41-48

[5] Fan J L, Wu L F, Zhang F C, et al. Evaluation of drip fertigation uniformity affected by injector type, pressure difference and lateral layout[J]. Irrigation and Drainage, 2017, 66(4): 520-529.

[6] Li H, Li H, Huang X Q, et al. Numerical and experimental study on the internal flow of the venturi injector[J]. Processes, 2020, 8(1): 64.

[7] Wang H T, Wang J D, Yang B, et al. Simulation and optimization of venturi injector by machine learning algorithms[J]. Journal of Irrigation and Drainage Engineering, 2020, 146(8): 04020021.

[8] 周良富，金永奎，薛新宇. 电磁阀开关模式下文丘里施肥器吸肥特性研究[J]. 农业工程学报，2019，35(22)：277-284.

Zhou Liangfu, Jin Yongkui, Xue Xinyu. Suction characteristics of venturi injector in solenoid valve switch on&off mode[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2019, 35(22): 277-284. (in Chinese with English abstract)

[9] 陈囡囡，朱德兰，柏杨，等. 水肥一体化灌溉施肥机吸肥性能试验研究[J]. 节水灌溉，2019(5)：17-20，26.

Chen Nanan, Zhu Deilan, Bai Yang, et al. Experimental study on fertilization performance of integrated water and fertilizer fertigation machine[J]. Water Saving Irrigation, 2019(5): 17-20, 26. (in Chinese with English abstract)

[10] 王海涛，王建东，杨彬，等. 施肥机管路布置对文丘里施肥器吸肥性能的影响[J]. 排灌机械工程学报，2019，37(6)：534-539.

Wang Haitao, Wang Jiandong, Yang Bin, et al． Effect of pipeline layout of fertilizer applicator on performance of venturi injector[J]. Journal of Drainage and Irrigation Machinery Engineering (JDIME), 2019, 37(6): 534-539. (in Chinese with English abstract)

[11] 王秋良，王振华，吴文勇，等. 基于CFD的文丘里施肥器收缩与扩散段结构优化[J]. 节水灌溉，2019(9)：46-52.

Wang Qiuliang, Wang Zhenhua, Wu Wenyong, et al. Structure optimization of contrsction and diffusion section of venturi fertilizer based on CFD[J], Water Saving Irrigation, 2019(9): 46-52. (in Chinese with English abstract)

[12] Abbasi E, Saadat S, Jashni Ayoub K, et al. A novel method for optimization of slit venturi dimensions through CFD simulation and RSM design[J]. Ultrasonics Sonochemistry, 2020, 67: 105088.

[13] Shi H B, Li M D, Liu Q X, et al. Experimental and numerical study of cavitating particulate flows in a venturi tube[J]. Chemical Engineering Science, 2020, 219: 115598.

[14] 张超，梁忠伟，刘晓初，等. 基于ANSYS的文丘里施肥器水力特性研究[J]. 农业与技术，2019，39(8)：1-4.

[15] 严海军，王子君，陈燕. 文丘里施肥器空化过程高速摄像分析[J]. 排灌机械工程学报，2014，32(10)：901-905，920.

Yan Haijun, Wang Zijun, Chen Yan. High-speed photography analysis on cavitation of venturi injector[J]. Journal of Drainage and Irrigation Machinery Engineering, 2014, 32(10): 901-905, 920. (in Chinese with English abstract)

[16] Wang Z N, Liao Y S, Wang W C. An intermittent flow structure in airlift pump by using an annular venturi injector[J]. Journal of Applied Fluid Mechanics, 2021, 14(3): 949-961.

[17] Cheng P, Li Q L, Chen H Y, et al. Study on the dynamic response of a pressure swirl injector to ramp variation of mass flow rate[J]. Acta Astronautica, 2018, 152: 449-457.

[18] 李欢，高亮，张梦，等. 改进型螺旋纹文丘里施肥器的设计与实现[J]. 河北农业大学学报，2019，42(3)：115-119.

Li Huan, Gao Liang, Zhang Meng, et al. Design and implementation of an improved venturi fertilizer apparatus with helical blades[J]. Journal of Hebei Agricultural University, 2019, 42(3): 115-119. (in Chinese with English abstract)

[19] 王永涛，刘坚，李家春，等. 并联四通道文丘里射流器吸肥结构优化与仿真[J]. 中国农机化学报，2021，42(6)：182-190.

Wang Yongtao, Liu Jian, Li Jiachun, et al. Optimization and simulation of fertilizer absorption structure of parallel four-channel venturi jet[J]. Journal of Chinese Agricultural Mechanization, 2021, 42(6): 182-190. (in Chinese with English abstract)

[20] 范兴科，孔令阳. 滴灌系统中文丘里施肥器的选配方法[J]. 灌溉排水学报，2014，33(1)：26-29.

Fan Xingke, Kong Lingyang. The selection of venturi injector in the drip irrigation system[J]. Journal of Irrigation and Drainage, 2014, 33(1): 26-29. (in Chinese with English abstract)

[21] 王海涛，王建东，杨彬，等. 非对称结构文丘里施肥器数值模拟[J]. 排灌机械工程学报，2018，36(11)：1098-1103.

Wang Haitao, Wang Jiandong, Yang Bin, et al. Numerical simulation of venturi injector with non-axis-symmetric structure[J]. Journal of Drainage and Irrigation Machinery Engineering, 2018, 36(11): 1098-1103. (in Chinese with English abstract)

[22] 贺向丽，唐中. 文丘里施肥器及施肥设备：CN113439514 A[P]. 2021-9-28.

[23] Zhang H G, Tang S Y, Yue H R, et al. Comparison of computational fluid dynamic simulation of a stirred tank with polyhedral and tetrahedral meshes[J]. Iranian Journal of Chemistry & Chemical Engineering-International English Edition, 2021, 39(4): 311-319.

[24] Xing S B, Wang Z H, Zhang J Z, et al. Simulation and verification of hydraulic performance and energy dissipation mechanism of perforated drip irrigation emitters[J]. Water, 2021, 13(2), 171.

[25] Costa M G, Leite J M, Beckedorff L, et al. Static pressure behavior of gas-liquid flows along a venturi[J]. Journal of the Brazilian Society of Mechanical Sciences and Engineering, 2021, 43(11): 498.

[26] Song Y C, Shentu Y Q, Qian Y L, et al. Experiment and modeling of liquid-phase flow in a venturi tube using stereoscopic PIV[J]. Nuclear Engineering and Technology, 2021, 53(1): 79-92.

[27] Shi H B, Lui Q X, Nikrityuk P. Numerical study of mixing of cavitating flows in a venturi tube[J]. Canadian Journal of Chemical Engineering, 2022, 99(3): 813-828.

[28] 张建阔，李加念，吴昊，等. 基于双吸肥口的低压文丘里施肥器设计与试验[J]. 农业工程学报，2017，33(14)：115-121.

Zhang Jiankuo, Li Jianain, Wu Hao, et al. Design and experiment of low pressure venturi injector based on double fertilizer inlets[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2017, 33(14): 115-121. (in Chinese with English abstract)

[29] Zhang L, Wei Z Y, Zhang Q. Structural optimization of the low-pressure venturi injector with double suction ports based on computational fluid dynamics and orthogonal test[J]. Desalination and Water Treatment, 2021, 214: 347-354.

[30] Zhang Z H, Li W W. Calculation of the strength of vortex currents induced by vortex generators on flat plates and the evaluation of their performance[J]. Energies, 2022, 15(7): 2442.

[31] 邢少博，张金珠，王振华，等. 不同工作压力下穿孔形灌水器内的旋涡作用分析[J]. 排灌机械工程学报，2021，39(10)：1075-1080.

Xing Shaobo, Zhang Jinzhu, Wang Zhenhua, et al． Analysis of vortex action in perforated emitters under different working pressures[J]. Journal of Drainage and Irrigation Machinery Engineering, 2021, 39(10): 1075-1080. (in Chinese with English abstract)

[32] Song Y C, Wang D Z, Yin J L, et al. Experimental studies on bubble breakup mechanism in a venturi bubble generator[J]. Annals of Nuclear Energy, 2019, 130: 259-270.

[33] 李红，汤攀，陈超，等. 中国水肥一体化施肥设备研究现状与发展趋势[J]. 排灌机械工程学报，2021，39(2)：200-209.

Li Hong, Tang Pan, Chen Chao, et al. Research status and development trend of fertilization equipment used in fertigation in China[J]. Journal of Drainage and Irrigation Machinery Engineering, 2021, 39(2): 200-209. (in Chinese with English abstract)

[34] Manzano J, Palau C V, De Azevedo B M, et al. Geometry and head loss in venturi injectors through computational fluid dynamics[J]. Journal of the Brazilian Association of Agricultural Engineering, 2016, 36(3): 482-491.

[35] 马炎超. 涡漩对迷宫流道灌水器水流流态的数值影响分析[J]. 节水灌溉，2021，3：73-78.

Ma Yanchao. Numerical analysis of the effect of vortex on flow pattern of emitter with labyrinth flow channel[J]. Water Saving Irrigation, 2021, 3: 73-78. (in Chinese with English abstract)

[36] Kozak J, Rudolf P, Hudec M, et al. Investigation of the cavitation within venturi tube: influence of the generated vortex[EB/OL]. (2018-02-27) Advances In Hydroinformatics: Simhydro 2017-Choosing the Right Model In Applied Hydraulics, 1049-1067.

[37] 陈江林，吕宏兴，石喜，等. T型三通管水力特性的数值模拟与试验研究[J]. 农业工程学报, 2012，28(5)：73-77.

Chen Jianglin, Lyu Hongxing, Shi Xi, et al. Numerical simulation and experimental study on hydrodynamic characteristics of T-type pipes[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2012, 28(5): 73-77. (in Chinese with English abstract)

Effects of diffusion section structure on vortex characteristics and fertilizer absorption performance of non-axisymmetric Venturi injector

Wang Zhenhua, Hu Guirong, Liu Ningning, Liu Pingnan, Cao Yuan, Zhang Dongnan

(1.,,,832000,; 2.-,,832000,; 3.,,832000,)

Fertilization equipment is one of the important components of low-pressure irrigation systems. A Venturi injector is widely used in the agricultural irrigation system, due to its simple structure, low price, and convenient use. The non-axisymmetric Venturi injector normally presents a better fertilizer absorption performance than the axisymmetric one. There are many studies on the response relationship between the fertilizer absorption performance and structural parameters of the Venturi injector. But, only a few studies are focused on the response relationship between the vortex characteristics and structural parameters. Therefore, this study aims to explore the influence mechanism of vortex on the non-axisymmetric Venturi injector in the low-pressure irrigation system, in order to improve the fertilizer absorption performance. An analysis was made to compare the vortex characteristics, fertilizer absorption performance, and the response relationship between fertilizer absorption performance and vortex characteristics of non-axisymmetric Venturi injectors with arc diffusion section and non-axisymmetric Venturi injectors with linear diffusion section. A test platform was developed to evaluate the fertilizer absorption performance. The fertilizer inlet of the non-axisymmetric Venturi injector was set as the atmospheric pressure, where the outlet was 0.04 MPa, and the water inlet was controlled between 0.07 and 0.25 MPa. A pressure gradient was taken for each 0.02 MPa to carry out the experimental test of fertilizer absorption performance. At the same time, the computational fluid dynamics software was selected to carry out the vortex analysis and visualization of the flow field. The mathematical modeling, meshing and simulation of Venturi injectors were carried out to compare with the physical test. The Tecplot post-processing software was used to analyze the numerical simulation, where the spline curve was used to locate the vortex. The results show that the diffusion section of the non-axisymmetric Venturi injector as an arc structure was conducive to reducing the vortex area, and the local energy loss in the vortex area, in order to improve the energy conversion efficiency of the non-axisymmetric Venturi injector. The vortex area and intensity decreased by 28.41%-42.37%, and 6.64%-35.65%, respectively, in the non-axisymmetric Venturi injector with the arc diffusion section under the same difference between inlet and outlet pressure. Once the outlet pressure was 0.04 MPa, the rate of fertilizer absorbed by the non-axisymmetric Venturi injector with the linear diffusion section increased gradually with the increase of inlet and outlet pressure difference, and finally tended to be stable. However, when the non-axisymmetric Venturi injector with the arc diffusion reached the maximum amount of fertilizer absorption, the amount of fertilizer absorption decreased with the increase of inlet and outlet pressure difference. The non-axisymmetric Venturi injector with the arc diffusion section presented a 50.64%-103.22% and 48.15%-98.25% increase in the amount and efficiency of fertilizer absorption, respectively, compared with the linear diffusion section. There was a gradually reduced increase in the amount of fertilizer absorption and its efficiency with the increase of the inlet and outlet pressure difference. The vortex region presented a significant effect on the fertilizer absorption performance of the non-axisymmetric Venturi injector (≤0.05). The center point of the vortex region was gradually downward and backward with the increase of inlet and outlet pressure difference. When the inlet and outlet pressure difference was 0.03 MPa, the distance between the vortex boundary and the throat of the non-axisymmetric Venturi injector with the linear diffusion section was 0, indicating that the direct impact of the vortex on the formation of negative pressure in the throat. The vortex in the non-axisymmetric Venturi injector in the arc diffusion section was far away from the throat, without a direct effect on the formation of negative pressure in the throat. However, there was a gradually decreased distance between the vortex boundary and the throat of the non-axisymmetric Venturi injector in the arc diffusion section. The influence of the vortex on the throat increased gradually with the increase of the inlet and outlet pressure difference. A decrease in the area or intensity of the vortex area and an increase in the distance from the vortex boundary to the throat can greatly contribute to the better performance of fertilizer absorption in the non-axisymmetric Venturi injector. The finding can provide a strong reference for the structural design of non-axisymmetric Venturi injectors.

CFD; numerical analysis; Venturi injector; diffusion section structure; fertilizer absorption performance; vortex

10.11975/j.issn.1002-6819.2022.16.007

S224.21

A

1002-6819(2022)-16-0061-09

王振华，胡贵荣，刘宁宁，等. 扩散段结构对非对称文丘里施肥器旋涡特征及吸肥性能的影响[J]. 农业工程学报，2022，38(16)：61-69.doi：10.11975/j.issn.1002-6819.2022.16.007 http://www.tcsae.org

Wang Zhenhua, Hu Guirong, Liu Ningning, et al. Effects of diffusion section structure on vortex characteristics and fertilizer absorption performance of non-axisymmetric Venturi injector[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2022, 38(16):61-69. (in Chinese with English abstract) doi：10.11975/j.issn.1002-6819.2022.16.007 http://www.tcsae.org

2022-06-02

2022-08-14

兵团重大科技项目（2021AA003）；兵团南疆重点产业创新发展支撑计划项目（202DB004）；兵团重点领域创新团队项目（2019CB004）；石河子大学创新发展专项（CXFZ201905）

王振华，博士，教授，博士生导师，研究方向为节水灌溉理论与技术。Email：wzh2002027@163.com