非平衡体系-热库纠缠定理与热输运
杜鹏理,陈子昊,苏 禹,王 尧,徐瑞雪,严以京
(中国科学技术大学,合肥微尺度物质科学国家研究中心,化学物理系,量子信息与量子科技前沿协同创新中心,能源材料化学协同创新中心,合肥230026)
1 Introduction
Quantum transport of heat and particles has attracted much attention in the past years.On one hand,it is closely related to the fundamental physics such as nonequilibrium thermodynamics in the quantum regime.On the other hand,it also plays important roles in such as energy material and quantum information applications.Theoretical studies have been mainly carried out in terms of nonequilibrium Green’s function(NEGF)methods[1,2].
In this work,we exploit the well-established system-bath entanglement theorem(SBET)[3,4],with extension to nonequilibrium transport scenario.Adopted here is the Gauss-Wick’s environment ansatz[5,6]that is commonly adopted in various quantum dissipation theories.These include the formally exact Feynman-Vernon influence functional theory[7],and its time-derivative equivalence the hierarchical equations of motion(HEOM)formalism[8―14].While the existing SBET deals with response functions only[3,4],the extended theory is concerned with the nonequilibrium steady-state quantum transport current through molecular junctions.In this context,the extended SBET provides an alternative approach to the NEGF formalism.It is worth noting that the new theory is established on the basis of the generalized Langevin equation,which can readily support the evaluation on entangled system-bath correlation functions,which are closely related to nonequilibrium thermodynamics in the quantum regime.The conventional fluctuation-dissipation theorem(FDT),which relates correlation functions and response functions,is only applicable to the equilibrium scenario.There are no general relations between the nonequilibrium correlation functions and response functions.It would be anticipated that the present Langevin equation-based method is a viable approach toward such as fluctuation theorem far from equilibrium in the quantum regime.For clarity,we focus on the quantum heat transport formalism.The extension to electron current transport would be straightforward on the basis of the fermionic SBET[4].
In the present work,we present the well-established SBET for the response functions[3],with extension to the nonequilibrium transport scenario.We construct a novel SBET,on the basis of a generalized Langevin equation,which readily leads to NEGF formalism for the quantum heat transport current.
2 Extended System-Bath Entanglement Theorem
2.1 Langevin Equation for Hybrid Bath Dynamics
System-bath entanglement plays a crucial role in dynamic and thermal properties of complex systems.This is concerned with a currently active topic in quantum mechanics of open systems.Recently,we have constructed the SBET[3,4].This theorem comprises exact relations between the entangled system-bath response functions and those of local anharmonic systems.Applications have been demonstrated with Fano interference spectroscopy[3].The SBET has also been exploited in the establishment of the thermodynamic free-energy spectrum theory[4].
To extend this theory to the nonequilibrium scenario,we should include multiple bath reservoirs with different temperatures,so that heat transport is anticipated.The total system-and-bath composite Hamiltonian reads

The system HamiltonianHSand dissipative modesare arbitrary.Theα-reservoir bath Hamiltonian and the hybrid bath modes are modelled with
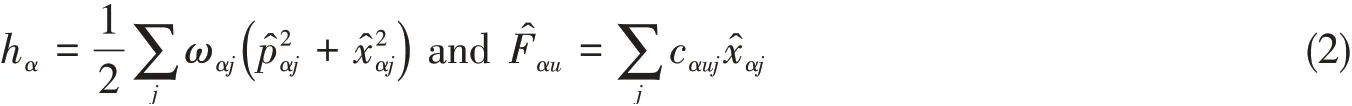
respectively,which together constitute the so-called Gauss-Wick’s environment[5,6].The simplicity arises from the fact that the interacting bath commutators are allc-variables,i.e.,
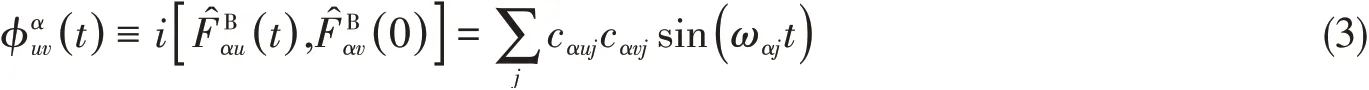
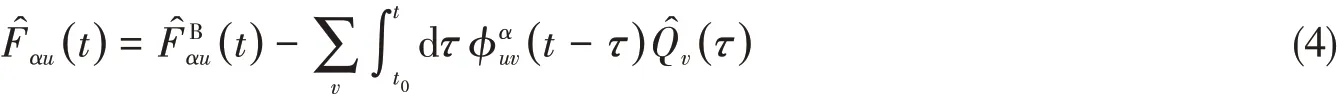

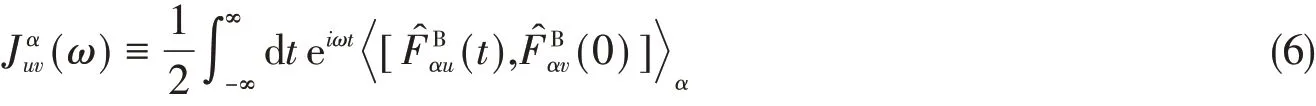
Its microscopic equivalence reads[cf.Eq.(2)]
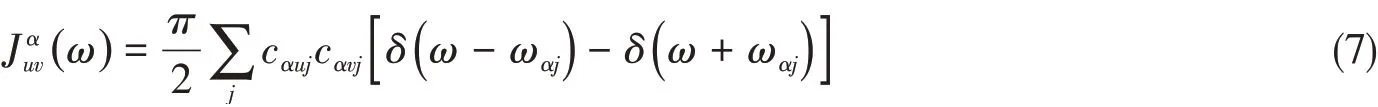
It is worth noting that the Langevin equation(4),together with the property of Eq.(3),will give rise to some interesting relations between the entangled system-bath properties and the local system ones,as bridged with the bare-bath
2.2 System-bath Entanglement Theorem for Response Functions and Expectation Values
The SBET is a type of input-output formalism,in which the local system properties,such as

are the input functions,whereas the nonlocal correspondences,

and

are the output functions.Here,

are defined in the total composite space,at nonequilibrium steady-state scenario,with(·)≡Tr[(·)ρstT],the ensemble average over the total composite space steady-state density operator.It is easily to verify that the established SBET does include the general nonequilibrium scenario[3].The final results,in terms of the matrices,are
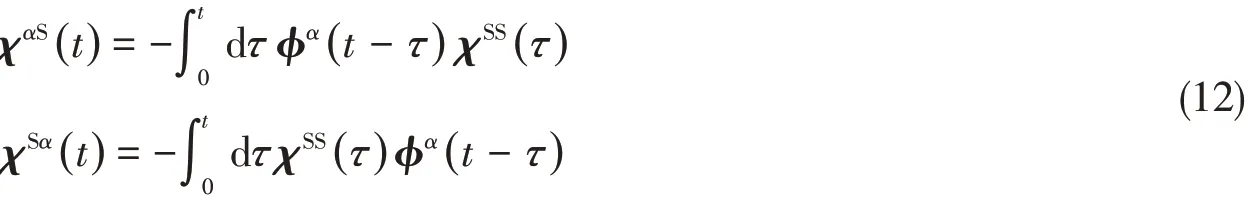
and

and
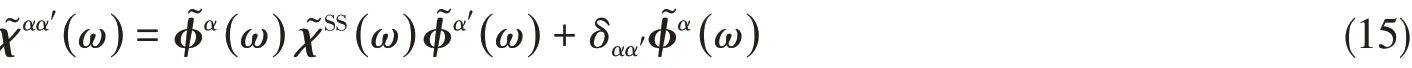
Moreover,Eq.(4)will also give rise to the following input-output relations for the expectation values[4],

where

3 Onset of Heat Current
3.1 Heat Current
Let us start with the heat current transferring from the specifiedα-reservoir to the central system.The related current operator would read[cf.Eq.(1)with Eq.(2)]
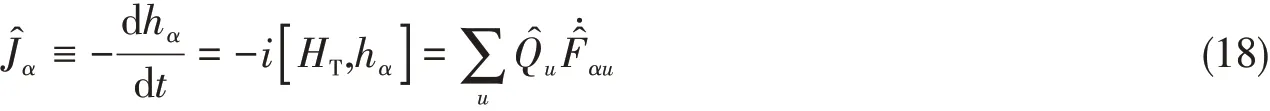
It is noticed there is another convention of heat current operator definition that engages the hybrid bath modesThe others are just linear combinations of above two definitions.The existing dissipaton equation of motion theory can be exploited to the direct evaluation on the transport current and the noise spectrum[19―21].
The quantity of interest in this section is

The direct evaluation can be carried out by exploiting the established dissipaton-equation-of-motion(DEOM)theory[21].In the following,we will establish the extended SBET for the indirect evaluation of Eq.(19).The new theory can be numerically validated with respect to the aforementioned direct evaluations.
3.2 The Extended System-Bath Entanglement Theory
It is noticed that the transport current consists of absorptive(ω>0)and emissive(ω<0)components.In this contact,we decompose the hybrid bath operator,in Eq.(2)as

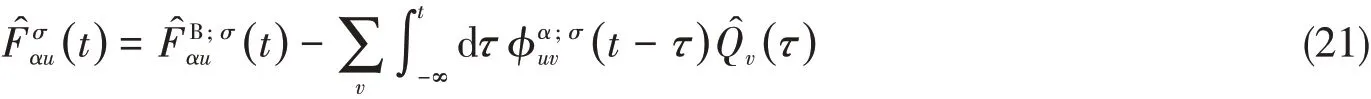

but also
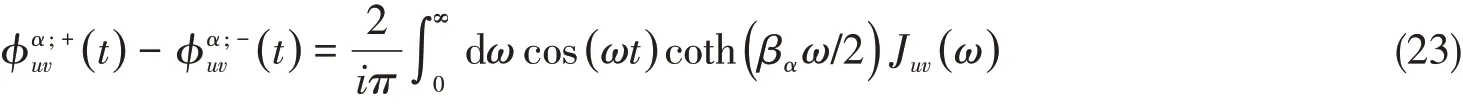
for the required canonical ensemble properties.
To compute the heat current,Eq.(19),we use Eq.(21)to obtain Moreover,the identitiesresult in
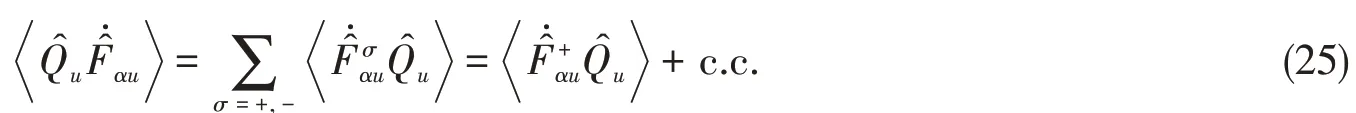

Now,it is readily to obtain
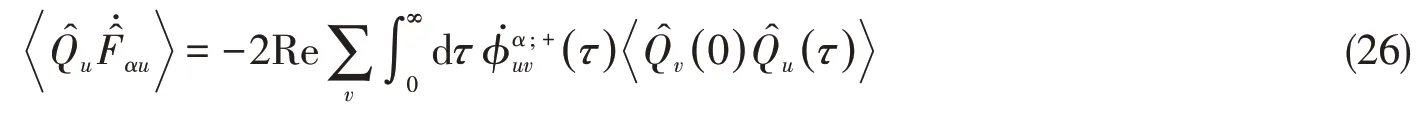
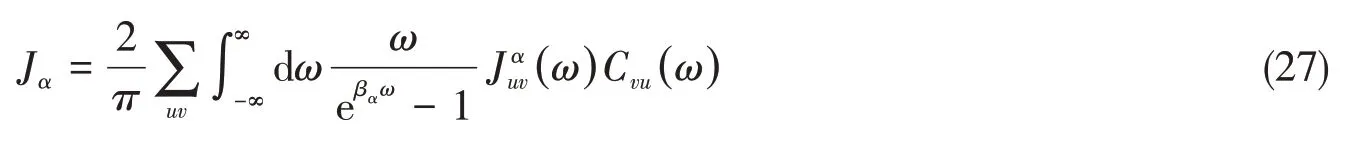
where
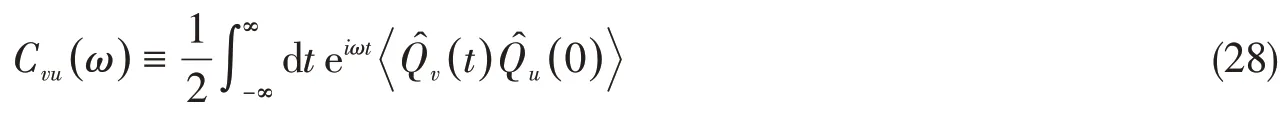
It is easy to show that Eq.(27)is identical to the Meir-Wingreen’s NEGF formalism[23].The latter has been constructed on the basis of Schwinger-Keldysh closed time contour formalism[1,2].Evidently,the present generalized Langevin equation-based approach is much simpler.
3.3 Numerical Validations
For illustrations,consider the total composite Hamiltonian,HTof Eq.(1),withhB=hL+hRand




Table 1 Direct versus indirect approach to the heat current J L,as expressed in Eq.(27)
4 Conclusions
In summary,we revisit the NEGF formalismviathe generalized Langevin equation(4).The present approach can be readily extended to the entangled system-bath correlation functions that would be closely related to nonequilibrium thermodynamics in the quantum regime.
This work is supported by the National Key Research and Development Program of China(No.2017YFA0204904)and the National Natural Science Foundation of China(No.21633006).

