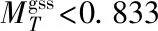双模随机晶场对混合spin-1/2和spin-1纳米管系统磁化强度的影响

李晓杰，信苗苗，蔡秀国，刘恩超

(齐鲁理工学院，济南 250200)

1 Introduction

Since the Blume-Capel (BC) model was established in 1966[1-2]，the magnetization properties，thermodynamic properties and phase diagrams of BC models on a variety of lattices have been studied using different methods. Zhang and Yan studied the phase transition behavior of a mixed spin system in a simple cubic lattice when the external magnetic field follows a three-mode random distribution[3]. In the same year，Zhang and Yanalso studied the critical behavior of a mixed spin system in a simple cubic lattice when both the external magnetic field and the exchange interaction follow a bimodal random distribution[4]. In literature [5]，the compensation behavior and magnetization process of BC model in simple cubic lattice were studied by using the effective field theory. In literature [6]，the phase transition properties of honeycomb lattice when the external magnetic field obeying the bimodal discrete distribution were studied，and it was found that the exchange interaction among the external magnetic field，crystal field and spin affected the phase transition of the system and the system reentered. The research in literature [7] shows that the diluted crystal field has an effect on the magnetic properties and phase transition of the honeycomb lattice system. The results show that when the crystal field meets the dilution distribution，it has no effect on the phase transition of the system and the system will not show the three-critical phenomenon. In recent years，nanotubes have gradually become a hot topic in the field of magnetic properties research. In the experiment，Maoruietal. prepared SnO2nanotube materials using plant cellulose as the template. The test results showed that this SnO2nanotube material could improve the diffusion rate of lithium ions and effectively solve the problem of the expansion of electrode materials during charging and discharging[8]. It is found in literature [9] that magnetic nanotubes have obvious anisotropy. Theoretically，Zaim group studied the phase diagram and magnetic properties of 1 Ising model on the nanotube with external magnetic field consistent with three-mode distribution[10]. The results showed that the system was proved to have first-order phase transition，three-phase critical point and second-order phase transition，and re-entrant phenomenon was observed. Cankoetal. respectively discussed the magnetic properties and critical phenomena of the pure spin system and the mixed spin system in the nanotubes[11-13]，and discussed the influence of crystal field on the magnetic properties of the system. The results showed that there were first-order phase transitions and second-order phase transitions in the system. Kaneyoshi discussed the variation of the magnetization rate with temperature in the nanotubes[14]，and found that the magnetization rate of the system will be changed when the interaction between the outer shell and the inner shell's nearest neighbor spins is different. The results in literature [15] showed the magnetization and phase transition properties of BC model in the double-mode random crystal field，and obtained the relationship between the magnetization of the system and the temperature and the random crystal field，as well as the phase diagram. The results showed that the system would show different magnetic properties and phase transition behaviors in diluted crystal field，staggered crystal field and homogeneous crystal field. The thermodynamic and phase change properties of BEG model on nanotubes were discussed in literature [16]. Literature [17] studied the magnetization properties of BC model in nanotubes under the action of diluting crystal field，and the results showed that the internal energy，specific heat and free energy of the system under the action of diluting crystal field presented different magnetic properties. The online of this paper is as follows: In section 2，the BC model with bimodal random crystal fields is introduced and the formulae of the magnetizations are derived by the use of EFT. The system’s magnetization is presented in section 3. Finally，section 4 is devoted to a brief summary and conclusion.

2 The model and method

The schematic picture of an infinite cylindrical Ising nanotube is illustrated in Fig. 1(a). It consists of a surface shell and a core shell. Its cross section is presented in Fig. 1(b). Each point in Fig. 1 is occupied by a spin-1 Ising magnetic atom. Here，only the nearest neighbor interactions were considered. The exchange couplings between two magnetic atoms were represented by solid bonds，which were plotted between Fig. 1(a) and (b). To distinguish the atoms with different coordination numbers，circles，squares and triangles were used to describe different atoms. The circles and squares respectively represent magnetic atoms at the surface shell. The triangles are magnetic atoms constituting the core shell. It is obvious that the coordination numbers of atoms represented by circles，squares and triangles are 5，6，7，respectively. The bonds connecting the magnetic atoms represent the nearest-neighbor exchange interactions (J1，J2，J).

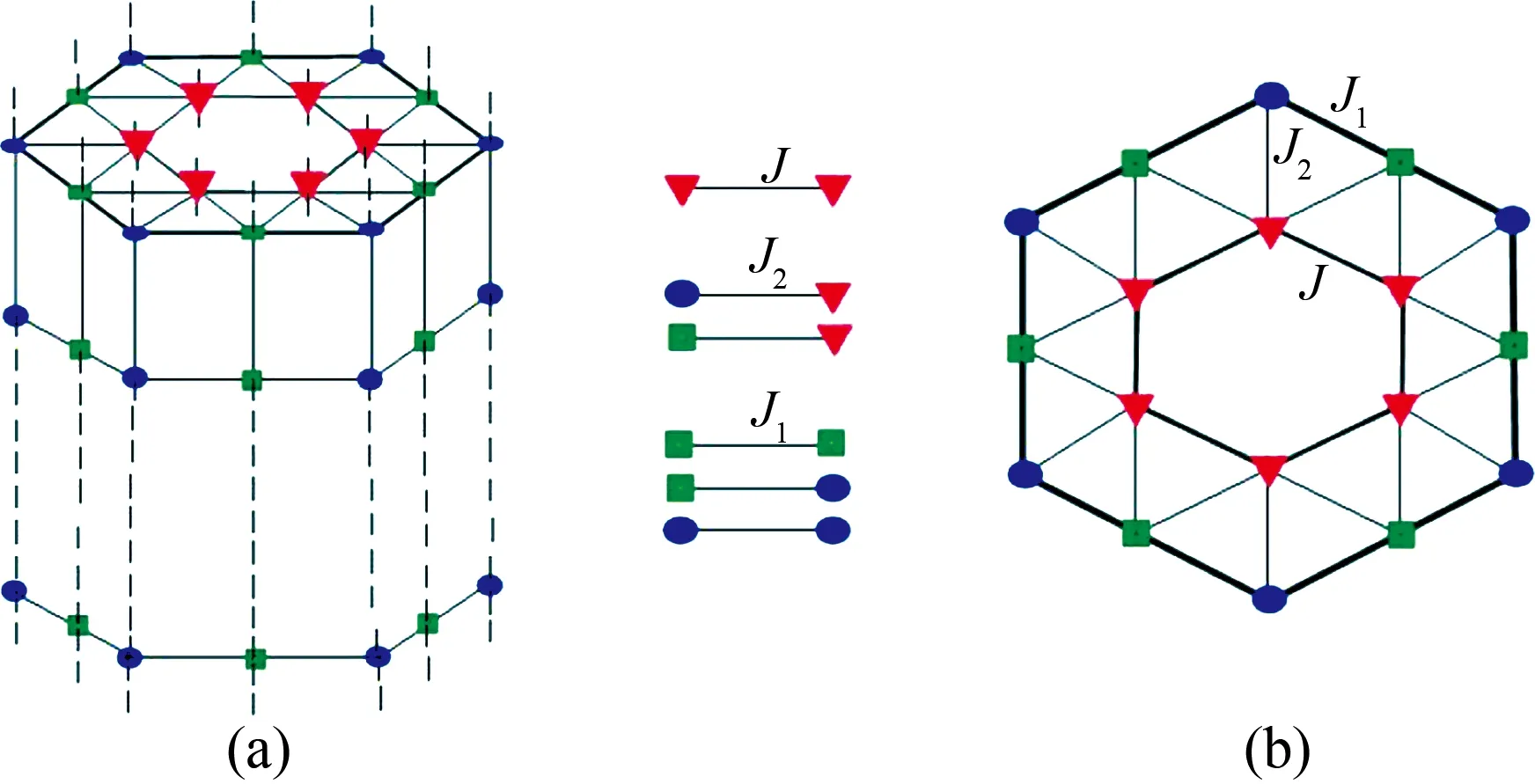
Fig.1 (a) The schematic picture of cylindrical nanotube; (b) the cross section of nanotube

The Hamiltonian of a cylindrical nanotube is expressed as

(1)

whereSandσare the Ising operator and the spin might take the valuesS=±1，0，σ=1/2. The first three summations over 〈···〉 denote pairs of nearest neighbors，the other summations are taken over the each lattice point.J1is the exchange interaction between two nearest-neighbor magnetic atoms at the surface shell and J is the exchange interaction in the core shell.J2is the exchange interaction between atoms at the surface and the core shell. In our model，Diis random crystal fields acting on atoms andDiis the site-dependent crystal field which obeys the following bimodal distribution:

P(Di)=pδ(Di-D)+(1-p)δ(Di-αD)

(2)

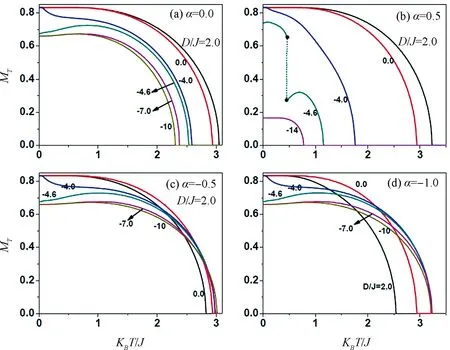
where 0≤p≤1，-1≤α≤1. Thepandαdenote the probability of random crystal fields adoptingDand the ratio of the crystal fields，respectively. Whenp= 1，the random crystal fields mixed spin-1/2 and spin-1 BC model becomes the common mixed spin-1/2 and spin-1 BC model. Whenp=0 andα=0，it degrades into Ising model，that is to say，no crystal field affects the process of magnetization. For 0

It can be obtained the longitudinal magnetizationsm1，m2at the surface shell andmcat the core shell for the nanotube within the framework of the EFT[18-20]:

(3a)

(3b)

(3c)

Here，the functionF(x) is defined by as follows:

pf(x,D)+(1-p)f(x,αD)

(4)

with

(5)

(6)

whereβ=1/kBT，Tis the absolute temperature andkBis the Boltzmann factor.

3 Results and discussions

For the convenience of following discussions，we defined the reduced parameters asJ1/J，J2/JandD/J. In the paper，we setJ1/J=1.0 andJ2/J= 1.0 to contrast our results with those of Ref. [12]. The magnetization curves and phase diagrams obtained numerically by solving Eqs.(3a)～(3c) were plotted.

We only study the effects of the dilute crystal field (α= 0) onMTbecause the magnetization curves are similar whenD/Jtakes a certain value withα0. The relations betweenMTand the temperature for differentD/Jare plotted in Fig. 2. Obviously，the behavior ofMTdepends on both the values ofD/Jandp. From Fig. 2(a)～(f)，forD/J< 0，aspincreases the critical temperatureskBTC/Jdecrease. However，forD/J>0，as p increaseskBTC/Jincrease.

Fig.2 Temperature depends of the averaged magnetization with some selected values of crystal field

The values on each curve denote the value of probabilityp.(a)D/J= 10.0，(b)D/J=-2.63，(c)D/J=-2.64，(d)D/J=-3.30，(e)D/J= -3.60，(f)D/J= -10.0.

Because different doped atoms may change the crystal field acting on spins，different α can be used to describe these conditions. For example，α=0.0,-1.0,-0.5,0.5 can respectively denote four typical distributions of random crystal fields: distributions of diluted crystal field，of symmetry staggered crystal field，of non-symmetry staggered one and of random positive (or negative) one. In order to further study the system’s magnetization behaviors，we plotted the magnetization versus temperature withp=0.25 and 0.75 for four aforementioned typical distributions in Fig. 3 and 4，respectively.

Fig.3 Temperature dependence of the averaged magnetization is presented with p= 0.25 for (a) α=0，(b) α=0.5，(c) α=-0.5 and (d) α=-1.0 with several values of D/J

Fig.4 (Color online) Temperature dependence of the averaged magnetization is presented with p=0.75，for (a)α=0.0，(b)α=0.5，(c)α=-0.5and (d) α=-1.0 with several values of D/J

In Fig. 4(a)～(d)，the system exhibits the first-order and second-order phase transition. When the negative crystal field is strong，the first-order phase transition disappears. Comparing the curves ofD/J=-7.0 in Fig. 3 with that in Fig. 4，the largerpis，the negative crystal field reduces the spontaneous magnetization more obviously.

In this work，we have studiedmixed spin-1/2 and spin-1 Ising nanotube with the bimodal random crystal fields by employing EFT. In particular，we have investigated the effects of probability，crystal field and the ratio of crystal field on the system. We have observed first-order and second-order phase transitions are affected by random crystal field. These factors compete with each other to make the system show richer phase transformation behavior than mixed spin-1/2 and spin-1 BC model with constant crystal field.