轻质长条形反射镜结构优化设计

王朋朋，辛宏伟，朱俊青，王永宪，许艳军，陈长征

轻质长条形反射镜结构优化设计

王朋朋1,2，辛宏伟1*，朱俊青1，王永宪1，许艳军1，陈长征1

1中国科学院长春光学精密机械与物理研究所，吉林 长春 130033；2中国科学院大学，北京 100049

为解决空间反射镜镜体质量和面形精度在轻量化设计过程中会引起相互冲突的问题，针对某型离轴三反光学系统的长条形主反射镜进行了结构优化设计研究，提出了一种基于SiC材料的中心支撑的轻量化结构，同时引入了多目标集成优化方法，以镜体质量(Mass)和面形(RMS)同时作为优化目标，得到一个反射镜最佳结构模型，其质量为2.32 kg，轻量化率达到了73.8%；然后，对反射镜支撑结构进行了结构设计和说明，并对该组件进行了仿真分析，在、、三轴方向1 g重力工况下的RMS值分别达到2.5 nm、2.2 nm、7.3 nm，4 ℃均匀温升载荷工况下的RMS值为3.2 nm，远小于设计要求的RMS≤/50(=632.8 nm)，满足设计要求。

长条形反射镜；中心支撑；轻量化；多目标集成优化；有限元分析

1 引 言

离轴三反(Three mirror anastigmat，TMA)光学系统具有无色差、无中心遮拦、大视场等特点，它已被广泛使用在空间光学遥感器中[1]。其中，长条形反射镜作为该系统核心元件，其镜体的轻量化设计可以减小热惯性，降低动载荷，极大地降低其支撑难度，提高动态特性。然而，轻量化程度增大的同时也带来了新问题，反射镜虽然比刚度呈上升趋势，但其结构的绝对刚度会呈现出一定程度的下降趋势，这种性能间的冲突问题使反射镜的结构设计成为研究热点[2-4]。

目前，Park等在反射镜研制过程中使用变密度法，设计出了第一块轻型反射镜[5]。辛宏伟对具有大长宽比的小型轻质长条形反射镜进行了研究，提出了单点挠性的支撑方式，从而减轻镜体质量，并兼顾成像质量[6]。朱俊青等主要针对规则模型，提出了一种集成参数优化设计方法，在某长条形反射镜实例中，以自动参数化建模为核心，集成参数优化后，确定了该镜三点支撑的分布位置和相应的模型参数[3]。包奇红等对长条形反射镜中心支撑与多点支撑两种支撑方式进行了详细研究，并依据仿真分析数据对比，发现中小型长条形反射镜的中心支撑优于多点支撑[7]。汪荃等对大口径圆形反射镜中心支撑和三点支撑两种支撑方式进行了详细研究，并根据相机在实际工况下的仿真分析结果，对两种支撑方式进行了对比择优[8]。

本文对空间某型TMA光学系统的主反射镜进行了结构优化设计研究，其口径为275 mm×147.6 mm。首先，使用经验公式指导初始结构设计，然后，结合集成优化设计方法，通过对镜坯参数进行向重力工况下的面形(root mean square, RMS)和镜体质量(mass)共同设为优化设计目标，充分考虑反射镜轻量化率的增加会引起其结构的绝对刚度一定程度下降的问题，即Mass减小而引起RMS增大的问题，来进行结构优化设计。最后，对其支撑结构进行了详细阐述和分析。

2 反射镜结构设计

2.1 材料的选择

对比表1的几种常用材料，碳化硅(SiC)材料具有较高的比刚度和尺寸稳定性等重要的物理性质，综合品质远超其他材料[9]，同时，还综合考虑到反射镜组件材料间的热特性匹配的问题，镜体采用反应烧结法，该加工工艺较成熟。因此，使用SiC作为镜体材料。

2.2 支撑方式选择

空间反射镜的支撑结构将应对复杂力、热、振动、冲击环境变化，因此支撑方式的选择对保证反射镜面形精度有着至关重要的作用。

根据支撑位置的不同，支撑形式主要有周边支撑和背部支撑。周边支撑的支撑位置主要是镜体侧边，为了不过大增加结构尺寸，应用范围受限，口径较小的反射镜中应用较多。背部支撑有中心和多点支撑，Hall[10]总结了确定支撑点数量的经验公式，随着中国科学院长春光学精密机械与物理研究所包奇红等[8]的研究发现，采用中心支撑的中小型反射镜性能优于多点支撑的同种反射镜，中心支撑利于减重，且降低整体设计难度，通过合理设计可保证RMS，故选用中心支撑的形式。

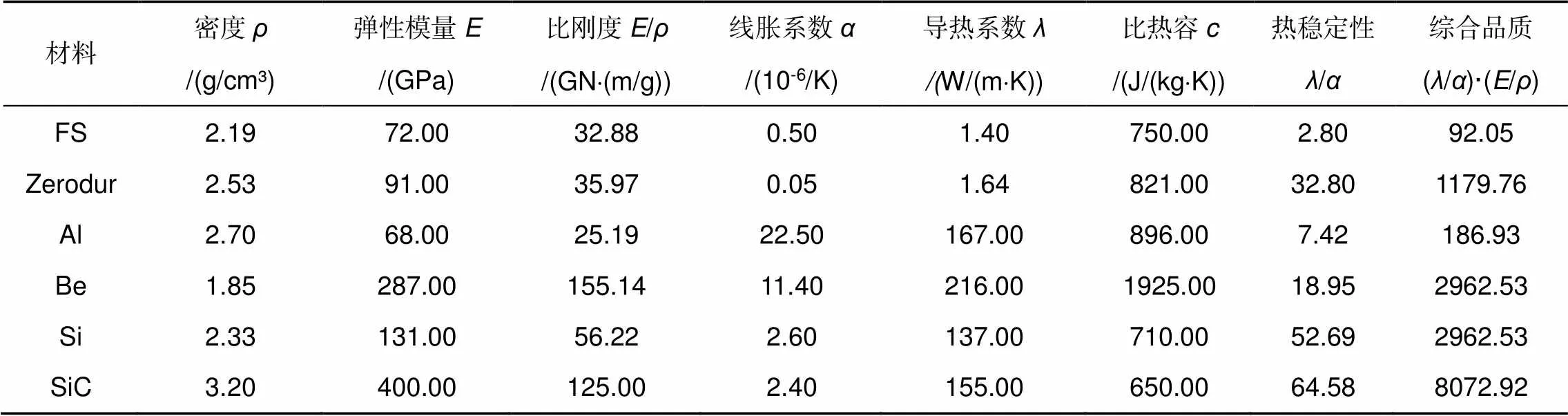
表1 常用反射镜材料的性能

Hindle[11]给出了分布位置所在半径范围的经验式：

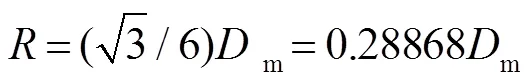
式中：为支撑孔所在的圆周半径，m为反射镜最大口径。此处取m为147.6 mm，由式(1)得≈42 mm。所以，单点中心孔直径最大极限应在Φ84 mm以内，根据本文反射镜的口径尺寸参数特点，确定中心支撑孔直径为Φ50 mm。

2.3 轻量化结构形式

Roberts[10,12-13]等人对圆形反射镜的径厚比D(/)与自重变化的关系进行了研究，并总结了经验式：

式中：为最大自重变形，单位为μm；为材料的密度，单位为kg/m3；为重力加速度，单位为m/s2；为圆盘直径，单位为m；为材料弹性模量，单位为GPa；t为径厚比。长条形反射镜同样可以参考此公式，可以看出，自重变形与材料的比刚度成反比，与径厚比的平方成正比。因而，重力作用下镜面的最大刚体位移试取允许较大安全值，初步选定反射镜的径厚比为8：1。

反射镜轻量化孔的形状包括三角形孔、四边形孔、六边形孔、蜂窝孔、扇形孔和圆形孔等，但是需要注意的是，轻量化孔的设计可能在光学表面形成“网格效应”，该问题会给系统的成像质量带来影响。“网格效应”与轻量化孔形式、材料、加工等因素密切相关，Vukobratovich[14]提出了相关经验式：

式中：max为最大网格变形量；为材料泊松比；为抛光压力；为轻量化孔内接圆直径；f(face thickness)为镜面厚度；为网格效应常数，网格效应常数与轻量化孔的形式有关，具体为：三角形孔=0.00151，正方形孔=0.00126，六角形孔=0.00111。可以看出，当其他条件相同时，三角形孔的“网格效应”要低于其他几种形式, 故本文选用三角形形状的轻量化孔。

反射镜背部结构形式包括倒角式、双折式、圆角式、浴盆式等。针对本文支撑方式，采用倒角式不仅可以便于加工、定位和装夹，而且在轻量化上也起到一定促进作用。

综上所述，可得到图1所示反射镜初步设计几何结构模型，需要说明的是图1中模型的结构参数仅为初始值，后续将进行合理优化。

图1 反射镜的初步设计几何结构模型

3 反射镜参数优化

3.1 镜体结构参数

结合图1，对反射镜进行结构参数设置，本文以镜体结构参数镜体高度(height)、镜面厚度(face thickness)、中心连接孔壁厚(hole thickness)、外沿壁厚(side thickness)、加强筋厚(rib thickness)和背部倒角(chamfer)作为设计变量，并结合当前工艺水平，对各设计变量进行优化范围限定，分别给予最大极限和最小极限，整理为表2。

在对倒角式的反射镜背部结构形式进行参数优化过程中，进行了变量转化，从而减小计算难度，提高参数优化速度。方法是将UG几何模型导入HyperMesh建立反射镜的壳单元网格模型，如图2所示，用三个有限元网格单元节点129388、29390和29389确定一个倒角平面，通过改变单元节点129388的向坐标来改变的大小。本文借助HyperMorph软件创建能够用于优化的形状变量，即利用图2中三个点定义倒角平面，通过节点129388的向单方向位移来转化高度的做法，被HyperMorph成功定义为可用于多目标优化软件HyperStudy直接识别的形状设计变量。

3.2 多目标集成优化

目前，在反射镜的结构设计过程中，单目标集成优化方法已较为成熟，例如以镜体结构参数为设计变量，以反射镜柔性变形为设计约束，并以镜体质量或体积为设计目标(或优化目标)，可得到较优结构模型，但往往得不到发展全局最优解，造成系统设计研究空间的极大浪费。为了解决该问题，应考虑兼顾Mass和RMS的最小化两个方面，充分利用设计空间，找到全局最优解，获得更好的设计综合指标的结果。文中反射镜向(轴向)自重变形相比向和向更显著，其RMS值也较难满足设计要求。因此，将向重力工况下的RMS值和Mass同时作为优化目标，而将向和向重力工况下的RMS值作为约束条件，结合表2的设计变量，进行优化设计。

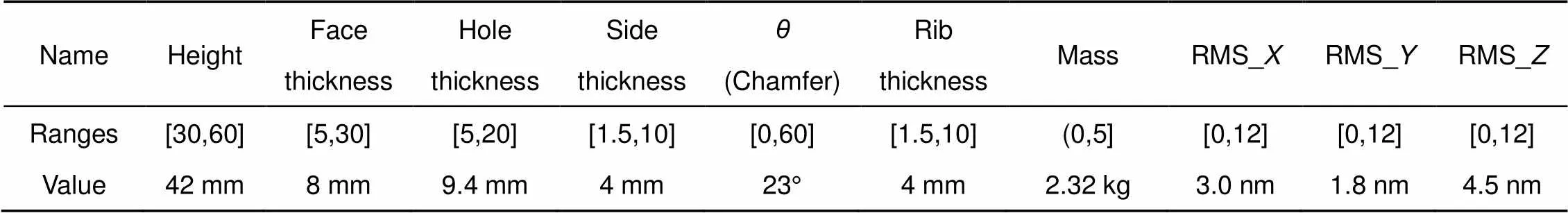
表2 设计变量和优化结果

图2 角度变量转化成坐标变量示意图

本文选用HyperStudy软件进行多目标优化，优化算法采用软件推介的全局响应面算法(global response surface method，GRSM)，该算法默认是处理多目标优化问题的，原理是内部建立响应面，每次迭代都会生成新的全局搜索而得到的设计点，利用新的设计点来更新此响应面，在最佳响应面上搜索出最优点，因而可获得很好的模型拟合，具有高效和实用特点。图3为多目标集成优化流程图。

3.3 优化结果

多目标优化数据结果通常需进行数值圆整，以便加工等。优化后取整结果见表2。

轻量化率计算公式：

其中：为轻量化率，o为原始实体模型质量，e为最终优化模型质量。优化后的模型质量为2.32 kg，与原始反射镜实体模型相比，轻量化率达到73.8%。、和向重力工况下的RMS值分别为3.0 nm、1.8 nm、4.5 nm。满足RMS≤/50(=632.8 nm）和Mass≤5 kg两个设计指标要求。

4 支撑结构设计

反射镜柔性支撑结构包括锥套、柔性元件、背板、过渡角板等。如图4所示，锥套是有一定锥度的套筒，具有一定的中心对称的径向尺寸变形，与反射镜胶接，并将反射镜与支撑结构连接起来，其材料必须采用与反射镜线胀系数相匹配的殷钢(Invar)；如图5所示，柔性元件采用一种双轴圆弧柔性铰链结构，具有体积小、无机械摩擦、无间隙和高灵敏度传动的特点，可通过自身的变形来改善镜面由于热应力所造成的面形误差[15]。该柔性元件分别连接锥套和背板，起到过渡作用，其材料则可以选用强度较高的钛合金(TC4)；背板选用高体份材料(SiC/Al)，进行轻量化后，再通过过渡角板与相机整体固定连接；过渡角板仅起连接作用，图4未给出具体结构形式。

图3 多目标集成优化流程图

图4 体支撑结构爆炸图

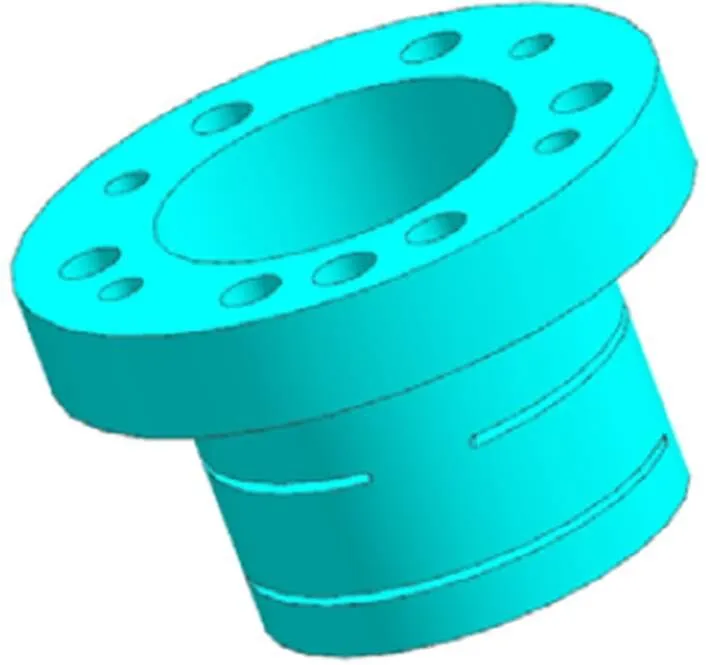
图5 柔性元件

在UG中建立三维模型(如图4)后，结合表3所列的材料密度属性，对反射镜组件进行测量，其质量为3.39 kg。

5 有限元分析

如图6所示，使用HyperWorks软件，将反射镜组件模型划分为有限元网格模型，然后使用Ansys和MSC_Nastran对反射镜组件进行仿真分析。

首先，进行模态分析，考察一阶固有模态是否会与空间相机的一阶固有模态重合或相近，图7为组件的一阶固有模态分析，其一阶频率为140 Hz≥100 Hz，满足动态刚度要求。

然后，进行静态分析，模拟两种工况：地面装调状态轴向1 g重力和温度载荷4 ℃。图8为轴向重力作用时反射镜的变形结果；、和向在1 g重力工况下的RMS值分别为2.5 nm、2.2 nm、7.3 nm；4 ℃均匀温升载荷工况下的RMS值为3.2 nm，满足成像光学系统的反射镜RMS≤/50(=632.8 nm)的要求。

最后，进行结构强度分析，通过在背板与过渡角板连接的螺栓孔处分别沿各轴向输入频率为10 Hz~200 Hz的单位加速度激励的方法，输出反射镜镜体的加速度-频率曲线。由图9可知，向、向和向加速度响应曲线的谐振峰均出现在140 Hz附近，在100 Hz内的频段没有出现谐振峰，满足设计要求。

表3 空间主要应用的结构材料列表

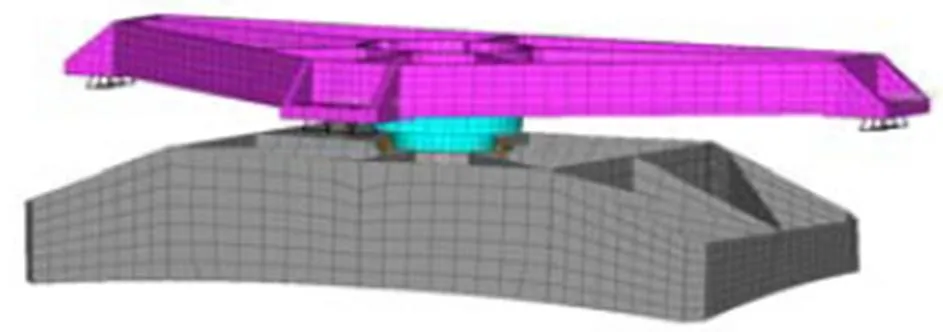
图6 反射镜组件有限元模型

图7 组件一阶固有模态分析

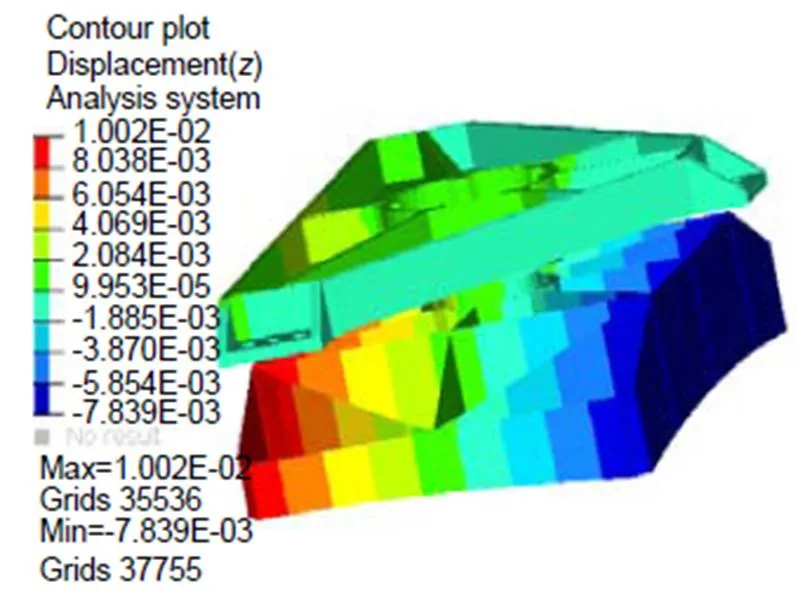
图8 轴向重力作用时反射镜的变形结

图9 加速度频率响应曲线。(a) X向；(b) Y向；(c) Z向

6 结 论

本文针对所述长条形反射镜基于SiC材料的中心支撑的形式进行结构优化设计，通过引入多目标集成优化的方法，在减小镜体质量的同时，尽可能保证了镜面的面形精度。仿真分析的结果显示，镜体质量仅为2.32 kg，轻量化率达到了73.8%；、和向在1 g重力工况下的RMS值分别为2.5 nm、2.2 nm、7.3 nm；4 ℃均匀温升载荷工况下的RMS值为3.2 nm，组件的一阶固有频率为140 Hz。该设计保证了成像质量和轻量化率，并且结构具有高静态刚度、高动态强度，满足设计要求。该方法为同类型轻质长条形反射镜的结构优化设计提供了参考经验，在采用中心支撑的形式下，获得了较优的面形精度。

[1] Zhang K K, Ruan N J, Fu D Y. Analysis and consideration of development of overseas space off-axis TMA system camera[J]., 2008, 29(3): 63–70.

张科科, 阮宁娟, 傅丹鹰. 国外空间用三反离轴相机发展分析与思考[J]. 航天返回与遥感, 2008, 29(3): 63–70.

[2] Zhang L, An Y, Jin G. Optical design of the uncoaxial three-mirror system with wide field of view and long focal length[J]., 2007, 36(2): 278–280.

张亮, 安源, 金光. 大视场、长焦距离轴三反射镜光学系统的设计[J]. 红外与激光工程, 2007, 36(2): 278–280.

[3] Zhu J Q, Sha W, Chen C Z,. Position layout of rear three point mounting for space rectangular mirror[J]., 2015, 23(9): 2562–2569.

朱俊青, 沙巍, 陈长征, 等. 空间长条形反射镜背部三支撑点的设置[J]. 光学精密工程, 2015, 23(9): 2562–2569.

[4] Xin H W, Guan Y J, Li J L,. Design of support for large aperture rectangular mirror[J]., 2011, 19(7): 1560–1568.

辛宏伟, 关英俊, 李景林, 等. 大孔径长条反射镜支撑结构的设计[J]. 光学精密工程, 2011, 19(7): 1560–1568.

[5] Park K S, Lee J H, Youn S K. Lightweight mirror design method using topology optimization[J]., 2005, 44(5): 053002.

[6] Xin H W. Design and analysis on the flexible structure of the optical reflector[J]., 2010, 27(7): 51–55.

辛宏伟. 小型轻质长条反射镜挠性支撑方案研究[J]. 光机电信息, 2010, 27(7): 51–55.

[7] Bao Q H, Sha W, Chen C Z,. Lightweight and optimization design of rectangular reflective mirror supported in centre[J]., 2017, 46(7): 157–163.

包奇红, 沙巍, 陈长征, 等. 中心支撑长条形反射镜轻型优化设计[J]. 红外与激光工程, 2017, 46(7): 157–163.

[8] Wang K, Xin H W, Xu H,. Lightweight design of fast steering mirror for space cameras[J]., 2019, 48(4): 0418001.

汪奎, 辛宏伟, 徐宏, 等. 空间相机快速反射镜的结构轻量化设计[J]. 红外与激光工程, 2019, 48(4): 0418001.

[9] Zhang X J, Li Z L, Zhang Z Y. Space telecope aspherical mirror structure design based on SiC material[J].2007, 36(5): 577–582.

张学军, 李志来, 张忠玉. 基于SiC材料的空间相机非球面反射镜结构设计[J]. 红外与激光工程, 2007, 36(5): 577–582.

[10] Friedman E.[M]. New YorkMcGraw Hill, 2003.

[11] Wang K J, Dong J H. Structural design of Ф2m-level large-diameter SiC reflector used in space remote sensor[J]., 2017, 46(7): 0718005.

王克军, 董吉洪. 空间遥感器Ф2m量级大口径SiC反射镜镜坯结构设计[J]. 红外与激光工程, 2017, 46(7): 0718005.

[12] Parks R E, Wortley R W, Cannon J E. Engineering with lightweight mirrors[J].1990, 1236: 735–743.

[13] Anderson D, Parks R E, Hansen Q M,. Gravity deflections of lightweighted mirrors[J].1982, 0332: 424–435.

[14] Vukobratovich D, Iraninejad B, Richard R M,. Optimum Shapes for Lightweighted Mirrors[J].1982, 0332: 419–423.

[15] Wang X Y, Zhou P W. Design of rectangular space mirror and its support structure[J].2017, 40(2): 6–9, 13.

王晓宇, 周平伟. 长条形空间反射镜镜体及其支撑结构设计[J]. 长春理工大学学报(自然科学版), 2017, 40(2): 6–9, 13.

Structural optimization design of lightweight rectangular reflective mirror

Wang Pengpeng1,2, Xin Hongwei1*, Zhu Junqing1, Wang Yongxian1, Xu Yanjun1, Chen Changzheng1

1Changchun Institute of Optics, Fine Mechanics and Physics, Chinese Academy of Sciences, Changchun, Jilin 130033, China;2University of Chinese Academy of Sciences, Beijing 100049, China

Explosion chart of mirror support structure

Overview:The research area of this paper is the field of off-axis three-reflective space optical remote sensor. As a core element of the system, the rectangular reflective mirror has been the focus. Increasing the degree of lightweight will also bring new problems, which cause a certain degree of structural strength reduction. Obviously, RMS(root mean square) will get worse. The purpose of this paper is to propose a feasible solution to this conflict of performance. First of all, select SiC as the mirror body material. Secondly, a flexible structure is based on the center support, which facilitates lightweight and reduces the overall design difficulty. Next, use the classical theoretical formula to create the initial structure of the mirror. The most important step is to introduce a multi-objective optimization method. The structural parameters of the lens body are used as design variables, and then the surface RMS values underandgravity conditions are used as constraints. It is the mass of the mirror and the RMS values under the most sensitive-direction gravity conditions that are commonly set as the optimization goal. Furthermore, using GRSM(global response surface method) algorithm for optimization iterations. A mirror optimal structure model is obtained with a mass of 2.32 kg. Compared with the solid mirror, the lightweight ratio is 73.8%. Besides, the mirror subassembly is designed. It includes a cone sleeve, a flexible component, and a backplane. The main considerations of the assembly are the stiffness of the materials and the thermal compatibility between each other. The specific explanation is as follows. Thermal expansion coefficient of the cone sleeve and the mirror need to be the same, and these are connected by glue. The flexible component adopts a flexible hinge structure so as to improve RMS of the mirror due to thermal stress. The backplane connects the mirror assembly to one space remote sensor. Therefore, the rigidity of the backplane must be qualified. Finally, the integrated performance of the assembly is simulated. It shows that the RMS value of the mirror reaches respectively 2.5 nm, 2.2 nm and 7.3 nm when gravity load is applied in the directions of,andaxes. Furthermore, the RMS value is 3.2 nm when the mirror subassembly is under the load condition of uniform temperature rise of 4 ℃, which is far less than the requirement of RMS≤/50(=632.8 nm). As a result, the data meets the design requirements. To sum up, the method provides reference experience for structural optimization design of the same type of lightweight rectangular reflective mirror.

Citation: Wang P P, Xin H W, Zhu J Q,Structural optimization design of lightweight rectangular reflective mirror[J]., 2020, 47(8): 200109

Structural optimization design of lightweight rectangular reflective mirror

Wang Pengpeng1,2, Xin Hongwei1*, Zhu Junqing1, Wang Yongxian1, Xu Yanjun1, Chen Changzheng1

1Changchun Institute of Optics, Fine Mechanics and Physics, Chinese Academy of Sciences, Changchun, Jilin 130033, China;2University of Chinese Academy of Sciences, Beijing 100049, China

In order to solve the problem that the mass and the surface figure accuracy of the space reflective mirror are often contradictory in the lightweight design process, a structural optimization design of a lightweight rectangular reflective mirror of an off-axis three-reflection optical system is performed. In this study, a lightweight structure based on the center support of SiC materials is proposed. At the same time, a multi-objective optimization method is introduced. With the RMS value and Mass as the optimization targets at the same time, a mirror optimal structure model is obtained with a mass of 2.32 kg. Compared with the solid mirror, the lightweight ratio is 73.8%. Then the mirror subassembly is designed and the integrated performance of it is simulated. It shows that the RMS value of the mirror reaches respectively 2.5 nm, 2.2 nm and 7.3 nm when gravity load is applied in the directions of,andaxes. Furthermore, the RMS value is 3.2 nm when the mirror subassembly is under the load condition of uniform temperature rise of 4 ℃, which is far less than the requirement of RMS≤/50(=632.8 nm). Therefore the data meets the design requirements.

rectangular reflective mirror; support in center; lightweight; multi-objective optimization; finite element analysis

TH751

A

10.12086/oee.2020.200109

: Wang P P, Xin H W, Zhu J Q,. Structural optimization design of lightweight rectangular reflective mirror[J]., 2020,47(8): 200109

王朋朋，辛宏伟，朱俊青，等. 轻质长条形反射镜结构优化设计[J]. 光电工程，2020，47(8): 200109

Supported by the Youth Program of National Nature Science Foundation of China (11803036)

* E-mail: xinhwciomp@sina.com

2020-03-31；

2020-06-06

国家自然科学基金青年基金资助项目(11803036)

王朋朋(1994-)，男，硕士研究生，主要从事空间光学遥感器光机结构设计及CAD/CAE工程分析。E-mail：1413439235@qq.com

辛宏伟(1970-)，男，博士，研究员，主要从事空间相机光机结构的研究。E-mail：xinhwciomp@sina.com