Calibrating HCM model for roundabout entry capacity under heterogeneous traffic

Abdullah Ahmad· Rajat Rastogi

Abstract Roundabout is a channelized intersection where traffic moves around a central island, clockwise for leftside driving and anti-clockwise for right-side driving.Efficiently designed roundabouts can handle traffic very smoothly without causing any delay. The capacity of roundabouts used to be calculated by the weaving theory in India. However, calculation of the entry capacity in the recent literature is based on critical gaps and follow-up times, and the Highway Capacity Manual of US (HCM 2010) provides an equation to estimate the entry capacity of a roundabout by using the flow in passenger car unit per hour(PCU/h),critical gaps and follow-up times at the entry section. In order to examine whether the HCM equation applies to Indian traffic condition or not,we collected data from five roundabouts in India in this study. Relevant data were extracted/estimated to calibrate parameters of the HCM equation. The PCU for a vehicle was estimated on the basis of lagging headway and width of the vehicle,and the critical gap value for a vehicle was estimated by minimizing the sum of absolute difference in a gap with respect to the highest rejected and accepted gaps.Results show that the critical gap values obtained under heterogeneous traffic conditions are much lower than those given in the literature for homogeneous traffic conditions. In addition, the modified HCM equation based on the critical gap values was verified using the field data taken during the formation of a continuous and stable queue at the entry of a roundabout.It was found that a multiplicative adjustment factor needs to be calculated for different sizes of roundabouts to ensure the adjusted HCM equation represents well the traffic condition prevailing in developing countries like India. A test conducted at another roundabout validated that the entry capacity estimated from the calibrated and adjusted HCM model was consistent with the field entry capacity,and the calibrated and adjusted HCM model could predict the entry capacity of an approach to a roundabout quite accurately.

Keywords Roundabout · Entry capacity · HCM ·Heterogeneous traffic · Gap acceptance

1 Introduction

Roundabout is an intersection with a roadway, which circulates around an island and caters to the traffic entering from three or more connecting legs or approaches.Roundabouts perform better than other types of intersection in terms of capacity and delay [1]. Their installation improves intersection safety by reducing the conflict points,crash severity,and causing drivers to reduce speeds in an intersection area[2,3].Due to such merits,they work as a traffic control measure at intersections. Their design provides a mechanism for merging and diverging of circulating and entering traffic flow streams. Priority goes to circulating traffic flow stream, whereas the entering traffic flow stream has to adjust till acceptable gaps are available in the circulating traffic flow stream. In a disciplined environment, the entering vehicles will give way to the circulating vehicles and will enter the roundabout on first safe opportunity available (gaps between circulating vehicles), with vehicle at the back following the leader. While making such a maneuver, longitudinally and laterally safe margins will be maintained by the drivers.This will remain more or less uniform if the drivers are disciplined or the traffic is homogenous in nature. The scenario may change under heterogeneous traffic flow and will change if the driver behavior is not disciplined. Under heterogeneous traffic flow, the gaps, longitudinally and laterally, will differ considerably because of varying size of vehicles.This stimulates the drivers of varying sized vehicles to occupy the available spaces laterally while maintaining absolute minimum clearances needed at the sides. Such behavior gets transformed into a habit which is defined by many as undisciplined driver behavior. On the other side,this can also be defined as optimal use of available road spaces, thus allowing more vehicles per unit space. The driver behavior is expected to change if the number of lanes is one instead of many. The above-mentioned opportunities may or may not be available on a single-lane carriageway. This will also depend upon the spaces available on road side which creates a feel of open available spaces for use. Therefore, the parameters like rate of inflow traffic, driver behavior, flow characteristics and geometric measures will have their impact on the volume of traffic that can enter the roundabout.This defines the entry capacity of a roundabout.

The traffic handling capacity of the roundabout had been estimated in the past as the capacity of the circulating(weaving) section. In such a case, the basis was the width of the circulating roadway,the mix of the vehicle types,the length of the weaving section between adjacent entry and exit, the approach and exit widths, etc. Wardrop [4] gave the formula for estimating the roundabout capacity in the late 1950s,which was based on the mentioned attributes.In India,the same approach was followed and documented in Indian Roads Congress code IRC-65 [5]. Both of them basically estimated the capacity of the weaving section. In the mid-1960s, the priority rule was implemented in Britain.The subsequent studies carried out on the validation of Wardrop’s formula under new traffic control (priority)condition indicated that it was not valid and gave inaccurate results. Going by the research findings, Britain changed the estimation formula for the capacity of a roundabout[6]. Later, the research carried by Pearce [7] and Waddell[8]separately concluded that traffic in the weaving section does not affect the capacity of the roundabout. Such findings necessitated the shift in the estimation approach for the capacity of the roundabout. It was felt that under the given traffic flow conditions, the number of vehicles that can enter the roundabout in a given time period would depend upon the circulating traffic flow (volume) in the circulating roadway, especially in front of the entry approach. As the traffic flow in a circulating roadway increases over the time period in a day,the traffic flow that can enter the roundabout circulating area from any approach leg would keep reducing. In other words, as the circulating traffic flow decreases, the entry traffic flow increases due to higher opportunities available to drivers for entering into the circulation area. This led to the new definition of roundabout capacity, named as ‘entry capacity.’ It is defined as the maximum number of vehicles that can enter the roundabout in a given time period with respect to the circulating traffic. Intuitively, these two traffic flow entities would be moving in opposite directions,and the relation between the two may or may not be linear in nature.If the nature of relationship is linear,then with no circulating traffic flow the entry capacity shall be the maximum, whereas under extremely heavy circulating traffic flow there shall not be any entry traffic flow. This shall result in queuing in an approach.Leaving acute traffic flow conditions,in most of the traffic flow conditions it has not been observed true.There remains some flow from the approach which merges into the circulating traffic flow. In such a scenario, it can be hypothesized that ‘the relationship between the two is not linear; rather, it may take an asymptotic shape(showing constant flow)after a sharp fall in the entry traffic flow with heavy increase in the circulating traffic flow.’

Examination of the literature indicates that researchers have estimated the entry capacity of a roundabout through two approaches, namely the empirical approach (or regression-based approach) and the gap acceptance-based approach. The empirical approach is based on the highest traffic flows observed during peak periods from a road into a roundabout circulation area.Such models can best reflect the local traffic flow condition, but their transferability to other locations remains questionable. On the other hand,the gap acceptance-based approach inherently considers the decision making of the drivers of entering vehicles to accept or reject the available gaps between circulating vehicles under the perceived traffic flow conditions.C¸alis¸kanelli et al. [9] applied the regression analysis method to compare the already available roundabout capacity models in the literature, where the data were collected at four multilane and five single-lane roundabouts in Izmir, Turkey. They found that the gap acceptance method gives more accurate results than the other models.The Highway Capacity Manual of US (HCM 2010) presented a gap acceptance model of roundabout entry capacity,which is dependent upon the values of the critical gap and follow-up time [10]. This manual is extensively used in different parts of the world for estimating the capacity of a traffic facility. The approach assumes that lane discipline is strictly followed by drivers and the entry behavior of drivers is also normal.However,this is not the case of traffic condition in developing countries like India.In India,a large variety of vehicles ply on roads with heavy traffic flows. Due to no enforcement of lane discipline,formation of parallel lanes of different sized vehicles often converts, for instance, a single-lane system to a two-lane system and a two-lane system converts to a three-lane system. In the case of merging operations, such vehicles force their entry into the stream; bigger-size vehicles for entry into the circulating traffic stream also utilize the gap formed at times. This can also be noted from the relative average values of critical gap and follow-up time used in the USA which are 4.5 s and 3.2 s, respectively, and are much higher than the real values (e.g., critical gaps range 1.5-2.5 s) observed in India [11]. Therefore, the entry capacity model proposed in HCM 2010 does not have direct transferability to the traffic conditions as above in developing countries like India. It is expected that the application of HCM models may result in unrealistic results under such conditions. This gave stimulus to conduct this research and led to the formation of the hypothesis that ‘Estimation of entry capacity of an approach to a roundabout by HCM method in Indian traffic condition needs modification through calibration of its parameters.’

A group of researchers in India has already initiated some work on this aspect. Ahmad et al. [12] studied roundabout capacity models of different countries like France, Germany and the USA (developed countries with lane discipline)and Jordan(developing country).Their aim was to examine the possibility of implementing any of them in Indian traffic conditions with or without modifications.Analysis was done for a roundabout with a central island diameter of 37 m. The pattern of change in field entry flow versus circulating traffic flow in India was observed to be similar to US model (HCM 2010), but the magnitude of field entry flow was differing too much. The HCM 2010 model gave lower values of entry flow, while quite high value of entry flow was observed at negligible circulating flow values. Mahesh et al. [13]also studied the HCM 2010 model with a similar aim. The relationship between entry traffic flow and circulating flow was found to be negative exponential.The entry traffic flow value was found to be moderate at negligible circulating traffic flow as compared to that of Ahmad et al. [12]. Critical gap and follow-up time were extracted in Indian condition and used to modify the HCM 2010 equation. Varying adjustment factors were proposed with respect to circulating traffic flow for roundabouts using the adjusted HCM 2010 model.Though both of the approaches tried to improve upon the existing method available in IRC-65 [5], they had two deficiencies.Firstly,both of the works proposed adjustment factors to be multiplied to the already adjusted HCM 2010 model (double adjustment needed), without giving an opportunity of direct computation of entry traffic flow.Secondly,the passenger car units(PCUs),used to make the heterogeneous traffic homogenous (as per the requirement of HCM model), were taken from IRC-65 (1976), a 42-year-old guideline. This indicated toward the need for redefining the PCU values for different categories of vehicles on roundabouts, considering the variability and improvement in vehicle technology, and the possible change in driver behavior over the years.

The main objectives of this study are (1) to estimate PCU values for different types of vehicles typically found on a roundabout in developing countries and (2) to calibrate the HCM 2010 entry capacity equations for its adaptation to the heterogeneous traffic, and develop a model of entry capacity using traffic flow and geometric data.

The remainder of this paper is organized as follows.Section 2 introduces the entry capacity model given in HCM 2010. Section 3 introduces the site selection, field data collection and extraction of required data from video film. Section 4 deals with estimation of PCU values on roundabouts, and Sect. 5 estimation of critical gap and follow-up time. Then, Sect. 6 discusses the transferability of HCM 2010 model and provides the relationship between entry capacity and circulating traffic flow. Section 7 deals with the validation of the developed model, and Sect. 8 gives conclusions.

2 HCM 2010 entry capacity model

The HCM 2000 is one of the popular models of roundabout entry capacity based on gap acceptance. In the 2010 version of the Highway Capacity Manual,a detailed procedure was developed for estimating the entry capacity of roundabouts in the USA[14].HCM 2010 suggests use of Eq. (1)to estimate entry capacity (Ce) of roundabouts.

where Vcdenotes circulating traffic flow(PCU/h);A and B are parameters calculated as below:

in which tfis follow-up time (s) and tcis critical gap (s).

Parameters A and B and the corresponding gap acceptance parameters (tf, tc) are given in Table 1.

Table 1 HCM 2010 roundabout parameters (A, B, tf and tc)

Table 2 Geometric features of selected roundabouts (m)

3 Data collection and extraction

Data were collected on five roundabouts in the city of Chandigarh, India. Salient geometric features are listed in Table 2. The roundabouts were in semi-urban area, with negligible interference caused by pedestrians and traffic signals of nearby intersections. The terrain was plain, with no-parking and adequate sight distance being ensured on all the approaches. The video-recorded data were used for the analysis. View was ensured by mounting the video camera on its stand and then placing it at the roof top of a building located near the roundabout. The data collection was done in the months of September to November 2013,which were considered to be the normal months as the traffic flow was least affected by the environmental influences during this period. The videos were captured either from 8 a.m.to 12 a.m.or from 4 p.m.to 7 p.m.on a typical clear weekday.

The traffic, especially in India and developing countries in general, is highly heterogeneous in nature. Vehicles in the traffic stream were divided into five categories, viz.small cars or standard cars (SCs), big cars (BCs), motorized two-wheelers (2Ws), motorized three-wheelers (3Ws)and heavy vehicles (HVs) [15]. Physical dimensions and rectangular plan area of these vehicles are given in Table 3.

The traffic volume data were extracted at five locations.The average traffic composition at entry and in the circulating roadway is given in Table 4.

The data of lagging headway were extracted from the recoded film when played on the computer screen. The lagging headway is defined as the time difference between the leading vehicle and the following vehicle when their rear bumper successively crosses the reference line as shown in Fig. 1. An example set of data is also shown frame by frame in Fig. 1.

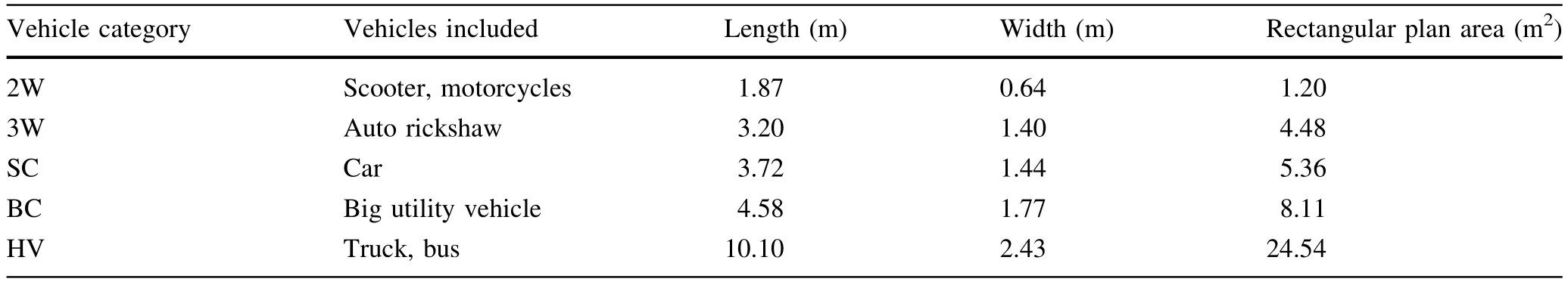
Table 3 Vehicle categories and their dimensions

Table 4 Traffic composition details at different roundabouts

Fig. 1 Frame-by-frame sample data for lagging headway

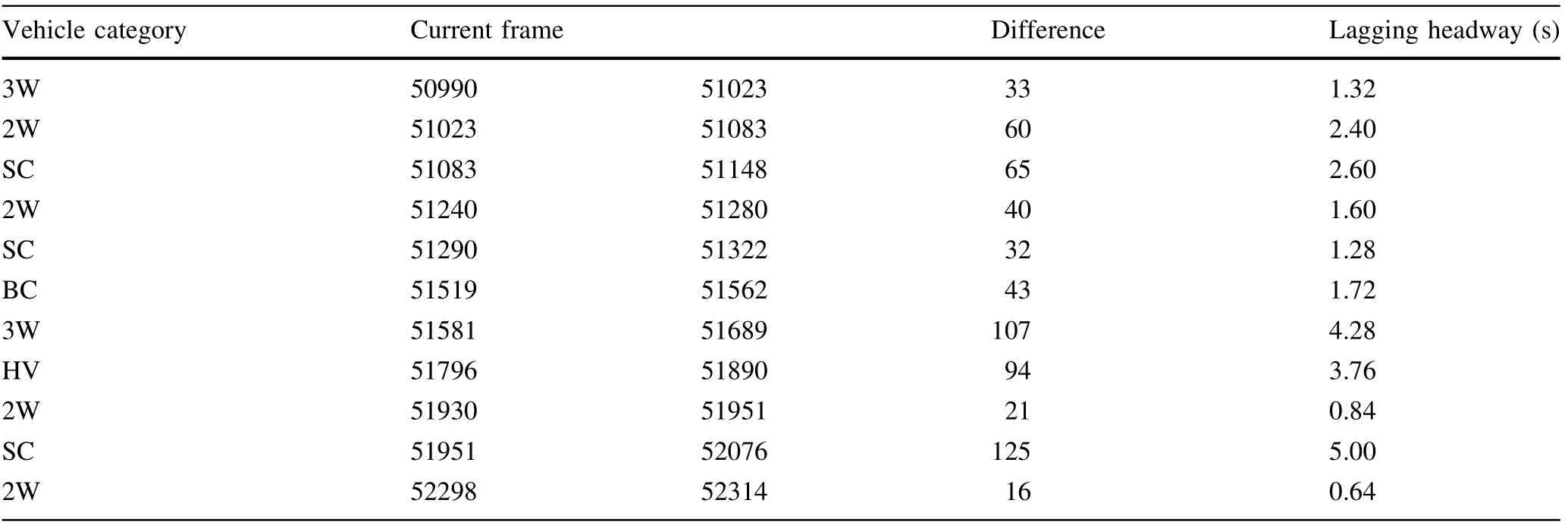
Table 5 Sample sheet for measuring lagging headway

A sample sheet showing extraction of data and estimation of lagging headway is shown in Table 5.Here,vehicle category means that the vehicle which is following and for which lagging headway is measured.

Once the data were extracted, the PCU factors were estimated for different categories of vehicles, using standard car as a base. This required to convert heterogeneous traffic into homogeneous one (in PCU units) so that the same can be used in HCM 2010 formula for estimating entry capacity.

4 Estimation of passenger car units

Mixed traffic condition generally prevails on roads in most developing countries like India. These vehicles are quite different in their static and dynamic characteristics. To account for this non-uniformity in the traffic stream, we need to convert all vehicles into PCU values [16]. The concept of PCU was initially introduced in HCM 1965,and considerable research effort has been directed toward estimation of equivalency factors for various roadway types since then [17]. PCU values used at roundabouts in India are based on old studies prior to 1976. Since then,technology in vehicles has changed. They have become operationally more efficient and maneuverable. These emphasize the need to estimate the PCU values for different categories of vehicles at roundabouts in India under changed traffic conditions and vehicle operations.

Several methods for estimating PCU values have been reported in the literature, including the headway ratio method [18, 19], the simulation method [20, 21] and the speed based method [22]. As entering vehicles or circulating vehicles in a roundabout move at almost similar speeds,speed shall not be taken as an indicator of vehicular interaction at roundabouts. Factors like the average width and length of each vehicle type and the average gap between the vehicles in the circulating traffic stream look appropriate and are considered in this study. Different categories of vehicles ply on roundabouts and occupy space laterally without lane discipline under heterogeneous traffic condition.Therefore,the vehicle width is considered to be an important parameter in PCU estimation. The proposed method utilizes the width and lagging headway of a vehicle with respect to a standard car.The vehicle length and the average gap between the vehicles constitute the lagging headway. Considering all these factors, Eq. (4) is proposed to determine the PCU of vehicle type j.

where PCUjdenotes the PCU of vehicle type j,wjthe width of vehicle type j(m),wcthe width of the standard car(m),hjthe average lagging headway of vehicle type j(s),and hcthe average lagging headway of standard car (s). The average estimated PCU values and sample sizes for different types of vehicles related to roundabout ID are given in Table 6.

The estimated values of PCU for different vehicles are found to be quite similar to those given in the literature[5, 23, 24] except for motorized two-wheelers. The estimated PCU value for motorized two-wheelers is considerably lower than that used in different countries irrespective of developed or developing ones. It is attributable to the behavior of motorized two-wheelers as they can occupy smaller spaces between two vehicles in a bid to complete their maneuver as quickly as possible.

In continuation of the above comments on the behavior of drivers of different categories of vehicles, the same was ascertained by examining the gap acceptance/rejection behavior of the drivers at a roundabout in heterogeneous condition. This is also a needed input value in HCM 2010 equation for the estimation of entry capacity of a roundabout.

Table 6 The average estimated PCU values and sample sizes for different types of vehicles

5 Critical gap and follow-up time

Different methods have been proposed in the literature to determine the critical gap for a vehicle during the past decades [25]. The maximum likelihood method (MLM) is generally reckoned to be the most acceptable method[26-28]. However, the maximum likelihood method gives very trivial results in the case of limited priority condition,where the highest rejected gap would be zero and natural log of zero would be undefined.To deal with this situation,Ahmad et al. [11] proposed a new method by minimizing the sum of absolute difference in a gap with respect to the highest rejected and accepted gaps. This method gives quite good results under heterogeneous traffic condition as compared to other existing methods and does not fail even if there is no rejection of gap,which is very common under heterogeneous traffic conditions prevailing in developing countries. The equation for estimating the critical gap is given below:

where Abs() is the absolute value function, Aiis the accepted gap by the ith entering vehicle (s), Riis the highest rejected gap by the ith entering vehicle(s),and tcis the critical gap value (s).

For estimating the critical gap (tc), the total difference(the sum of absolute values of differences) should be minimized by an iterative process. The critical gap values are estimated using this method.These are given in Table 7 for the five sites.

Follow-up times represent the process by which multiple vehicles that are queued at an approach can enter the roundabout using the same gap in the circulating flow. In this study, the follow-up time could be measured only for motorized two-wheelers and small cars. There were substantial cases of a small car following a small car or a motorized two-wheeler following a motorized twowheeler.Similar data could not be generated for the rest of the vehicles as cases of big cars, motorized three-wheelers and heavy vehicles following the respective same type of vehicles were few.

The follow-up time and the ratio of follow-up time to critical gap are given in Table 7 for motorized twowheelers and small cars. The average ratio of follow-up time to critical gap is 0.64. The reported ratio in the literature is 0.60 or more [29-31]. Therefore, the follow-up time was taken as ‘0.64 times the critical gap’ for all vehicles in this study.

The estimated PCU, critical gap and follow-up time values for different vehicle categories were used as input parameters in the HCM 2010 equation to estimate the entry capacity of a roundabout.The transferability of HCM 2010 model will be discussed in the following section.

6 Transferability of HCM 2010 model

On a multilane roundabout, the critical gap can be calculated either by considering entering lane and circulating lane separately or by combining the entering lanes andcirculating lanes into single entering and circulating streams, respectively [32]. HCM 2010 uses the first approach by considering right lane entry and left lane entry separately since the lane markings are properly positioned in the US roundabouts and vehicles maintain the lane discipline.It has been observed in India that lane markings on the roundabouts are usually absent and drivers enter the circulating area using smaller gaps without following the concept of lane. For this reason, the second approach was used to estimate the critical gap in the present study.

Table 7 The critical gap, follow-up time and their ratio

Table 8 HCM parameters under heterogeneous traffic

In a mixed traffic situation, the critical gap (tc) and follow-up time (tf) are different for different types of vehicles (Refer to Table 7). To address this problem, Dahl and Lee [33] suggested the use of volume weighted average of critical gap values. The same approach is also used in this study, and the critical gap value for a mixed traffic stream (tcm) is calculated using Eq. (6).

where tciis the critical gap for vehicle type i (s), and Piis the proportional share (fraction) of vehicle type i in the traffic stream on a subject approach.

The HCM 2010 equation is modified for heterogeneous traffic conditions using modified values of tcmand tf. Calculated values of the HCM parameters under heterogeneous traffic conditions are given in Table 8.

The entry capacity calculated using modified HCM model is compared with the field values. The circulating traffic flow and entry traffic flow data were collected during periods of continuous and stable queuing at the entry leg of the roundabout. These data were extrapolated to the equivalent hourly flow as suggested by Al-Masaeid and Faddah [34]. The scatter plots of entry capacity (Ce) and circulating traffic flow (Vc) at five roundabouts are shown in Fig. 2.The field capacity follows an exponential relation with circulating flow, as also suggested in the literature[10, 13, 34]. The specific Ce-Vcrelation fitted from field data is given by Eqs. (7)-(11) for respective roundabouts:For roundabout R1:

The modified HCM 2010 equations(parameters given in Table 8) are also plotted in Fig. 2 for respective sites. As can be seen, the field model follows the modified HCM model at roundabouts R1,R2and R3.The gap between entry capacities estimated from modified HCM model and the field model for roundabouts R4and R5has widened. The average gap between the plots of field model and modified HCM model is 60, 50, 100, 225 and 295 PCU/h for roundabouts R1-R5, respectively. That is, the gap between modified HCM model and the field model is increasing with the size of the roundabout.This is attributed to that the estimated stream critical gaps at roundabouts R4and R5are nearly the same as that estimated at roundabouts R2and R3.It can be inferred that the stream critical gap value becomes constant and the entry capacity using modified HCM equations does not increase for the larger size of the roundabouts. However, the field entry capacity increases with an increase in the size of the roundabout.This result is consistent with the findings of the previous study, which reported that the entry capacity increases as its diameter increases [35].

Fig. 2 Comparison between field data and modified HCM model

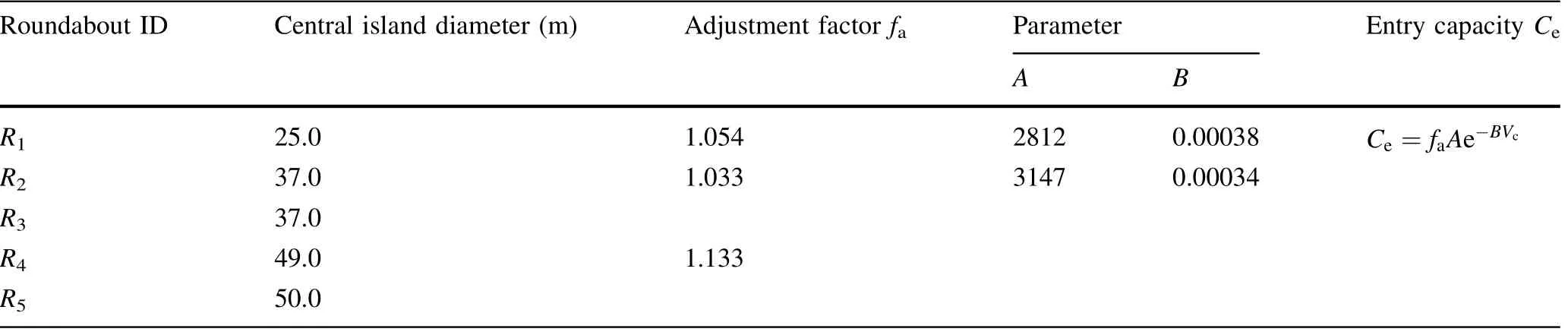
Table 9 Final calibrated HCM model and adjustment factors for different sizes of roundabouts

It may be noted here that the modified HCM models are found differing from the field models.Therefore,there is a need to use a multiplicative adjustment factor in modified HCM models to satisfy the traffic condition prevailing in developing countries like India. The stream critical gap values at roundabouts R4and R5are nearly the same as that estimated at roundabouts R2and R3. Therefore, roundabouts R2, R3, R4and R5are grouped together for further analysis. The adjustment factor (fa) is defined as the ratio between the field entry flow value and that given by the modified HCM model. The final calibrated HCM model and adjustment factors for different sizes of roundabouts are given in Table 9.

7 Validation of the model

A test has been made to validate the proposed model. The field capacity values were extracted at another roundabout having central island diameter of 51 m, in the same city(sector 42-43-52-53).The circulating roadway width,entry width and exit width of the selected roundabout were 10.5,12.0 and 12.0 m, respectively. The field entry capacity values of the selected roundabout have been compared with those estimated by the proposed model. These values are plotted against each other,as shown in Fig. 3.As observed in Fig. 3,the R2value for the plot between the field values and estimated values by the proposed model is 0.7239,when the constant was fixed at zero, indicating the good agreement between the two values. The field capacity values differed by ± 4% from the estimated ones.

Fig. 3 Comparison between field and calibrated HCM models

Table 10 The z test: two samples for means

A z test statistical analysis has been used to compare the means of field capacity values and estimated values by the proposed model. The null hypothesis (H0) is ‘There is no significant difference between the mean of field capacity values and estimated values by proposed model.’ The results of z test analysis are given in Table 10.Based on the p-value and t-stat,the null hypothesis is not rejected at 95%level of confidence and there is no significant difference between the means of both capacity values.

The calibrated HCM models are also compared with the field models to see the difference between these models.These are plotted in Fig. 4 for roundabouts with central island diameters of 25, 37 and 50 m. The field entry capacity model for roundabout R1is differing by only± 1% from the calibrated HCM model, and ± 2% and± 1% for roundabouts R2and R3, respectively. The field entry capacity models for roundabouts R4and R5follow the calibrated HCM model. The difference between field and calibrated HCM models is less than ± 1% for these roundabouts.

8 Conclusions

Fig. 4 Field entry capacity versus estimated entry capacity

Table 11 Final calibrated HCM model and adjustment factors for different sizes of roundabouts

Data collected at five roundabouts in India were used to calibrate the HCM 2010 equation for estimating the entry capacity under heterogeneous traffic condition. The analysis is presented in three parts: estimation of PCUs,estimation of critical gap for different categories of vehicles and modification to HCM equation for its use in developing countries catering to heterogeneous traffic.The estimation of PCU values for different categories of vehicles was based on the vehicle width and lagging headway.The PCU values obtained by this method are partly comparable with those given in the literature. PCU values, as given in Table 6, may be used at roundabouts under heterogeneous traffic in developing countries like India.The critical gap values obtained under heterogeneous traffic conditions are very low when compared with those given in the literature for homogeneous traffic condition.They are less than 2.67 s and increase with an increase in the size of the vehicle. Low values of critical gap also signify the impatience behavior of drivers under heavy heterogeneous flow in developing countries. The ratio of follow-up time to critical gap is found to be 0.64.

The HCM 2010 equation for estimating the entry capacity was modified for its adaptation to heterogeneous traffic conditions.Parameters A and B of the HCM equation were calculated on the basis of critical gap values obtained from the field data. The entry capacity estimated from the modified HCM equations was compared with the field entry capacity,showing that the modified HCM models are different from the field models. Therefore, an attempt to use an adjustment factor in modified HCM models is made to satisfy the traffic condition prevailing in developing countries like India. The final calibrated HCM model and adjustment factors (fa) for different sizes of roundabouts are given in Table 11. The approach presented here is different than that used in HCM 2010 which is based on number of lanes at entry and circulating roadway. As already mentioned, the drivers are not following the lane discipline in developing countries, and it is not wise to replicate the HCM 2010 approach here. Rather, the data suggest that traffic behavior on roundabouts is carriageway based and differs with respect to the size of the roundabout.Hence, final models are mainly governed by size, with tentative difference due to number of lanes at entry and circulating roadway,and are being taken care of by a factor‘fa.’

The entry capacity estimated from calibrated HCM model was compared with the field entry capacity at another roundabout,and the results were found consistent.Therefore,the final calibrated HCM models are suitable for estimating the entry capacity at roundabouts under heterogeneous traffic conditions in developing countries like India.This also confirmed our hypothesis that HCM model needs to be calibrated before applying to heterogeneous traffic condition prevailing in developing countries like India.

Open AccessThis article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use,distribution,and reproduction in any medium,provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.