雷达伺服系统的高跟踪精度改进型自抗扰控制器
姜仁华 刘闯 宁银行 谢洋


Abstract:In order to solve the problem that tracking accuracy of radar servo system is affected by the disturbance quantity of airborne platform and the acceleration of object, the improved auto-disturbance-rejection control (I-ADRC) method, with high tracking precision, is proposed. Considering more disturbance factors of airborne radar platform, such as the mechanical vibration, airflow, environment temperature, and attitude change of airborne, the model compensation method was introduced in the I-ADRC to reduce the load for extended state observer (ESO), which overcomes the observation accuracy decrease for the ESO caused by the large disturbance in traditional ADRC. Moreover, in the I-ADRC, the feed-forward compensation of acceleration was also adopted to eliminate the model error of servo system resulted from the acceleration of maneuvering target. Compared with traditional PI control, the airborne radar servo system with the proposed I-ADRC show its more high accuracy and more strong anti-interference, and the system can quickly track the target in the state of acceleration.
Keywords:airborne radar servo system; platform disturbance; acceleration; auto-disturbance-rejection control; model compensation; feed-forward compensation
0 引 言
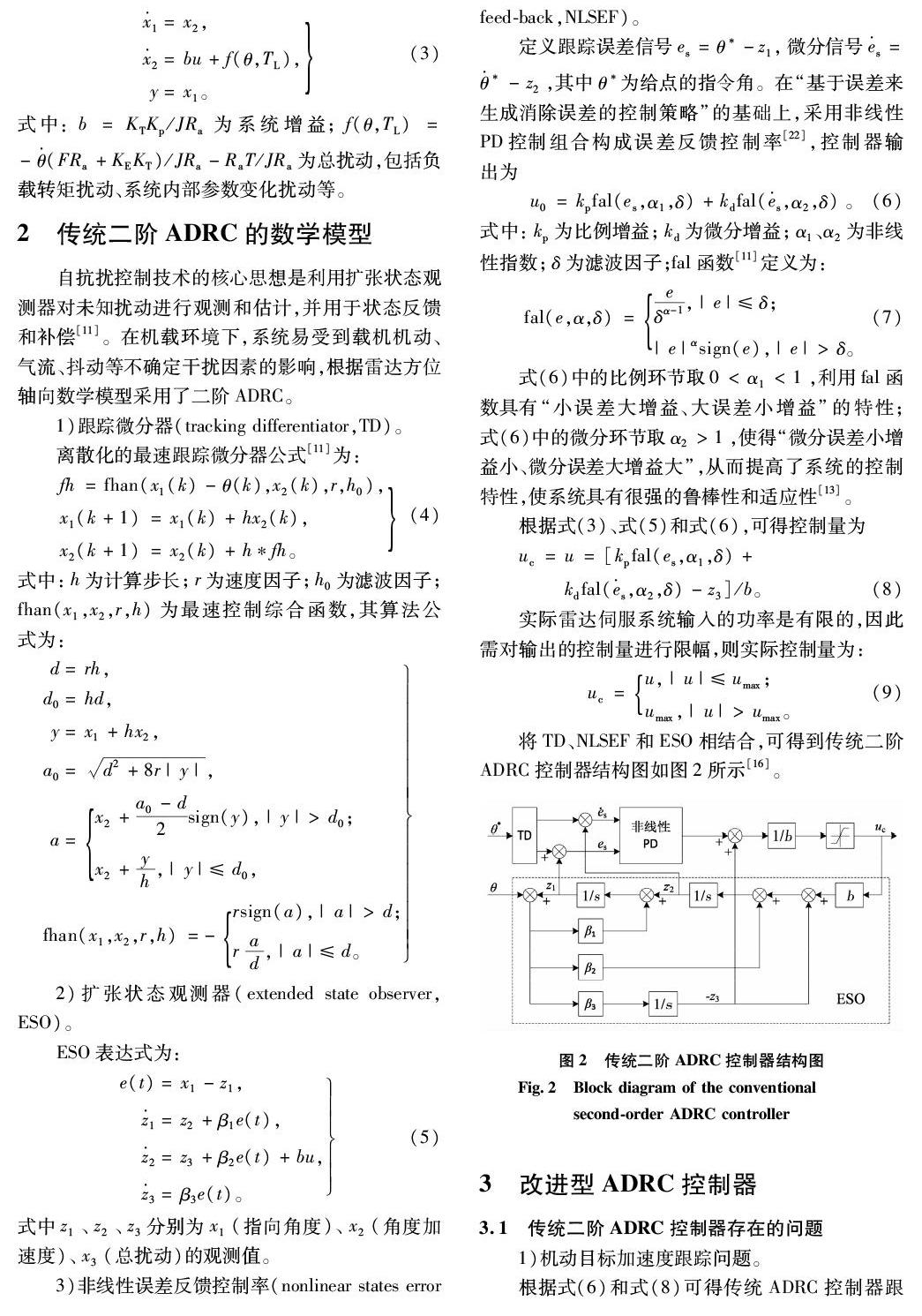
位置伺服系統是机载雷达的重要组成部分,其控制性能的好坏直接影响雷达的目标探测实时性和稳定性等性能。在雷达进行目标跟踪时,伺服机构易受到载机姿态、振动、气流等外部干扰及目标位置随动的影响,因此雷达位置伺服系统应具有高静态稳定性、动态响应能力,以及较强的抗扰动能力[1-2]。传统雷达位置伺服系统的控制仍以PID控制为主,PID控制虽然结构简单,但针对机动目标位置跟踪时,存在跟踪滞后,同时易受外部扰动的影响。随着现代战争目标机动能力的提升,传统PID控制难以满足高性能跟踪要求[3]。近年来,随着微处理器和现代控制理论的发展,非线性PID控制[4-6]、滑模变结构控制[7]和时间最优控制[8-9]等多种先进控制策略应用于位置伺服系统中,但由于控制结构复杂或系统功率受限等问题,限制了在雷达伺服系统中的应用。
韩京清研究员在分析了PID控制技术的基础上,提出了一种自抗扰控制(active disturbance rejection control, ADRC)新型非线性控制方法,该控制方法具有良好的动/静态特性,利用扩张状态观测器对内外部负载扰动进行提前观测和补偿,具有良好的鲁棒性[10-13]。自抗扰控制技术已经广泛应用于位置伺服系统各种控制领域[14-17],并达到了很好的抗扰动性能。但是,在机载雷达伺服系统中进行目标航迹跟踪时,伺服系统受载机平台扰动及目标加速度等因素影响,扩张状态观测器观测的扰动量变化幅度大,观测器负担重,难以保证扰动量获得很高的估计精度,且跟踪雷达的主瓣波束一般较窄,导致动态滞后过大而使目标超出雷达的主瓣波束宽度,造成目标丢失[18]。文献[19-20]采用模型补偿自抗扰控制器,将扰动中的已知部分分离出来以降低扩张状态观测器观测的扰动量幅度,提高观测精度。文献[21]在采用模型补偿应用与二阶自抗扰控制器的基础上,引入输入微分前馈补偿自抗扰控制器的建模误差,通过仿真和实验验证减少了时变速度输入的跟踪误差。
针对机载雷达伺服系统工作环境特点,在借鉴文献[19-21]设计思路的基础上,将模型补偿自抗扰控制方法应用于跟踪雷达位置伺服系统中,提出了一种基于改进型模型补偿的自抗扰控制方法,将系统已知扰动通过计算分离出来,降低观测器的估计幅度,提高估计精度,同时针对目标位置角加速度问题,通过输入前馈补偿的方式消除建模误差,提高对机动目标的跟踪精度。最后在某型雷达伺服产品中进行了实验验证,提高了载机的作战效能,具有较大的军事和经济意义。
1 机载雷达伺服系统模型
以雷达伺服系统方位轴向控制为研究对象,方位轴向系统数学模型如图1所示。
5 结 论
将自抗扰控制技术应用于机载雷达位置伺服系统中,由于载机平台对伺服系统扰动较大,通过模型补偿的方式降低扩张状态观测器扰动观测量,提高扰动估计精度,通过前馈补偿的方式引入机动目标的加速度信号,减少自抗扰控制建模误差,从而提高系统的对机动目标的跟踪精度。实验表明,采用改进型自抗扰控制器的雷达伺服系统,相对于传统ADRC控制和模型补偿ADRC控制,在匀速扫描和目标跟随过程中,伺服系统的跟随精度有明显的提高,有效地抑制了载机平台扰动和目标机动加速度对雷达伺服系统的影响,提高了系统的抗干扰性和跟随精度。
参 考 文 献:
[1] 蒋兵兵,盛卫星,张仁李,等. 雷达目标角度跟踪环路滤波器设计新方法[J].电子与信息学报,2015,37(12):2948.
JIANG Bingbing, SHENG Weixing, ZHANG Renli,et al. A novel design method of radar target angle tracking loop filter[J]. Journal of Electronics and Information Technology,2015,37(12):2948.
[2] 何辉文,彭程,赵梅,等. 雷达伺服系统的频域辨识与控制方法研究[J]. 现代雷达,2013,35(9):53.
HE Huiwen, PENG Cheng, ZHAO Mei, et al. A study on frequency domain identification and control method in radar servo system[J]. Modern Radar, 2013,35(9):53.
[3] 朱莹,王金广,高其娜,等. 噪声干扰下雷达角度跟踪时滞伺服系统仿真[J]. 系统仿真学报,2014,26(8):1814.
ZHU Ying, WANG Jinguang, GAO Qina, et al. Noise jam on angle tracking radar delay servo system simulation[J]. Journal of System Simulation, 2014,26(8):1814.
[4] 韩京清. 非线性PID控制器[J]. 自动化学报,1994,20(4): 487.
HAN Jingqing. Nonlinear PID controller[J]. Acta Automatica Sinica,1994,20(4): 487.
[5] 陈幼平,张代林,艾武,等. 基于DSP的直线电机位置伺服控制策略研究[J]. 电机与控制学报,2006,10(1): 61.
CHEN Youping, ZHANG Dailin, AI Wu, et al. Research on the DSP-based control strategies of a positioning servo system of the linear motors[J]. Electric Machines and Control,2006,10(1): 61.
[6] 王江,王静,费向阳. 永磁同步电动机的非线性PI速度控制[J]. 中国电机工程学报,2005,25(7):125.
WANG Jiang, WANG Jing, FEI Xiangyang. Nonlinear PI speed control of permanent magnet synchronous motor[J]. Proceedings of CSEE,2005,25(7):125.
[7] 姜静, 伍清河. 滑模变结构控制在跟踪伺服系统中的应用[J]. 电机与控制学报, 2005, 9(6): 562.
JIANG Jing, WU Qinghe. Sliding mode variable structure control for the tracking servo system[J].Electric Machines and Control, 2005, 9(6): 562.
[8] 許天舒, 史小平. 一类不确定非线性系统的最优控制[J]. 哈尔滨理工大学学报, 2000, 5(6): 82.
XU Tianshu, SHI Xiaoping. Optimal control of a class of uncertain nonlinear systems[J]. Journal Harbin University of Science and Technology, 2000, 5(6): 82.
[9] 胡金高,程国扬. 鲁棒近视时间最优控制及其在电机伺服系统的应用[J]. 电工技术学报,2014,29(7):163.
HU Jingao, CHENG Guoyang.Robust procimate time-optimal control with application to a motor servo system[J]. Transactions of China Electrotechnical Society,2014,29(7):163.
[10] 韩京清. 自抗扰控制器及其应用[J]. 控制与决策,1998,13(1):19.
HAN Jingqing. Auto-disturbances-rejection controller and its applications[J].Control and Decision,1998,13(1): 19.
[11] 韩京清. 自抗扰控制技术—估计补偿不确定因素的控制技术[M]. 北京:国防工业出版社,2008.
[12] 高志强. 自抗扰控制思想探究[J]. 控制理论与应用,2013,30(12):1498.
GAO Zhiqiang. On the foundation of active disturbance rejection control[J].Control Theory and Application,2013,30(12):1498.
[13] 王丽君,李擎,童朝南,等. 时滞系统的自抗扰控制综述[J]. 控制理论与应用,2013,30(12):1521.
WANG Lijun, LI Qing, TONG Chaonan, et al. Overview of active disturbance rejection control for systems with time-delay[J]. Control Theory and Applications,2013,30(12): 1521.
[14] 丛麟骁,黄旻,赵宝玮. 自抗扰控制在航空成像稳定平台上的应用[J]. 光电工程,2014,41(9): 38.
CONG Linxiao, HUANG Min, ZHAO Baowei. ADRC application on a stable platform for aerial imaging[J]. Opto-Electronic Engineering, 2014, 41(9): 38.
[15] 周涛,朱景成. 机载光电跟踪平台伺服系统自抗扰控制[J]. 光电工程,2011,38(4):31.
ZHOU Tao, ZHU Jingcheng. An active disturbance rejection controller in the servo system of an air-borne opto-electronic tracking platform[J]. Opto-Electronic Engineering, 2011, 38(4): 31.
[16] 魏永清, 許江宁, 马恒. 自抗扰控制器在陀螺稳定平台控制系统中的应用[J]. 电机与控制学报, 2017, 21(1): 39.
WEI Yongqing, XU Jiangning, MA Heng. Application of active disturbance rejection controller in gyro-stabilized platform control system[J]. Electric Machines and Control, 2017, 21(1): 39.
[17] 腾福林,胡育文,李宏胜,等. 基于自抗扰控制器的交流位置伺服系统[J]. 电气传动,2011,41(11): 46.
TENG Fulin, HU Yuwen, LI Hongsheng, et al. AC position servo system based on active-disturbance rejection controller[J]. Electric Drive, 2011, 41(11): 46.
[18] 万其,邵兵,胡芳芳. 雷达伺服系统中非线性控制技术研究[J]. 现代雷达,2010,32(8):70.
WAN Qi, SHAO Bing, HU Fangfang. A study on non-liner control technology in radar servo systems[J]. Modern Radar, 2010, 32(8):70.
[19] 刘志刚,李世华. 基于永磁同步电机模型辨识与补偿的自抗扰控制器[J]. 中国电机工程学报,2008,28(24):118.
LIU Zhigang, LI Shihua. Active disturbance rejection controller based on permanent magnetic syschronous motor model identification and compensation[J]. Proceedings of the CSEE, 2008, 28(24): 118.
[20] 盖江涛,黄庆,黄守道,等. 基于模型补偿的永磁永磁同步电机自抗扰控制[J]. 浙江大学学报(工学版),2014,48(4): 581.
GAI Jiangtao, HUANG Qing, HUANG Shoudao, et al. Active-disturbance rejection controller for permanent magnet synchronous motor based on model compensation[J]. Journal of Zhejiang University (Engineering Science), 2014, 48(4): 581.
[21] 左月飞,张捷,刘闯,等. 针对时变输入的永磁同步电机改进型自抗扰控制器[J]. 电工技术学报,2017,32(2):162.
ZUO Yuefei, ZHANG Jie, LIU Chuang, et al. A modified adaptive disturbance rejection controller for permanent magnetic synchronous motor speed-regulation system with time-varying input[J]. Transactions of China Electrotechnical Society, 2017, 32(2): 162.
[22] 韩京清. 利用非线性特性改进PID控制律[J]. 信息与控制,1995,24(6): 358.
HAN Jingqing. The improvement of PID control law by using nonlinearity[J]. Information and Control, 1995, 24(6): 358.
[23] 韩京清,张荣. 二阶扩张状态观测器的误差分析[J]. 系统科学与数学,1999,19(4): 465.
HAN Jingqing, ZHANG Rong. Error analysis of the second order ESO[J]. Journal of Systems Science and Mathematical Sciences, 1999, 19(4): 465.
[24] 张文革. 时间尺度与自抗扰控制器[D]. 北京:中国科学院系统科学研究所,1999.
(编辑:邱赫男)

