Establishment of probabilistic model for Salmonella Enteritidis growth and inactivation under acid and osmotic pressure

Yujio Shi,Hong Liu,Bozhng Luo,Yngti Liu,Siyun Yue,Qing Liu,Qingli Dong,*

a School of Medical Instrument and Food Engineering,University of Shanghai for Science and Technology,Shanghai 200093,PR China

b Department of Food Hygiene,Shanghai Municipal Center for Disease Control and Prevention,Shanghai 200336,PR China

Abstract The growth and survival characteristic of Salmonella Enteritidis under acidic and osmotic conditions were studied.Meanwhile,a probabilistic model based on the theory of cell division and mortality was established to predict the growth or inactivation of S.Enteritidis.The experimental results demonstrated that the growth curves of planktonic and detached cells showed a significan difference (p ＜0.05) under four conditions,including pH5.0+0.0%NaCl,pH7.0+4.0%NaCl,pH6.0+4.0%NaCl,and pH5.0+4.0%NaCl.And the established primary and secondary models could describe the growth of S.enteritis well by estimating four mathematics evaluation indexes,including determination coefficien (R2),root mean square error (RMSE),accuracy factor (Af) and bias factor (Bf).Moreover,sequential treatment of 15% NaCl stress followed by pH 4.5 stress was the best condition to inactivate S. Enteritidis in 10 h at 25°C.The probabilistic model with Logistical or Weibullian form could also predict the inactivation of S.Enteritidis well,thus realize the unificatio of predictive model to some extent or generalization ofinactivation model.Furthermore,the primary 4-parameter probabilistic model or generalized inactivation model had slightly higher applicability and reliability to describe the growth or inactivation of S.Enteritidis than Baranyi model or exponential inactivation model within the experimental range in this study.

Keywords: Acid;Osmotic pressure;Salmonella Enteritidis;Probabilistic model;Unification Generalization

1.Introduction

In recent years,foodborne pathogens have caused a lot of serious human infections[1,2],and salmonellosis rank the top of bacterial foodborne infections all around the world[2,3].In particular,S.Enteritidis has brought more threat to human’s health than other serotypes ofSalmonellaspp.[4].In addition,acid and osmotic pressure are two hurdle factors commonly used in food processing to ensure food safety[5],and have been proved to be effective on inhibiting the growth and inactivation ofSalmonellaspp.[6,7].Therefore,studying the growth and inactivation ofS.Enteritidis under acid and osmotic pressure during food processing could reduce the foodborne illness caused bySalmonellaand improve the accuracy of quantitative microbial risk assessment[8].

In addition,Salmonellacould contaminate the production lines and attach on the surfaces of equipment for a long time[9].The attached bacterial cells could enter other foods and form detached cells through direct or indirect contact with food and the surface of the production equipment[10].And the planktonic cells are also one of the important sources of food contamination [7].So studying and comparing the growth of planktonic and detachedS.Enteritidis cells under new environment could help understand how to control the growth ofS.enteritis with different states better.

Different sequential treatments of acid and osmotic pressure may influenc the inactivation of foodborne pathogens.Some researchers have explored the best processing sequence to inactivate pathogens more efficientl [11-13].Shadbolt et al.[11]demonstrated that sequential treatment with acid(pH 3.5)and then 10% NaCl stress had better effect on inactivatingEscherichia colithan the treatment with reverse sequence or singlestressordoublestressesusedsimultaneouslyduring simulated fermentation and maturation of meat and cheese.However,the effect of sequential treatments of acid and osmotic pressure on inactivation ofS.Enteritidis has been rarely reported,and remains to be further verified

In the past few years,mathematical models have been used to predict microbial growth,e.g.Baranyi model,Gompertz model,and Logistic model,etc.[14-16].Usually,such above mentioned models have been established on the assumption that microbial growth curves have three periods,i.e.lag phase,exponential growth phase,and stationary phase,respectively[17,18],and these models were also developed at microbial population level.With the progress of detecting technology,a f ow imaging system [19],microfluidic device [20]and flw cytometry[21]were typically applied for the research ofindividual bacterial cells.Meanwhile,the models based on individual cell level were also gradually developed and proved to be useful to simulate the growth and division ofindividual bacterial cells,e.g.individual-based models (IbMs) [22]and the stochastic model[23].

In addition,microbial growth and inactivation have been generally considered as different phenomenon,and predicted by different mathematical models [24].Some attempts have been done to realize the unificatio of predictive models for microbial growth and inactivation simultaneously,e.g.Baranyi et al.[25]attempted to mirror the microbial inactivation as the microbial growth,and then used Baranyi model [14]to describe microbial inactivation successfully.However,this mirroring modeling method has been questioned academically due to the nature of microbiological behavior,so the approach to describe and combine microbial growth and inactivation better needs to be further explored.

Research about the probabilistic modeling approach at individual cell level has been reported previously.Such as Horowitz et al.[24]established probabilistic models for single cells and large-population cells,and the established models were based on the assumption that an alive cell would become one of three states,i.e.division,mortality or being alive,respectively,with different probabilistic values.However,the probabilistic model was only primary model reflectin the numbers of bacteria over time,the secondary model describing the effectiveness of environmental conditions on the fitte parameters of probabilistic models has been seldom established.

Therefore,the aims of this study were to(a)model the effect of acid and osmotic pressure onS.Enteritidis growth and inactivation through a probabilistic modeling approach based on the theory of cell division and mortality; (b) develop a secondary model forS.Enteritidis growth;and(c)compare the established probabilistic models forS.Enteritidis growth and inactivation with Baranyi model or exponential inactivation model within the experimental range.

2.Materials and methods

2.1.Inoculum preparation

One strain ofS.Enteritidis(ATCC 13076),stored in preservative tube for strain at-80°C refrigerator,was activated firstl in Tryptose Soya Broth(TSB),then incubated on Tryptose Soya Agar (TSA),and maintained at 4°C until ready for use.One colony ofS.Enteritidis was picked up from TSA and transferred into 100 mL sterile TSB in a 250 mL flask The flas was incubated in a biological shaker(THZ-103B,Shanghai Yiheng Instrument Co.Ltd.,Shanghai,P.R.China)at 110 rpm for 16 h at 37°C until the target microorganism reached stationary phase.The inoculum was harvested by centrifugation at 6000 rpm for 10 min at 4°C,washed twice and resuspended in 10 mL sterile saline solution approaching 109CFU/mL and taken as the working culture solution.

2.2.Growth trials

A full factorial design with three pH values(5.0,6.0 and 7.0)and three NaCl contents(0.0,2.0,and 4.0%)was applied to the growth experiments according to previous reports [6,26].The condition of pH 7.2 and 0.5%NaCl was chosen as the control treatment.Different levels of pH and NaCl were obtained by adding lactic acid,sodium hydroxide and sodium chloride.

Sterile stainless steel(SS)coupons(Grade 304,50 mm×20 mm×0.8 mm,No.2b finish were selected as the material to obtain the cells adhered on a solid surface.Each SS coupon was immersed in 30 mL TSB(pH 7.2+0.5%NaCl)inoculated with 0.5 mLS.Enteritidis solution in sterile centrifuge tubes.The tubes were placed in a high precision incubation chamber(HWS-150,Shanghai Bilang Instrument Co.Ltd.,Shanghai,P.R.China)for 72 h at 25°C(±0.5°C)under static conditions.

After incubation for 72 h,ten (10) mL bacterial suspension was removed from the centrifuge tube to harvest the planktonic cells by centrifugation.The cells were washed twice and re-suspended in sterile saline solution.The SS coupons were removed from the centrifuge tubes to detach the cells adhered on the surfaces.The cells on the SS coupons were obtained by the bead vortexing method [27].The planktonic and detached cells were diluted with sterile saline solution to obtain inoculum approximating 105CFU/mL,and then inoculated in TSB with different acid and osmotic pressures at 25°C.Samples of bacterial suspension were periodically removed from TSB untilS.Enteritidis reached the stationary phase and surface plated on Hektoen Enteric(HE)agar after serial dilution.The numbers ofS.Enteritidis were counted after the HE plates were incubated for 24 h at 37°C.Two replicates of experiments were conducted for each environmental condition.

2.3.Inactivation trials

To study the effect of different sequential treatment of acid and osmotic pressure onS.Enteritidis inactivation,cultures were exposed to different environmental conditions,including (1)stress with 15% NaCl in TSB for 10 h (15% NaCl stress for simplifie expression);(2)stress in TSB at pH 4.5 for 10 h(pH 4.5 stress);(3)stress in TSB with 15%NaCl and pH 4.5 simultaneously for 10 h(15%NaCl+pH 4.5 stress);(4)stress in TSB with 15%NaCl firs for 5 h and then at pH 4.5 for another 5 h(15%NaCl-pH4.5 stress);and(5)stress in TSB at pH 4.5 firs for 5 h and then with 15% NaCl for another 5 h (pH 4.5-15%NaCl stress).In each combination,TSB was either added with NaCl to adjust the osmotic pressure or with lactic acid or NaOH to adjust the pH as the conditions required.

For each environmental condition,one(1)mL working culture solution ofS.Enteritidis was inoculated into each flas containing 100 mL sterile TSB.Then all flask were placed in a shaker with the speed of 60 rpm at 25°C.The numbers ofS.Enteritidis at the particular time intervals were observed according to the methods mentioned above.Two replicates were conducted for each environmental condition.

2.4.General probabilistic model at individual cell level

Due to the study of Horowitz et al.[24],a probabilistic model was assumed that an alive cell might divide,die,or be alive(neither divide nor die)with different probabilistic values after a short time interval,ignoring the situation under which the cell might adapt,get injured,or recover from injury.The probabilistic model based on cellular level could predict the behavior of microbial population.Consequently,the probabilistic model would also be helpful to describe the growth and inactivation ofS.Enteritidis under acid and osmotic pressure in this study.The integral form of general probabilistic model for microbial population was shown as follows:

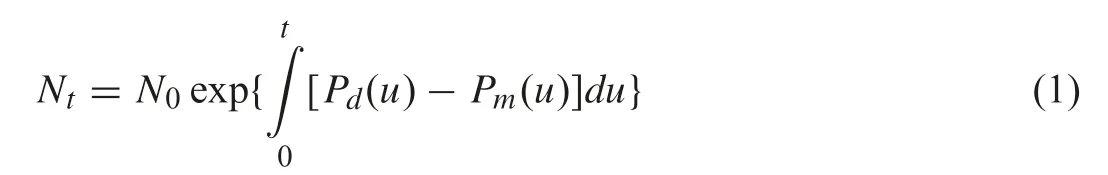
Wheretisthetime(h);N0orNtistheinitialnumbersorpredicted numbers of bacterial cells at 0 ort(CFU/mL);uis a dummy variableoft;Pd(t)orPm(t)isthedivisionormortalityprobability rate function,meaning the probability of division or mortality per unit time,andPd(t) andPm(t) became the probability of division or mortality when multiplied by a short time interval.

2.5.Establishment of primary probabilistic model for S.Enteritidis growth

Pd(t) orPm(t) could be define by Logistic expression as follows[24]:

Wherepdorpmis the asymptotic value ofPd(t) orPm(t);kdorkmis the maximum specifiincrease rate ofPd(t) orPm(t);andtdortmis the corresponding time required to the maximum specifiincrease rate ofPd(t)orPm(t),respectively.

The probability function limitg(t)was define as follows:

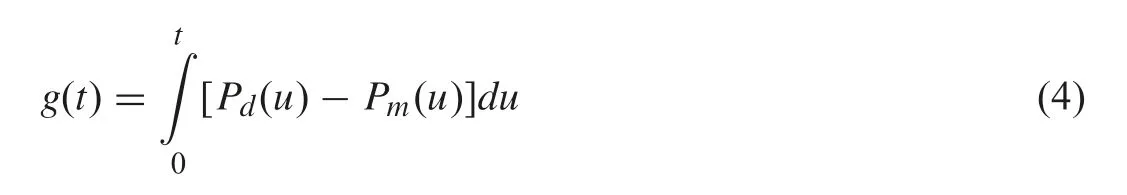
Then the Eqs.(2)and(3)were plugged into Eq.(4),andg(t)would become the form as follows:

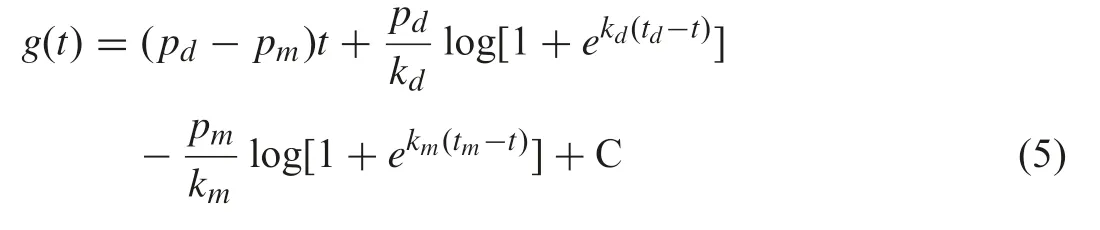
And

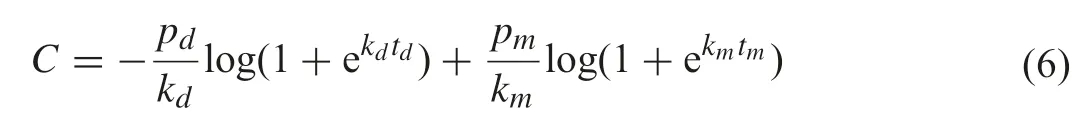
WhereCis a constant term which comes from the integral of Eq.(4),and it can improve the accuracy of predictive model.

So Eq.(1)could be transformed as follows:

Eqs.(5)-(7) were the probabilistic models forS.Enteritidis growth.Notice that the above models had six parameters,and the parameters could be simplifie as four parameters whenpd=pm=pandkd=km=k,or as f ve parameters whenkd=km=konly.For the purpose of simplifying models,4-parameter and 5-parameter models were applied to fithe growth curves of planktonic or detached cells under different acid and osmotic pressure using Origin 9.0 software(OriginLab Corporation,Northampton,USA).The model with better goodness-of-fiwas obtained by comparing the values ofR2orChi-Sqr.

2.6.Establishment of secondary model for S.Enteritidis growth

The modifie square root model[28]was applied to establish the secondary model using Matlab R2014a software (Math-Works Corporation,USA).The relation between awand the contentofNaClcouldbefoundinthestudyofChirifeandResnik[29].The modifie square root model was as follows:

Wherezrepresentsthefourorfieparametersoftheprobabilistic model;bis a constant;and the values ofawminandpHminare theoretical minimum awand pH from parameter estimation.

The determination coefficien (R2),root mean square error(RMSE),accuracy factor(Af)and bias factor(Bf)[30,31]were used to evaluate the goodness-of-fiof the secondary model.The mathematic evaluation formulas were as follows:

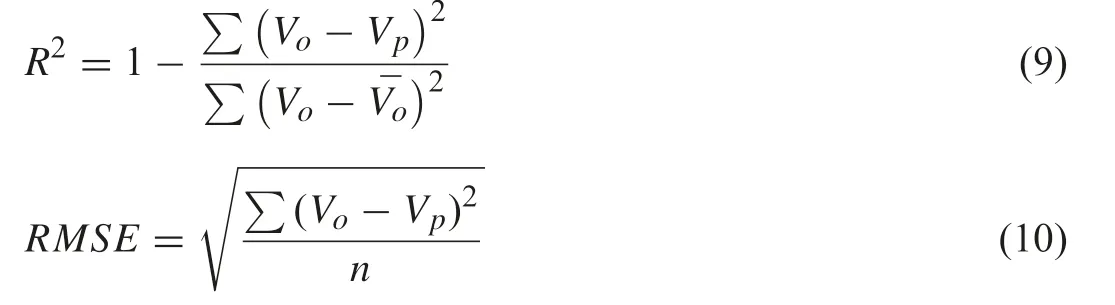
WhereVois the observed value;Vpis predicted value;andnis numbers of observed values.

2.7.Establishment of unitized predictive model

Microbial inactivation phenomenon would arise whenPm(t)＞0 andPd(t)=0 orPm(t)＞＞Pd(t) according to Eq.(1)[32].So Eqs.(5)-(7) could be simplifie as 3-parameter or 5-parameter model whenPd(t)=0 orkd=km=k,respectively.Then the 3-parameter and 5-parameter models could be applied to fithe survival curves with a fla shoulder using Origin 9.0 software,thus realize the unificatio of growth and inactivation model to some extent.

2.8.Establishment of generalized inactivation model

Microbial survival curves might have other shapes such as upward(“tailing”)and downward(“shoulder”)concavities[33].So 3-parameter and 5-parameter models couldn’t be used for the survival curves with all shapes.Among some previous studies[34,35],Weibullian model has the ability to describe both kinds of survival curves and log-linear ones.Therefore,the model with Weibullian form was applied to describe the inactivation ofS.enteritisat each stage of each environmental conditions,thus to realize the generalization ofinactivation model.

AssumingPm(t)＞0 andPd(t)=0,the model would be further simplified So Eq.(1)would become the following form:

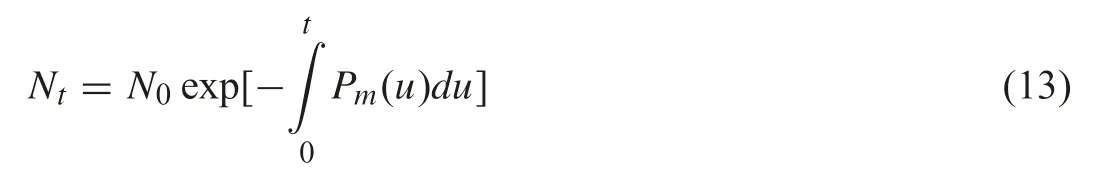
ThenPm(t)was define as follows:

So

Where bothbandnare coefficient related to environment factors.

The Eq.(16)was applied to fithe survival curves ofS.Enteritidis under different processing sequences of acid and osmotic pressure.

2.9.Comparison of probabilistic and traditional models

Baranyi model [14]or exponential inactivation model [35]is one of the widely used growth model or inactivation model,and has good application to predict the growth or inactivation of bacteria [36].The growth curves of planktonic or detachedS.Enteritidis were fitte by Baranyi model [14]using DMFit 3.5 software (Institute of Food Research,UK).The survival curves ofS.Enteritidis were fitte by the exponential inactivation model[35].Then the primary probabilistic model(Eqs.(5)-(7)) were compared with Baranyi model,and generalized inactivation model (Eq.(16)) was compared with exponential inactivation model.And four mathematic evaluation indexes,i.e.R2,RMSE,AfandBf,were used to compare the fittin effect of probabilistic and traditional models.

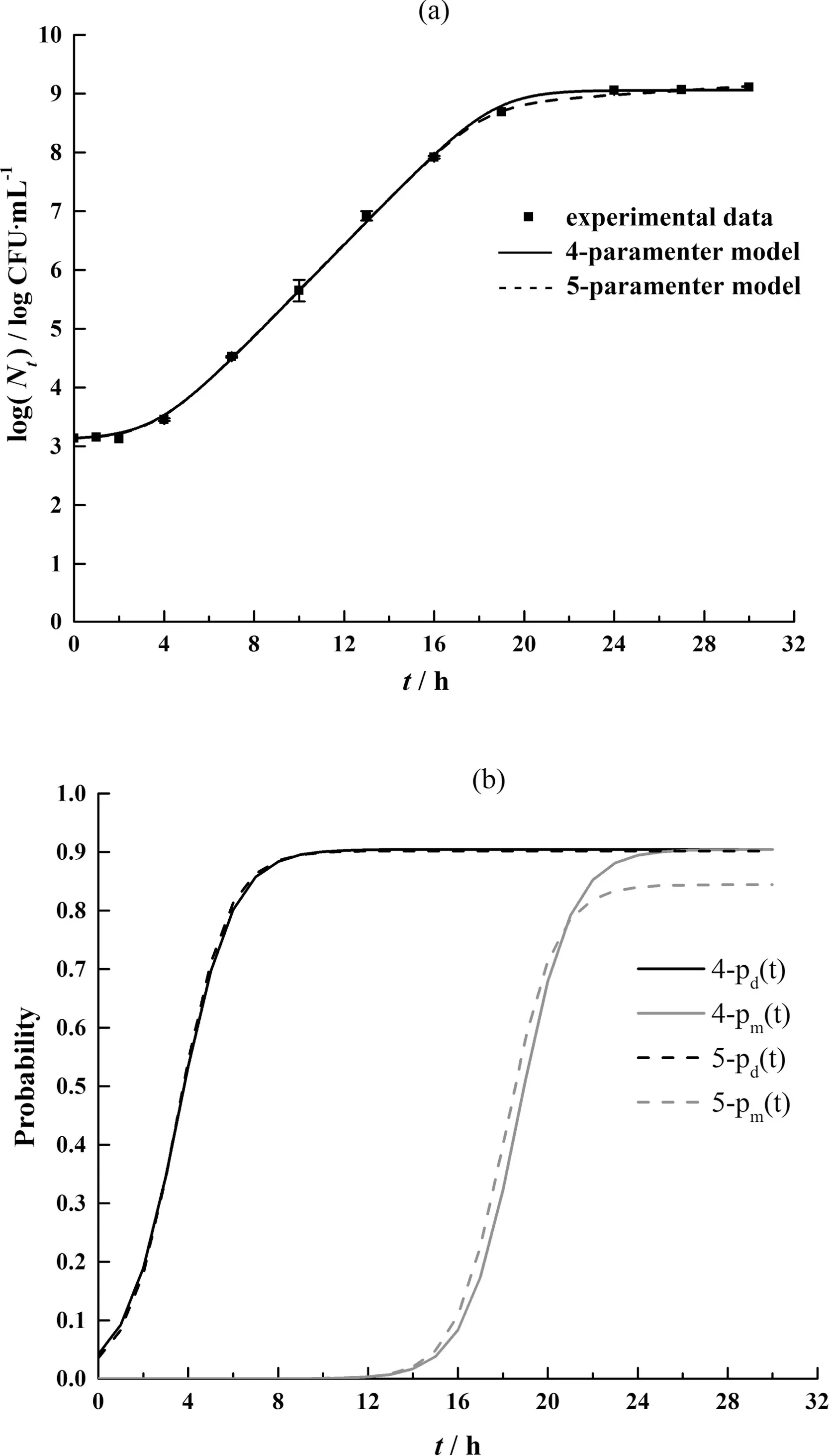
Fig.1.The growth of detached cells fitte by 4-parameter and 5-parameter models under pH7.2+0.5%NaCl stress.(a) S. Enteritidis numbers over time;(b)Pd(t)and Pm(t)over time.

2.10.Statistical analysis

The effects of different environmental conditions on the growth or inactivation curves ofS.Enteritidis were analyzed by Data Processing System (DPS) 7.05 software (Hangzhou Ruifeng information technology Co.Ltd.,Hangzhou,P.R.,China).The differences between kinetic parameters of theS.Enteritidis growth or inactivation curves were determined by analysis of variance(ANOVA)using SPSS 18.0 software(IBM Corporation,New York,USA).Test statistics were regarded as significan difference whenp ＜0.05.

3.Results and discussion

3.1.Establishment of primary probabilistic model for the growth of planktonic and detached S.Enteritidis cells

Both 4-parameter and 5-parameter models [Eqs.(5)-(7)]could describe the growth of planktonic or detached cells well.The growth of detached cells under pH 7.2+0.5%NaCl stress was taken as an example,and the fitte curves were shown in Fig.1.This probabilistic modeling approach could reflec not onlyS.Enteritidis numbers over time,but alsoPd(t)andPm(t)over time.Meanwhile,determination coefficient (R2) of both models were above 0.99,so 4-parameter and 5-parameter models had goodness-of-fifor the growth of detached cells under pH7.2+0.5%NaCl stress.And similar results could be found in the growth ofS.Enteritidis under other environmental conditions.

According to as Ockham’s razor,when two models describe the same pattern,the model with smaller number of adjustable parameters should be chosen [32].This study also indicated that the growth ofS.Enteritidis could be described better by 4-parameter model than by 5-parameter model based on the values ofR2orChi-Sqr.So 4-parameter model was applied to describe the growth of planktonic or detached cells for the following studies.

As shown in Fig.2,the growth curves of planktonic and detached cells were rather similar under most of experimental conditions (p≥0.05,detailed analytic results were not shown).Furthermore,the curves showed significan differences(p ＜0.05,detailed analytic results were not shown)when planktonic and detached cells were under the four environments as follows:pH 5.0+0.0% NaCl,pH 7.0+4.0% NaCl,pH 6.0+4.0% NaCl and pH 5.0+4.0% NaCl,respectively.This might be due to the physiological history and the variability of bacterial cells [37,38].Although the planktonic and detached cells existed in the same system during pre-incubation,the planktonic cells had been growing in the liquid environment,and the detached cells had been adsorbed on the surface of stainless.It should be worth noting that detached cells derived from attached cells had higher tolerance to the harsher environment compared with the planktonic cells[39,40].In addition,the variability of bacterial cells themselves could also affect their growth.Koutsoumanis [38]indicated that variability of bacterial cells would be greater when the growth conditions became harsher.And the above four environment conditions belonged to low-acid or hypersaline environment which were not applicable for the bacterial growth.Consequently,the physiological history and the variability of planktonic and detached cells themselves madebacteriabehavedifferentgrowthcharacteristicsunderlowacid or hypersaline environment.

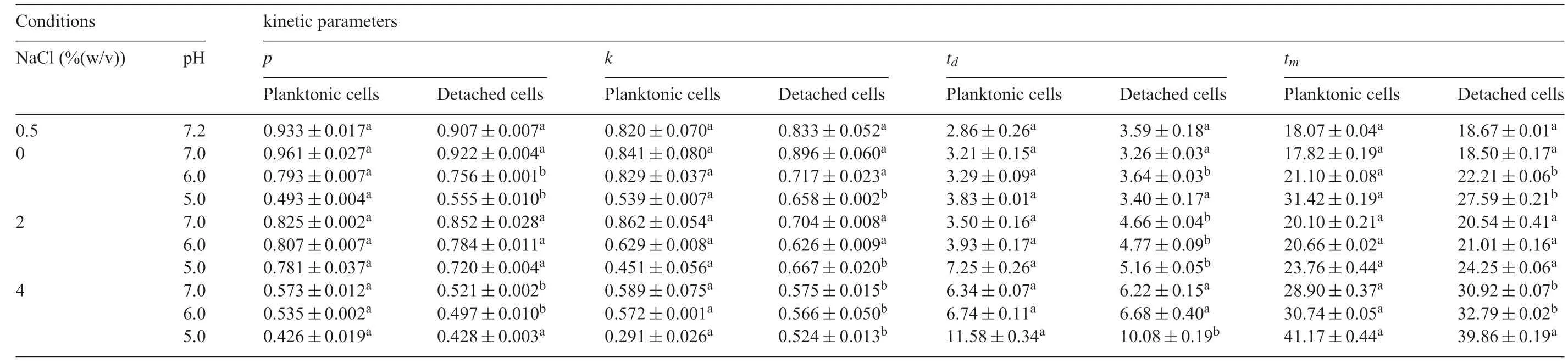
Table1 The kinetic parameters obtained for planktonic and detached cells growth fitte by 4-parameter model under different acid and osmotic pressure.

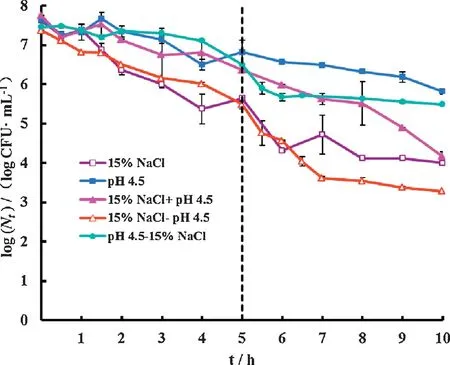
Fig.3.Survival curves of S.Enteritidis under acid and osmotic pressure applied separately,sequentially or simultaneously.

As shown in Table1,the kinetic parameters of planktonic and detached cells also showed significan differences(p ＜0.05)under the four low-acid or hypersaline environmental conditions as follows:pH 5.0+0.0% NaCl,pH 7.0+4.0% NaCl,pH 6.0+4.0% NaCl and pH 5.0+4.0% NaCl,respectively.Meanwhile,the parameterspandkdecreased gradually,tdandtmincreased gradually with the decrease of pH values or the increase of NaCl contents under most of environmental conditions.

3.2.Establishment and estimation of the secondary model for S.Enteritidis growth

Table2 summarized the parameters and mathematic evaluation indexes of secondary model.All of theRMSEwere low,and determination coefficient (R2) of the secondary models were above 0.70 (Table2).Since the range of environment under whichSalmonellaspp.could grow was wide with pH 4.0-9.0[41]or 0-12% NaCl (aw0.919-1.000) [26],respectively,the estimated values ofawminandpHminwere within or near the acceptable range.So the above results showed that the modifie root square model had good applicability.It should be worth noting thatAfandBfof secondary model shown in Table2 were also within the acceptable range according to the opinion of Ross et al.[42].To sum up,the effect of acid and osmotic pressure onp,k,1/td,and 1/tmfor planktonic and detached cells could be described well by the modifie square root model.This work might be a meaningful trial to establish the secondary model reflectin the influenc of environment factors on the fitte parameters of probabilistic model at individual cell level.

3.3.Inactivation of S.Enteritidis under different processing sequences of acid and osmotic pressure

Statistical analysis indicated that fieS.enteritissurvival curves had significan differences(p ＜0.05)under corresponding processing sequences according to Fig.3.The effects of 15%NaCl-pH4.5 stress and 15%NaCl stress onS.Enteritidis inactivation were significantl greater (p ＜0.05) than those of the other three conditions.Furthermore,the numbers ofS.Enter-itidis under 15%NaCl-pH4.5 stress decreased more rapidly than those under the 15%NaCl stress in 10 h at 25°C.Therefore,15%NaCl-pH4.5 stress was the optimal condition to inactivateS.Enteritidis in 10 h at 25°C.

Table2 The coefficient and mathematic evaluation indexes of the secondary model for planktonic and detached cells growth.

The optimal processing sequence of acid and osmotic pressure to inactivateS.Enteritidis in this study was different with that in previous researches [11-13],which indicated that the sequence oflow acid applied firstl and subsequent high osmotic pressure had the best effect to inactivateEscherichia coli,L.monocytogenes,orS.Typhimurium.The discrepancy might be due to the different experimental conditions,the different bacterial genera and species,and the different interaction effects of environmental factors[12].

3.4.Unification of predictive model for S.Enteritidis

The survive curves ofS.Enteritidis under 15%NaCl+pH4.5 stress and pH 4.5 stress had fla shoulders,and they could be fitte by 3-parameter and 5-parameter model,thus realize the unificatio of predictive model to some extent.Taking 15% NaCl+pH 4.5 stress as an example shown in Fig.4,the probabilistic modeling approach could estimateS.Enteritidis numbers,Pd(t)andPm(t)over time.Meanwhile,the 3-parameter model could describe the inactivation ofS.Enteritidis better than 5-parameter model based onR2orChi-Sqrvalues in this study.

3.5.Generalization ofinactivation model for S.Enteritidis

The survival curves ofS.Enteritidis at the two stages of pH4.5-15%NaCl stress had two typical Weibullian shapes,and they were taken as an example to show the applicability of the generalized inactivation model shown in Fig.5.Table3 summarized the kinetic parameters ofS.Enteritidis inactivation fitte by the model with Weibullian form.As shown in Table3,the kinetic parameters showed significan differences(p ＜0.05)under fie processing conditions according to One-Way ANOVA.

3.6.Comparison of the probabilistic and traditional model

Actually,both of the probabilistic model and traditional models (Baranyi model and exponential inactivation model) had goodness-of-fito describe the growth and inactivation ofS.Enteritidis.Nevertheless,apart from demonstrating predictive ability and internal consistency of models,the usefulness of a model should also be judged by its mathematical simplicity,flxibility and the number ofits adjustable parameters.

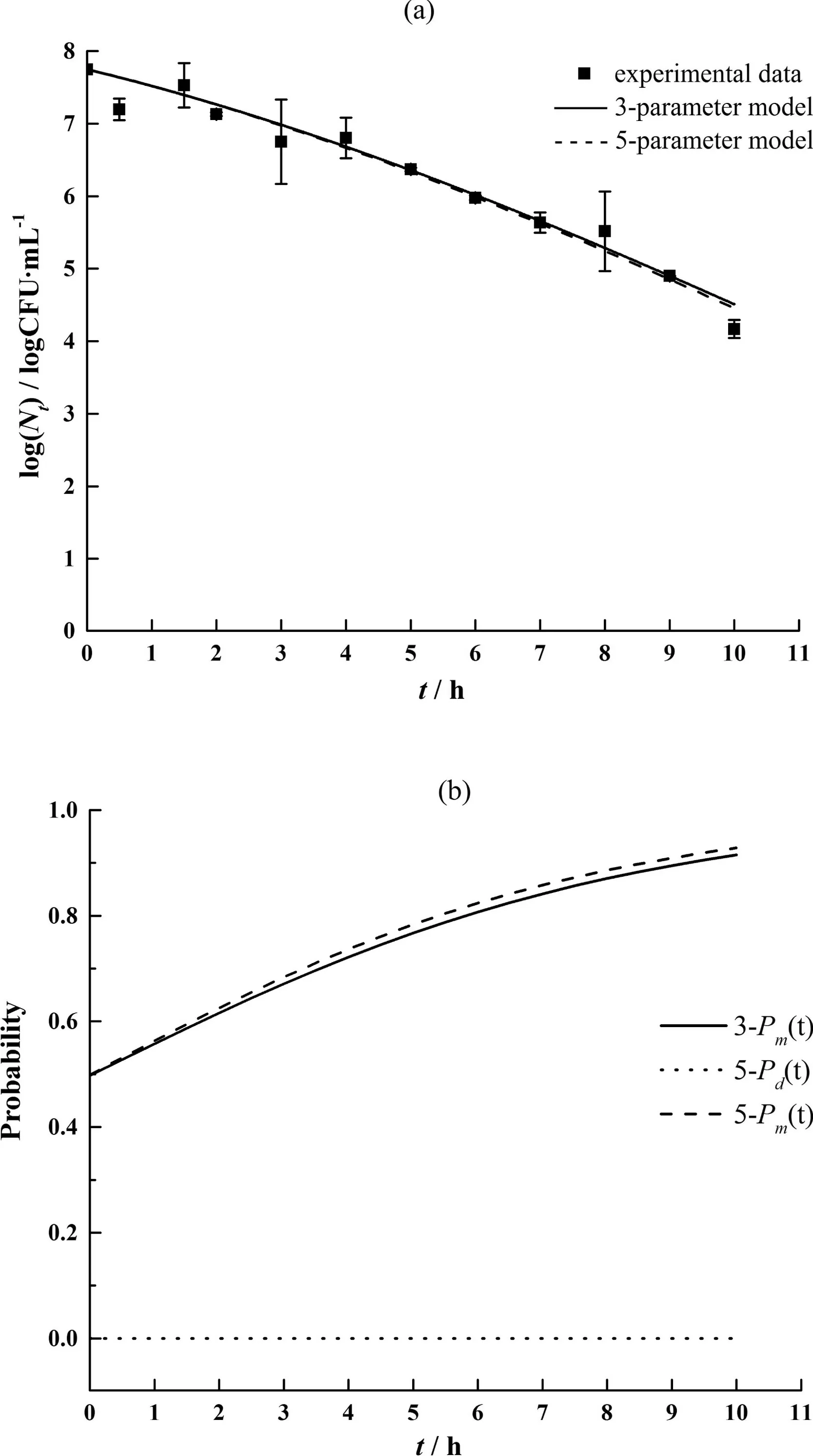
Fig.4.The inactivation of S. Enteritidis under 15%NaCl+pH4.5 stress fitte by 3-parameter and 5-parameter models.(a)S.Enteritidis numbers over time;(b)Pd(t)and Pm(t)over time.

As shown in Table4,determination coefficient (R2) of 4-parameter probabilistic model(Eqs.(5)-(7))and Baranyi model for planktonic and detached cells growth were higher than 0.9.Under most of the environment conditions,R2of 4-parameter probabilistic model was rather higher,andRMSEof 4-parameter probabilistic model was lower than that of Baranyi model for both planktonic and detached cells.Meanwhile,all values ofAfandBfwere within the acceptable range ofAf(1.0-1.3)andBf(0.90-1.05)[42].According to the evaluation method ofAfas described by Ross et al.[42],Afvalues of 4-parameter probabilistic model were smaller than those of Baranyi model for both planktonic cells and detached cells under most of the environment conditions.When it came to the evaluation ofBfreflectin the deviation degree of models,the 4-parameter probabilistic model had smaller deviation than Baranyi model for planktonic cells,but had bigger deviation than Baranyi model for detached cells under most of the environment conditions.In addition,both of 4-parameter probabilistic model and Baranyi model[14]have four adjustable parameters,but 4-parameter probabilistic model has simpler form than that of Baranyi model.Consequently,the 4-parameter probabilistic model had slightly higher applicability and reliability compared with Baranyi model to describeS.enteritisgrowth under most of environmental conditions in this study.

Similarly,according to the above evaluation method,the probabilistic model also had slightly higher applicability and reliability to describe the inactivation ofS.Enteritidis thanexponential inactivation model under most of environmental conditions in this study(Table5).

Table3 The kinetic parameters obtained for the inactivation of S.Enteritidis fitte by the model with Weibullian form.

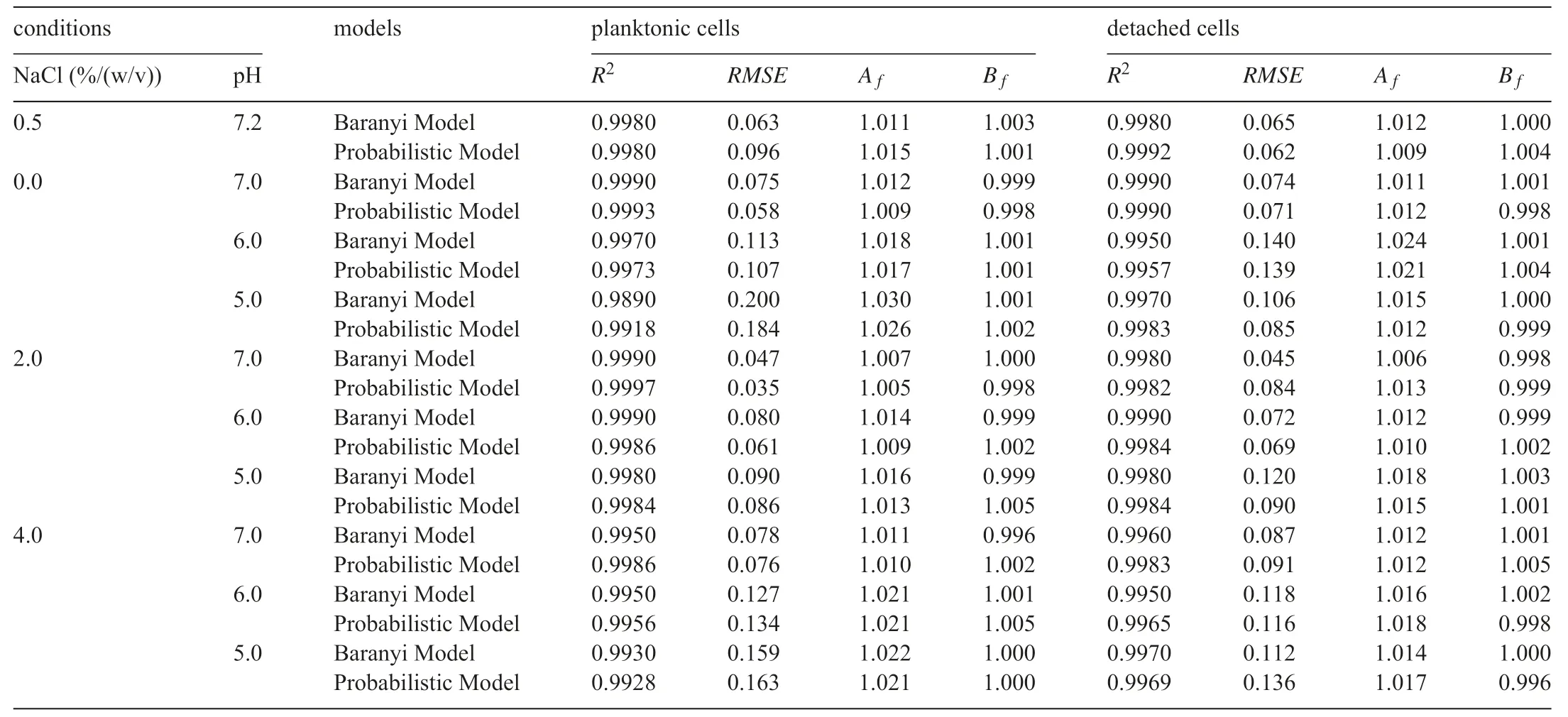
Table4 The mathematic evaluation indexes of Baranyi and 4-parameter probabilistic models for the planktonic and detached cells growth under acid and osmotic pressure.

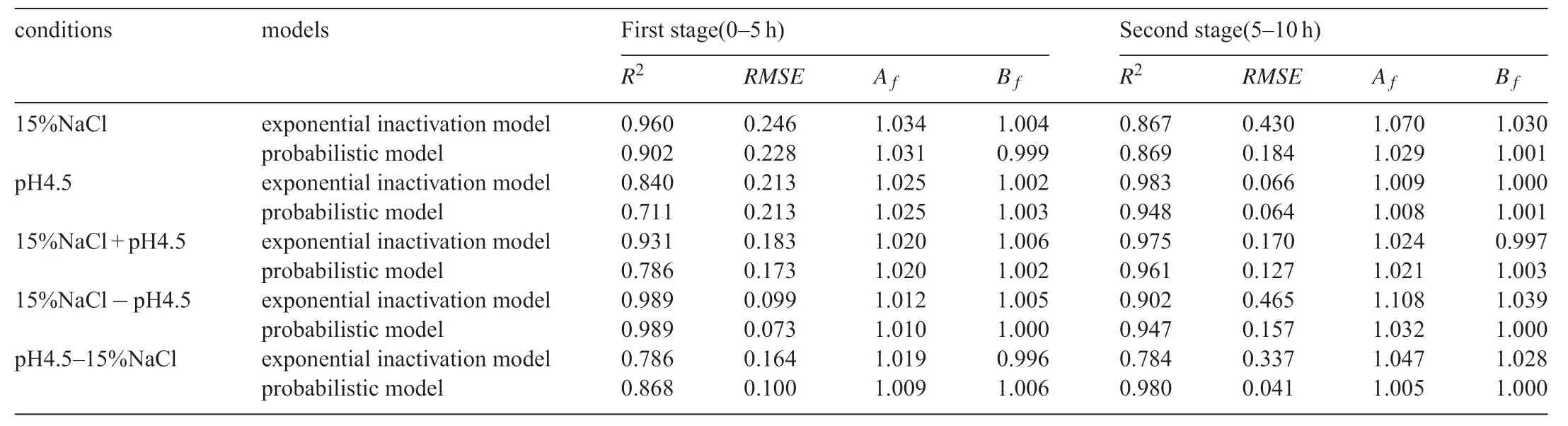
Table5 The mathematic evaluation indexes of exponential inactivation model and generalized inactivation probabilistic model with Weibullian form for S. Enteritidis inactivation under acid and osmotic pressure.

The established models in this study were not validated under a real food background.Because the determination coefficien(R2) was high,root mean square error (RMSE) was low,and the values ofpHmin,awmin,AfandBfabout the models were within acceptable range.Therefore,the growth and inactivation ofS.enteritiscould be predicted well by the probabilistic model and the modifie square root model under the experimental conditions.In addition,this study mainly focused on the methods of modeling,and the validation of the established model will be conducted in the following study.

Fig.5.The inactivation of S.Enteritidis under pH4.5-15%NaCl stress fitte by the model with Weibullian form.(a)S.Enteritidis numbers over time;(b)Pm(t)over time.

4.Conclusion

Acid and osmotic pressure could affect the growth ofS.Enteritidis.In detail,the growth curves of planktonic and detached cells were similar (p≥0.05) under most of experimental conditions.However,their curves showed significan differences (p ＜0.05) under the four environments,including pH 5.0+0.0%NaCl,pH 7.0+4.0%NaCl,pH 6.0+4.0%NaCl and pH 5.0+4.0%NaCl,so did the growth kinetic parameters.In addition,not only the determination coefficient (R2)of the primary probabilistic models and secondary models were high andRMSEwere low,but also the values of awmin,pHmin,AfandBfabout the secondary models were within the acceptable range under the experimental conditions.

The influenc of the different processing sequences of acid and osmotic pressure on theS.Enteritidis inactivation was different.The sequence of 15% NaCl stress followed by pH 4.5 stress was the best condition to inactivateS.Enteritidis in 10 h at 25°C by the significan analysis.And the probabilistic model with logistical or Weibullian form could realize the unificatio to some extent or generalization of predictive model.

The results finall proved that the primary 4-parameter probabilistic model or generalized inactivation model had slightly higher applicability and reliability than Baranyi model or exponential inactivation model to describeS.Enteritidis growth or inactivation within the experimental range in this study.This workprovidedanalternativemethodtodeveloppredictivemodel forSalmonellaspp.during the food processing,and a theoretical reference to improve accuracy of quantitative microbial risk assessment.

Acknowledgments

This work has been financiall supported by the National Natural Science Foundation of China (NSFC 31271896 and 31371776),the project in the National Science & Technology Pillar Program during the Twelfth Five-year Plan Period(2015BAK36B04),and the project of Science and Technology Commission of Shanghai Municipality(15395810900).