On transition of type V interaction in double-wedge flow with non-equilibrium effects

Wentao Xiong,Yujian Zhu,Xisheng Luo

Advanced Propulsion Laboratory,Department of Modern Mechanics,University of Science and Technology of China,Hefei 230026,China

On transition of type V interaction in double-wedge flow with non-equilibrium effects

Wentao Xiong,Yujian Zhu,Xisheng Luo∗

Advanced Propulsion Laboratory,Department of Modern Mechanics,University of Science and Technology of China,Hefei 230026,China

H I G H L I G H T S

·Transition of regular reflection(RR)-Mach reflection(MR)in type V shock interaction in double-wedge flow is studied considering the thermochemical non-equilibrium effects.

·Transition mechanism between RR and MR of type V interaction is changed by the non-equilibrium effects.

·Non-equilibrium effects lead to a lager critical wedge angle and a larger hysteresis interval.

A R T I C L E I N F O

Article history:

Received 31 May 2016

Received in revised form

18 August 2016

Accepted 19 August 2016

Available online 1 October 2016

Shock interaction

Non-equilibrium effects

Shock polar

Hypersonic flow

The transition between regular reflection(RR)and Mach reflection(MR)of type V shock–shock interaction on a double-wedge geometry with high temperature non-equilibrium effects is investigated by extended shock-polar method and numerical simulation.First,the critical angles of transition from detachment criterion and von Neumann criterion are determined by the extended shock-polar method considering the non-equilibrium effects.Then wave patterns and the transition process are numerically obtained.Results of the critical transition angles from shock-polar calculation and numerical simulation show evident disagreement,indicating transition mechanism between RR and MR of type V interaction is changed.By comparing with the frozen counterpart,it is also found that non-equilibrium effects lead to a larger critical wedge angle and a larger hysteresis interval.

©2016 The Authors.Published by Elsevier Ltd on behalf of The Chinese Society of Theoretical and Applied Mechanics.This is an open access article under the CC BY-NC-ND license(http:// creativecommons.org/licenses/by-nc-nd/4.0/).

Shock–shock interaction is a common phenomenon in many aerodynamic configurations.Such shock interactions cause high localized heat loads and pressure oscillations on the vehicle surface,and subsequently have significant impacts on the performance and reliability of hypersonic flights.Type V shock interaction in hypersonic double-wedge flow has gained a lot of attention for it may cause high peak pressure loads and high frequency pressure variations on the wedge surface.However,most previous researches on type V interaction were based on the calorically perfect gas model and few exceptions dealt with the real gas effectswhich play a significantroleinhypersonic flows.Olejniczak et al.[1]investigated the high enthalpy double-wedge flows using experimental methods as well as numerical simulations,and the reasons causing the difference between the numerical simulation and experimental results were analyzed.Nompelis et al.[2]studied the effect of vibrational non-equilibrium on the heat-transfer rate in hypersonic double-wedge flow.Tchuen et al.[3]indicated the double-wedge flow field was highly sensitive to real gas effects which will significantly change the shock shape,the thickness of the shock layer,and the pressure oscillations.

There are two fundamental wave patterns in type V interaction.Figure 1(a)and(b)shows an overall regular reflection (RR)and an overall Mach refection(MR)of type V interaction,respectively.Here,Sw1 is the impinging shock emanating from the first wedge,Sw2 is the impinging shock emanating from the second wedge.Bs is the bow shock generated by the second wedge.Transmitted shock Sw3 and the shock Sw2 undergo a RR or MR.UTP,MTP,and LTP denote the upper triple point,middle triple point,and lower triple point,respectively. In this letter,we will study the transition between these two reflections in hypersonic double-wedge flow with the first wedge angles of 10°–30°considering the thermochemical nonequilibrium effects.The shock polar analysis with non-equilibrium effects[4]is used to theoretically calculate the critical angles of transition angles determined by detachment criterion and von Neumann criterion.The wave patterns and the process of the transition are investigated through two-dimensional inviscid numerical simulations[4].Two-temperature model and Park’s five specieschemicalkineticsmodel[5]areadoptedtocharacterizethe flow.Thenumericalsolutiontwo-dimensionalvectorizedadaptive solver(VAS2D)[6]based on the finite volume method with an unstructured mesh is adopted for the Euler equation,which has beenwellvalidatedintheshock–bodyinteractionandtheshock–bubble interaction[6,7].The type of shock interaction that occurs in double-wedge flow depends on the relevant non-dimensional parameters.Inthisstudy,werestricttheMachnumberMa=9,mole fractions of the gas species N2:O2=0.79:0.21,temperatureTv=T∞=500 K,pressurep=0.1 atm(1 atm=1.0×105Pa), the lengths of the wedgesL1=L2=0.1 m.The original mesh in the computation is 1.8 mm×1.8 mm,and a maximum adaptation level of 6 is used in all simulations.

Fig.1.Schematic diagram of regular reflection and Mach reflection of type V interaction.

Fig.2.Shock polar diagram for type V interaction.

Fig.3.Critical transition angles resulting from shock polar analysis and numerical simulation.

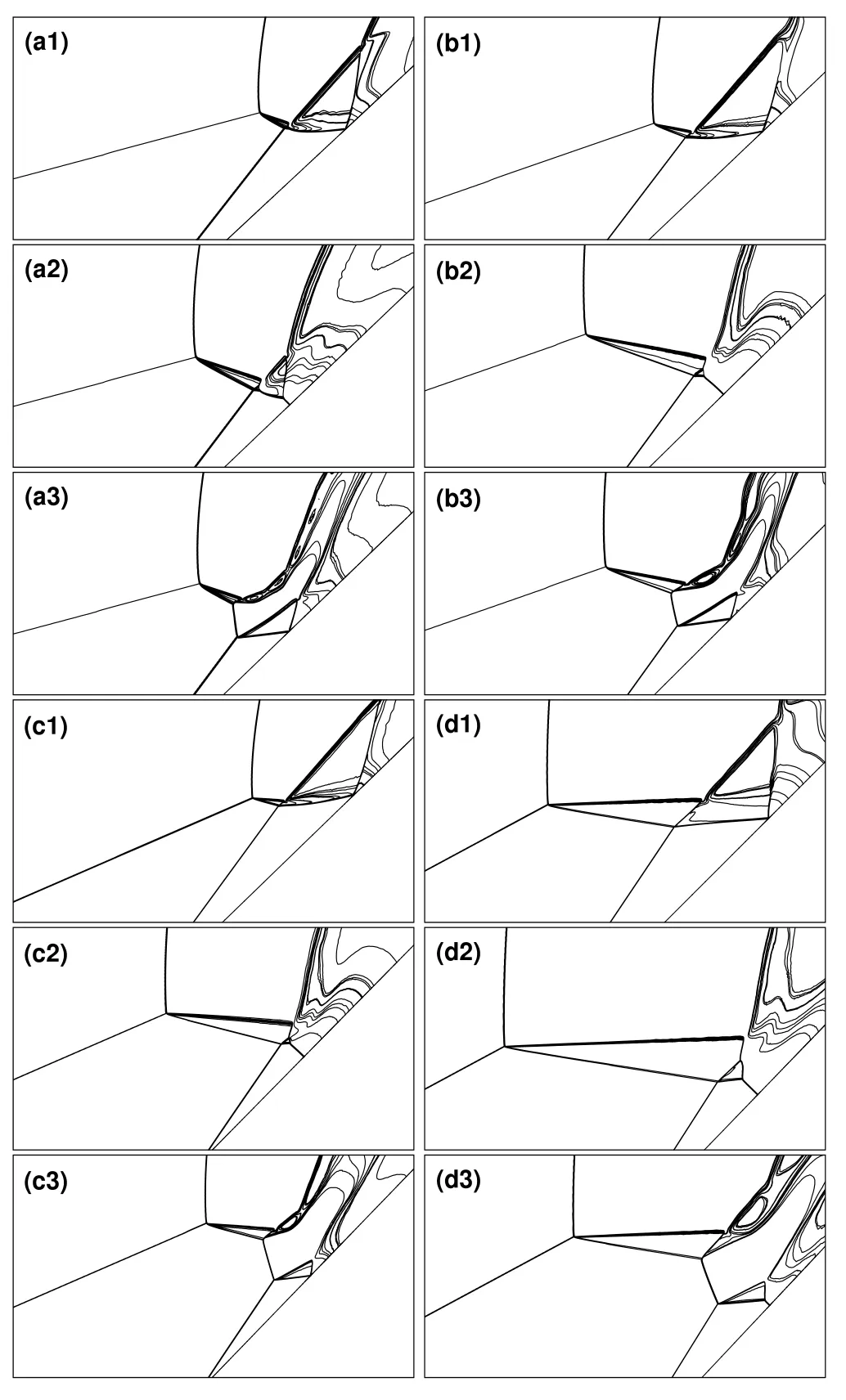
Fig.4.Transition process from RR to MR of type V interaction in non-equilibrium flow.θ1=10°,(a1)θ2=43°,(a2)θ2=43.5°→43.7°,(a3)θ2=43.7°→44°; θ1=14°,(b1)θ2=43.6°,(b2)θ2=44.2°→ 44.5°,(b3)θ2=44.7°→ 44.9°; θ1=18°,(c1)θ2=44.1°,(c2)θ2=45.2°→ 45.5°,(c3)θ2=45.8°→ 46°; θ1=22.5°,(d1)θ2=45°,(d2)θ2=45.5°→46°,(d3)θ2=46.5°→46.8°.

The non-equilibrium shock polar[4]diagrams for type V interaction with different wedge angles are used to analyze the critical angles determined by detachment criterion and von Neumann criterion,as shown in Fig.2.The vertical axis corresponds to pressure behind the oblique shock and is normalized by the incoming flow pressure.Whenθ2is larger thanθD,the transition RR→MR of type V interaction occurs.Whenθ2is smaller thanθvon,the transition MR→RR of type V interaction occurs.The critical angles of the transition RR↔MR obtained by shock polar diagram for nonequilibrium gas are shown in Fig.3,and the critical values from numerical simulations are also illustrated in Fig.3.Here,symbol‘°’denotes the theoretical transition value and symbol‘·’denotes the computed transition value.The solid lineθDis obtained by detachment criterion,while the solid lineθvonis obtained by von Neumann criterion.The dashed line‘RR→MR’indicates the critical angles of transition RR→MR arising from numerical simulation,and the dashed line‘MR→RR’indicates the critical angles of transition MR→RR arising from numerical simulation.Figure 3 also indicates the existence of hysteresis phenomenon between the transition RR↔MR of type V interaction in double-wedge flow with non-equilibrium effects,and there is a hysteresis interval of about 1°in all cases.However,it is found that the hysteresis effect is inconspicuous for frozen cases.At the first wedge angles of 10°and 22.5°,there is a hysteresis interval of about 0.1°.The nonequilibrium effects extend the hysteresis interval for the transition RR↔MR of type V interaction in double-wedge flow.

Fig.5.Transition from RR to MR of type V interaction in frozen flow,θ1=10°, (a1)θ2=40°,(a2)θ2=40°→ 40.2°,(a3)θ2=40.2°→ 40.4°;θ1=22.5°, (b1)θ2=43.4°,(b2)θ2=43.4°→43.6°,(b3)θ2=43.6°→43.8°.

Figure 4 represents the transition process of the RR→MR of type V interaction with series of wedge angles obtained from computations.It is clearly observed in the Mach number contours that the transition of RR→MR is related to the Mach stem generating at the second wedge.With the first wedge angle fixed, when the second wedge angle is small,the transmitted shock Sw3(see in Fig.1)emanating from the upper triple point and the leading wedge shock Sw2 emanating from the leading edge of the secondwedgeundergoanoverallRR,andoneofthereflectedshock Sw5 is re-reflected from the second wedge in an RR,as shown in Fig.4(a1)–(d1).Increasing the second wedge angle gradually,the shockinteractionremainsanoverallRRattheLTP,butthereflected shock Sw5 performs an MR over the second wedge surface,as showninFig.4(a2)–(d2).TheMachstemgrowswiththeincreasing of the angle of the second wedge,and finally the triple point of the MR emanating from second wedge surface collides with the regular intersection point LTP,which leads to the occurrence of the overall MR between the shock wave Sw3 and Sw2,as shown in Fig.4(a3)–(d3).The critical anglesθ2for RR→MR of type V interaction obtained by computations and theoretical shock polar method are compared in the(θ1,θ2)plane in Fig.3.It is found that the critical wedge angles of transition RR→MR by numerical simulations are smaller than the theoretical results based on detachmentcriterion.Thatcanbeascribedtothecollisionbetween the triple points which advances the RR→MR transition.The conclusion considering thermochemical non-equilibrium effects of the flow in our study appears to be consistent with the computations with a perfect gas model by Hu et al.[8].

Frozen computations,with the same initial condition and boundary condition but using a perfect gas model,are conducted to evaluate the influence of the non-equilibrium effects on the critical angles.Two frozen double-wedge flows were computed and the transitions of the shock wave configuration are illustrated in Fig.5.The transition RR→MR of type V interaction undergoes a different process at the first wedge angle of 10°.The transition in non-equilibriumflowisfinishedbyacollisionasmentionedabove, whilethetransitioninfrozenflowdoesnotundergosuchaprocess. The mechanism for the transition RR→MR of type V interaction at the first wedge angle of 10°in frozen flow has been explained for disturbance-induced transition by Hu et al.[9].In our study, it is found that the non-equilibrium effects change the transition mechanism,i.e.the transition undergoes a collision.Moreover,it is observed that the non-equilibrium effects make the critical wedge angles larger than the frozen ones.

Fig.6.Transition process from MR to RR of type V interaction in non-equilibrium flow,θ1=10°,(a1)θ2=43.7°,(a2)θ2=43.5°→43.3°,(a3)θ2=43.3°→43°; θ1=14°,(b1)θ2=44.5°,(b2)θ2=44.5°→ 44°,(b3)θ2=44°→ 43.8°; θ1=18°,(c1)θ2=46°,(c2)θ2=46°→ 45.7°,(c3)θ2=45.7°→ 45.3°; θ1=22.5°,(d1)θ2=47°,(d2)θ2=47°→46.8°,(d3)θ2=46.8°→46.5°.

The transition process of the MR→RR of type V interaction with series of wedge angles obtained from numerical simulations is shown in Fig.6.It is seen by Mach number contour that the transition process of MR→RR of type V interaction obeys the same principle for different wedge angles.With the first wedge angle θ1fixed,the computation starts at an angle ofθ2which leads to an overall MR of type V interaction.With theθ2decreasing gradually,the Mach stem emanating from the MTP and LTP(see in Fig.1(b))becomes shorter.With further decreasing theθ2to critical value,the Mach stem disappears and the shock wave configuration changes to an overall RR of type V interaction.The criticalvaluesofθ2obtainedfromtheoreticalshockpolarapproach and numerical results are compared in the(θ1,θ2)plane in Fig.3.It is found that there is a great difference between the critical values of transition MR→RR obtained from von Neumann criterion and numerical simulation.That means the mechanism of the transition MR→RR is not the von Neumann criterion any more.With the second wedge angleθ2decreasing,MTP moves down until it collides with LTP,which leads to the occurring of MR→RR.Frozen computations are also performed to investigate the influence of the non-equilibrium effects on the transition MR→RR of type V interaction.As illustrated in Fig.7,the frozen transitions areconsistent with the ones in non-equilibrium flows.However,the critical values ofθ2in frozen flows are smaller than those in the non-equilibrium case.

Fig.7.Transition from MR to RR of type V interaction in frozen flow,θ1=10°, (a1)θ2=41°,(a2)θ2=41°→ 40.6°,(a3)θ2=40.6°→ 40.3°;θ1=22.5°, (b1)θ2=44.5°,(b2)θ2=44.5°→44°,(b3)θ2=44°→43.7°.

In conclusion,the transition between RR and MR of type V interaction in hypersonic double-wedge flow was investigated by considering the non-equilibrium effects.The results showed that there was a significant difference between the critical wedge anglesofthesecondwedgeobtainedbytheoreticalmethodandthe numerical simulation,indicating transition mechanism between RR and MR of type V interaction was changed.The collisions between the shock interaction points of type V interaction caused the transition.Hysteresis phenomenon was also observed.By comparing with the frozen counterpart,it was also found that nonequilibrium effects lead to a larger critical wedge angle and a larger hysteresis interval.

[1]J.Olejniczak,G.Candler,M.Wright,et al.,Experimental and computational study of high enthalpy double-wedge flows,J.Thermophys.Heat Transfer 13 (1999)431–440.

[2]I.Nompelis,G.Candler,M.Holden,Effect of vibrational nonequilibrium on hypersonic double-cone experiments,AIAA J.41(2003)2162–2169.

[3]G.Tchuen,Y.Burtschell,D.Zeitoun,Numerical study of the interaction of type IVr around a double-wedge in hypersonic flow,Comput.Fluids 50(2011) 147–154.

[4]J.Li,Y.Zhu,X.Luo,On type VI–V transition in hypersonic double-wedge flows with thermo-chemical non-equilibrium effects,Phys.Fluids 26(2014)086104.

[5]C.Park,Review of chemical-kinetic problems of future NASA missions.I-Earth entries,J.Thermophys.Heat Transfer 7(1993)385–398.

[6]M. Sun, K. Takayama, Conservative smoothing on an adaptive quadrilateral grid,J. Comput. Phys. 150 (1999) 143–180.

[7]Z.Zhai,M.Wang,T.Si,et al.,On the interaction of a planar shock with a light polygonal interface,J.Fluid Mech.757(2014)800–816.

[8]Z.Hu,R.Myong,M.Kim,et al.,Downstream flow condition effects on the RR→MR transition of asymmetric shock waves in steady flows,J.Fluid Mech. 620(2009)43–62.

[9]Z.Hu,Y.Gao,R.Myong,et al.,Geometric criterion for RR↔MR transition in hypersonic double-wedge flows,Phys.Fluids 22(2010)016101.

∗Corresponding author.

E-mail address:xluo@ustc.edu.cn(X.Luo).

http://dx.doi.org/10.1016/j.taml.2016.08.011

2095-0349/©2016 The Authors.Published by Elsevier Ltd on behalf of The Chinese Society of Theoretical and Applied Mechanics.This is an open access article under the CC BY-NC-ND license(http://creativecommons.org/licenses/by-nc-nd/4.0/).

*This article belongs to the Fluid Mechanics