Coriolis effect on responses of rotating thin piezoelectric hollow cylinder
Wenyao Liu∗,Weiqiu Chen
aHaitian School,Ningbo Polytechnic,Ningbo 315800,China
bDepartment of Engineering Mechanics,Zhejiang University,Hangzhou 310027,China
Coriolis effect on responses of rotating thin piezoelectric hollow cylinder
Wenyao Liua,∗,Weiqiu Chenb
aHaitian School,Ningbo Polytechnic,Ningbo 315800,China
bDepartment of Engineering Mechanics,Zhejiang University,Hangzhou 310027,China
H I G H L I G H T S
·Coriolis effect is considered for the first time in the analysis of a rotating piezoelectric hollow cylinder.
·A different strategy is employed to derive the equation governing the radial displacement,which is then solved approximately but analytically when the shell is thin enough.
·Numerical examples show that the Coriolis effect can be significant under certain conditions in active control of the shell.
A R T I C L E I N F O
Article history:
Received 22 September 2016
Accepted 7 October 2016
Available online 4 November 2016
Coriolis effect
Rotating cylinder
Piezoelectric material
Analytical solution
Coriolis effect is considered in the analysis of a rotating piezoelectric hollow cylinder.An inhomogeneous Bessel equation governing the radial mechanical displacement is derived,which can be approximated as an Euler type differential equation when the cylinder is very thin.Numerical examples show that the Coriolis effect can be significant under certain conditions.
©2016 The Author(s).Published by Elsevier Ltd on behalf of The Chinese Society of Theoretical and Applied Mechanics.This is an open access article under the CC BY-NC-ND license(http:// creativecommons.org/licenses/by-nc-nd/4.0/).
Rotating structures have long been of significant research interest because of their practical importance in various engineering applications[1–4].With the emergence of smart structures[5,6], efforts have also been made on the study of rotating piezoelectric plates and shells[7,8].In a relatively recent paper,Galic and Horgan[9](denoted as GH hereafter for brevity)derived a closed form exact solution for a rotating solid/hollow cylinder and some interesting issues were addressed and highlighted.
In this letter,we further take account of the Coriolis effect in the investigationon theresponse ofa rotatingpiezoelectrichollow cylinder.A relatively simple derivation of the equation governing the radial displacement is presented,which leads to a Bessel equation with inhomogeneous term.For the sake of simplicity, only very thin cylindrical shells are considered in this letter.Thus, the Bessel equation can be treated approximately as an Euler type differential equation,of which the solution is well known. Numerical calculation is finally performed and some interesting observations are discussed.
The basic equations of an infinite radially polarized piezoelectric cylinder for the axisymmetric problem can be easily found.When the cylinder is rotating about its axis at constant angular velocityω,the equation of motion with Coriolis effect is[10,11]

whereσrrandσθθare respectively the radial and circumferential normal stress components,uris the radial displacement,ρdis the material density,andris the radial coordinate in the cylindrical coordinate system(r,θ,z)with its origin located on the axis.


whereAis an arbitrary constant.Second,from the constitutive relations

whereφis the electric potential,cij,eij,andεijare respectively the elastic,piezoelectric,and dielectric constants,it is obtained that

where

Substituting Eq.(4)into Eq.(1),yields
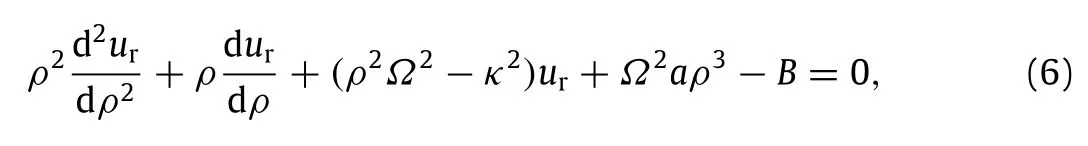
whereρ =r/aandΩ = ρdω2a2/c33are the dimensionless radial coordinate and angular velocity,respectively,withabeing the inner radius of the hollow cylinder,and

Equation(6)is an inhomogeneous Bessel equation whose solution consists of two parts.The general solution part is a linear combination of the well known Bessel functions of the first and second kinds,while the particular part is represented by the so-called Lommel functions[12,13].The solution is a little complicated,and from Eq.(4),the expression for the electric potentialφwillfurthercontainintegralsinvolvingBesselfunctions and Lommel functions.In this letter,however,we will just pay our attention to the effect of Coriolis acceleration on very thin hollow cylinders,for which Eq.(6)can be approximated as
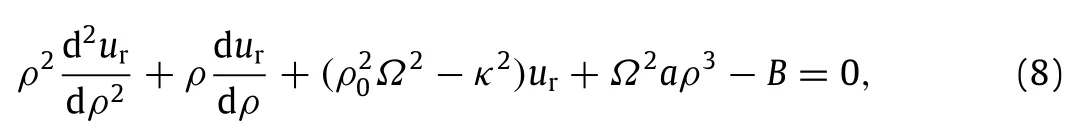
whereρ0=R/a,andR= (a+b)/2 is the mean-radius of the cylinder,withbbeing the outer radius of the hollow cylinder.Note that such an approximation technique has been widely used and validated in many branches of applied mechanics [10,14–16].Obviously,when the thickness of the hollow cylinder decreases,thesolutionbasedonEq.(8)willbecomemoreandmore accurate.Equation(8)is an Euler equation,whose solution is very straightforwardandomittedhereforbrevity.Actually,thesolution contains three arbitrary constants:Two are the integral constants directly related to the general solution of Eq.(8),while the another one isA,appearing in the particular solution through the constantB.The three unknown constants can be determined from the boundary conditions at the inner and outer surfaces of the cylinder (one mechanical at each surface and one electric).It is noted that, when the electric potential is specified at the surfaces,one can only determine the electric difference between the two surfaces, since the constant electric potential plays a role like the rigid body displacement.On the other hand,when electric displacement is specified,only the condition at one surface should be considered, and the one at another surface becomes a natural result because of the requirement of self-equilibrium of electric displacement.
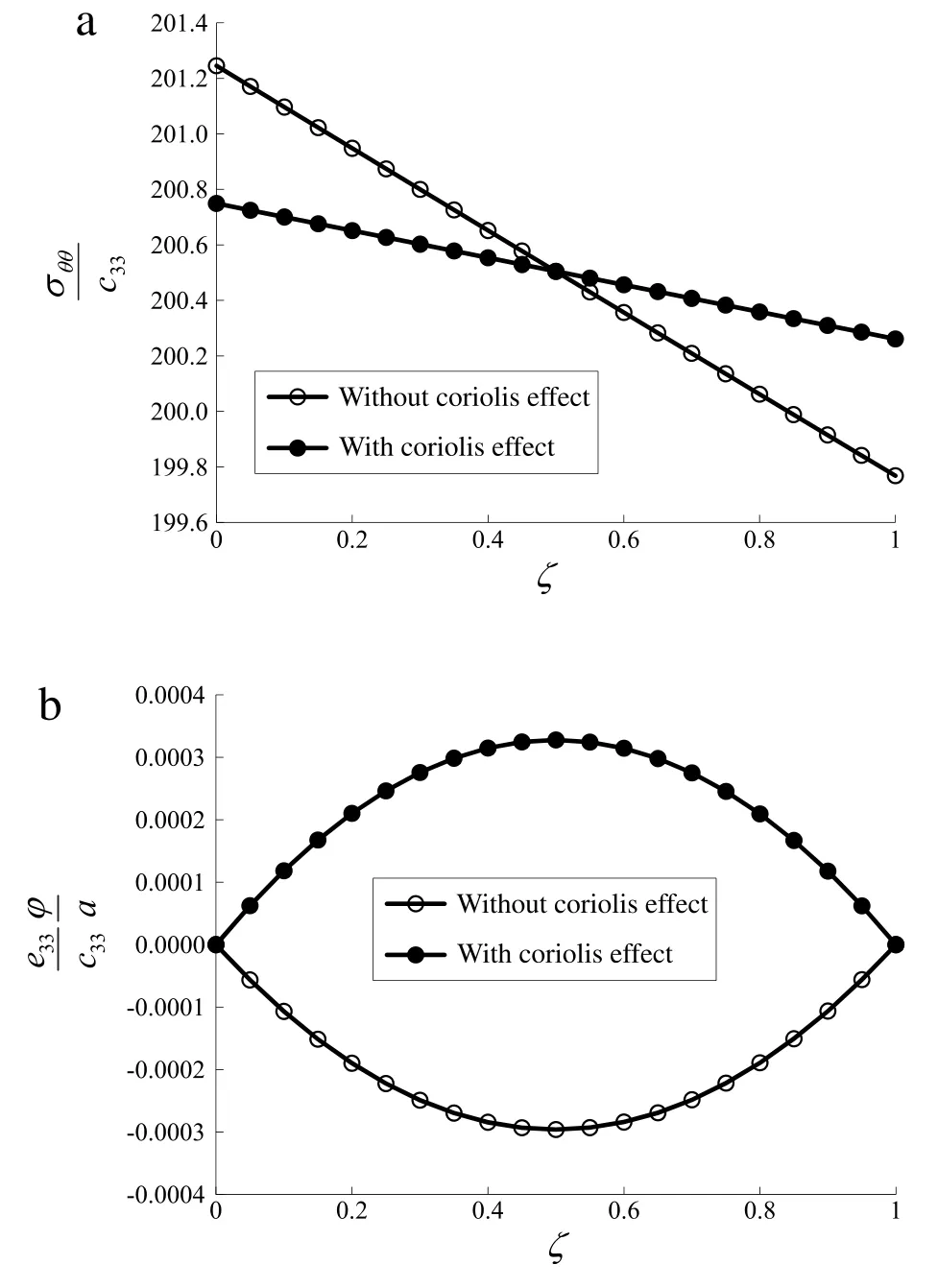
Fig.1.Responses of the rotating cylinder in Case A(Ω=1).
It is obvious that when the Coriolis effect is neglected,Eq.(8) is exact without any approximation.This equation is simpler than that of the fourth-order one as mentioned in GH.Although our solution contains only three arbitrary constants,and that of GH contains four arbitrary constants,the two solutions are exactly the same because the additional constant in GH is related to the constant electric potential only,which contributes nothing to the electroelastic field in the cylinder.
Fornumericalillustration,weassumethattherearenoexternal mechanical forces acting on the inner and outer surfaces of the cylinder.In addition,we consider two types of electric boundary conditions:Case A is just Case 1 in GH(i.e.closed-circuit on both theinnerandoutersurfaces),whileinCaseB,theelectriccondition in Case A is replaced with zero electric displacement at either surface.From the previous studies[14,16],we can conclude that when the thickness-to-mean radius ratio of the cylinderh/R= 1/200,the error due to the approximation introduced in this note can be neglected,provided that the dimensionless angular velocity Ωis not very large.In fact,we have also performed the calculation according to the exact solution of Eq.(5),and found the two are almost identical.

Fig.2.Responses of the rotating cylinder in Case A(Ω=3).

Fig.3.Electric potential distributions in the rotating cylinder in Case B.
Results are presented in Figs.1–3 for a lead zirconate titanate (PZT-4)hollow cylinder withh/R=1/200,where two values of Ωare considered,i.e.,Ω =1 andΩ =3.A new dimensionless radial coordinateζ=(r-a)/(b-a)is used such that the inner and outer surfaces correspond toζ=0 andζ=1,respectively. The material constants of PZT-4 can be found in Ref.[17]asc33= 1.15×1011Pa,c11=1.39×1011Pa,c13=7.43×1010Pa,e31=-5.2 C/m2,e33=15.1 C/m2,andε33=5.62×10-9F/m. For both cases,we assume that the electric potential at the inner surface is zero.The distribution ofσrris not given in Figs.1 and 2 because the Coriolis effect on it is completely negligible.Although the Coriolis effect onσθθis also little,it does lead to a more uniform distribution along the thickness direction,as shown in Figs.1(a)and 2(a),which is favorable for the practical structure. The significance of Coriolis acceleration effect is clearly shown in Figs.1(b)and2(b)whenconsideringtheelectricpotential.InFig.3, we give the distributions of electric potential for two values ofΩ. The phenomena shown in Fig.3(a)and(b)are both of practical importance because the electric potential difference is generally used as a primary parameter in the feedback control of smart structures.Figure 3(a)indicates that the practical control may be overfullwhentheCorioliseffectisnotconsideredinthetheoretical prediction.Furthermore,Fig.3(b)implies the possibility of a completely opposite action that may be induced in practice.
In this letter,we considered the response of a rotating piezoelectric hollow cylinder by taking the Coriolis effect into consideration.A different strategy was adopted to derive the governing equation,which seems to be simpler than that reported in the literature when the Coriolis effect is absent.For cylinders with very small thickness,the equation was simplified and an analytical solution was derived.It was shown numerically that the Coriolis effect may become very important in the active control of rotating structures due to the coupling between the elastic and electric fields.
Acknowledgments
The work was supported by the National Natural Science Foundation of China(11321202)and the Fundamental Research Funds for the Central Universities(2016XZZX001-05).
[1]G.H.Bryan,On the beats in the vibration of a revolving cylinder or bell,Proc. Cambridge Philos.Soc.7(1890)101–111.
[2]Y.Chen,H.B.Zhao,Z.P.Shen,Vibrations of high speed rotating shells with calculations for cylindrical shells,J.Sound Vib.160(1993)137–160.
[3]X.M.Zhang,Parametric analysis of frequency of rotating laminated composite cylindrical shells with the wave propagation method,Comput.Methods.Appl. Mech.Engrg.191(2002)2029–2043.
[4]Y.X.Chen,P.Q.Liu,Z.H.Tang,et al.,Wind tunnel tests of stratospheric airship counter rotating propellers,Theor.Appl.Mech.Lett.5(2015)58–61.
[5]I.Chopra,Review of state of art of smart structures and integrated systems, AIAA J.40(2002)2145–2187.
[6]A.Abdelkefi,A.Hasanyan,J.Montgomery,et al.,Incident flow effects on the performance of piezoelectric energy harvesters from galloping vibrations, Theor.Appl.Mech.Lett.4(2014)022002.
[7]W.Q.Chen,H.J.Ding,Exact static analysis of a rotating piezoelectric spherical shell,Acta Mech.Sin.14(1998)257–265.
[8]W.Q.Chen,H.J.Ding,J.Liang,The exact elasto-electric field of a rotating piezoceramic spherical shell with a functionally graded property,Int.J.Solids Struct.38(2001)7015–7027.
[9]D.Galic,C.O.Horgan,The stress response of radially polarized rotating piezoelectric cylinders,J.Appl.Mech.70(2003)426–435.
[10]R.A.DiTaranto,M.Lessen,Coriolis acceleration effect on the vibration of a rotating thin-walled circular cylinder,J.Appl.Mech.31(1964)700–701.
[11]C.T.Loy,K.Y.Lam,Vibrations of rotating thin cylindrical panels,Appl.Acoust. 46(1995)327–343.
[12]I.S.Gradshteyn,I.M.Ryzhik,Table of integrals,Series,and Products,seventh ed.,Academic Press,New York,2007.
[13]C.F.Lü,J.S.Yang,J.Wang,et al.,Power transmission through a hollow cylinder by acoustic waves and piezoelectric transducers with radial polarization, J.Sound Vib.325(2009)989–999.
[14]J.Q.Ye,K.P.Soldatos,Three-dimensional stress analysis of orthotropic and cross-plylaminatedhollowcylindersandcylindricalpanels,Comput.Methods Appl.Mech.Engrg.117(1994)331–351.
[15]Y.Tanigawa,Some basic thermoelastic problems for nonhomogeneous structural materials,Appl.Mech.Rev.48(1995)287–300.
[16]J.Zhu,W.Q.Chen,G.R.Ye,et al.,Waves in fluid-filled functionally graded piezoelectric cylinders:A restudy based on the reverberation-ray matrix formulation,Wave Motion 50(2013)415–427.
[17]M.L.Dunn,M.Taya,Electroelastic field concentrations in and around inhomogeneities in piezoelectric solids,J.Appl.Mech.61(1994)474–475.
∗Corresponding author.
E-mail address:1182301832@qq.com(W.Liu).
http://dx.doi.org/10.1016/j.taml.2016.10.001
2095-0349/©2016 The Author(s).Published by Elsevier Ltd on behalf of The Chinese Society of Theoretical and Applied Mechanics.This is an open access article under the CC BY-NC-ND license(http://creativecommons.org/licenses/by-nc-nd/4.0/).
*This article belongs to the Solid Mechanics
 Theoretical & Applied Mechanics Letters2016年6期
Theoretical & Applied Mechanics Letters2016年6期
- Theoretical & Applied Mechanics Letters的其它文章
- Synergy to discovery and innovation—Growth of nanorods
- Buckling and post-buckling analyses of size-dependent piezoelectric nanoplates
- Numerical simulation of domain switching in multilayer ferroelectric actuators
- On the role of piezoelectricity in phonon properties and thermal conductivity of GaN nanofilms
- On transition of type V interaction in double-wedge flow with non-equilibrium effects
- Numerical simulation of Gurney flap on SFYT15thick airfoil
