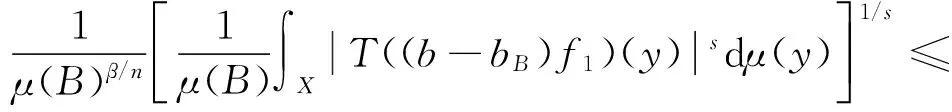Lipschitz函数和非光滑核奇异积分算子的交换子
谢佩珠
(广州大学 数学与信息科学学院, 广东 广州 510006)
Lipschitz函数和非光滑核奇异积分算子的交换子
谢佩珠
(广州大学 数学与信息科学学院, 广东 广州 510006)

交换子;Lipschitz函数; 非光滑核;Triebel空间
0 Introduction
Inthispaper,weassumethat(X,d,μ)isaspaceofhomogeneoustypewithinfinitemeasure,thatisμ(X)=∞.Forallcontinuousfunctionsfwithcompactsupport,thereexistsameasurablefunctionK(x,y)suchthat
Tf(x)=∫XK(x,y)f(y)dμ(y)
holdsforalmostallxnotinthesupportoff,thenwecallK(x,y)beanassociatedkernelofT.InRef.[1],DUONG,etal.havedefinedthesingularintegraloperatorwithnon-smoothkernel.AnoperatorTiscalledsingularintegraloperatorwithnon-smoothkernelifitsatisfiesthefollowing:
(i)Thereexists“generalizedapproximationstotheidentity” {At}t>0,whichsatisfythecondition(6)inSection1,suchthatT-AtThaveassociatedkernelskt(x,y)andwhend(x,y)≥c1t1/m,
(1)
holds for someγ,m>0 and
(ii) There exists “generalized approximations to the identity” {Bt}t>0, which satisfy the condition (6) in Section 1, such that the associated kernelsKt(x,y) ofT-TBtsatisfy
(2)
for ally∈X, wherec2andc3are positive constants.
In Ref.[2], DUONG, et al. have proved that ifTis a singular integral operator with non-smooth kernel and bounded onLq(X) for some 1 (3) for 1 Throughout the paper, the letter “C” will denote (possibly different) constants that are independent of the essential variables. Letμbe a measure onXand letdbe a metric onX. Then we call topological spaceXto be a space of homogeneous type if it satisfies the doubling property, that is, there exists a constantC≥1, such that for all ballsB(x,r)={y∈X:d(y,x) μ(B(x,2r))≤Cμ(B(x,r))<∞. For the definition of homogeneous type space, one can see Ref.[9], Chapter 3. Using the doubling property, we can obtain that there existC,n>0 such that μ(B(x,λr))≤Cλnμ(B(x,r)) (4) holds for allλ>1. The parameternis a measure of the dimension of the space. We can also obtain that there existCandN,0≤N≤nsuch that for allx,y∈Xandr>0 (5) holds. Indeed, using triangle inequality ofdand (4), we can obtain (5) withN=n. It is easy to see that for the Euclidean spacesRn, we can letN=0. Now, we define the Hardy-littlewood maximal functionMrf, 1≤r<∞. That is Ifr=1,we denoteM1fbyMf. “Generalized approximations to the identity” {At}t>0previously appeared in Ref.[1]. We call {At}t>0be “generalized approximations to the identity” if the associated kernelsat(x,y) ofAtsatisfy (μ(B(x,t1/m)))-1s(d(x,y)mt-1) (6) wheremis a positive constant andsis a positive, bounded, decreasing function satisfying (7) for someζ>0, wherenandNare constants in (4) and (5). Using (5) and (7), we have (8) Now we define Triebel spaces associated with “generalized approximations to the identity” {At,t>0}. (9) Wehavethefollowinglemmas. Lemma1[10]For0<β<1, 1≤q<∞,wehave ItiseasytoknowthattheaboveLemmasallhavetheircounterpartinspacesofhomogeneousXwithalmostidenticalproofswheneverμ(X)=∞. Lemma3[1]Foreveryp∈[1,∞),thereexistsaconstantCsuchthatforeveryf∈Lp(X), Atf(x)≤CMf(x). ItisprovedinRef.[1]thatifTisanoperatorboundedonL2(X)andsatisfying(i)and(ii)inSection0,thenTisboundedonLp(X)forall1 ProofofTheorem1 Foranarbitraryfixedx∈X,chooseaballB(x0,r)whichcontainsx.Fixf∈Lp(X),p>1andletf1=f2Bandf2=f-f1.Choosetworealnumbersrandsgreaterthan1suchthat1 [b,T]f=(b-bB)Tf-T((b-bB)f1)-T((b-bB)f2), and AtB[b,T]f=AtB((b-bB)Tf)- AtBT((b-bB)f1)-AtBT((b-bB)f2), I+II+III+IV+V. Letr′ be the dual ofrsuch that 1/r+1/r′=1. Using the Holder inequality and Lemma 2, we have By Lemmas 2, 3 and theLpboundedness ofT, Similarly, by Lemmas 1, 2, 3, and theLpboundedness ofT, we obtain WenowconsiderthetermV.Usingtheassumption(i),wehave We now take the supremum over allBsuch thatx∈B, andLpthe norm of both sides, we conclude that TheTheorem1isproved. [1] DUONG X T, MCINTOSH A. Singular integral operators with non-smooth kernels on irregular domains[J]. Rev Mat Iberoamer, 1999, 15(2): 233-265. [2] DUONG X T, YAN L X. Commutators of BMO functions and singular integral operators with non-smooth kernels[J]. Bull Austral Math Soc, 2003, 67(2): 187-200. [3] JANSON S. Mean oscillation and commutators of singular integrals operators[J]. Ark Mat, 1978, 16(1): 263-270. [4] CHANILLO S. A note on commutators[J]. Indiana Univ Math J, 1982, 31(1): 7-16. [5] BRAMANTI M, CERUTTI M. Commutators of singular integrals on homogeneous spaces[J]. Bull Un Mat Ital, 1996, 10(4): 843-883. [6] CHEN Y P, ZHU K.Lpbounds for the commutators of oscillatory singular integrals with rough kernels[J]. Abstract Appl Anal, 2014, 2014(6):1-8. [7] CHEN Y P, DING Y.Lpbounds for the commutators of singular integrals and maximal singular integrals with rough kernels[J]. Trans Amer Math Soc, 2015, 367(3): 1585-1608. [8] LI P T, MO Y, ZHANG C Y. A compactness criterion and application to the commutators associated with Schrödinger operators[J]. Math Nachr, 2015(2), 288:235-248. [9] COIFMAN R R, WEISS G. Analyse harmonique non-commutative sur certains espaces homognes[M]. Berlin: Springer, 1971. [10]PALUSZYNSKI M. Characterization of the Besov spaces via the commutator operator of Coifman, Rochberg and Weiss[J]. Indiana Univ Math J, 1995, 44(1): 1-18. 【责任编辑: 周 全】 Commutators of Lipschitz functions and singular integral operators with non-smooth kernels XIE Pei-zhu (School of Mathematics and Information Sciences, Guangzhou University, Guangzhou 510006, China) O174Documentcode:A Foundation items: Supported by NNSF of China (11401120); Foundation for Distinguished Young Teachers in Higher Education of Guangdong Province (YQ2015126); Foundation for Young Innovative Talents in Higher Education of Guangdong (2014KQNCX111); Innovation Program of Higher Education of Guangdong(2015KTSCX105). 1671- 4229(2016)05-0027-04 O A Received date: 2016-04-26; Revised date: 2016-05-06 Biography: XIE Pei-zhu(1982-), female, lecturer. E-mail: xiepeizhu82@163.com Keywords:commutators;Lipschitzfunctions;non-smoothkernels;Triebelspaces

1 Definitions and preliminary results

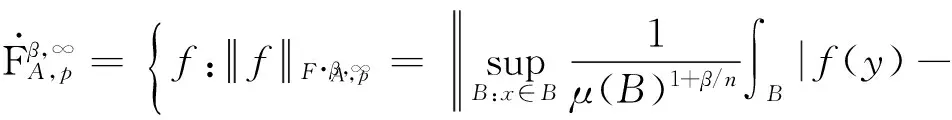




2 The proof of the main results