谱Galerkin方法的超几何收敛

陶霞

（湖南理工学院 数学学院，湖南 岳阳 414006）

谱Galerkin方法的超几何收敛

陶霞

（湖南理工学院 数学学院，湖南 岳阳 414006）

介绍了求解第一类Volterra积分方程的谱Legendre-Galerkin方法和谱Chebyshev-Galerkin方法.数值算例表明，谱Galerkin方法不仅收敛速度快，而且能达到超几何收敛.

第一类Volterra积分方程；谱Legendre-Galerkin方法；谱Chebyshev-Galerkin方法；超几何收敛

1 引言及预备知识

许多物理和工程实际问题，如带记忆性质材料的热传导问题，多孔结构粘弹性的压缩问题和核反应堆中的热交换过程等，都可以归结为Volterra积分方程或者Volterra积分微分方程［1-2］.积分项的存在，表明这类方程具有物理过程的记忆或反馈性质，这使得它与传统的微分方程有着本质上的区别.为准确地描述这类物理过程，必须考虑系统对过去经历的记忆效应.因此，数值计算积分方程不仅存储量大，而且时间开销大.如何高效快速且高精度地求解积分方程是一大难点，也是众多学者迫切希望得以有效解决的难题.

本文主要考虑第一类Volterra积分方程

积分算子定义为

其中：核函数k( x, s)和函数f( x)充分光滑；u( x)为未知函数.通过简单的线性变换，任意区间上的第一类Volterra积分方程都能转化成为式（1）的形式.

2 谱Galerkin方法

从而得到矩阵形式

3 数值算例

表1 谱Legendre-Galerkin方法的误差和误差

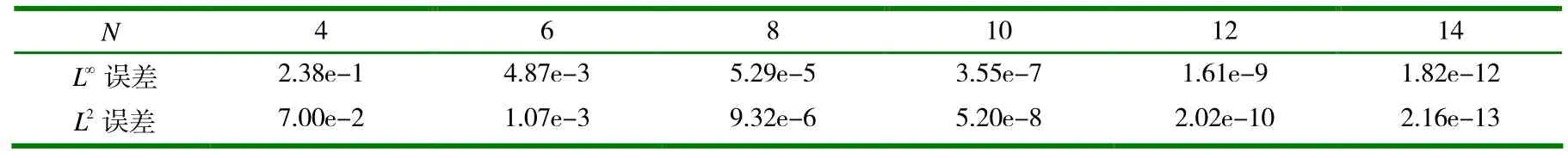
表1 谱Legendre-Galerkin方法的误差和误差

N 4 6 8 10 12 14 L 误差 2.38e-1 4.87e-3 5.29e-5 3.55e-7 1.61e-9 1.82e-12 L2误差 7.00e-2 1.07e-3 9.32e-6 5.20e-8 2.02e-10 2.16e-13

考察谱Chebyshev-Galerkin方法的收敛性质.对于式（3），取为Chebyshev多项式，运用公式（5）计算得到相应的数值解，并计算谱Chebyshev-Galerkin方法的误差和误差（见表2和图2）.

表2 谱Chebyshev-Galerkin方法的误差和误差

表2 谱Chebyshev-Galerkin方法的误差和误差

N 4 6 8 10 12 14 L 误差 1.87e-1 3.43e-3 3.41e-5 2.12e-7 8.85e-10 2.06e-11 L2 误差 1.23e-1 1.94e-3 1.71e-5 9.67e-8 3.72e-10 8.37e-12

图2 谱Chebyshev-Galerkin方法误差图

图1 谱Legendre-Galerkin方法误差图

由图1和图2可以看出，谱Legendre-Galerkin方法和谱Chebyshev-Galerkin方法同样具有超几何收敛性质.

［1］ Canuto C，Hussaini M Y，Quarteroni A，et al.Spectral methods：Fundamentals in single domains［M］.Newyork：Spring-Verlag，2006：75-90

［2］ Shen J，Tang T.Spectral and high-order methods with applications［M］.Beijing：Science Press，2006：105-131

［3］ Xie Z Q，Li X J，Tang T.Convergence analysis of spectral Galerkin methods for Volterra type integral equations［J］.J Sci Comput，2012，53：414-434

［4］ Tao X，Xie Z Q，Zhou X J.Spectral Petrov-Galerkin methods for the second kind Volterra type integro-differential equations［J］. Numer Math Theor Meth Appl，2011，4（2）：216-236

［5］ Zhang Z.Superconvergence of spectral collocation and p-version methods in one dimensional problems［J］.Math Comput，2005，74：1621-1636

［6］ Zhang Z.Superconvergence of a Chebyshev spectral collocation method［J］.J Sci Comput，2008，34：237-246

［7］ 李气发，谢资清，陶霞.谱Legendre-Galerkin方法求解线性积分微分方程的超几何收敛性分析［J］.湖南师范大学自然科学学报，2013，36（2）：1-7

［8］ Huang Can，Tang Tao，Zhang Zhimin.Supergeometric convergence of spectral collocation methods for weakly singular Volterra and Fredholm integral equations with smooth solutions［J］.J Comput Math，2011，29（6）：698-719

Supergeometric convergence of spectral Galerkin method

TAO Xia
（School of Mathematics，Hunan Institute of Science and Technology，Yueyang 414006，China）

Introduces spectral Legendre-Galerkin method and spectral Chebyshev Galerkin method for solving the first kind Volterra integral equations.Numerical results demonstrate that spectral Galerkin method not only convergent fast，but also has supergeometric convergence.

first kind Volterra integral equations；spectral Legendre-Galerkin method；spectral Chebyshev-Galerkin method；supergeometric convergence

O211.9

A

10.3969/j.issn.1007-9831.2016.06.002

1007-9831（2016）06-0006-03

2016-04-10

国家自然科学基金数学天元基金项目（11426103）；湖南省重点学科建设项目；湖南省高校科技创新团队支持计划资助项目

陶霞（1982-），女，湖南湘阴人，讲师，博士，从事偏微分方程数值解研究.E-mail：xtaohn@163.com