THE LIFE-CYCLE MODEL WITH BEQUEST MOTIVES

YANG Fan，CAI Dong-han，CHEN Zhong-bin

(1.School of Mathematics and Statistics，Wuhan University，Wuhan 430072，China）

(2.School of Economics and Management，Wuhan University，Wuhan 430072，China）

THE LIFE-CYCLE MODEL WITH BEQUEST MOTIVES

YANG Fan1，CAI Dong-han1，CHEN Zhong-bin2

(1.School of Mathematics and Statistics，Wuhan University，Wuhan 430072，China）

(2.School of Economics and Management，Wuhan University，Wuhan 430072，China）

In this paper，we study how bequest motives affect the individual's consumption and saving behaviours.By using the first conditions to solve the optimization problem，it is obtained that the individual has optimal consumption stream and bequest to maximize the all life utility under the given condition and the individual will consume more and leaves more bequest when his income increases or gets more heritage.Furthermore，the effects of four concrete bequest motives function on individual's consumption and saving behaviour are studied.It is proved that the greater the strength of the bequest motive，the greater the savings rate and bequest in the last three bequest motives and the individual's consumption increases，savings decreases with the increase of the threshold for the threshold bequest motives.

life-cycle model；bequest motive；optimal consumption path

2010 MR Subject Classification：91B99；49J15；34C60

Document code：AArticle ID：0255-7797（2016）05-1019-09

1 Introduction

Bequest motives are long recognized as potentially important determinants of saving patterns.However，there is very little work directed at describing how they affect optimal consumption patterns.

In the paper［1］，Jousten introduced a linear bequest motive into a standard life-cycle model to inquires bequests impact on annuity valuation.Recently，Dalgaard and Jensen integrated the bequest motives into classical Diamond model to study their effect on the process of capital accumulation and showed that if the bequest motive dominates，the scale effect is positive.If the life-cycle motive dominates，the scale effect is ambiguous and may even be negative［2］.

In this paper，we set up a life-cycle model with bequest motives by integrating the utility function of bequest into the life-cycle model provided by Futagami and Nakajima［3］and the method of dynamic optimization similar to［4］.It is proved the model has optimal consumption path and bequest and the individual consumes more and leaves more bequest when individual's income increased or gets more heritage.

The effects of four types of concrete bequest function on the individual's consumption and saving behaviour is discussed.In the cases of the logarithmic bequest motives and linear bequest motives，the closed-form expression of the individual's consumption and bequest are given.For the homothetic bequest motives and threshold bequest motives，we obtain that the individual has optimal consumption stream and bequest to maximize lifetime utility.It is proved that the greater the strength of the bequest motive，the greater the savings rate and bequest in the last three bequest motives and the individual's consumption increases，savings decreases with the increase of the threshold for the threshold bequest motives.

2 Setup the Model

Denote the consumption and asset of individuals who born at time 0 by c（t），a（t）at time t.The individual retires at time R and his finite lifespan is Ω＞R.Individuals derive satisfaction from their consumption and have bequest motives.We assume that individuals start their lives with assets（from heritage）and end up with bequest and have no debt，i.e.，

Assume that the individual provides inelastically a labor and earns the wage w（t）when he works and has no income when he retires，i.e.，the individual's income is given by

Therefore the individual budget constraint hence writes

where the interest rate r is a constant.

Assume that the utility functions of individual from consumption and bequest is given by

and φ（a（Ω），φ＇（·）＞0，φ＇＇（·）≤0.Then the individual's optimization problem is to maximize

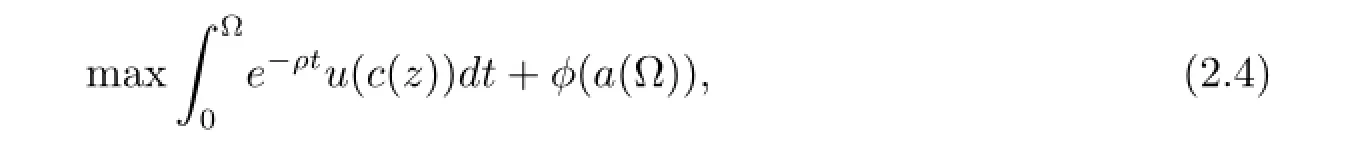
subject to（2.1）-（2.3），where ρ stands for the rate of time preference.

3 The Existence Of Optimal Consumption Path and Bequest

3.1 The First Order Conditions

The Hamiltonian for the problem is to solve the optimization problem is

The first order conditions and transversality condition are

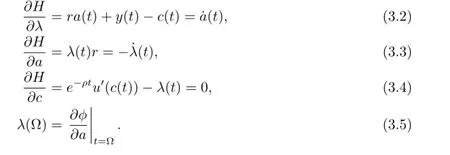
3.2 The Existence of Optimal Consumption Path and bequest

By（3.2），we have

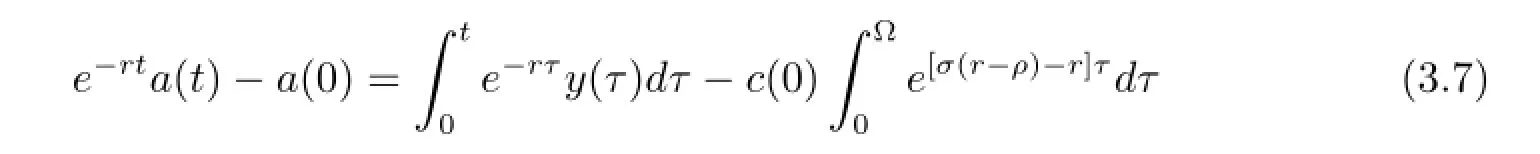
and

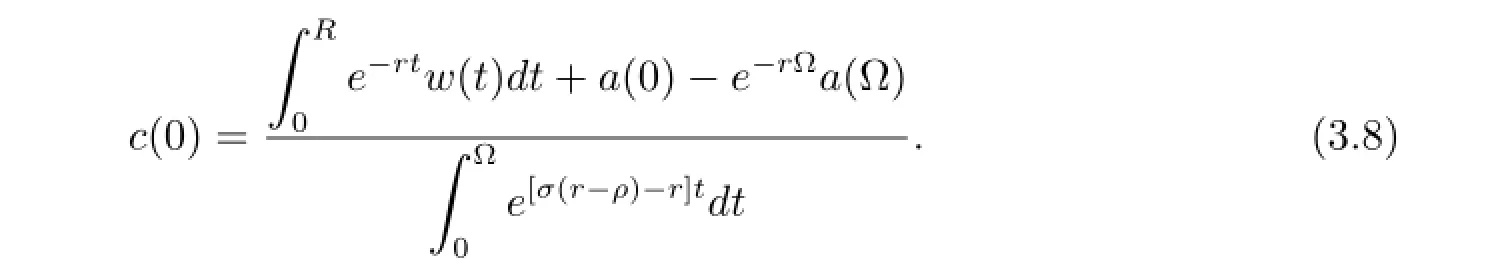
From the transversality condition（3.5），we obtain

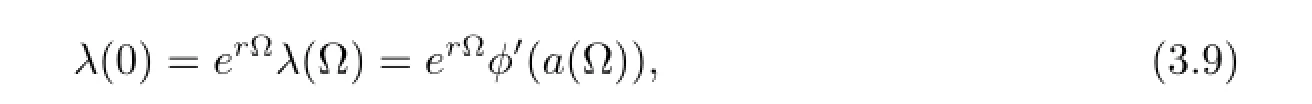
since λ（t）=λ（0）e-rt.By（3.4），u＇（c）=，we have（0）=λ（0），i.e.，

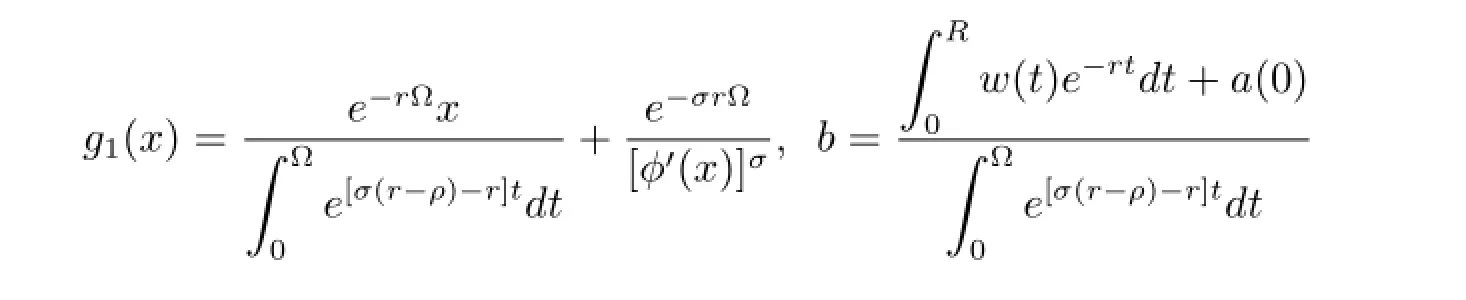
Letand g（x）=b-g1（x），then we have following lemma.

Lemma 3.1 If g1（0）＜b，then the function g（x）has a unique zero x1on the interval ［0，+∞）and there exists unique initial consumption c（0）and bequest a（Ω）such the equation （3.8）and（3.10）hold.

Proof Since

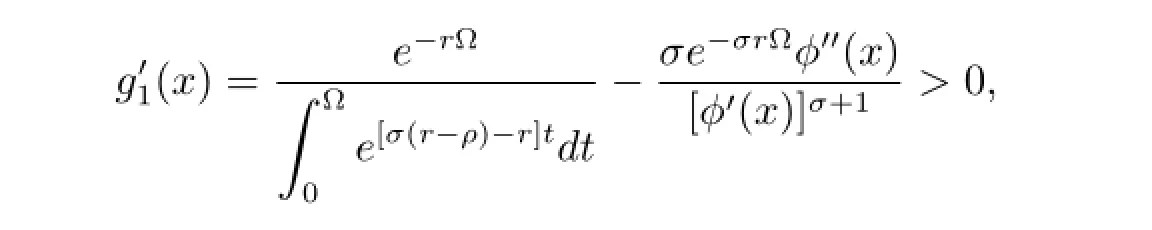
By Lemma 3.1，we obtain

Theorem 3.1 Under the condition of Lemma 3.1，the individual has optimal consumption path and bequest in his life cycle and the consumption path is given by（3.6），the initial consumption is determined by equation（3.8）and（3.10）.

From the proof of Lemma 3.1，we see that the zero x1increases when b become large. So from（3.8），we have following theorem.

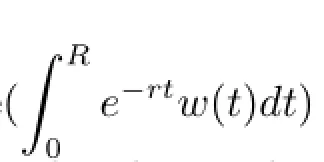
4 Four Specified Bequest Motives

In this section，the utility of individual from consumption is assumed to be logarithmic，i.e.，u（c）=lnc and the effects of four specified bequest motives on the individual's consumption and saving behaviour are discussed.

4.1 Logarithmic Bequest Motives

In this subsection，we assume that the utility functions of individuals from the bequest is logarithmical，i.e.，φ［a（Ω）］=lna（Ω）.From

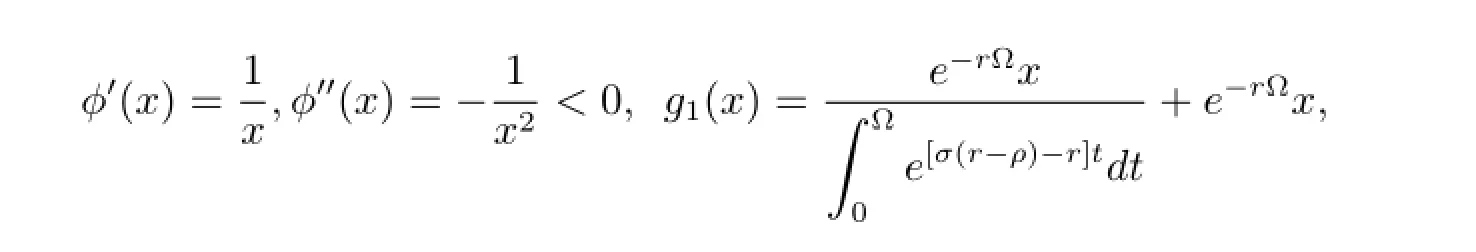
g1（0）=0 and the condition of Lemma 3.1 holds.Hence we have following theorem.

Theorem 4.1 Under logarithmic bequest motives，the individual's consumption pathand bequest and are given by

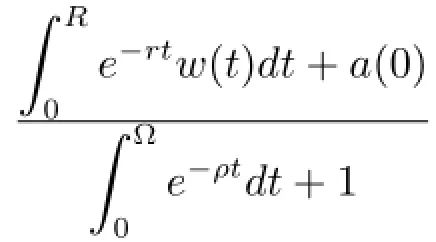
4.2 Linear Bequest Motives

In this subsection，we consider the case of linear bequest.With linear bequest motives，preferences over consumption and bequests are quasilinear and bequests are luxury goods. People with linear bequest motives leave bequests only if they have more than enough wealth to purchase their desired consumption stream.They leave any wealth in excess if this amount as bequests.Linear bequest motives are sometimes used to approximate altruistic bequest motives［4］which arise from concern about the welfare of one's heirs，and are sometimes used to describe“joy-of-giving”bequest motives［5］，which arise from enjoying giving for its own sake.Most altruists should have approximately linear bequest motives because bequests are typically small relative to recipients'total wealth.A linear bequest motive matches Hurd and Smith's estimates of the increase in anticipated bequests during the 1990s boom in asset markets almost perfectly［6］.

The utility function of linear bequest motives is given by

and from the transversality condition（3.5），

So from θ1=λ（0）e-rΩand c（0）=，we have

By（3.8），we have

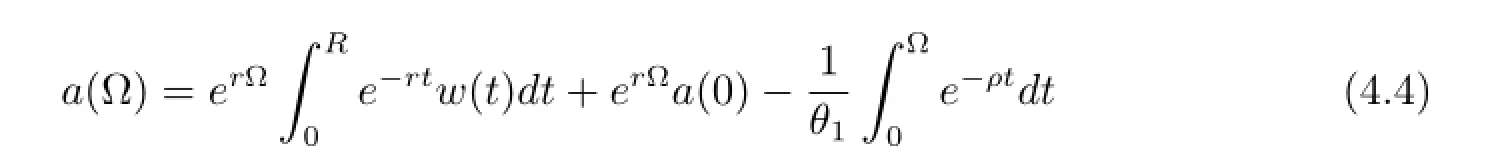
and following theorems.

Theorem 4.2 Under the linear bequest motives，the optimal consumption path and bequest are given by（4.3）and（4.4）.

Theorem 4.3 The greater the strength of the bequest motive θ1，the greater the savings rate and bequest.

Proof By（4.3）and（4.4），we have

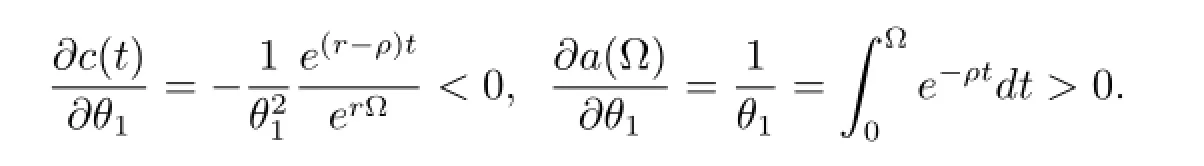
So the theorem holds.

4.3 Homothetic Bequest Motives

With this bequest motive，pover consumption and bequests are homothetic：people with twice as much wealth consume twice as much and leave bequests that are twice as large.This bequest motive is inconsistent with the evidence that bequests are luxury goods［7］.The utility function of homothetic bequest motives is given by

and

The condition of Lemma 3.1 satisfies.So from Theorem 3.1，we have following theorem.

Theorem 4.4 Under the homothetic bequest motives bequest，the individual has optimal consumption stream and bequest to maximize lifetime utility.

Theorem 4.5 The stronger the bequest motive，the greater bequest and the saving rate.

Proof Let

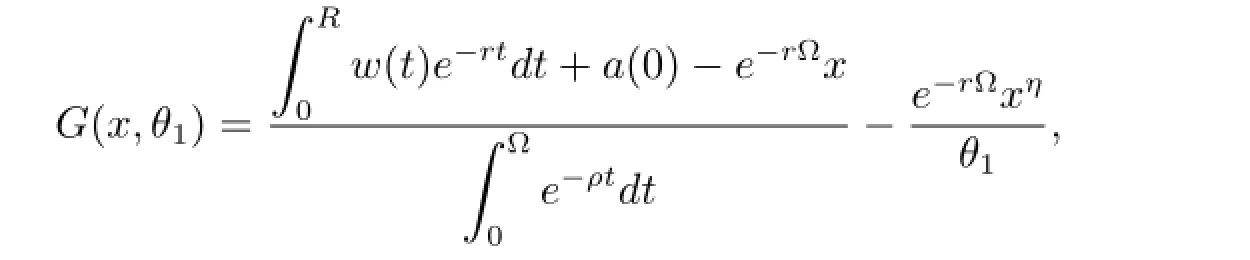
then from the proof of Lemma 3.1，there exists a unique x1＞0 such that G（x1，θ1）=0 for any given θ1＞0.Since

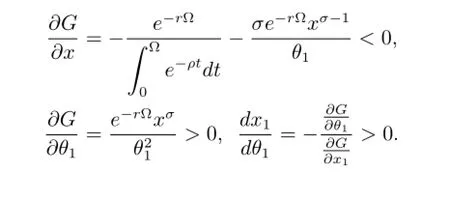
This implies that x1increases when θ1increases，i.e.，the bequest a（Ω）increases.By（3.8），the initial consumption c（0）decreases with θ1increasing as well as the consumption all life. This completes the proof of the theorem.

4.4 Threshold Bequest Motive

Threshold bequest motives used by De Nardi［7-8］are similar to linear bequest motives in that bequest are luxury goods.But they are unlike linear bequest motives in that the marginal utility of bequests decreases in the size of the bequest，which implies that people are risk averse over bequests.θ2determines the threshold wealth level below which an individual will consume all her wealth leaves no bequest.Richer individuals divide their wealth above the threshold between consumption and bequests in a fixed proportion.The larger is θ2，the higher is the threshold，and so the greater the extent to which bequests are luxury goods.

The utility function of threshold bequest motives is given by

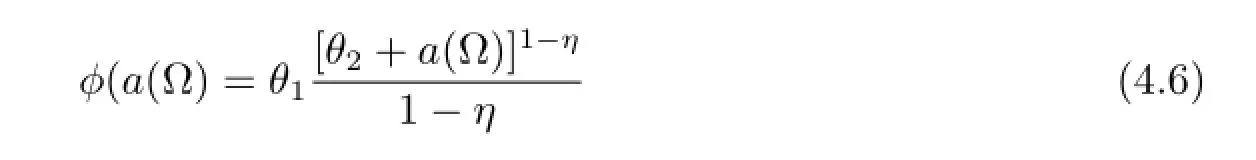
and φ＇（x）=θ1（θ2+x）-η，φ＇＇（x）=-ηθ1（θ2）x-η-1＜0，

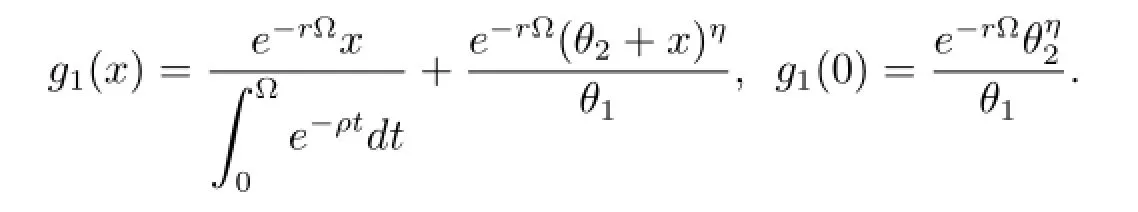
So from Theorem 3.1，we have following theorem.

Theorem 4.7 The stronger the bequest motive，the greater bequest and the saving rate.

Proof Let

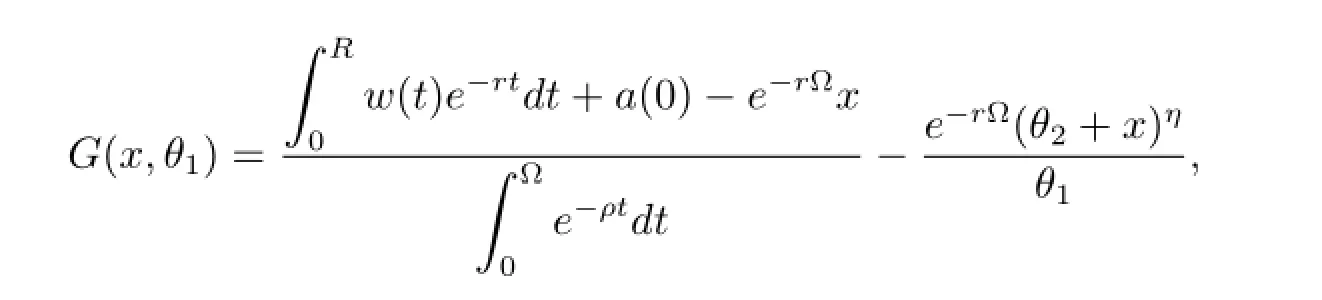
then from the proof of Lemma 3.1，there exists a unique x1＞0 such that G（x1，θ1）=0 for any given θ1＞0.Since

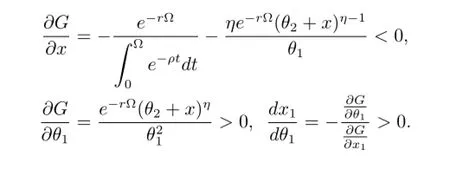
This implies that x1increases when θ1increasing，i.e.，the bequest increases.By（3.8），the initial consumption c（0）decreases with θ1increasing as well as the consumption all life.This completes the proof of the theorem.

Theorem 4.8 With the increase of the threshold θ2，consumption will increase，the savings will decrease.

Proof Let

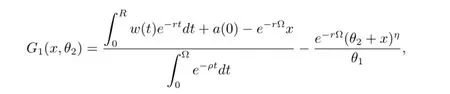
then from the proof of Lemma 3.1，there exists a unique x1＞0 such that G（x1，θ1）=0 for any given θ2＞0.

Since

This implies that x1decrease when θ2increases，i.e.，the bequest a（Ω）decreases.By（3.8），the initial consumption c（0）increases as well as the consumption all life.This completes the proof of the theorem.

5 Conclusions

In this paper，the conditions of the initial consumption and bequest needed to satisfy is first given by the first order conditions to solve the individual all life utility maximization problem.From（3.8），we first see that the individual consumes less and saving more with bequest motives（with a（Ω）＞0）than that without bequest motives（a（Ω）=0）.Then we obtain that the individual consumes more and leaves more bequest when individual's income increased or gets more heritage.Under a given condition，it is proved that there exists positive bequest when the bequest has utility for the individual and the individual chooses a optimal consumption path to maximize his all life utility.This is the reason why the Asian countries such as China has high saving rate［9-11］.

The effects of four specified bequest motives function on the individual's consumption and saving behaviours are discussed.For the cases of the logarithmic bequest motives and linear bequest motives，the initial consumption and bequests can be solved by the first order conditions.So the optimal consumption path and asset path has a closed-form expression.The effects of the strength of bequest motives on individual's consumption and saving behaviours are also inquired.It is proved that the greater the strength of the bequestmotive，the greater the savings rate and bequest in the last three bequest motives and the individual's consumption increases，savings decreases with the increase of the threshold for the threshold bequest motives which implies that the bequest are luxury goods.

References

［1］Jousten A.Life-cycle modeling of bequests and their impact on annuity valuation［J］.J.Pub.Econ.，2001，79（1）：149-177.

［2］Dalgaard C，Jensen M K.Life-cycle savings，bequest，and a diminishing impact of scale on growth［J］. J.Econ.Dyn.Contr.，2009，33（9）：1639-1647.

［3］Futagami K，Nakajima T.Population aging and economoc growth［J］.J.Macroeco.，2001，23（1）：31-44.

［4］Cai Donghan.The Ramsey model with endogenous demographic transition and simulation（I）［J］.J. Math.，2011，31（2）：191-196.

［5］Lockwood L M.Bequest motives and the annuity puzzle［J］.Rev.Econ.Dyn.，2012，15（2）：226-243.

［6］Kopczuk W，Lupton J P.To leave or not to leave：The distribution of bequest motives［J］.Rev. Econ.Stud.，2007，74（1）：207-235.

［7］Hurd M D，Smith J P.Expected bequests and their distribution［J］.NBER Working Paper，2000，9142，National Bureau of Economic Research，Inc，http：//www.nber.org/papers/w9142.

［8］De Nardi，M.Wealth inequality and intergenerational links［J］.Rev.Econ.Stud.，2004，71（3）：743-768.

［9］De Nardi M，French E，Jones J B.Why do the elderly save？The role of medical expenses［J］.J. Polit.Econ.，2010，118（1）：39-75.

［10］Loayza N，Schmidt-Hebbela K，Serv´en L.Saving in developing countries：an overview［J］.World Bank Econ.Rev.，2000，14（3）：393-414.

［11］Horioka C Y，Wan J.The determinants of household saving in China：A dynamic panel analysis of provincial data［J］.J.Money Cred.Bank，2007，39（8）：2077-2096.

具有遣赠动机的生命周期模型

杨帆1，蔡东汉1，陈忠斌2
（1.武汉大学数学与统计学院，湖北武汉430072）
（2.武汉大学经济与管理学院，湖北武汉430072）

本文研究遗赠动机对个人消费与储蓄的影响.利用解优化问题的一阶条件，得出在给定的条件下个人存在最优的消费流和遗产使得个人的终身效用达到最大化，当个人获得的收入或遗产增加时，个人将消费更多并留下更多的遗产.进一步，文中在四种具体的遗赠动机函数下研究了遗赠动机对个人消费与储蓄行为的影响，证明在后三种遗赠动机下，储蓄与遗产随遗赠动机的强度增加而赠加；在门槛动机下，随着门槛的提高，个人的消费上升，储蓄下降.

生命周期模型；遗赠动机；最优消费路径

MR（2010）主题分类号：91B99；49J15；34C60O232

date：2015-06-23Accepted date：2016-01-04

Supported by National Natural Science Foundation of China（71271158）.

Biography：Yang Fan（1983-），male，born at Yunmeng，Hubei，master，major in mathematical economy.