离散元法之振动磨介流数值模拟与试验

杨小兰 刘极峰 周亚军 王治金

摘要： 为研究磨介细观运动过程及粉碎机理，构建基于离散元法之振动磨磨介流数值模拟模型，按频幅大小设定两种振强等级、4种模式的工况组合，导出筒体运动方程，求得筒体中心运动轨迹、磨介流速矢场、速度云图等真实运动，数值模拟结果表明：相对其他模式，C模式（中频高幅）乏能区至少减小8%，筒内前端磨介动能分别为其他模式的1.26～22.31倍，其动能增加使得磨介间撞击效果强，为超微颗粒细化试验的参数优化提供了富有价值的参考；在普通和新型振动磨上分别进行试验，经激光粒度仪检测、高速摄影机记录，分别取得d（50） = 1.6，0.2 μm、带宽4.8，0.3 μm的检测结果，明显体现出新型振动磨颗粒细化和带宽窄化效果，成为超硬超微粉体细化方面的技术试验实证。关键词： 磨介流； 离散元法； 数值模拟； 振强等级； 试验比对

中图分类号： TD453+.2文献标识码： A文章编号：1004-4523（2016）03-0479-09

DOI：10.16385/j.cnki.issn.10044523.2016.03.014

引言

作为一种较为理想的细磨与超细粉磨设备，振动磨的结构有多种形式[12]，以单筒下置式振动磨为例，物料和磨介装在弹簧支撑的筒体内，偏心激振器将激振力传递给与之刚性连接的筒体，筒体将运动和力传递给筒内磨介，使物料在磨介撞击等力能作用下被研磨或粉碎。磨介的运动形式直接影响着振动磨的粉磨效果，故分析磨介细观运动过程是研究振动磨的关键问题之一[3]。

为研究磨介的细观运动特性，近年来诸多学者不断进行探索。苏乾益等利用高速摄像机对磨介运动进行拍摄，获得磨介的分布图像，建立了振幅和能量的数学模型[4]；尹忠俊等人通过摄像技术记录磨介的运动过程，建立磨介间的冲量与能量传递的数学模型[5]；唐果宁等通过高速摄像建立磨介的运动模型[6]。由于磨介运动的复杂性，高速摄像虽能观察磨介分布随时间变化的情况，却不能具体到每个磨介的运动方向与速度；数学模型通常是基于图像信息和一定假设建立的，能够描述磨介整体的宏观运动，对于磨介群体内部以及单颗磨介的细观运动很难涉及。

离散元法（Distinct Element Method，DEM）是研究不连续离散体力学行为的一种数值方法[7]，该方法在粉体的流动、分叉或成流等过程的模拟中获得了成功应用；PFC是基于离散元法来模拟圆形颗粒运动和相互作用的软件，在水利地质、岩土力学等领域，分析离散颗粒工程问题中已有应用。

V Murariu用PFC模拟不同密度、不同粒径圆形粒子在FHS中的运动及碰撞[8]；B P BHoomans利用PFC模拟在颗粒与墙体是完全弹性与完全光滑情况下，相同密度但不同粒径的颗粒的分离情况[9]；R D Morrison用PFC对粉碎机进行模拟取得一定进展[10]；在振动磨中应用PFC模拟振动磨磨介群及磨介形成的磨介流细观力学的情况，尚未见报道。

参照作者超微颗粒细化样机的试验数据，建立筒体截面的PFC模型，旨在模拟不同频幅组合下磨介流的真实运动，可获得筒体形心轨迹，磨介流速度矢场图像，再现磨介流运动过程，获得高速摄像试验中无法得到的磨介流内部流动信息，即不同参数组合下磨介的响应程度，为单个磨介的运动轨迹和速度分析，以及筒内磨介流的动能应变能变化、力链分布、力矢场、温度场等动力学分析作铺垫，为破解超微颗粒的聚团瓶颈[1112]，获取超微颗粒细化试验所需的参数选择调整，提供有价值的参考，为深入了解磨介流的动力学特性及能量分布打下基础。

5结论

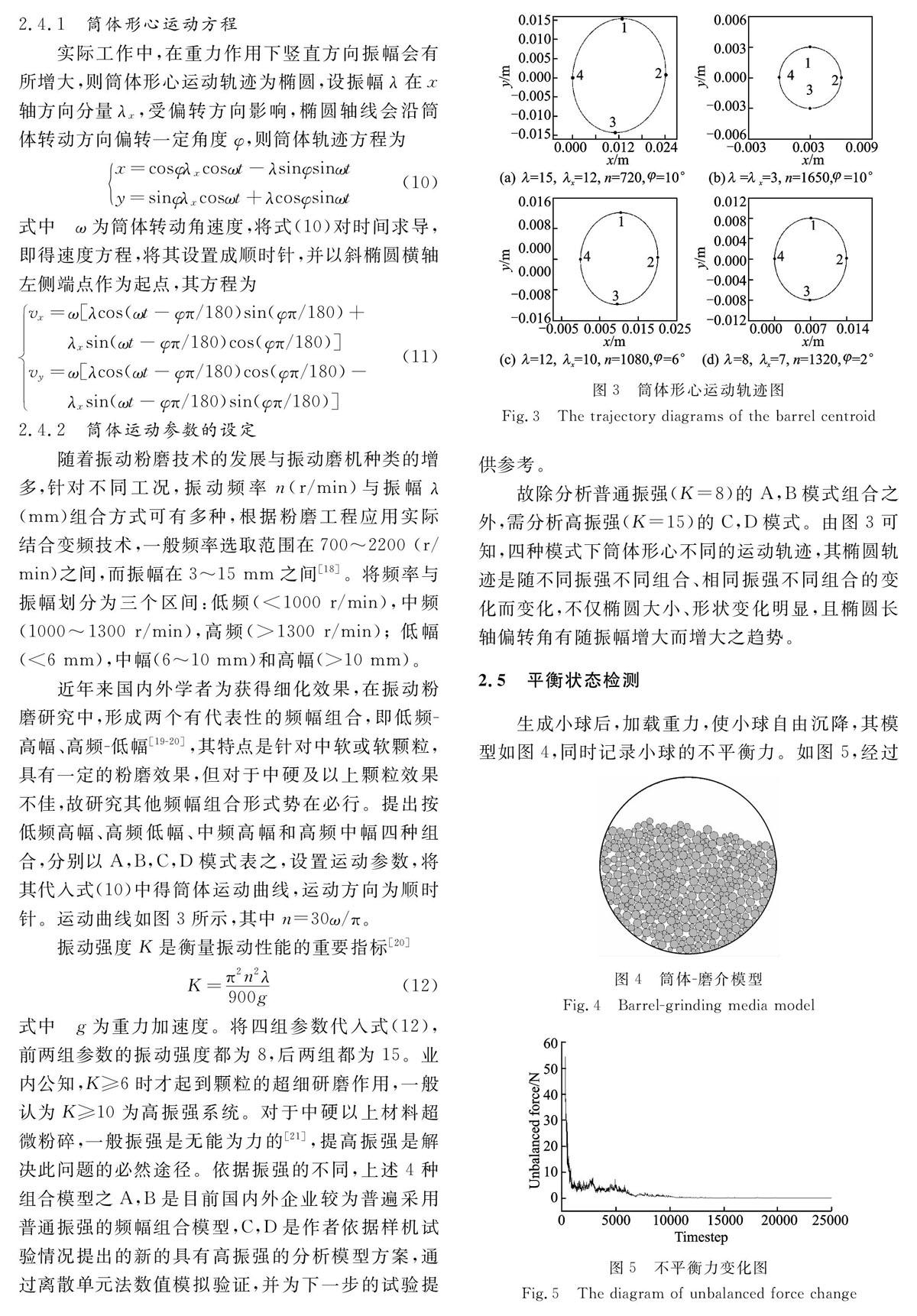
1） 进行4种频幅组合模式的PFC振动磨运动学数值模拟，获得筒体中心运动轨迹、磨介流的速矢场等运动图像，通过比对分析可知，高幅情况下磨介速度场的梯度分布明显，磨介可形成单股磨介流；高频组合下则磨介为多股磨介流，在磨介流分岔与汇合处形成低速区；两种高振强组合的粉磨效果要明显优于一般振强的组合：在高频中幅的D模式中，磨介运动速度是B模式的约2倍，磨介流形成的规模大，碰撞效果显著，但两者的磨介平均速度都不大；与A，B，D模式相比，中频高幅的C模式磨介流成流曲率大、速度大，能量传输快，能量利用率高，高速磨介多，磨介间的冲击碰撞效果强，有利于实现超微颗粒细化。

2） 在普通和新型振动磨上，同时进行两磨机基本技术参数相同的金刚石超微粉磨试验，以便验证数值模拟结果，经MS 2000型马尔文激光粒度仪检测数值、Mikrotron Gmbh高速摄影机记录图像，表明中频高幅的C模式与其它模式相比，在磨介流形成的速矢及平均速度相对较大，速矢无明显分叉，成股性好，试验及检测记录显示出C模式的新型磨机粉体细化及窄化带宽的明显效果，成为超硬颗粒振动粉碎细化方面的技术进步实证。

参考文献：

[1]盖国胜，陶珍东. 粉体工程[M].北京：清华大学出版社.2009.

Gai Guosheng， Tao Zhendong. Powder Engineering[M]. Beijing： Tsinghua University Press， 2009.

[2]Yang Xiaolan， Liu Jifeng， Jia Minping. Preparation of superhard and ultrafine particles by highintensity chaotic hibration[J]. JOURNAL OF VIBRATION ENGINEERING & TECHNOLOGIES， 2014， 2（3）：265—277.

[3]Hoon Lee， Heechan Cho， Jihoe Kwon. Using the discrete element method to analyze the breakage rate in a centrifugal/vibration mill[J]. Powder Technology， 2010， 198（3）：364—372.

[4]苏乾益，刘政.振动磨机磨介运动规律及参数设计[J].南方冶金学院学报，2002，23（1）：13—18.

Su Qianyi， Liu Zheng. The regularity of medium motion in a vibration and design of parameters[J]. Journal of Southern Institute of Metallurgy， 2002， 23（1）：13—18.

[5]Wang M H， Yang R Y， Yu A B.DEM investigation of energy distribution and particle breakage in tumbling ball mills[J]. Powder Technology， 2012， 223（6）：83—91.

[6]唐果宁，彭猛.振动磨机离散磨介动力学模型研究[J].中国机械工程，2008，19（11）：1347—1350.

Tang Guoning， Peng Meng. Research on dynamics model of disperse mill medium group for vibration mill[J]. China Mechanical Engineering， 2008，19（11）：1347—1350.

[7]An Xizhong， Yang Runyu， Dong Kejun， et al. DEM study of crystallization of monosized spheres under mechanical vibrations[J]. Computer Physics Communications， 2011， 182（9）： 1989—1994.

[8]Murariu V， Svoboda J， Sergeant P. The modeling of the separation process in a Ferrohydrostatic separator[J]. Minerals Engineering， 2005，18：449—457.

[9]Hoomans B P B， Kuipers J A M， Van swaaij W P M. Ganular dunamics simulation of segregation phenomena in bubbling gasfluidized beds [J]. Powder Technology， 2000，109：41—48.

[10]Morrison R D， Cleary P W. Towards a virtual comminution machine[J]. Minerals Engineering， 2008，21：770—781.

[11]Hua Min， Xu Dayong， Zhou Ru， et al. Simulation Research of superfine powder extinguishing agent movement[J]. Procedia Engineering， 2012， 45： 1031—1038.

[12]冯锐.我国超细粉碎机械的研究进展[J]. 硫磷设计与粉体工程，2008，（5）：13—17.

Feng Rui. Progress of research for super fine crushers in China[J]. SP & BMH RELATED ENGNEERING， 2008，（5）：13—17.

[13]Zhao X L， Evans T M.Discrete simulations of laboratory loading conditions[J]. Int.J.Geomech.，2009， 9（4）：169—178.

[14]Itasca Consulting Group Inc. PFC Particle Flow Code in 2 Dimensions[M]. Theory and Background. Minneapolis， Minnesota， 1999.

[15]孙其诚，王光谦. 颗粒流动力学及其离散模型评述[J]. 力学进展，2008，38（1）：87—100.

Sun Qicheng， Wang Guangqian. Review on granular flow dynamics and its discrete element method[J]. Advances in Mechanics， 2008，38（1）：87-100.

[16]Itasca Consulting Group Inc. PFC Particle Flow Code in 2 Dimensions[M]. Fish in PFC. Minneapolis， 2002.

[17]周国庆，周杰，陆勇，等.颗粒流程序（PFC）中阻尼参数的适用性研究[J].中国矿业大学学报， 2011，40（5）：667—672.

Zhou Guoqing， Zhou Jie， Lu Yong， et al. Selection of damping parameters used in a particle flow code （PFC2D）[J]. Journal of China University of Mining & Technology， 2011，40（5）：667—672.

[18]Zhang Chunlin. Discussion on selection and application of vibration grinding machine working parameters in magnetic powder aspects[J].Technological Development of Enterprise， 2011，30（13）：13—14.

[19]DrIng JJ Jeng， Prof Dr-Ing E Gock. Sizing of tube vibration mills with the aid of simulation model based on machine dynamics[J]. AnfbdreitungsTecknik 1992，33（7）：108—115.

[20]Zheng Yongjiang， Xie Zhaohui， Li Yigeng， et al. Spatial vibration of rolling mills[J]. Journal of Materials Processing Technology，2013， 213（4）： 581—588.

[21]Yang Xiaolan， Liu Jifeng， Zou Jingchao. Research on new techniques of superfine grinding for superhard powder by high vibration intensity[J]. Chinese Mechanical Engineering， 2009， 20（42）：2917—2921.

Discrete element on the numerical simulation and experiment of

vibration grinding medium flowYANG Xiaolan1，2， LIU Jifeng2，1， ZHOU Yajun2， WANG Zhijin1（1.College of Mechanical Engineering，Nanjing Institute of Technology， Nanjing 211167， China；

2.College of Engineering，Huanghe S & T University， Zhengzhou 450015， China）Abstract： In order to research the mesoscopic movement process and the mechanism of smashing， a numerical simulation model of the vibration grinding medium flow is built based on discrete element theory. According to various amplitude and frequency combinations， the vibration intensity is divided into two levels in four models， and the equation of the motion is derived. Consequently， the motion trajectory of the barrel center， the vector field of the grinding medium flow and the cloud picture of speed are all obtained. The results show that in mode C（midfrequency with highamplitude） the energy lacking area is reduced to at least 8%. The kinetic energy of the medium flow in the front part inside the barrel is equivalent to 1.26 to 22.31times of other modes.The increase of the kinetic energy makes the impact among the media more effective， and hence provides a valuable reference to the study of test parameter optimization in superfine grain refinement process. Experiments are conducted on both the regular and the newly developed grinding millsand the results recorded by laser particle size analyzer and high speed camera show that the refinement of particles is from d （50） = 1.6 m down to 0.2 mand the band width is narrowed from 4.8 m to 0.3 m. This can serve as evidence on superhard superfine powder refining technology advancement.Key words： grinding medium flow； discrete element； numerical simulation； grade of vibration intensity； experiment comparison作者简介： 杨小兰（1964—），女，教授。电话：（025）86118255；Email：yxhh001@163.com