Extremal Values on Distance-Degree-Based Topological Indices of Cacti with r Cycles
WANG Yu-xi, CHEN Han-lin, DENG Han-yuan
(College of Mathematics and Computer Science, Hunan Normal University, Changsha 410081, China)
Extremal Values on Distance-Degree-Based Topological Indices of Cacti with r Cycles
WANG Yu-xi, CHEN Han-lin, DENG Han-yuan
(College of Mathematics and Computer Science, Hunan Normal University, Changsha 410081, China)

Keywordscactus;thedegreedistanceindex;theGutmanindex;theadditivelyweightedHararyindex;themultiplicativelyweightedHararyindex;extremalvalue
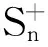
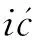
In this paper, we will consider the degree distance index [4,6,19], the Gutman index [2,12], the additively weighted Harary index [1] and the multiplicatively weighted Harary index [17]. They are defined for a connected graphGas follows, repectively


WewilldeterminethemaximalvaluesoftheadditivelyweightedHararyindex,themultiplicativelyweightedHararyindexandtheminimalvalueofthedegreedistanceindex,theGutmanindexamongallcactiofordernwithrcycles,andcharacterizethecorrespondingextremalgraphs.
1Preliminaries
For a graphG, the first Zagreb indexM1(G) and the second Zagreb indexM2(G) ofGare, respectively

ThefirstZagrebcoindexandthesecondZagrebcoindexofGare,respectively

WenowintroducethefollowingnewinvariantsforanygraphG


NotethatM*(G)=2M2(G)-M1(G), N*(G)=M1(G)+M2(G).
Beforestatingourmainresults,wewilllistsomelemmasaspreliminaries,whichwillplayanimportantroleinthenextproofs.
IfG∈G(n,r), thenm=|E(G)|=n+r-1. The following lemma is obtained from Propositions 2 and 4 in [3].
Lemma1[3]IfG∈G(n,r),n≥2, then


Lemma 2(i) LetG∈G(n,r)withlargestM1(G).ThenthemaximumdegreeΔ(G)=n-1.
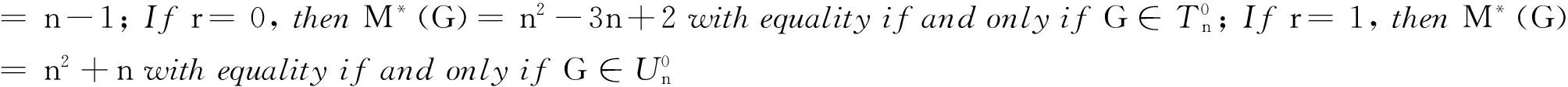
(iii)LetG∈G(n,r)withlargestN*(G).ThenΔ(G)=n-1.
2Main results
Inthissection,wewillcharacterizethemaximalgraphswithrespecttothemaximalvaluesoftheadditivelyweightedHararyindex,themultiplicativelyweightedHararyindexandtheminimalgraphwithrespecttothedegreedistanceindex,theGutmanindexamongallcactiofordernwithrcycles.
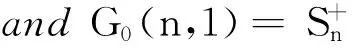
Theorem 3LetG∈G(n,r).Then
(i) M1(G)≤n2-n+6rwithequalityifandonlyifG≅G0(n,r).

(iii) N*(G)≤2n2+2nr-3n+8r+1withequalityifandonlyifG≅G0(n,r).
Now,westudythemaximalvaluesoftheadditivelyweightedHararyindex,themultiplicativelyweightedHararyindexandtheminimalvalueofthedegreedistanceindex,theGutmanindexamongallcactiofordernwithrcycles.

ProofBythedefinitionofHA,wehave

with equality if and only ifG≅G0(n,r).
Theorem5IfG∈G(n,r), thenDD(G)≥(3n+4r- 4)(n-1)-6rwith equality if and only ifG≅G0(n,r).
ProofBythedefinitionoftheDDindex,wehave



M1(G)+4(n+r-1)(n-1)-2M1(G) (by Lemma 1(1))=
4(n+r-1)(n-1)+M1(G)≥
(3n+4r-4)(n-1)-6r(by Theorem 3(i))
with equality if and only ifG≅G0(n,r).

ProofBythedefinitionofHM,wehave


Theorem7IfG∈G(n,r), then Gut(G)≥2n2+6nr-5n-4r2-16r+3 with equality if and only ifG≅G0(n,r).
ProofBythedefinitionoftheGutmanindex,wehave
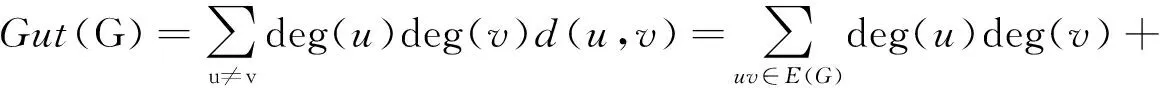
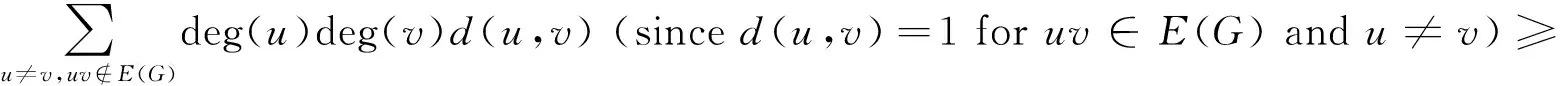
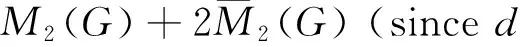
M2(G)+4(n+r-1)2-2M2(G)-M1(G) (by Lemma 1(2))=
4(n+r-1)2-(M1(G)+M2(G))=
4(n+r-1)2-N*(G) (by the difinition ofN*(G))≥
2n2+6nr-5n+4r2-16r+3 (by Theorem 3(iii))
with equality if and only ifG≅G0(n,r).
By selectingr=0 orr=1 in Theorems 4-7, we can get the following results.
Corollary8Amongalltreesofordern,

(ii)theuniquetreewiththeminimaldegreedistanceindexDDisthestarSn,andDD(Sn)=3n2-7n+4[15];

(iv)theuniquetreewiththeminimalGutmanindexGutisthestarSn,andGut(Sn)=2n2-5n+3[2].
Corollary9Amongallunicyclegraphsofordern,




References:
[1]ALIZADEH Y, IRANMANESH A, DOT. Additively weighted Harary index of some composite graphs [J]. Discr Appl Math, 2013,313(1):26-34.
[2]ANDOVA V, DIMITROV D, FINK J,etal. Bounds on Gutman index [J]. Commun Math Comput Chem, 2012,67(2):515-524.
[5]CHEN S B, LIU W J. Extremal modified Schultz index of bicyclic graphs [J]. Commun Math Comput Chem, 2010,64(3):767-782.
[6]DANKELMANN P, GUTMAN I, MUKWEMBI S,etal. On the degree distance of a Graph [J].Discr Appl Math, 2009,157(13):2773-2777.
[7]DANKELMANN P, GUTMAN I, MUKWEMBI S,etal. The edge-Wiener index of a graph [J].Discr Appl Math, 2009,309(10):3452-3457.
[8]DENG H, KRISHNAKUMARI B, VENKATAKRISHNAN Y,etal. Multiplicatively weighted Harary index of graphs [J]. J Comb Optim, 2013,30(4):1125-1137.
[10]DOBRYNIN A A, KOCHETOVA A A. Degree distance of a graph: a degree analogue of the Wiener index [J]. J Chem Inf Comput Sci, 1994,34(5):1082-1086.
[11]FENG L. The Gutman index of unicyclic graphs [J]. Discr Math Algo Appl, 2012,4(3):31-39.
[12]FENG L, LIU W. The maximal Gutman index of bicyclic graphs [J]. Commun Math Comput Chem, 2011,66(2):699-708.
[13]GUTMAN I. Selected properties of the Schultz molecular topological index [J]. J Chem Inf Comput Sci, 1994,34(5):1087-1089.
[14]HUA H, ZHANG S. On the reciprocal degree distance of graphs [J]. Discr Appl Math, 2012,160(7-8):1152-1163.
[16]IVANCIUC O, BALABAN T S, BALABAN A T,etal. Reciprocal distance matrix, related local vertex invariants and topological indices [J]. J Math Chem, 1993,12(1):309-318.
[17]LI S, ZHANG H. Some extremal properties of the multiplicatively weighted Harary index of a graph [J]. J Comb Optim, 2014,31(3):1-8.
[19]TOMESCU I. Some extremal properties of the degree distance of a graph[J]. Discr Appl Math, 1999,98(1-2):159-163.
[20]TOMESCU I. Unicyclic and bicyclic graphs having minimum degree distance [J]. Discr Appl Math, 2008,156(1):125-130.
[21]XU K, DAS K C, BALACHANDRAN S. Maximizing the Zagreb indices of (n,m)-graphs [J]. Commun Math Comput Chem, 2014,72(3):641-654.
[22]XU K, DAS K C, KLAVŽAR S,etal. Extremal (n,m)-graphs with respect to distance-degreebased topological indices [J]. Commun Math Comput Chem, 2014,72(3):865-880.
[23]XU K, LIU M, DAS K C,etal. A survey on graphs extremal with respect to distance based topological indices [J]. Commun Math Comput Chem, 2014,71(3):461-508.
[24]ZHONG L, XU K. Inequalities between vertex-degree-based topological indices [J]. Commun Math Comput Chem, 2014,71(3):627-642.
(编辑HWJ)
DOI:10.7612/j.issn.1000-2537.2016.04.013
收稿日期:2015-07-31
基金项目:国家自然科学 项目(61572190);湖南省研究生创新 项目(CX2015B162)
*通讯作者,E-mail:hydeng@hunnu.edu.cn
中图分类号O157.5
文献标识码A
文章编号1000-2537(2016)04-0078-06
具有r个圈的仙人掌图关于距离-度指数的极值
王雨溪,陈翰麟,邓汉元*
(湖南师范大学数学与计算机科学学院,中国 长沙410081)
摘要设G=(V,E)是一个连通图.G的基于距离-度的拓扑指数一般定义为(u,v)),其中F=F(x,y,z)是一个函数,deg(u)是顶点u的度,d(u,v)是u和v之间的距离.若F分别是(x+y)z,xyz,(x+y)z-1和xyz-1,则IF(G)就分别是距离指数DD(G),Gutman指数Gut(G),和加权Harary指数HA(G)与积加权Harary指数HM(G).本文确定了具有r个圈的仙人掌图关于和加权Harary指数与积加权Harary指数的最大值,以及关于度距离指数与Gutman指数的最小值;并刻画了对应的极图.
关键词仙人掌图;度距离指数;Gutman指数;和加权Harary指数;积加权Harary指数;极值

