Zygmund定理一个推广

李俊福,肖建斌,高丹丹

(杭州电子科技大学理学院,浙江 杭州310018)



Zygmund定理一个推广

李俊福,肖建斌,高丹丹

(杭州电子科技大学理学院,浙江 杭州310018)

摘要：任福尧在Zygmund函数基础上定义了Λ**函数，并获得Λ**函数的充分必要条件.该文讨论复数域C上一种新的Zygmund型函数类函数.运用类比Λ**函数的充要条件证明方法，证明了函数的p次积分平均值的充要条件，即属于平行推广，这些结论具有重要的理论意义，进一步丰富Zygmund函数类的理论.

关键词：Zygmund函数；Λ**函数；函数

0引言

1预备知识

1.1基本定义以及符号

定义1[1]Λα函数.设f(x)在复数域C上有定义，以2π为周期的复值函数，若存在正常数A使得对于任意x,y∈C,都有|f(x)-f(y)|≤A|x-y|α,0<α<1.

定义2[1]Λ*函数.设f(x)是复数域C上的连续函数，存在一个正常数A，使得对于任意的x∈C和任意的h>0，都有|f(x+h)-2f(x)+f(x-h)|≤Ah.

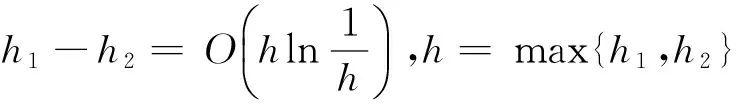
在上面3类函数定义的基础上进行定义：

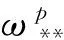
本文中的A属于正常数，在不同的位置可以表示不同的值.

1．2相关定理

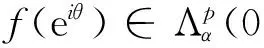
2主要定理

证明1)充分性

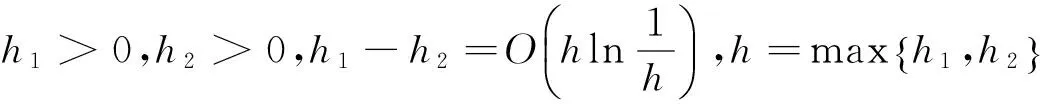
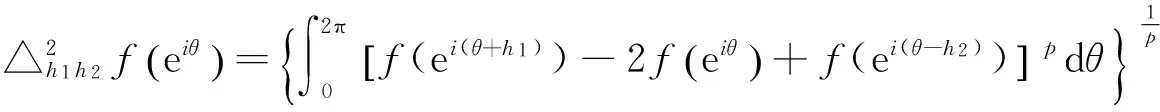
由闵科夫斯基不等式可得：

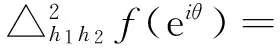
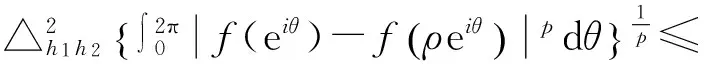
令h=1-ρ(0<ρ<1),h→0，可得：

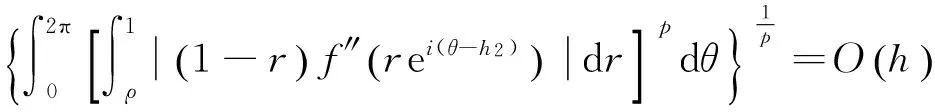
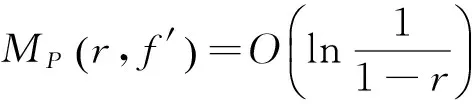
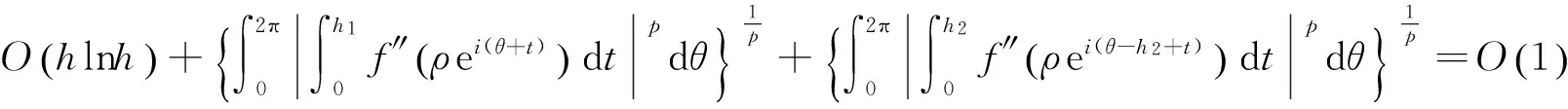
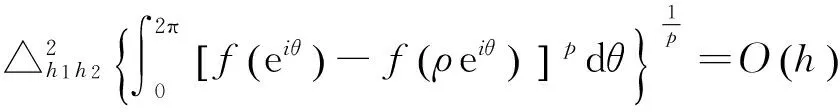
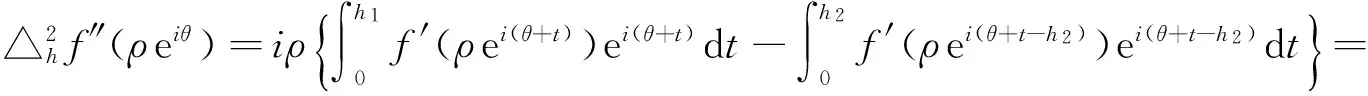
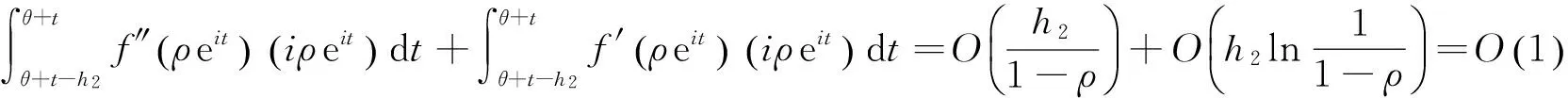
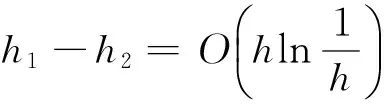
2)必要性

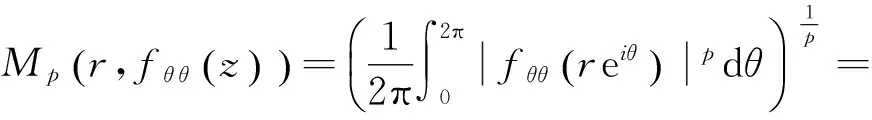
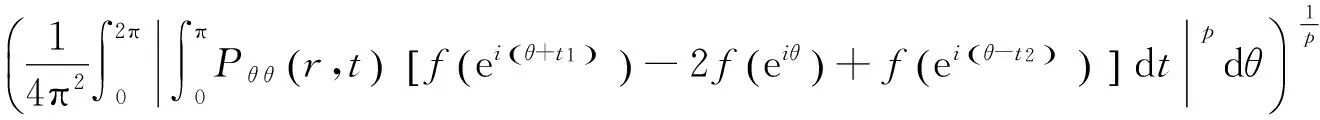
由闵科夫斯基不等式可得：

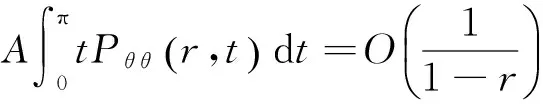
3结束语

本文给出了一种新的Zygmund型函数类，通过研究获得了Zygmund型函数类的充分必要条件，对研究Zygmund型函数类一致区间的定理和性质具有重要的意义，接下来将对其应用需要做进一步探究.

参考文献

[1]DUREN P L. THEORY OF HpSPACE[M].New York:Academic press,1970:33-51,74-80.

[2]任福尧.一个Zygmund定理的推广及应用[J].数学研究与评论,1988,8(3):391-400.

[3]RUDIN W.实分析与复分析(英文版)[M].北京:机械工业出版社,2006:50-54.

[4]王朝祥,黄心中.闭区间上Zygmund函数的延拓定理[J].华侨大学学报(自然科学版),2006,27(2):119-122.

[5]朱来义.一致区域和Zygmund定理[J].科学通报,1992,13:1153-1156.

[6]韦金生,朱来义.一致区域和Zygmund性质[J].数学杂志,1995,15(2):215-218.

[7]ZHU K H. Spaces of Hoomorphic Functions in the Unit Ballof Cn[M].New York:Springer-Verlag,2004,241-250.

Promoting a Zygmund Theorem

LI Junfu, XIAO Jianbin, GAO Dandan

(SchoolofScience,HangzhouDianziUniversity,HangzhouZhejiang310018,China)

Abstract：Ren Fuyao defines Λ**function on the basis of the Zygmund function, and obtains necessary and sufficient conditions for Λ**function. In the paper we discuss the necessary and sufficient conditions for the issue of a new class of functions of Zygmund type function on the complex field C. The use of Λ**function analog proof necessary and sufficient conditions to give proof of the p-th integral average value functions necessary and sufficient conditions that belong to parallel promotion, these conclusions are important theoretical significance, thus enriching the theory Zygmund function classes.

Key words：Zygmund function; Λ**function; function

DOI：10.13954/j.cnki.hdu.2016.04.019

收稿日期：2015-11-20

基金项目：浙江省自然科学基金资助项目(LA13A010018)

作者简介：李俊福(1989-),男,安徽阜阳人,硕士研究生,基础数学.通讯作者：肖建斌教授，E-mial:xjb@hdu.edu.cn.

中图分类号：O174.5

文献标识码：A

文章编号：1001-9146(2016)04-0090-04