非对易空间中的三维谐振子Wigner函数

王兴忠，李 康（．宁波大红鹰学院，浙江宁波3575；．杭州师范大学物理系，浙江杭州3）



非对易空间中的三维谐振子Wigner函数

王兴忠1，李 康2
（1．宁波大红鹰学院，浙江宁波315175；2．杭州师范大学物理系，浙江杭州311121）

摘 要：概述了量子相空间分布函数引入的依据、非对易空间与非对易相空间的基本性质；讨论了非对易空间中量子相空间分布函数的具体表现形式；给出了在对易空间和非对易相空间中定态Schrödinger方程和Wigner函数能量本征方程的表述式；并对照了它们在3种空间中的演变规律．最后，给出了在三维非对易空间和非对易相空间中谐振子模型Wigner函数的表达式．研究结果可以应用到更复杂的物理体系中．

关 键 词：非对易时空；相空间分布函数；Wigner分布函数

在量子力学的相空间框架中，量子现象可以用尽可能多的经典语言来描述．这种描述只要求处理常数方程而不是算符方程．

在非对易空间中量子相空间分布函数是一个重要的研究领域．1948年MOYAL利用量子相空间中的Wigner分布函数，探讨了非对易空间问题，找到了一种量子化方法，即Moyal星乘法．此方法和之前已有的量子化方法（正则量子化、路径积分量子化等）等价．与其他量子相空间分布函数相比，Wigner分布函数可以进行莫尔星乘量子化，即可以作为量子力学的一种表述形式．

许多复杂的物理模型可以分解为谐振子的组合，而谐振子的Wigner函数有明确的表达形式，所以对此进行深入研究具有十分重要的意义．

1 对易空间中的量子相空间分布函数

1．1 对易空间中的位置与动量关系

对易空间中的位置与动量关系满足：

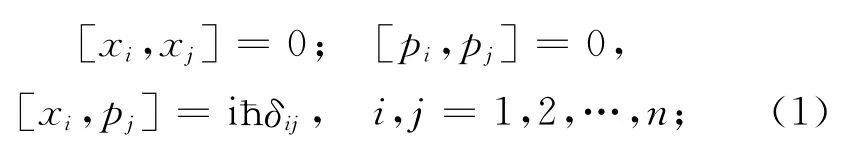
量子相空间分布函数F（q，p，t）的定义式［1］：

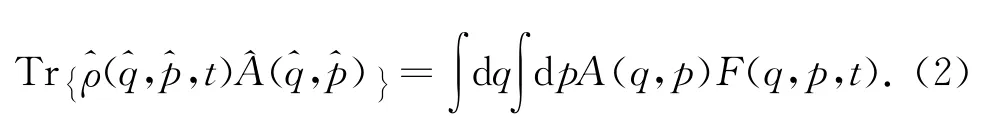
根据Cohen分类表［2］，量子相空间分布函数F（q，p，t）的一般类型可以用如下方程来定义：

1．2 三维对易空间中的Wigner函数定义式

由式（3），取f（ξ，η）＝1，即得Wigner分布函数［3］：

此空间中的Wigner函数能量本征方程由星乘形式表示，为

已知，三维谐振子（其质量为μ，频率为ω）的Hamilton量为

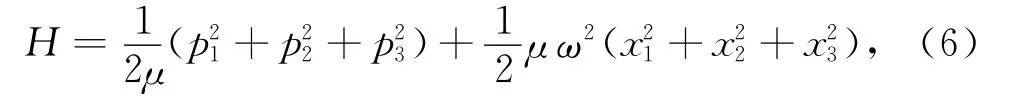
其在坐标表象下的Schrödinger方程为

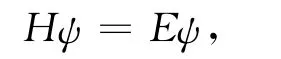
解此方程可得［4］：

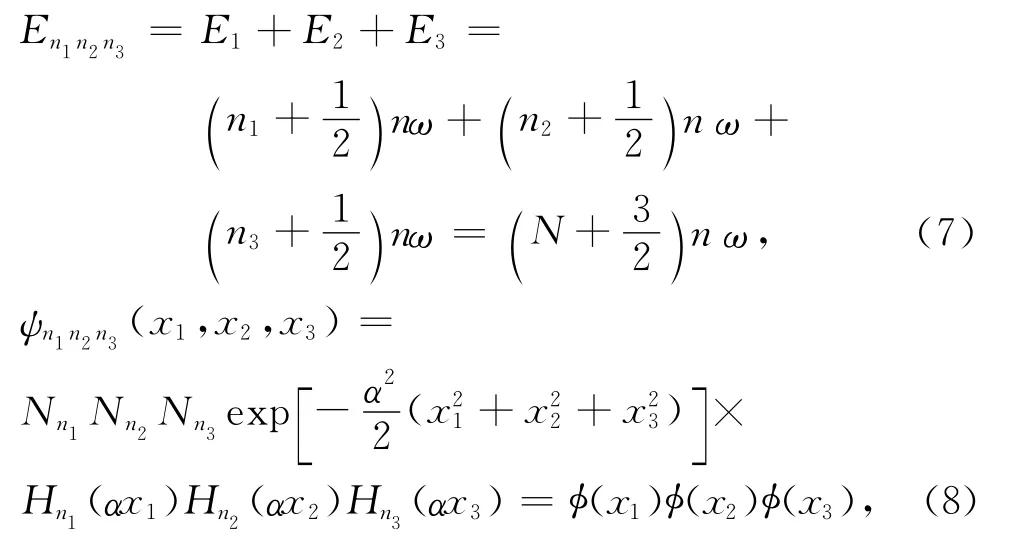
把式（8）直接代入式（4）或式（5），都可以得到三维对易相空间中谐振子的Wigner函数表示式［5］，即

2 非对易空间中的三维谐振子Wigner函数［6］

非对易空间（NC space）中的位置与动量关系满足［7］：

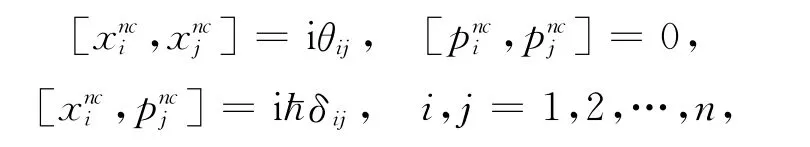
其中，

非对易空间中的薛定谔方程通常可表示为

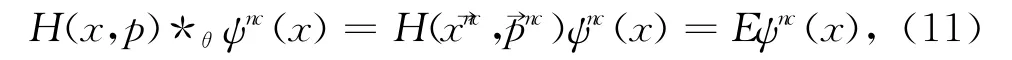
此空间中相关Bopp变换的表示式为

参照式（3），有非对易空间的三维Wigner分布函数表达式：

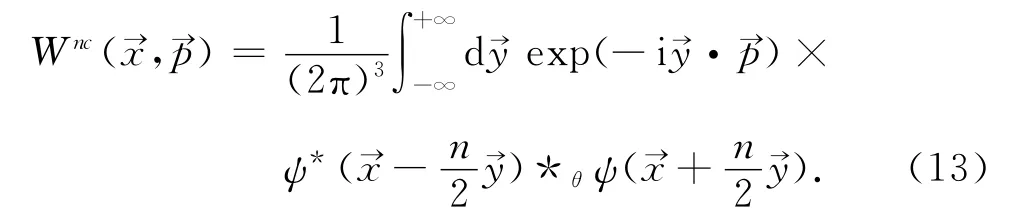
非对易空间中关于Wigner函数的能量本征方程为

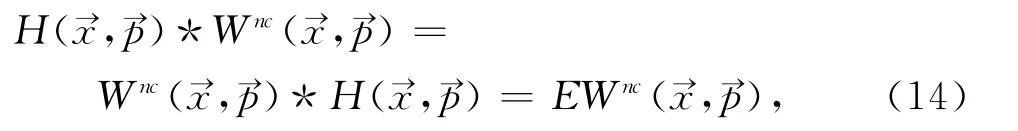
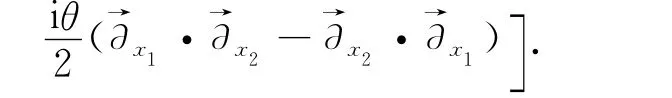
Wigner分布函数的能量本征方程经过式（12）Bopp变换后，可写成如下形式：

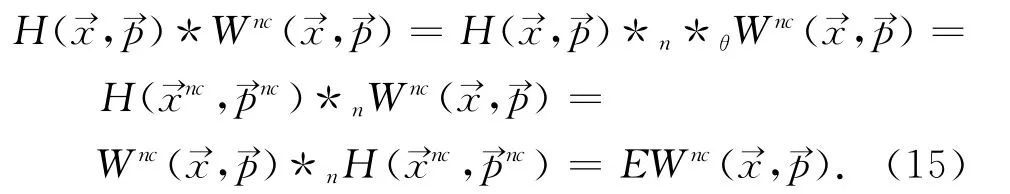
在非对易空间中，三维谐振子的Hamiltonian为（令μ＝1，ω＝1）：

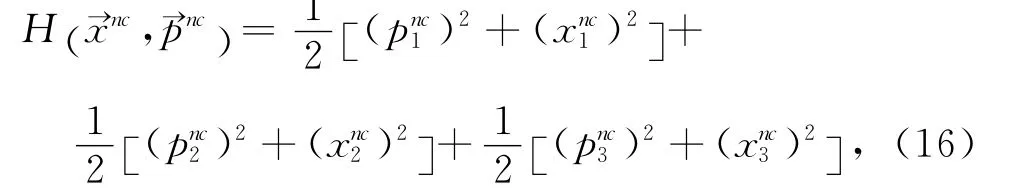
把式（16）代入式（15），经计算可得三维谐振子在非

对易空间中用对易空间中的坐标与动量表示的Wigner函数：

3 非对易相空间中三维谐振子的Wigner函数［8］

非对易相空间（NC phasc space）中的位置与动量的关系满足：

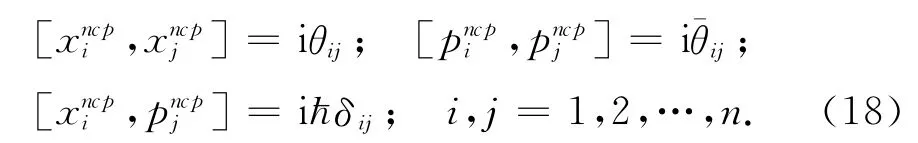
非对易相空间中的定态Schrödinger方程可以表示为

此空间中相应的Bopp变换式：

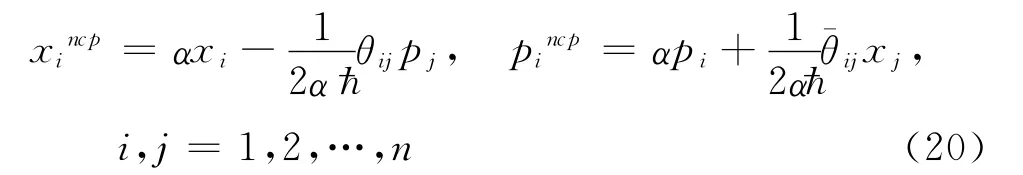
同样，三维非对易相空间中的Wigner分布函数可以表示为

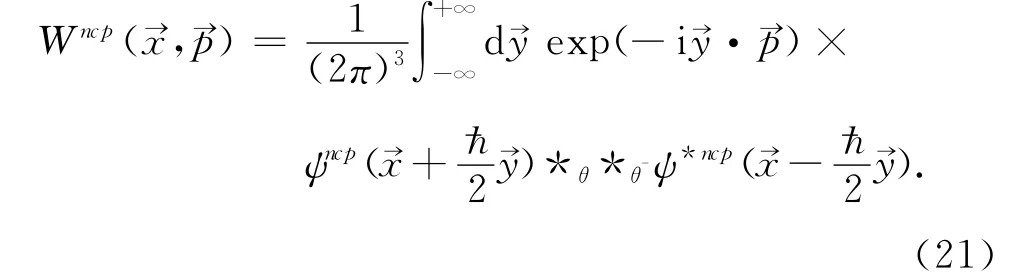
非对易空间中关于Wigner分布函数的能量本征方程可表示为

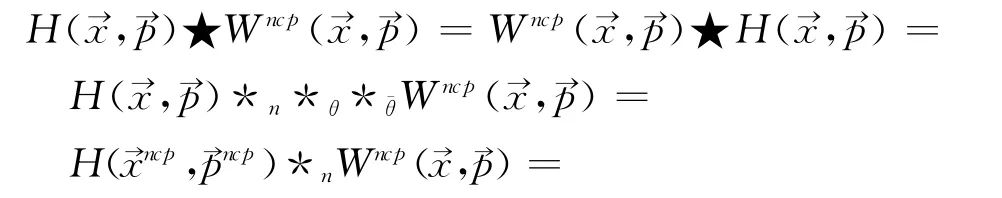
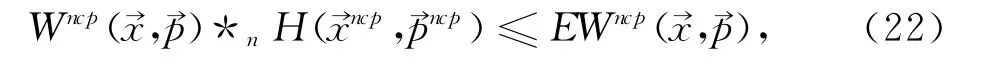
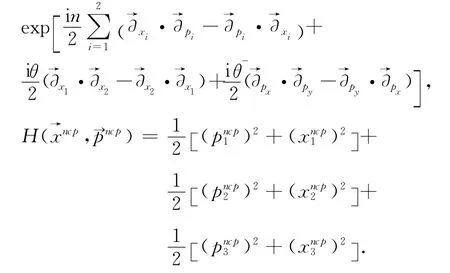
把三维谐振子的波函数式式（8）代入非对易相空间Wigner分布函数的定义式式（21），或利用求解非对易相空间中Wigner分布函数的本征方程式（22），都可以得到三维谐振子在非对易相空间中的Wigner函数：

再利用Bopp变换，最后用对易空间中的坐标与动量来表示处于非对易相空间中的三维谐振子Wigner分布函数，可得如下式子：

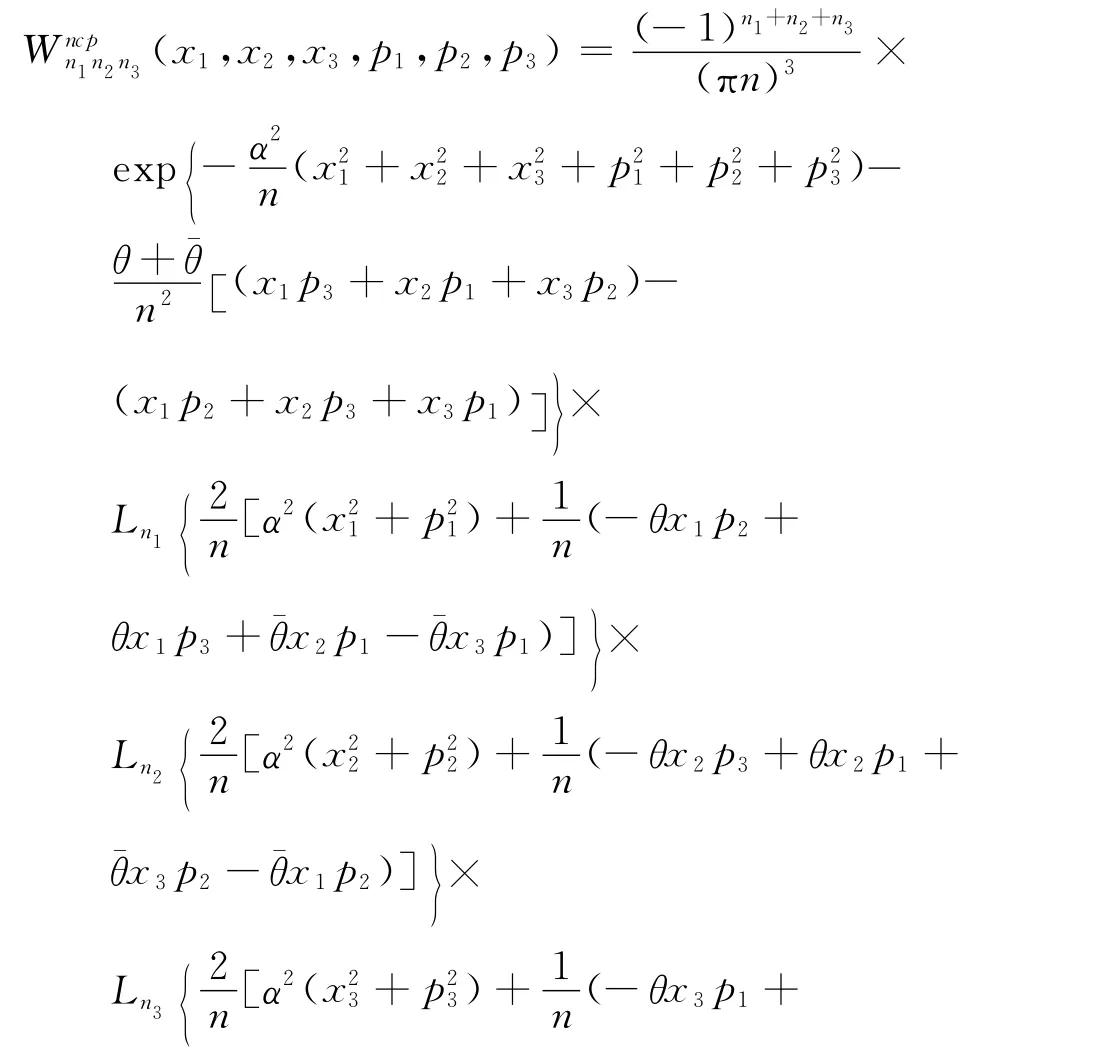
4 结 论

简析了量子相空间分布函数引入的基本依据．系统概述了常规的对易空间、非对易空间与非对易相空间的基本性质．给出了在这3种空间中的定态Schrödinger方程各自的表达式以及Wigner函数能量本征方程的表达式，从中可以清晰地看到这些表示式在3种空间中的递进演变规律．最后，给出了在非对易空间和非对易相空间中的三维谐振子的Wigner分布函数表达式．

参考文献（References）：

［1］ LEE H W．Theory and application of the quantum phase－space distribution functions［J］．Physics Reports，1995，259：147－211．

［2］ COHEN L．Generalized phose space distributions［J］．J Math Phys，1966（7）：781．

［3］ WIGNER E．On the quantum correction for thermodynamic equilibrium［J］．Phys Rev，1932，40：749－759．

［4］ 喀兴林．高等量子力学［M］．第2版，北京：高等教育出版社，2001：118．KA Xinglin．Advanced Quantum Mechanics［M］．2nd ed，Beijing：Higher Education Press，2001：118．

［5］ 马凯，李康，王剑华．非对易相空间中的Moyal方程和Wigner函数［J］．浙江大学学报：理学版，2011，38（2）：163－167．MA Kai，LI Kang，WANG Jianhua．Moyal equation and Wigner function in non－commutative phase space ［J］．Journal of Zhejiang University：Science Edition，2011，38（2）：163－167．

［6］ LI Kang，WANG Jianhua，DULAT S，et al．Wigner functions for Klen－Gordon oscillator in non－commutative space［J］．Int J Theor Phys，2010，49：134－143．

［7］ LI Kang，DULAT S．The Aharonov－Bohm effect in non－commutative quantum mechanics［J］．Eur Phys J，2006，C46：825－828．

［8］ LI Kang，WANG Jianhua，CHEN Chiyi．Representation of noncommutative phase space［J］．Modern Physics Letter A，2005，20（28）：2165－2174．

The Wigner function of the three dimensional harmonic oscillator in non－commutative space

WANG Xingzhong1，LI Kang2（1．Ningbo Dahongying University，Ningbo 315175，Zhejiang Province，China；2．Department of Physics，Hangzhou Normal University，Hangzhou311121，China）

Journal of Zhejiang University（Science Edition），2016，43（1）：075－078

Abstract：Firstly，the basic introduction to the distribution function in quantum phase space and the basic properties of non－commutative space and non－commutative phase space were briefly introduced．Then the specific forms of the quantum phase space distribution functions in non－commutative space were also discussed；The specific forms of expression of the static Schrödinger equation and the energy eigenvalue equation of Wigner function were introduced in commutative space and non－commutative phase space；The evolution rule of the forms in the three dimension spaces had been clearly proposed by comparison．Finally，the Wigner distribution functions of the harmonic oscillator in three dimensional non－commutative space were given explicitly．The result of the study can be applied to more complex physical systems．

Key Words：non－commutative space－time；phase space distribution functions；Wigner distribution function

作者简介：王兴忠（1966－），男，工程师，硕士，主要从事量子相空间分布函数研究及电化教育工作，E－mail：wangxz665049＠163．com．

基金项目：国家自然科学基金资助项目（11175053，11475051）．

收稿日期：2015－03－23．

DOI：10．3785／j．issn．1008－9497．2016．01．013

中图分类号：O 411．1

文献标志码：A

文章编号：1008－9497（2016）01－075－04