广义mKdV方程的精确解研究

张红伟，张强，黄英

(1湖南科技大学建筑与规划学院，湖南湘潭411100；2楚雄师范学院数学与统计学院，云南楚雄675000)

广义mKdV方程的精确解研究

张红伟1,2，张强2，黄英2

(1湖南科技大学建筑与规划学院，湖南湘潭411100；2楚雄师范学院数学与统计学院，云南楚雄675000)

modified Korteweg-de Vries（mKdV）方程是一个精典的孤子方程。利用行波变换法把广义mKdV方程转化为常微分后，再利用降阶法和初等积分法求出了广义mKdV方程的一系列的精确行波解。

广义mKdV方程；精确解；行波变换法；降阶法

引言

广义modified Korteweg-de Vries（mKdV）方程

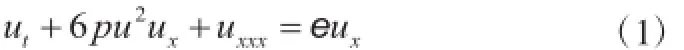
具有丰富的物理背景，其中，为任意常数。当时，方程（1）成了经典的mKdV方程，它可作为非调和晶格中描述等离子和声子多重作用的孤立子模型[1]，而当是一个充分小的正数时，方程（1）就变成了扰动mKdV方程，它主要出现在准一维固体的研究中，用于描述原子链和液晶流体力学[2]。特别地，作变换之后,方程（1）被变成了KdV-mKdV组合方程

该方程是等离子体物理和固体物理中的重要模型[1]。一般而言，p=1时，称

为“好的”广义mKdV方程，p=-1时，称

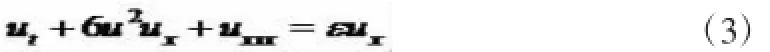
为“坏的”广义mKdV方程。

mKdV方程是一个精典的孤子方程，有关它的精确解有很多学者[3]-[9]研究，但有关扰动mKdV方程和的研究结果并不多见[10]。所以，有必要对方程（1）的行波解进行研究。

一、“好的”广义mKdV方程的解

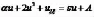
其中，A、B为积分常数。接下来，只需要对常微分方程(4)进行求解，就可以找到方程(2)的特解。

把方程(4)变为

若A=B=0，则用分离变量法可以求解方程(5)，从而得到方程(2)的钟状孤立波解

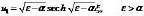
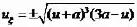
求解之后得到一个有理解

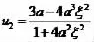
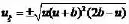
从而得到方程（2）的周期解

利用椭圆积分求解(6)式可得另外两个特殊的周期解

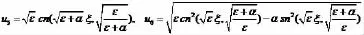
在u1-u5中，ε可以等于零，当ε=0时,这些解就是经典mKdV方程的行波解，但u6在中，ε不能等于零，说明只能是广义mKdV方程(2)的解。此外，后面四种类型的解在相关研究[11]-[16]中极少甚至没有出现过。

二、“坏的”广义mKdV方程的解

经过相同的行波变换和降阶处理后，方程(3)变为

和钟状孤立波解

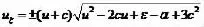
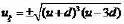
求解之后得到一个有理解

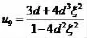
解之得下列周期解

和

在u1-u9以及u12中，ε可以等于零，但u10-u11在中，ε不能等于零，说明典型的mKdV方程不具有形如u10-u11的解，它们只能是广义mKdV方程(2)的解。

[1]李志斌.非线性数学物理方程的行波解[M].北京:科学出版社，2006.

[2]ABLOWITZMJ,CLARKSONPA.Solitons,Nonlinear EvolutionsandInverseScattering[M].Cambridge：Cambridge University Press，1991.

[3]陈登远.孤子引论[M].北京:科学出版社，2006.

[4]黄英，杨波，马祖彦.MKdV方程的新精确孤立波解[J].河南城建学院学报，（2010）（1）.

[5]HUANG Y，MA Y，LI B R.Skill application of the theory: new solitary wave solutions and periodic solutions of the modified KdV equation[J].International journal of Functional Analysis,Operator Theory and Applications，2011(01).

[6]WADATI M.The modified Korteweg-de Vries equation[J]. Phys Soc，1972（32）.

[7]YANG Z Y,JIANG T.Application of Bifurcation method for gengralized MKdV equation[J].Jouranl of Yuannan University,2002(01).

[8]套格图桑，斯仁道尔吉.MKdV方程和mKP方程的新精确孤立波解[J].高校应用数学学报A辑,2007(1).

[9]HIROTA R.Exact solution of the modified Korteweg-de Vries equation formultiple collisions of solitons[J]. Phys Soc，1972(33).

[10]JIAO X Y,ZHENG Y,WU B.Approximate homotopysymmetry and infiniteseries solutions to the perturbed mKdV equation[J].Applied Mathematics and Computation,2012(218).

[11]WAZWAZAM.Multiple-solitonsolutionsofthe perturbed KdV equation Communications in Nonlinear Science and Numerical Simulation[J].2010(15).

[12]ZARMI Y.From single-to multiple-soliton solutions oftheperturbedKdVequation[J].PhysicaD: Nonlinear Phenomena,2008(237).

[13]VEKSLER A,ZARMI Y.Freedom in the expansion and obstaclestointegrabilityinmultiplesoliton solutions of the perturbed KdV equation[J].Physica D:Nonlinear Phenomena,2006(217).

[14]JIAO X Y,ZHANG H Q.An extended method and its applicationtoWhitham-Kaupequationand two-dimensional perturbed KdV equation[J].Applied Mathematics and Computation,2006(172).

[15]YU J,ZHANG W J,GAO X M.Dynamical behavior in the perturbed compound KdV-Burgers equation[J].Chaos, Solitons and Fractals,2007(33).

[16]GU C H,HU H S,ZHOU,Z X.Darboux Transformation In Soliton Theory And Its Applications On Geometry[J]. Shanghai Scientific and Technical Publishers,2005.

责任编辑：李凡生

Study on the Exact Solutions to the Generalized mKdV Equation

ZHANG Hong-wei,ZHANG Qiang,HUANG Ying (1.Hunan University of Science and Technology,Hunan Xiangtan,411100;2.Chuxiong Normal University,Yunnan Chuxiong,675000)

In the paper,the modified Korteweg-de Vries(mKdV)equation is reduced to an ordinary equation by means of the method of travelling transformation,then the methods of reduction order and elementary integral are used for solving a series of exact solutions to the mKdV equation.

generalized mKdV equation,exact solution,travelling transformation method,reduction order method

0175.2

A

1674-8891（2016）03-0004-03

2015-11-23

云南省教育厅资助项目（编号：2012Y130）；国家自然科学基金资助项目（编号：11261001）。

张红伟（1986—），男，河南项城市人，湖南科技大学建筑与规划学院硕士研究生，研究方向：非线性发展方程；黄英（1973—），女，云南大姚人，云南省楚雄师范学院数学与统计学院教授，主要从事非线性发展方程和无穷维动力系统的研究。