非自反Banach空间中的Lagrange型凸泛函

龚丽燕，张秋园

(广东工业大学 应用数学学院，广东 广州 510520)



非自反Banach空间中的Lagrange型凸泛函

龚丽燕，张秋园

(广东工业大学 应用数学学院，广东 广州 510520)

摘要:在非自反Banach空间X中讨论了Lagrange型凸泛函及其对偶的一些性质.引入了两个广义次微分概念，进一步研究了它们之间的关系，并指出了非自反Banach空间中的Lagrange型凸泛函具有B自对偶性.

关键词:非自反Banach空间； 弱*下半连续； 凸泛函

凸函数是许多数学分支中的一个重要研究对象，其性质的研究受到各学科领域的广泛关注.1996年，Rockafellar[1]在Banach空间上研究了凸泛函的次微分及其一些性质.2008年，Ghoussoub[2-3]在凸泛函的条件下提出自反的Banach空间上用自对偶变分法解决一类不适合用Euler-Lagrange法的偏微分方程，随后Galichon[4]、Ricceri[5]等对变分理论的研究均是建立在凸泛函的基础上.可见，凸泛函的应用非常广泛.

本文受此启发,参考文献[6-8]中的变分理论，对非自反Banach空间中对Legendre-Fenchel对偶变换和某些向量场的性质进行了探讨，这些结果对研究非自反Banach空间中的变分理论有着重要作用.

1预备知识

首先，回顾一些相关概念及定理，其他概念可参考文献[9-11].

定义1[12]如果赋范空间X到X**的自然映射是满射的，则称X是自反的，记X=X**.

定义3[14]若X是实局部凸空间，泛函φ:X→R∪{+∞}，则φ*:X*→R∪{+∞}为

定义4[14]若φ,ψ是Banach空间X中的的下半连续凸泛函，则

φ*ψ=inf{φ(y)+ψ(x-y);y∈x}.

引理1[12]任一赋范空间X与其二次对偶空间X**的某一子空间等距线性同构.

引理2[14]令h(x)=

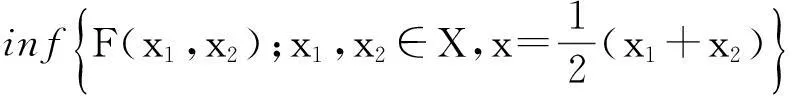
引理3[14]定义在X×X上的函数g(x1,x2)=‖x1-x2‖2，其中X是Banach空间，则

φ**=φ；

2主要结果

定理1若X是非自反Banach空间，f1,f2:X*→R∪{+∞}是两个弱*下半连续凸泛函，定义

h(x)=

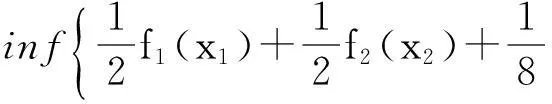
h*(p)=

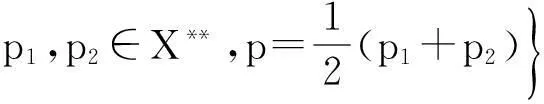
证明令F(x1,x2)=g1(x1,x2)+g2(x1,x2),

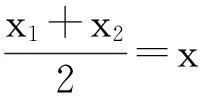
下证h(x)的Legendre对偶变换为h*(p).

由引理2可得

根据引理3可得

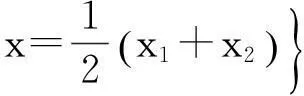
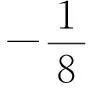
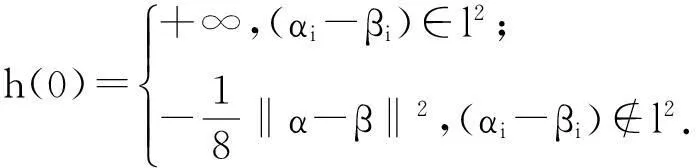
根据Legendre-Fenchel变换,可得

同理

应用定理1的结论

h*(p)=

通过直接计算与使用定理1的结论求出h(x)的Legendre-Fenchel变换h*(p)结果相同.

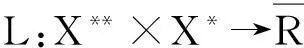
δ*L(x)=

δ*L(x)可以是空集.命题[16]：x→δ*L(x)是单调映射.若X是自反Banach空间，则δ*L(x)=δL(x)[4]，否则不一致[16].

L(x+y,p+q)-L(x,p)≥

++t.

令t→0+，得L(x+y,p+q)-L≥

又X**×X*⊃X×X*，

例1设

L:l∞×l1→R，l∞,l1均是非自反Banach空间，定义L(x,p)=‖x‖l∞+‖p‖l1,其中

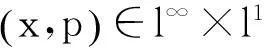
(1) 求δ*L(x).

+≤L(x+y,p+q)-L(x,p)=

‖x+y‖l∞+‖p+q‖l1-‖x‖l∞-‖p‖l1≤

‖y‖l∞+‖q‖l1

(1)

其中x,y∈c0;p,q∈l1.

式(1)对任意(y,q)∈c0×l1均成立，则

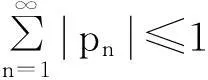
可得，‖x‖l∞≤1时，δ*L(x)=B(0,1)，否则，δ*L(x)=Ø.

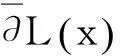
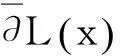
B:X**→X**是一有界线性算子，定义B*:X*→X*如下，且=,∀f∈X*,y∈X**.

命题：B*是一有界线性算子，且‖B‖=‖B*‖，所以B*有界.

证明线性性显然，令‖f‖=‖y‖=1，一方面，因为B是有界线性算子，则有≤‖B‖‖f‖‖y‖，所以B*有界，且‖B*‖≤‖B‖；反之B*是有界线性算子，故≤‖B*‖‖f‖‖y‖，得‖B‖≤‖B*‖，因此‖B‖=‖B*‖.

定理3若X是非自反Banach空间，设

φ*(Bx)+φ(p)，L*(B*p,Bx)=

证明因为L*(B*p,Bx)=

L(x,p)=φ*(Bx)+φ(p)，所以有

L*(B*p,Bx)=

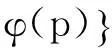
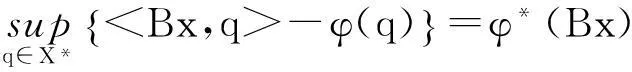
又φ**=φ，故L*(B*p,Bx)=L(x,p).证毕.

参考文献：

[1] ROCKAFELLAR R T.Characterization of the subdifferentials of convex funcions[J].Pacific Journal of Math,1966,17(3):497-509.

[2] GHOUSSOUB N. A variational theory for monotone vector fields[J]. Journal Fixed Point Theory Applications,2008,1(4):107-135.

[3] GHOUSSOUB N.Maximal monotone operators are selfdual vector fields and vice-versa[EB/OL].(2006-10-16)[2008-02-02].http:∥arxiv.ora/abs/math/0610494v1.

[4] GALICHON A, GHOUSSOUB N.Variational representations for N-cyclically monotone vector fields[EB/OL].(2012-07-10)[2013-10-02].http:∥arxiv.ora/abs/1207.2408v3.

[5] RICCERI B.A general variational principle and some of its applications[J].Journal of Computational and Applied Mathematics,2000,1(113):401-410.

[6] AUCHMUTY G.Duality for non-convex variational principles[J].Journal of Differential Equation,1983,1(50):80-145.

[7] HESTENSE M R.On variational theory and optimal control theory[J].SIAM,1965,3(1):23-48.

[8] LI S J,YANG X Q,CHEN G Y.Vector Ekeland variational principle[J].Nonconvex Optimization and Its Appl,2000,(38):321-333.

[9] CHEN Y Q,CHO Y J.Nonlinear Operator Theory in Abstract Spaces and Applications[M].New York: Nova Science Publishers,Inc,2004.

[10]ROCHAFELLAR R T.Convex Analysis[M].New Jersey:Princeton University Press,1972.

[11] EKELAND I.On the variational principle[J]. Journal of Mathematical Analysis and Applications,1974,47(2):324-353.

[12] 姚泽清,苏晓冰,郑琴,等.应用泛函分析[M].北京:科学出版社,2007.

[13] 郭大钧.非线性泛函分析[M].2版.山东:山东科学技术出版社,2001.

[14] GHOUSSOUB N.Self-dual Partial Differential Systems and Their Variational Principles (Springer Monographs in Mathematics)[M]. New York: Springer,2008.

[15] 蒋平川,王伟.非自反空间内的自对偶lagrange凸泛函[J].广东工业大学学报,2013,30(4):107-110.

JIANG P C,WANG W. About the self-dual lagrangian convex functional within the non-reflexive space[J].Journal of Guangdong University of Technology,2013,30(4):107-110.

[16] CHEN Q Y, CHO Y J.Monotone type operators in non-reflexive Banach spaces[J].Fixed Point Theory Applications,2014:119.doi:10.1186/1687-1812-2014-119.

Lagrange Convex Functional in Non-reflexive Banach Space

Gong Li-yan, Zhang Qiu-yuan

(School of Applied Mathematics, Guangdong University of Technology, Guangzhou 510520, China)

Abstract:This paper discusses the Lagrange convex functional and the duality property in non-reflexive Banach space. In the discussion, two concepts about generalized subdifferential have been introduced and their relationship has been studied. Also it is pointed out that the Lagrange convex functional has the B-self-dual property.

Key words:non-reflexive Banach space; weak*lower semi-continuous; convex functional

中图分类号:O177.91

文献标志码:A

文章编号:1007-7162(2016)01- 0073- 04

doi:10.3969/j.issn.1007- 7162.2016.01.014

作者简介:龚丽燕(1989-)，女，硕士研究生，主要研究方向为非线性泛函分析.

收稿日期:2014- 05- 29