裂尖具线性分布约束应力的运动裂纹模型及其解析解
第一作者唐雪松男,教授,博士,1964年生
裂尖具线性分布约束应力的运动裂纹模型及其解析解
唐雪松,陈旻炜,高常辉
(长沙理工大学土木与建筑学院力学系,长沙410114)
摘要:以恒定速度运动的Griffith裂纹解析解为著名的Yoffe解。静止裂纹的条状屈服模型即Dugdale模型,将其推广到运动裂纹模型时发现,当裂纹运动速度跨越Rayliegh波速时,裂纹张开位移COD趋于∞,且表现为间断。通过在裂尖引入一个约束应力区及两个速度效应函数,假设约束应力为线性分布,采用复变函数方法,求得动态应力强度因子SIF与裂纹张开位移COD的解析解。新的结果,在Rayleigh波速下裂纹张开位移连续且为有限值。给出裂纹张开位移的一些数值结果,获得了一些有意义的结论。
关键词:运动裂纹;Ⅰ型裂纹;约束应力;复变函数方法;应力强度因子SIF;裂纹张开位移COD
基金项目:973项目资助(2015CB057705);国家自然科学基金资助(51378081)
收稿日期:2013-09-30修改稿收到日期:2014-02-14
中图分类号:O346.1文献标志码:A
A model of moving crack with a linear distribution of restraining stresses in crack tip zone
TANGXue-song,CHENMin-wei,GAOChang-hui(Department of Mechanics, School of Civil Engineering and Architecture, Changsha University of Science and Technology, Changsha 410114, China)
Abstract:The analytical solution of moving Griffith crack model with a constant speed is well known as Yoffe solution. For a static crack, its strip yielding model is well known as Dugdale model. It is found that when Dugdale model is generalized to the moving crack case, the crack opening displacement (COD) is discontinuous and approaches to positive and negative infinite at Rayleigh wave speed. Here, a restraining stress zone was attached to the crack tip while two speed effect functions were introduced assuming the restraining stress zone has a linear distribution. The complex function approach was employed to solve the problem. Analytical solutions of dynamic stress intensity factor (SIF) and crack opening displacement (COD) were then obtained. The new COD result was continuous and finite at Rayleigh wave speed. Some numerical results of COD were presented. Some valuable conclusions were obtained.
Key words:moving crack; model I crack; restraining stress; complex function approach; stress intensity factor (SIF); crack opening displacement (COD)
关于运动裂纹的研究与求解,不但具有重要学术意义,还具有一定实用参考价值。若仅考察运动裂纹尖端,可一定程度反映动态裂纹扩展的一些力学行为。Yoffe[1]最早获得了以恒定速度运动的Griffith裂纹模型的解析解,即著名的Yoffe解。关于运动裂纹的研究仍然是当前国际上的热点研究问题。近期的一些研究成果包括:电磁弹性材料反平面剪切下运动裂纹问题的研究[2],压电双材料中含接触区的界面运动裂纹的研究[3],压电双材料含有限穿透裂纹沿界面运动问题的研究[4],电磁弹性矩形板中运动裂纹问题的研究[5],正交各向异性双材料中界面裂纹附近螺型运动位错的研究[6],微观、细观与宏观尺度下裂纹尺寸与速度相互影响的研究[7],宏观主裂纹运动速度接近剪切波速时微观裂尖钝化效应的研究[8]等。国内近期研究成果包括,关于宏微观双尺度运动裂纹解析求解的研究[9],对Ⅲ型运动裂纹均布载荷与集中载荷作用下的断裂动力学问题的研究[10],对黏结于均匀材料基底上功能梯度材料涂层平面运动裂纹问题的研究[11],对功能梯度压电板条中电绝缘型运动裂纹电弹性场的研究[12],对不同压电介质界面上的反平面运动裂纹的研究[13],等。
由于数学上的限制,对运动裂纹问题一般限制速度在Rayleigh波速以下。实际上,裂纹运动速度不但可达到Rayleigh波速,还可超过剪切波速[14]。本文在运动裂纹尖端引入一个约束应力区,约束应力大小、分布与裂尖材料的损伤程度和运动速度有关。约束应力区的概念由Sih等[15]提出,随后基于约束应力区建立了多种多尺度裂纹模型[15-19],还建立了宏微观跨尺度疲劳裂纹扩展统一模型[20]。本文主要工作是在运动裂纹尖端引入约束应力区后,裂纹张开位移在Rayleigh波速下实现了连续,且直到剪切波速都是连续的。
1约束应力区概念与分析模型
约束应力区概念如图1示,图1(a)为无损伤的单向受拉板。切开一个长度为a的切口,则切口存在约束应力,如图1(b)所示。图1(c)所示为一个长度为a的裂纹。若σ0=σ∞,则情况(b)等价于情况(a),表示材料无损伤。若σ0=0,则情况(b)等价于情况(c),表示材料完全损伤。若0<σ0<σ∞,表示材料有一定程度损伤而又没有完全分离,介于情况(a)与(c)之间。所以,可用约束应力区描述材料的损伤状态。

图1 约束应力区概念 Fig.1 Concept of restraining stress zone

图2 裂尖具有约束应力区的运动裂纹模型 Fig.2 Moving crack model with a restraining stress zone attached to the crack tip

ξ=x1-vt,η=x2
(1)
考虑到裂纹尖端材料有一定程度损伤,裂尖损伤区用一约束应力区来描述,约束应力区内约束应力的分布为R(χ),χ为局部位置坐标。分布函数R(χ)依赖于裂尖区材料的损伤及裂纹运动速度v,本文考虑线性分布的约束应力,即
R(χ)=f(v)(a1χ+a2),a≤χ≤c
(2)
2基本控制方程
不计休力,二维弹性动力学基本控制方程为Navier方程
(3)
式中:λ与μ为Lame常数,ρ为质量密度,ui为位移。引入体积变形势函数φ(x1,x2,t)和剪切变形势函数Ψ(x,y,t),有
(4)
则式(3)成为

(5)
(6)
采用式(1)的运动坐标系(ξ,η),式(5)成为
(7)
(8)
引入复变量ζd和ζs
ζd=ξ+iαdη,ζs=ξ+iαsη
(9)
及解析函数F(ζd)与G(ζs),两个位移势函数可表示
φ(ξ,η)=Re[F(ζd)],ψ(ξ,η)=Im[G(ζs)]
(10)
如此,位移与应力解答可表示为[17]
u2(ξ,η)=-Im[αdF′(ζd)+G′(ζs)]
(10)
σ11(ξ,η)=
(11)
σ22(ξ,η)=
(12)
σ12(ξ,η)=
(13)
因此,关键是由边界条件确定出两个解析函数。
3问题的求解
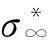
(14)
(15)
(16)
(17)
由式(15)知,D是速度v的函数,当v=cR时,D=0,由此条件可得出Rayleigh波速计算公式为
(18)
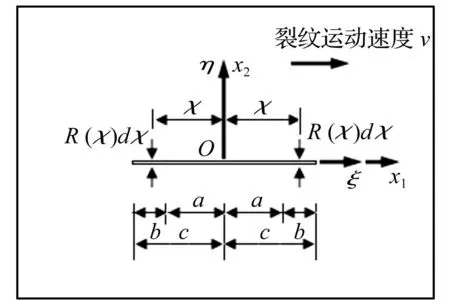
图3运动裂纹表面受两对对称集中力作用 Fig.3 Two pairs of concentrated forces symmetrically applied to the moving crack surfaces
下面考虑约束应力单独作用的结果。如图3示,对称位置上dχ上分布力的合力R(χ)dχ可看成是集中力。运动裂纹表面上两对对称集中力作用下,解析函数的解答为[7]
(19)
式(2)代入式(19)中,并从a到c积分,即可得出约束应力作用下的解答,有
(20)
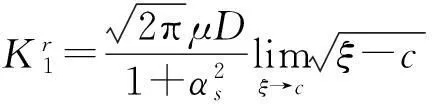
(21)
式(20)代入式(21)中,可求出
(22)
远场应力与约束应力共同作用的结果为
F″(ζd)=F″r(ζd)+F″σ(ζd)
(23)
(24)
(25)
式(16)、(22)代入式(25)中,得
(26)
注意:c=a+b,由式(26)可确定出裂尖损伤区尺寸b。
裂纹表面张开位移COD的定义为
(27)
式(10)、(14)、(20)、(23)、(24)代入式(27)中,求出
(28)
(29)
(30)
4数值结果与讨论
假设材料为铝合金LY12,材料参数见表1。
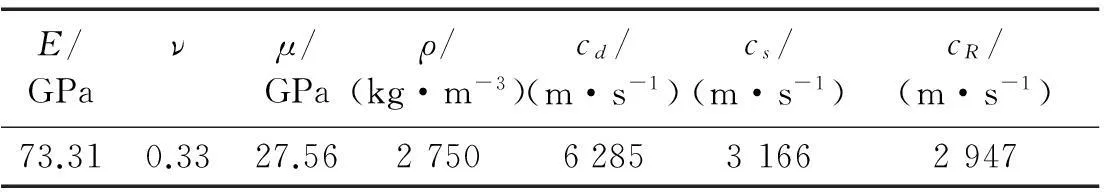
表1 铝合金LY12的材料参数
当取g(v)=f(v)=1,a1=0,a2=σ0时,此即运动裂纹Dugdale模型。取σ0=10 MPa,σ∞/σ0=0.4,ξ=0,图4给出裂纹中点张开位移随运动速度的变化曲线。由图4知,vcR时,COD趋于正无穷。v>cR时,COD为负值,且运动速度大于cR情况下逼近cR时,COD趋于负无穷。
为解决以上问题,引入了两个速度函数g(v)与f(v),参照文献[8],可取
(31)
考虑约束应力为三角形分布,取
(32)
即:χ=a时约束应力为0,χ=c时约束应力为σ0。由式(26)知,此时约束应力区(即损伤区)长度b与速度v无关,仅取决于比值σ∞/σ0,数值结果见表2,裂尖损伤区随作用荷载的增大而明显增大。
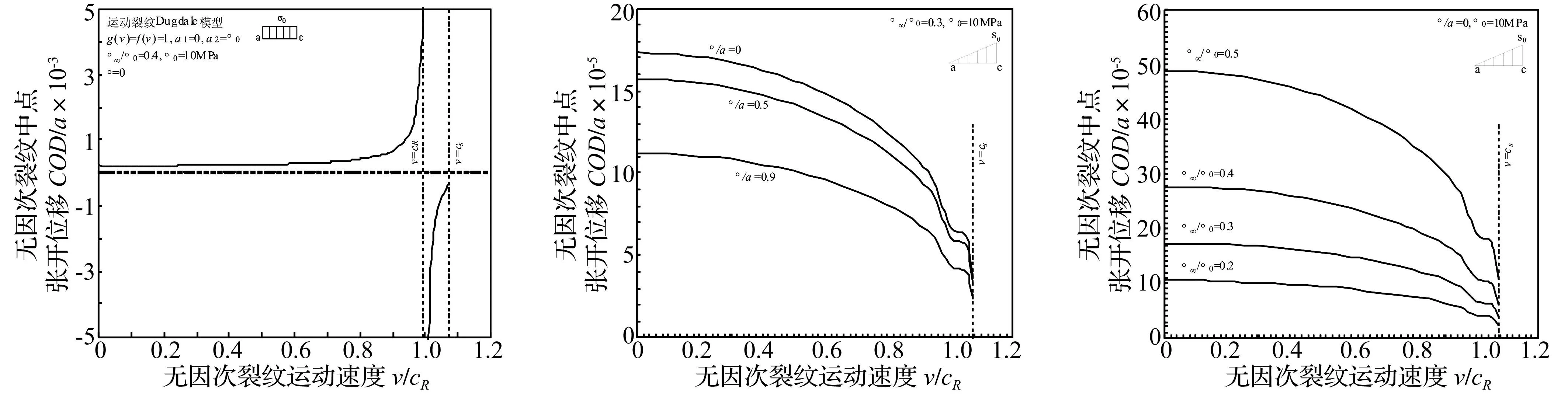

图4 裂纹中点张开位移随运动速度的变化曲线:运动裂纹Dugdale模型Fig.4Normalizedcrackopeningdisplacementatthemiddlepointversusnormalizedcrackmovingspeedcurves:movingcrackDugdalemodel图5 不同位置裂纹张开位移随运动速度的变化曲线Fig.5Normalizedcrackopeningdisplacementversusnormalizedcrackmovingspeedcurvesatdifferentlocation图6 不同应力下裂纹中点张开位移随运动速度的变化曲线Fig.6Normalizedcrackopeningdisplacementatthemiddlepointversusnormalizedcrackmovingspeedfordifferentstressratio
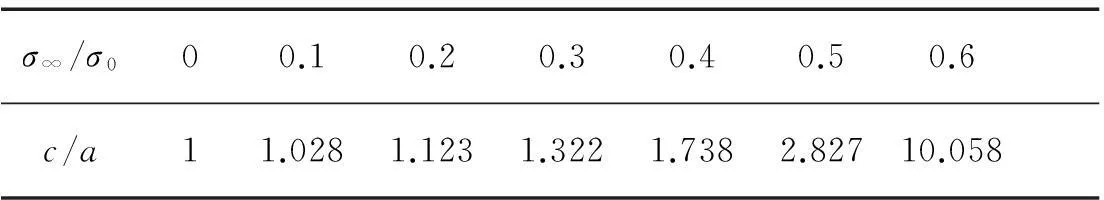
表2 长度比c/a随应力比σ ∞/σ 0的变化:σ 0=10 MPa

(33)
(34)
类似地:v=cR时,式(28)中的f(v)/D成为0/0,由罗必塔法则,得
(35)
可见,引入速度函数f(v)后,COD在v=cR时连续且为有限值。图5也证实,运动速度从0直到剪切波速cs,COD都是连续的,跨越Raylrigh波速时也是连续的。由图5知,裂纹张开位移随运动速度增大而减小。
不同作用应力下,裂纹中点张开位移随运动速度的变化曲线如图6示,随荷载的增大,张开位移随之明显增大。图7是不同速度下裂纹张开位移随横坐标位置的变化曲线。图8是不同应力下裂纹张开位移随横坐标位置的变化曲线,图中显示随作用应力的增加,裂纹张开位移及裂尖损伤区长度明显增大。

图7 不同速度下裂纹张开 位移沿横坐标的变化曲线 Fig.7 Normalized crack opening displacement versus normalized coordinate ξ/a for different crack moving speed

图8 不同应力下裂纹张开位移沿横坐标的变化曲线 Fig.8 Normalized crack opening displacement versus normalized coordinate ξ/a for different stress ratio
5结论
(1)研究运动裂纹问题,裂尖损伤区用约束应力区描述。设约束应力为线性分布,采用复变函数解法,获得了裂尖损伤区长度与裂纹表面张开位移的解析解。
(2)Dugdale运动裂纹模型,在Rayleigh波速下裂纹张开位移为正、负无穷大。通过引入两个速度效应函数,成功实现了裂纹运动速度从0、到跨越Rayleigh波速、直到剪切波速,裂纹张开位移的连续变化。
(3)数值计算结果表明,随作用应力的增大,裂尖损伤区长度及裂纹张开位移显著增大。随裂纹运动速度的增加,裂纹张开位移明显减小。
参考文献
[1]Yoffe E Y. The moving griffith crack [J]. Philosophical Magazine, 1951, 42(7): 739-750.
[2]Hu Ke-qiang, Li Guo-qiang.Constant moving crack in a magnetoelectroelastic material under anti-plane shear loading [J]. International Journal of Solids and Structures, 2005, 42(9-10): 2823-2835.
[3]Hermann K P, Komarov A V, Loboda V V.On a moving interface crack with a contact zone in a piezoelectric bimaterial [J]. International Journal of Solids and Structures, 2005, 42(16-17): 4555-4573.
[4]Lapusta Y, Komarov A, Labesse-Jied F, et al. Limited permeable crack moving along the interface of a piezoelectric bi-material [J]. European Journal of Mechanics-A/Solids, 2011, 30(5): 639-649.
[5]Hu Ke-Qiang, Kang Yi-Lan, Qin Qing-Hua. A moving crack in a rectangular magnetoelectroelastic body [J]. Engineering Fracture Mechanics, 2007, 74(5): 751-770.
[6]Xie C, Liu Y W. Cracking characteristics of a moving screw dislocation near an interfacial crack in two dissimilar orthotropic media [J]. Theoretical and Applied Fracture Mechanics, 2008, 50(3): 214-219.
[7]Sih G C, Jones R.Crack size and speed interaction characteristics at micro-, meso- and macro-scale [J]. Theoretical and Applied Fracture Mechanics, 2003, 39(2): 127-136.
[8]Tang X S, Sih G C. Kinetics of microcrack blunting ahead of macrocrack approaching shear wave speed [J]. Theoretical and Applied Fracture Mechanics, 2004, 42(2): 99-130.
[9]唐雪松. 宏微观双尺度运动裂纹模型面内拉伸下的解析解[J]. 振动与冲击, 2011, 30(3): 100-108.
TANG Xue-song.Analytical solution for macro/micro dual scale moving crack model under the in-plane tension[J]. Journal of Vibration and Shock, 2011, 30(3): 100-108.
[10]王云涛, 吕念春, 程靳, 等. Ⅲ型运动裂纹在两种边界条件下的位错分布函数[J]. 工程力学,2008,25(10): 117-121.
WANG Yun-tao, LÜ Nian-chun, CHENG Xin, et al. Dislocation distribution functions of mode Ⅲ moving crack under two boundary conditions[J]. Engineering Mechanics, 2008,25(10): 117-121.
[11]程站起, 华利民, 卫兴. 功能梯度材料涂层平面运动裂纹分析[J]. 力学季刊, 2008, 29(1): 78-84.
CHENG Zhan-qi, HUA Li-min, WEI Xing.Moving crack analysis in a functionally graded coating under plane deformation[J]. Chinese Quarterly of Mechanics, 2008, 29(1): 78-84.
[12]胡克强, 仲政, 金波. 功能梯度压电板条中电绝缘型运动裂纹的电弹性场[J]. 力学季刊, 2003, 24(3): 371-378.
HU Ke-qiang, ZHONG Zheng, JIN Bo. Electroelastic field for an impermeable moving crack in a functionally graded piezoelectric strip[J].Chinese Quarterly of Mechanics, 2003, 24(3): 371-378.
[13]陈增涛, 余寿文. 不同压电介质界面上的反平面运动裂纹[J]. 固体力学学报, 1999, 20(1): 77-81.
CHEN Zeng-tao, YU Shou-wen.Anti-plane crack moving along the interface dissimilar piezoelectric materials[J]. Chinese Journal of Solid Mechanics, 1999, 20(1): 77-81.
[14]Rosakis A J, Samudrala O, Coker D. Cracksfaster than the shear wave speed [J]. Science, 1999, 284(5418): 1337-1340.
[15]Sih G C, Tang X S. Dual scaling damage model associated with weak singularity for macro-scopic crack possessing a micro-/meso-scopic notch tip [J]. Theoretical and applied fracture mechanics, 2004, 42(1): 1-24.
[16]Tang X S, Sih G C. Weak and strong singularities reflecting multiscale damage: micro-boundary conditions for free-free, fixed-fixed and free-fixed constraints [J]. Theoretical and Applied Fracture Mechanics, 2005, 43(1): 5-62.
[17]Tang X S, Sih G C. Edge dislocations generated from a microcrack under initial residual stress of non-uniform distribution [J]. Theoretical and Applied Fracture Mechanics, 2005, 44(3): 208-233.
[18]Sih G C, Tang X S.Triple scale segmentation of non-equilibrium system simulated by macro-micro-atomic line model with mesoscopic transitions [J]. Theoretical and Applied Fracture Mechanics, 2005, 44(2): 116-145.
[19]Tang X S, Sih G C.Equilibrium mechanics model of multiscaling by segmentation: asymptotic solution for macro-meso-micro damage in anti-plane shear deformation [J]. Theoretical and Applied Fracture Mechanics, 2005, 44(1): 1-15.
[20]唐雪松, 赵小鹏. 疲劳裂纹扩展行为的跨尺度分析方法[J]. 工程力学, 2012, 29(10): 20-26.
TANG Xue-song, ZHAO Xiao-peng.Multiscaling analysis approach of fatigue crack growth behavior [J]. Engineering Mechanics, 2012, 29(10); 20-26.
[21]Freund L B. Dynamic Fracture Mechanics[M]. London: Cambridge University Press, 1990. E Y. The moving griffith crack [J]. Philosophical Magazine, 1951, 42(7): 739-750.
[2]Hu Ke-qiang, Li Guo-qiang.Constant moving crack in a magnetoelectroelastic material under anti-plane shear loading [J]. International Journal of Solids and Structures, 2005, 42(9-10): 2823-2835.
[3]Hermann K P, Komarov A V, Loboda V V.On a moving interface crack with a contact zone in a piezoelectric bimaterial [J]. International Journal of Solids and Structures, 2005, 42(16-17): 4555-4573.
[4]Lapusta Y, Komarov A, Labesse-Jied F, et al. Limited permeable crack moving along the interface of a piezoelectric bi-material [J]. European Journal of Mechanics-A/Solids, 2011, 30(5): 639-649.
[5]Hu Ke-Qiang, Kang Yi-Lan, Qin Qing-Hua. A moving crack in a rectangular magnetoelectroelastic body [J]. Engineering Fracture Mechanics, 2007, 74(5): 751-770.
[6]Xie C, Liu Y W. Cracking characteristics of a moving screw dislocation near an interfacial crack in two dissimilar orthotropic media [J]. Theoretical and Applied Fracture Mechanics, 2008, 50(3): 214-219.
[7]Sih G C, Jones R.Crack size and speed interaction characteristics at micro-, meso- and macro-scale [J]. Theoretical and Applied Fracture Mechanics, 2003, 39(2): 127-136.
[8]Tang X S, Sih G C. Kinetics of microcrack blunting ahead of macrocrack approaching shear wave speed [J]. Theoretical and Applied Fracture Mechanics, 2004, 42(2): 99-130.
[9]唐雪松. 宏微观双尺度运动裂纹模型面内拉伸下的解析解[J]. 振动与冲击, 2011, 30(3): 100-108.
TANG Xue-song.Analytical solution for macro/micro dual scale moving crack model under the in-plane tension[J]. Journal of Vibration and Shock, 2011, 30(3): 100-108.
[10]王云涛, 吕念春, 程靳, 等. Ⅲ型运动裂纹在两种边界条件下的位错分布函数[J]. 工程力学,2008,25(10): 117-121.
WANG Yun-tao, LÜ Nian-chun, CHENG Xin, et al. Dislocation distribution functions of mode Ⅲ moving crack under two boundary conditions[J]. Engineering Mechanics, 2008,25(10): 117-121.
[11]程站起, 华利民, 卫兴. 功能梯度材料涂层平面运动裂纹分析[J]. 力学季刊, 2008, 29(1): 78-84.
CHENG Zhan-qi, HUA Li-min, WEI Xing.Moving crack analysis in a functionally graded coating under plane deformation[J]. Chinese Quarterly of Mechanics, 2008, 29(1): 78-84.
[12]胡克强, 仲政, 金波. 功能梯度压电板条中电绝缘型运动裂纹的电弹性场[J]. 力学季刊, 2003, 24(3): 371-378.
HU Ke-qiang, ZHONG Zheng, JIN Bo. Electroelastic field for an impermeable moving crack in a functionally graded piezoelectric strip[J].Chinese Quarterly of Mechanics, 2003, 24(3): 371-378.
[13]陈增涛, 余寿文. 不同压电介质界面上的反平面运动裂纹[J]. 固体力学学报, 1999, 20(1): 77-81.
CHEN Zeng-tao, YU Shou-wen.Anti-plane crack moving along the interface dissimilar piezoelectric materials[J]. Chinese Journal of Solid Mechanics, 1999, 20(1): 77-81.
[14]Rosakis A J, Samudrala O, Coker D. Cracksfaster than the shear wave speed [J]. Science, 1999, 284(5418): 1337-1340.
[15]Sih G C, Tang X S. Dual scaling damage model associated with weak singularity for macro-scopic crack possessing a micro-/meso-scopic notch tip [J]. Theoretical and applied fracture mechanics, 2004, 42(1): 1-24.
[16]Tang X S, Sih G C. Weak and strong singularities reflecting multiscale damage: micro-boundary conditions for free-free, fixed-fixed and free-fixed constraints [J]. Theoretical and Applied Fracture Mechanics, 2005, 43(1): 5-62.
[17]Tang X S, Sih G C. Edge dislocations generated from a microcrack under initial residual stress of non-uniform distribution [J]. Theoretical and Applied Fracture Mechanics, 2005, 44(3): 208-233.
[18]Sih G C, Tang X S.Triple scale segmentation of non-equilibrium system simulated by macro-micro-atomic line model with mesoscopic transitions [J]. Theoretical and Applied Fracture Mechanics, 2005, 44(2): 116-145.
[19]Tang X S, Sih G C.Equilibrium mechanics model of multiscaling by segmentation: asymptotic solution for macro-meso-micro damage in anti-plane shear deformation [J]. Theoretical and Applied Fracture Mechanics, 2005, 44(1): 1-15.
[20]唐雪松, 赵小鹏. 疲劳裂纹扩展行为的跨尺度分析方法[J]. 工程力学, 2012, 29(10): 20-26.
TANG Xue-song, ZHAO Xiao-peng.Multiscaling analysis approach of fatigue crack growth behavior [J]. Engineering Mechanics, 2012, 29(10); 20-26.
[21]Freund L B. Dynamic Fracture Mechanics[M]. London: Cambridge University Press, 1990.

