关于NA随机序列的一类强极限定理
屈红红 范爱华
(安徽工业大学数理科学与工程学院,安徽 马鞍山 243002)
关于NA随机序列的一类强极限定理
屈红红 范爱华
(安徽工业大学数理科学与工程学院,安徽 马鞍山 243002)
主要考虑同分布的NA随机序列(ξ,ξn)n∈N,满足或。通过把划分成四部分,得到一类强极限定理。
NA随机序列;三级数定理;大数定律
1 引言
设(ξ,ξn)n∈N是i.i.d随机变量,满足Eξ=0或。众所周知,对于任意一个常量(bn)n∈N,不成立[1-2](cf.Chowet al.(1961),Maller(1978),Adler(1989).
近来Andre Adler(2001,2002)考虑了以下强大定律的形式:

这里的(an,bn)n∈N是常量。这些强大定律被看作精确强大数定律。因为Andre Adler(2001,2002)在文中指出,存在一个几乎肯定非零极限,即使随机变量为零,或不存在[3-4]。
对于NA随机序列来说,通过Andre Adler(2001,2002),在本文中我们考虑了NA随机变量,并建立了一类极限定理。
定义1 如果对于{1,2,…,n }不相交子集A和B的每部分,随机变量的一有限集合ξ1,ξ2,…,ξn被认为是NA,如果

当f和g是逐渐增加且导致协方差存在。如果每个有限子集合是NA,那么随机变量的一组有限集合{ξt,t∈T}是NA。
这个定义由Alam and Saxena(1981)提出且由Joag-Dev和Proschan(1983)[5-6]仔细研究。同样地Joag-Dev和Proschan(1983)[8-9]指出并证明,许多著名的多变量的分布过程NA性质,比如(a)多项式,(b)不同多项式的卷积,(c)多元的超几何,(d)Dirichlet,(e)Dirichlet复合多项式,(f)负相关的正态分布,(g)排列分布,(h)不重复的随机抽样,并且(i)排列的联合分布。由于它在多元统计分析和可靠性的广泛应用中,最近NA概念已经得到了相当大的关注。对于更多的信息我们参考Han-Ying Liang(2000)[7]并引用其中。
引理1[10](Su Chun et al.1998)设(ξn)n∈N是NA随机变量的一个序列。如果,对于某个d>0,因此
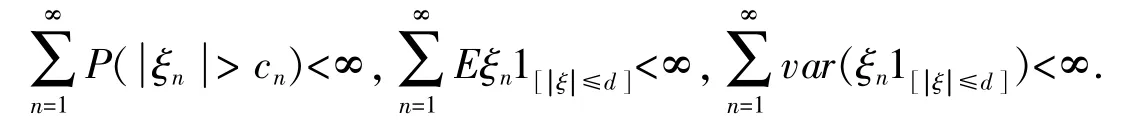
则

引理2[10](Su Chun et al.1998)设(ξn)n∈N是NA和fn不减少的Borel函数一列,然后(fn(ξn))n∈N也是NA。
2 主要的结果
我们首先考虑Eξ=0的情况。在这种情况下,我们首先给出下面的定义。
定义2 设ξ是一个随机变量有Eξ=0,我们称

作为缩短的一阶矩。
因为在Andrre Adler(2002),我们定义c作为对称性的参数,其中

定理1 设(ξ,ξn)n∈N是同分布的NA随机变量,有Eξ=0和,其中L(x)是缓慢的变化。设(an)n∈N和(bn)n∈N是那些如上定义的常量。如果

则有

其中b>0.


定理2 设ξ,(ξn)n∈N,是同分布的NA随机变量。有,这里L(x)是缓慢变化的,且(2.1)保留,然后


[1]Adler,A.Exact sequences for sums of independent random variables[J].Proceedings of theInternational Conference on Almost Everywhere Convergence in Probability and Ergodic Theory,Boston Academic Press∶11-29.
[2]Alam,K.&Saxena,K.M.L.Positive dependence in multivariate distributions[J].Comm.Statist.Theory Methods,1981,(A10)∶1183-1196.
[3]Andr′e Adler.Exact laws for multidimensionally indexed random variables[J].Journal of Multivariate Analysis.2001,(77)∶73-83.
[4]Andr′e Adler.Laws of large numbers for the maximal order statistics from a triangulararray[J].Bulletin of the Institute of Mathematics Academia Sinica,2002,(4)∶239-251.
[5]Block,H.W.&Savits,T.H.Some concepts of negative dependence[J].Ann.Probab.1982,(10):765-772.
[6]Chow,Y.S.&Robbins,H.On sums of independent random variables with infinitemoments and“fair”games[J].Proc.Nat.Acad.Sci.U.S.A.,1961,(47)∶330-335.
[7]Hanying,L.Complete convergence for weighted sums of negatively associated random variables[J].Statistics&Probability Letters.,2000,(48)∶317-325.
[8]Maller,R.A.Relative stability and the strong law of large numbers[J].Z.Wahrsch.verw.Gebiete,1978,(43)∶141-148.
[9]Joag-Dev,K.&Proschan,F.Negatively associated random variables with applications[J].Ann.Statist,1983,(11)∶286-295.
[10]苏淳,王岳宝.同分布NA序列的强收敛性[J].应用概率统计,1998,(2)∶131-140.
ON A CLASS OF STRONG LIMIT THEOREMS ON NEGATIVELY ASSOCIATED RANDOM VARIABLES
QU Hong-hong FAN Ai-hua
(School of Mathematics,Physics Science and Engineering,Anhui University of Technology,Ma'anshan Anhui 243002)
In this paper,the identically distributed NA random variables(ξ,ξn)n∈Nwhich are in accordance withorare taken into account.By dividinginto four parts,a class of strong limit theorems is obtained.
NA random variables;three-level mathematical theorem;law of large numbers
O211.4
A
1672-2868(2015)06-0010-04
责任编辑:陈 侃
2015-10-24
安徽工业大学研究生创新基金(项目编号:2015130)
屈红红(1983-),女,陕西咸阳人。安徽工业大学数理科学与工程学院,硕士研究生。研究方向:概率论与极限理论。
——卡文迪什测定万有引力常量

