具有分布时滞脉冲Cohen-Grossberg神经网络的稳定性分析

张 韬，苏亚坤，朱 进

(渤海大学 数理学院，辽宁锦州 121000)

Cohen-Grossberg神经网络是由Cohen和Crossberg于1983年提出的［1］，被广泛地应用于模式识别、记忆与信号处理、图象处理与计算技术等领域。然而，在实际应用中时滞、脉冲是不可避免的，且时滞、脉冲对神经网络的稳定性有着巨大的影响［2-8］，因此有关时滞脉冲Cohen-Crossberg神经网络的研究［9-19］已逐渐引起人们的关注，研究脉冲型时滞神经网络具有极其重要的意义。

1 问题描述及相关假设

神经网络模型如下:

初始条件x(t0+s)=φ(s)，0≤τij(t)＜τij(t)≤η＜1，其中:x(t)=(x1(t)，x2(t)，…，xn(t))表示神经元状态向量;ai(·)表示放大函数;bi(·)表示适当的行为函数;fj，hj为神经元的激励函数;C=(cij)n×n，D=(dij)n×n，W=(wij)n×n分别表示连接权矩阵、时滞连接权矩阵和分布时滞连接权矩阵。固定时刻tk满足t1＜t2＜t3＜…，且在 tk时刻，Δ x(t )Rn」表示在tk时刻的状态变化，对所有的k∈N，Ik(0)=0。

要求神经网络模型满足以下假设:

1)存在正常数 Lj，Hj，j=1，2，…，n 使得

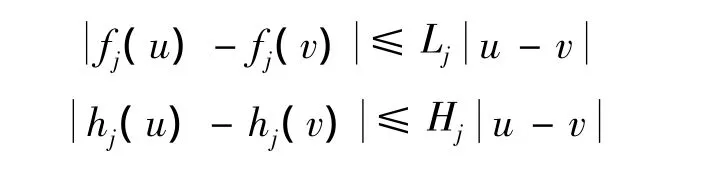
4)∃σk≥0，k∈N，有

5)∃μ ＞1，有 μτ≤inf{tk-tk-1};

6)max{ θk}≤M ＜ e2λμτ，M 是常数，θk=1+(2σk+);

7)延迟核函数 Kij，i，j=1，2…n是定义在［0，∞)上的实值非负函数，满足，其中λ是正常数。

2 主要结果

定理 在假设1)～7)下，如果∃λ＞0，正对角矩阵Q=diag(q1，…，qn)，使得

其中

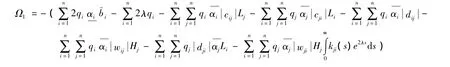
那么模型(1)的零解是全局指数稳定的。

证明 构造如下Lyapunov-Krasovskii泛函:

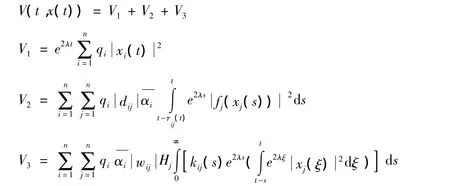
当 t≠tk时

利用条件1)～3)和2ab≤a2+b2得

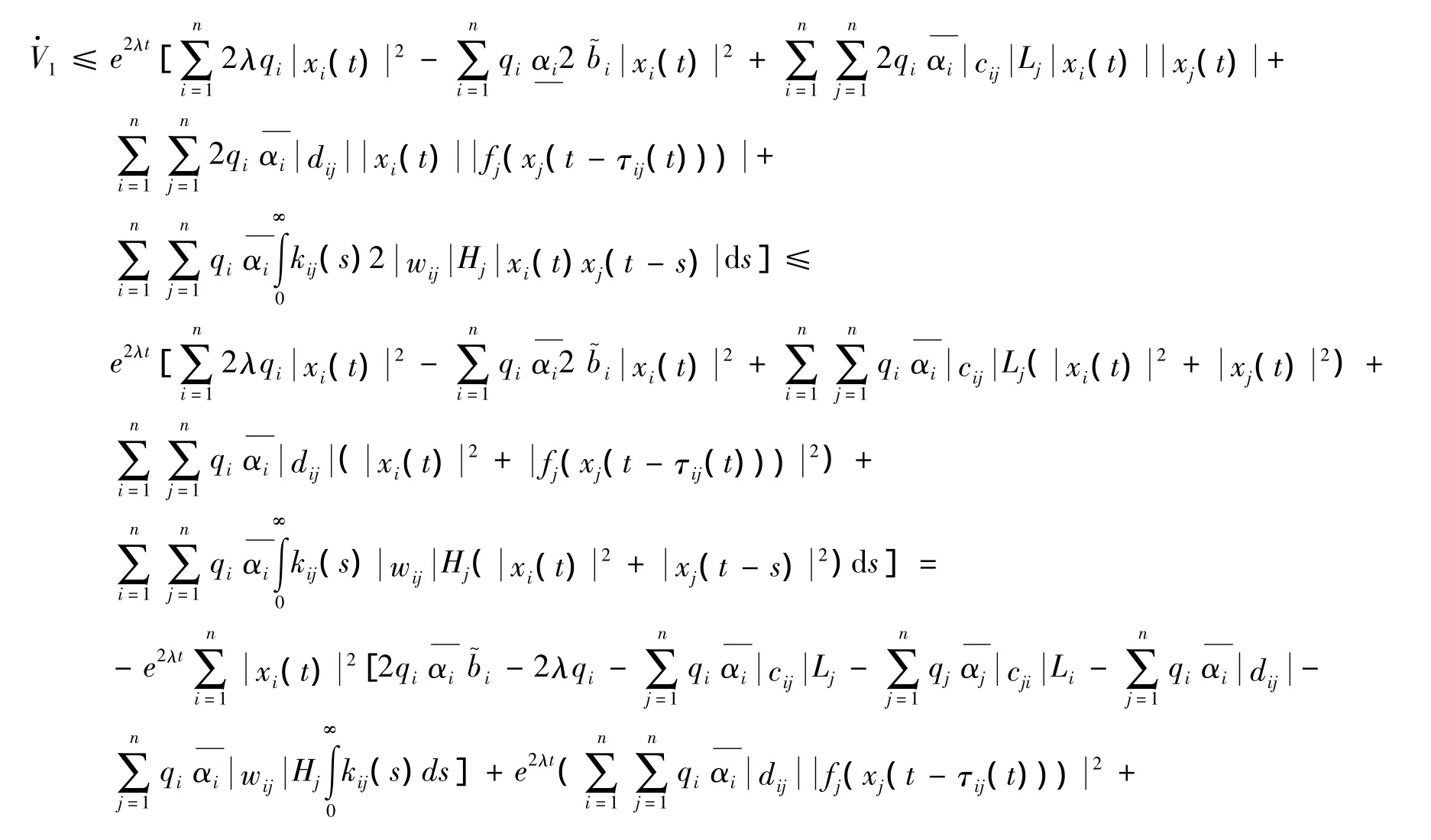
则

由V'＜0 知函数 V(t，x(t))是单调递减的，有 V(t，x(t))≤V(t0，x(t0))，

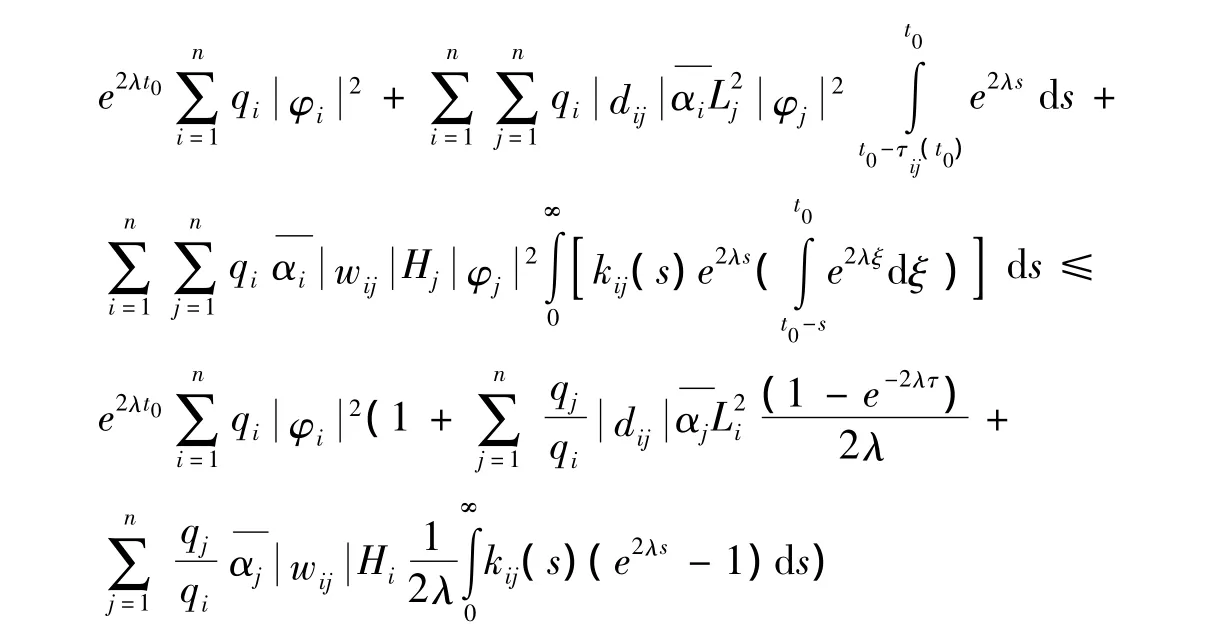
又因为

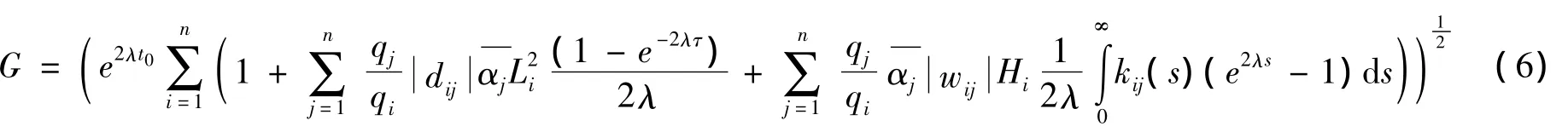
当t=tk时，根据假设4)～6)和指数稳定定义，有

由模型的任意解x(t，t0，x0)可得

由于μτ≤inf{tk-tk-1}，μτ≤t1-t0，μτ≤t2-t1，…，μτ≤tk-1-tk-2，求和得 (k-1)μτ≤t1-t0+t2-t1+…

3 数值算例

考虑下面的系统

其中 a1(x1(t))=3+sinx1(t)，a2(x2(t))=4+cosx1(t)。

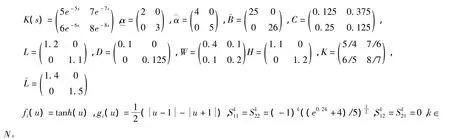
［1］Cohen M A，Grossberg S.Absolute stability of global pattern formation and parallel memory storage by competitive neural networks［J］.IEEE Trans Syst Man Cybern，1983，13(5):815-826.

［2］Liao X，Li C.Global attractivity of Cohen-Grossberg model with finite and infinite delays［J］.Math Anal Appl，2006，315(1):244-262.

［3］Huang T，Chan A，Huang Y，et al.Stability of Cohen-Grossberg neural networks with time varying delays［J］.Neural Networks，2007，20(8):868-873.

［4］Li T，Fei S.Stability analysis of Cohen-Grosserg neural networks with timevarying and distributed delays［J］.Neurocomputing，2008，71:1069-1081.

［5］Li K.Stability analysis for impulsive Cohen-Grossberg neural networks with time-varying delays and distributed delay［J］.Nonlinear Anal Real World Appl，2009，10(5):2784-2798.

［6］Zheng C D，Shan Q H，Wang Z.Novel stability criteria of Cohen-Grossberg neural networks with time-varying delays［J］.Int J Circuit Theory Appl，2012，40(3):221-235.

［7］Huang C X，Huang L H，He Y G.Mean square exponential stability of stochastic Cohen-Grossberg neural networks with unbounded distributed delays［J］.Discrete Dynamics in Nature and Society，2010(20):1-15.

［8］Wan L，Zhou Q.Exponential stability of stochastic reaction diffusion Cohen-Grossberg neural networks with delays［J］.Applied Mathematics and Computation，2008，206(2):818-824.

［9］Chen Y.Global Asymptotic Stability of Delayed Cohen-Grossberg Neural Networks［J］.IEEE Transactions on Circuit and Systems-I:Fundamental Theory and Applications，2006，53(2):351-357.

［10］Yang Z C，Xu D Y.Impulsive effects on stability of Cohen-Grossberg neural nettworks with variable delays［J］.Applied Mathematics and Cumputation，2006，177(1):63-78.

［11］Bai C Z.Stability analysis of Cohen-Grossberg BAM neural networks with delays and impulses［J］.Chaos，Solitons and Fractals，2008，35(2):263-267.

［12］Ping Z W，Lu J G.Global exponential stability of impulsive Cohen-Grossberg neural networks with continuously distributed delays［J］.Chaos，Solitons and Fractals，2009，41(1):164-174.

［13］Luo W P，Zhong S M，Yang J.Global exponential stability of impulsive Cohen-Grossberg neural networks with delays［J］.Chaos，Solitons and Fractals，2009，42(2):1084-1091.

［14］Li K L.Stability analysis for impulsive Cohen Grossberg neural networks with time varying delays and distributed delays［J］.Nonlinear Analysis:Real World Applications，2009，10(5):2784-2798.

［15］Lou X Y，Cui B T.Global exponential stability analysis of delayed Cohen-Grossberg neural networks with distributed delay［J］.International Journal of Systems Science，2007，38(7):601-609.

［16］Song Q K，Cao J D.Robust Stability in Cohen Grossberg Neural Network with both Time Varying and Distributed Delays［J］.Neural Process Lett，2008，27(2):179-196.

［17］Wang B X，Jian J G，Jiang M H.Stability in Lagrange sense for Cohen-Grossberg neural networks with time-varying delays and finite distributed delays［J］.Nonlinear AnaIysis:Hybrid Systems，2010，4(1):65-78.

［18］Wu W，Cui B T，Lou X Y.Global exponential stability of Cohen-Grossberg neural networks with distributed delays［J］.Mathematical and Computer Modelling，2008，47(9):868-873.

［19］Lu K，Xu D，Yang Z.Global attraction and stability for Cohen-Grossberg neural networks with delays［J］.Neural Networks，2006，19(10):1538-1549.