非自治二阶哈密顿系统周期解的存在性
石璐, 安天庆
(河海大学理学院,江苏南京210098)
非自治二阶哈密顿系统周期解的存在性
石璐, 安天庆*
(河海大学理学院,江苏南京210098)
用最小作用原理和临界点理论研究了一类非自治二阶哈密顿系统周期解的存在性问题。首先假设F(t,x)=F1(t,x)+F2(t,x)满足假设(A),再使F1满足次凸条件,并且在[0,T]上的积分趋于无穷,运用最小作用原理,得到一个解的新的存在性结果。另外,将F1在[0,T]上的积分趋于无穷这一条件减弱为F1在[0,T]的一个正测度子集E上的积分趋于无穷,运用最小作用原理,也能得到同样的结果。
次凸位势;周期解;临界点;最小作用原理
考虑非自治二阶哈密顿系统
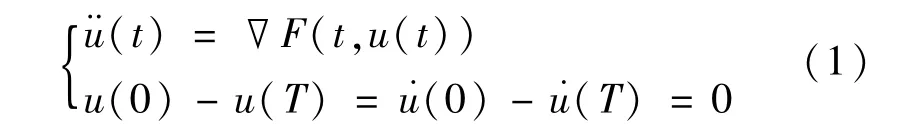
周期解的存在性问题。其中T>0,且F:[0,T]× RN→R满足下述假设:(A)对每个x∈RN,F(t,x)关于t可测,对a.e.t∈[0,T],F(t,x)关于x连续可微,且存在a∈C[R+,R+],b∈L1([0,T];R+),使得
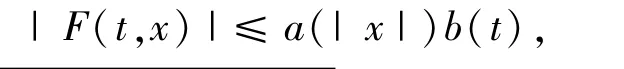
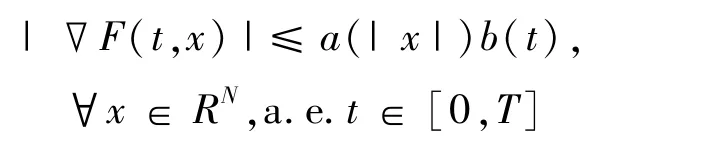
很多学者已经用最小作用原理证明了问题(1)至少有一个解,且为φ在上的最小值。文中受到文献[1-4]中结果与文献[5-7]中条件的激发,考虑了问题(1)中的次凸问题,同样通过运用最小作用原理,得到问题(1)的解的新结果。文中假设F(t,x)=F1(t,x)+F2(t,x)满足假设(A)。
1 主要结果
定理1 假设F1,F2满足假设(A),且


(2)∃r∈L1(0,T;R+),α∈[0,2),且
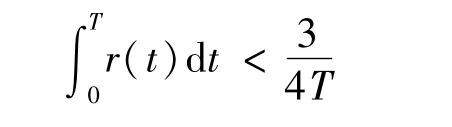
使得

注[2]若对于λ,μ>0,x,y∈RN,有
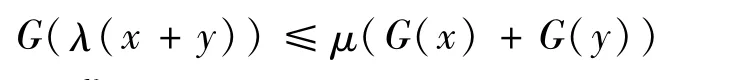
称函数F:RN→R为(λ,μ)次凸。
定理2 假设F1,F2满足假设(A),且
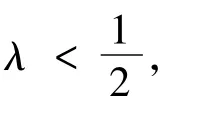
(2)∃r∈L1(0,T;R+),α∈[0,2),且
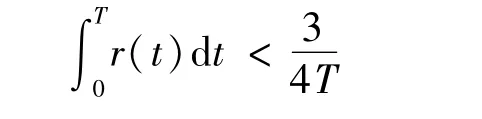
使得
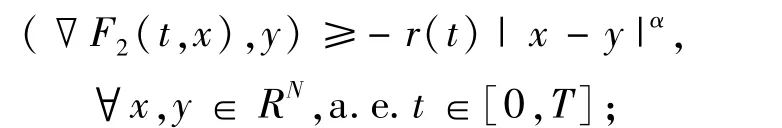
(3)∃E⊂[0,T],且meas(E)>0,使得
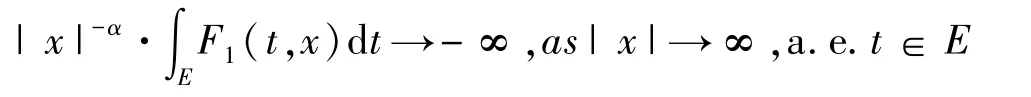
并且∃γ∈L1(0,T),使得
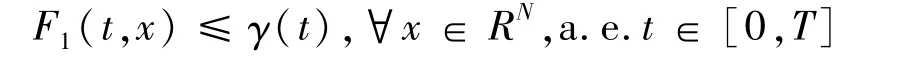
则问题(1)至少有一个解,且为φ在H1T(Ω)上的最小值。
2 定理的证明

从而有Sobolev不等式和Wirtinger不等式:
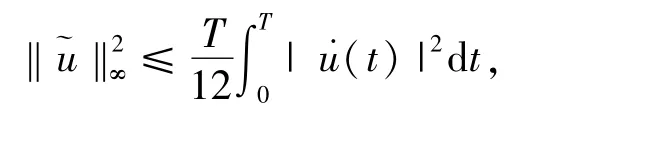
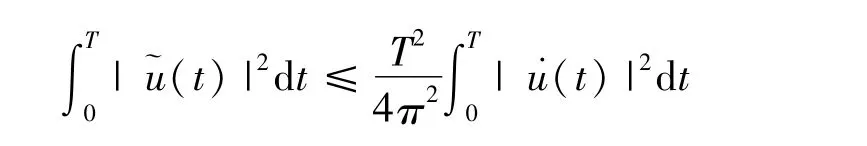
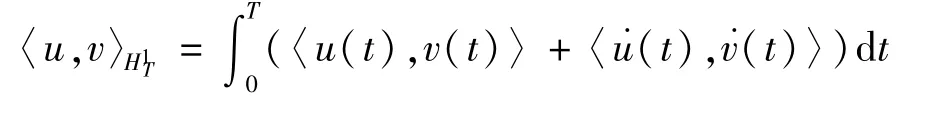
则对应的范数为
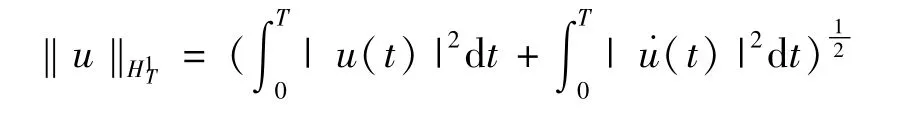
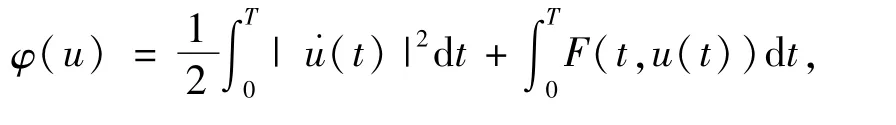

易知问题(1)的解即为φ的临界点。
由文献[1]可知,该泛函是下半弱连续的,因为强制的下半弱连续泛函必有最小值点,所以证明定理1和定理2时,只考虑泛函的强制性。
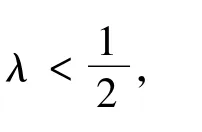
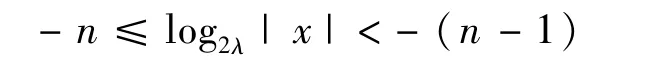
则由(2λ)-(n-1)<|x|≤(2λ)-n,有
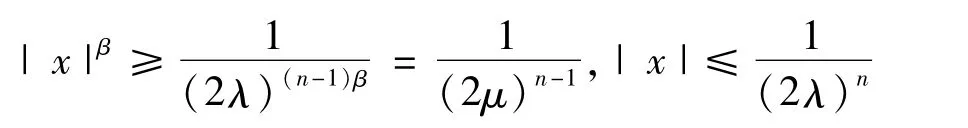
所以由条件(1)和假设(A),对于a.e.t∈[0,T]和所有的|x|≥1,有
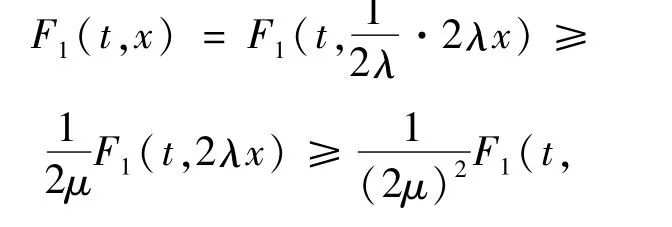
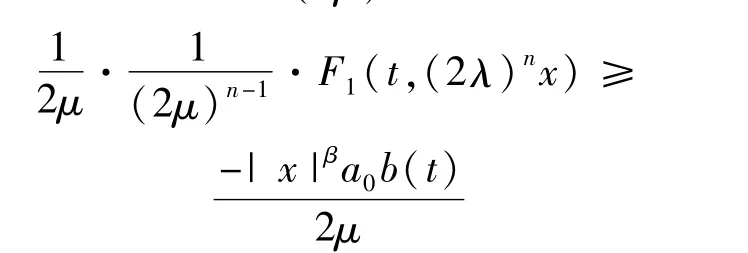
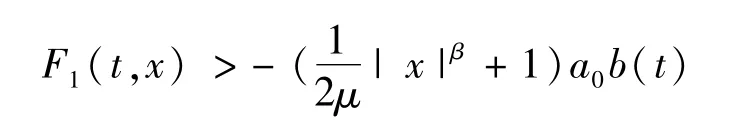
其中β<2。
又F1(t,·)是(λ,μ)次凸,则
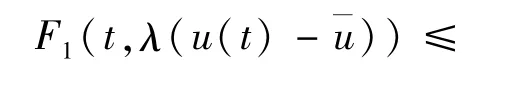
其中a0=max0≤s≤la(s)。
进一步,知道对于a.e.t∈[0,T]和所有的x∈RN,有
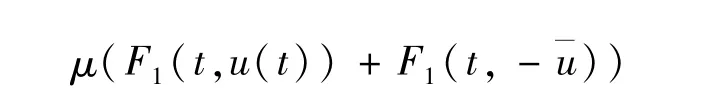
即
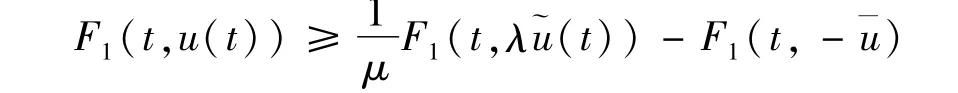
由条件(1)和Sobolev不等式,有

其中c1,c2均为正常数。
由条件(2),知道

所以


由于
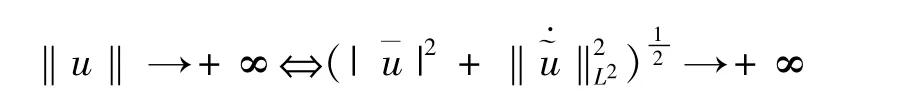
则由条件(3),β<2,α∈[0,2),知道

证毕。

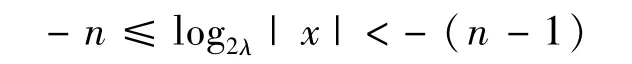
则由(2λ)-(n-1)<|x|≤(2λ)-n,有
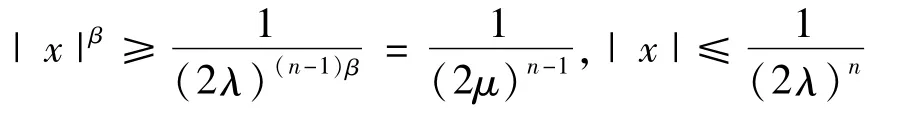
所以由条件(1)和假设(A),对于a.e.t∈[0,T]和所有的|x|≥1,有
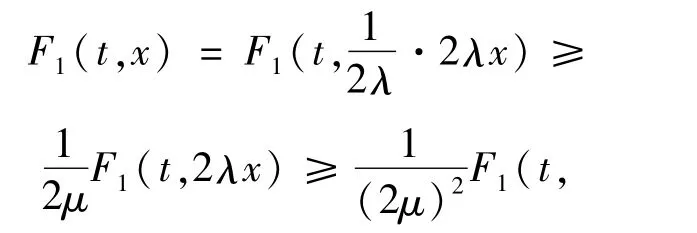
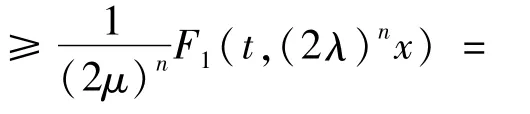

其中a0=max0≤s≤la(s)。
进一步,知道对于a.e.t∈[0,T]和所有的x∈RN,有
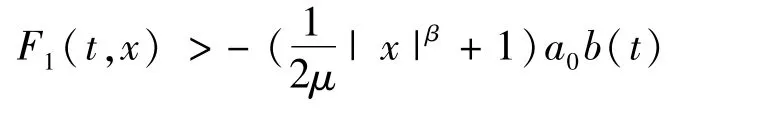
其中β<2。
又F1(t,·)是(λ,μ)次凸,则

即
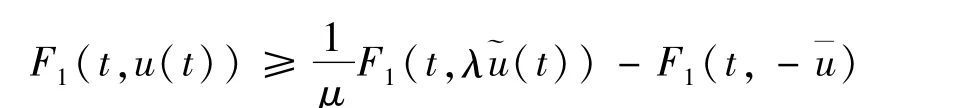
由条件(1)和Sobolev不等式,有
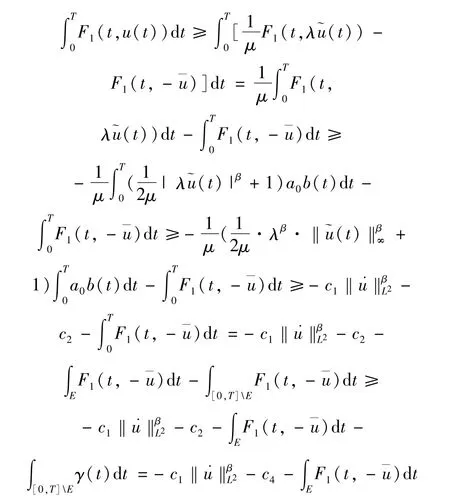
其中c1,c2,c4均为正常数。
由条件(2),知道
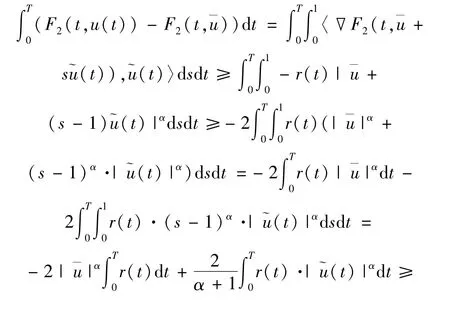
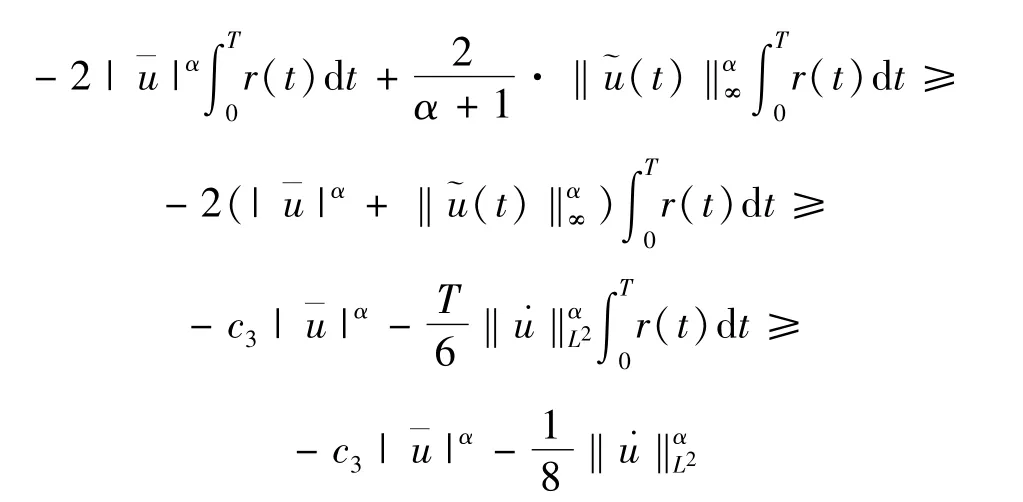
从而
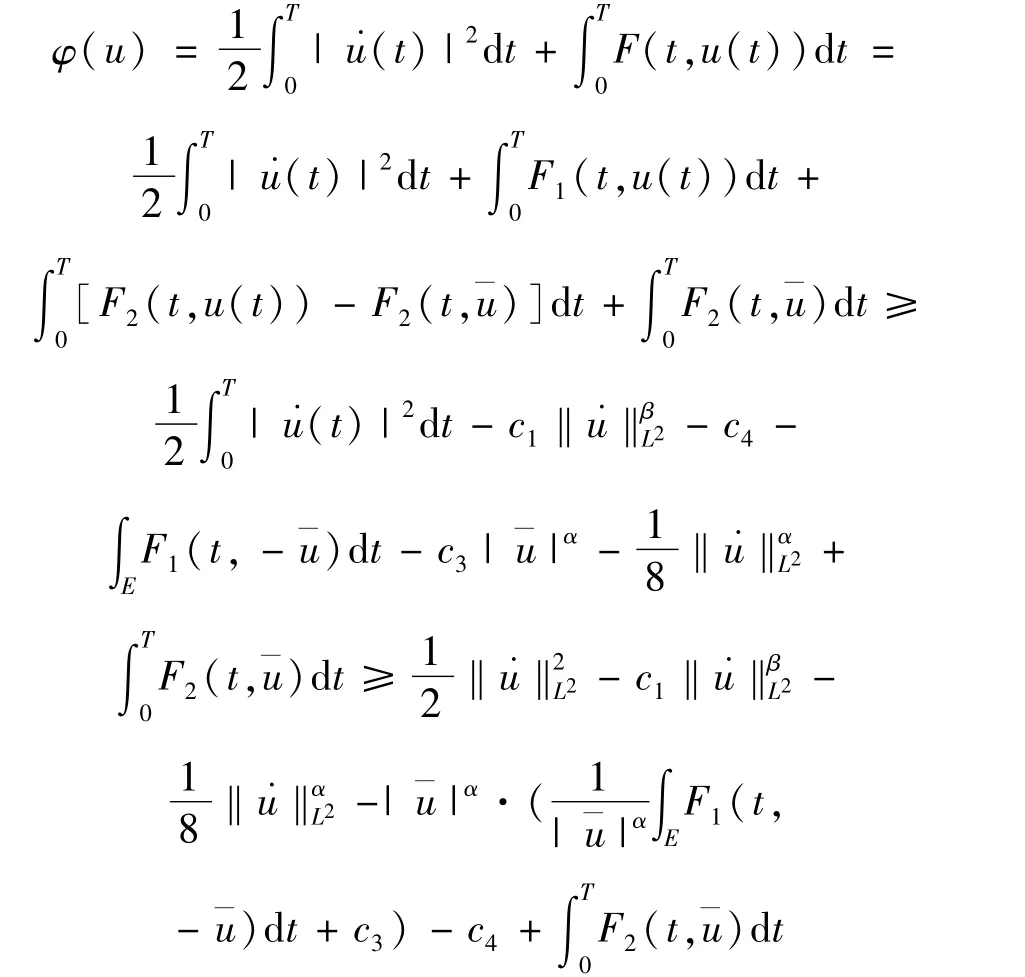
由于
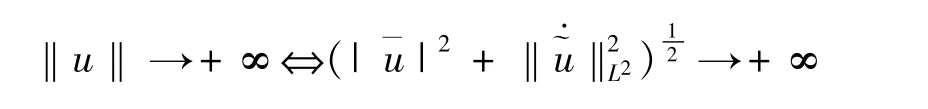
则由条件(3),β<2,α∈[0,2),知道
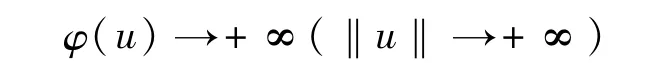
证毕。
[1]Mawhin J,Willem M.Critical Points Theory and Hamiltonian Systems[M].New York:Springer-Verlag,1989.
[2]WU Xingping,TANG Chunlei.Periodic solutions of a class of nonautonomous second order systems[J].JMath Anal Appl,1999, 236:227-235.
[3]孟凤娟.一类二阶哈密顿系统周期解的存在性[J].河南科学,2010,28(1):21-24.
MENG Fengjuan.Existence of periodic solutions for a class of second order Hamiltonian systems[J].Henan Science,2010,28 (1):21-24.(in Chinese)
[4]ZHAO Fukun,WU Xian.Periodic solutions of a class of nonautonomous second order systems[J].JMath Anal Appl,2004,296: 422-434.
[5]Nurbek Aizmahin,AN Tianqing.The existence of periodic solutions of nonautonomous second order Hamiltonian systems[J]. Nonlinear Analysis,2011,74:4862-4867.
[6]MA Jian,TANG Chunlei.Periodic solutions for some nonautonomous second order systems[J].JMath Anal Appl,2002,275:482-494.
[7]TANG Chunlei.Periodic solution of nonautonomous second order systems[J].JMath Anal Appl,1996,202:465-469.
(责任编辑:杨 勇)
Existence of Periodic Solutions for Nonautonom ous Second O rder Ham iltonian System
SHILu, AN Tianqing*
(College of Science,Hohai University,Nanjing 210098,China)
By using the least action principle and the critical point theory,this paper studies the existence of the periodic solutions for a class of non-autonomous second-order Hamiltonian systems.First,assuming that F(t,x)= F1(t,x)+F2(t,x)satisfies hypothesis(A),then F1meets the subconvex condition,and the integration tends to infinity in[0,T],we can obtain a new existence theorem by using the leastaction principle.Moreover,weweaken the condition that the integration of F1tends to infinity in[0,T]to the case that the integration of F1tends to infinity in a positive measure subset E of[0,T],we can get the same result by using the least action principle.
subconvex potential,periodic solutions,critical points,the least action principle
O 177
A
1671-7147(2015)01-0117-04
2014-09-12;
2014-09-25。
河海大学中央高校业务费项目(B12020128)。
石璐(1990—),女,江苏淮安人,基础数学专业硕士研究生。
*通信作者:安天庆(1963—),男,甘肃古浪人,教授,博士生导师。主要从事非线性泛函分析等研究。
Email:antq@hhu.edu.cn

