一类离散Lotka-Volterra系统持久性态的研究

曾晓云

(海军航空工程学院系统科学与数学研究所,山东 烟台 264001)

一类离散Lotka-Volterra系统持久性态的研究

曾晓云

(海军航空工程学院系统科学与数学研究所,山东 烟台 264001)

单时滞的Lotka-Volterra竞争系统的持久性,Saito等已经做了比较详尽的讨论,也得到了比较好的结论．但是Saito等只讨论了单时滞系统的情形,对于多时滞的较为复杂的系统并没有进一步讨论．本文将讨论多时滞的Lotka-Volterra竞争系统的持久性．持久性对于一个生态系统而言是一个非常重要的性质．在对系统做了一些合理的限定后,得到了关于该系统持久的一些充分性的结论．

周期性;离散的Lotka-Volterra系统;持久性

Saito等[1]考虑下列带有时滞的离散的Lotka-Volterra竞争系统

(1)

其中:

(2)

r1,r2,μ1,μ2是常数,满足r1>0,r2>0,μ1≥0,μ2≥0,k1,k2,l1,l2是非负整数．

最近,Zeng等[2]讨论了下列系统

(3)

其中:b(n),r(n),ai(n),cj(n),di(n),ej(n)都是正的周期为T的序列．

(4)

持久性对于一个生态系统而言是一个非常重要的性质．很多学者在这方面做了大量的研究,见文献[3-14].本文将讨论下列系统

(5)

其中:i=1,2,…,n;j=1,2,…,m.且

(6)

这里,x(k)表示被捕食种族在第k代的密度,y(k)表示捕食种族在第k代的密度,ai(k),ej(k)分别测度的是捕食与被捕食种族竞争行为的强度,b(k)表示的是被捕食种族内在生长率,r(k)表示的是捕食种族的死亡率．进一步假定:b(k),r(k)是有界正序列,ai(k),cj(k),di(k),ej(k)是有界非负序列,用{f(k)}表示任意有界序列,且

1 主要结果

为了证明本文的主要结果,引入以下引理．

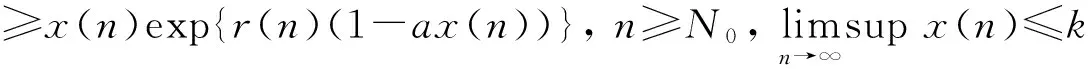
(7)

其中:

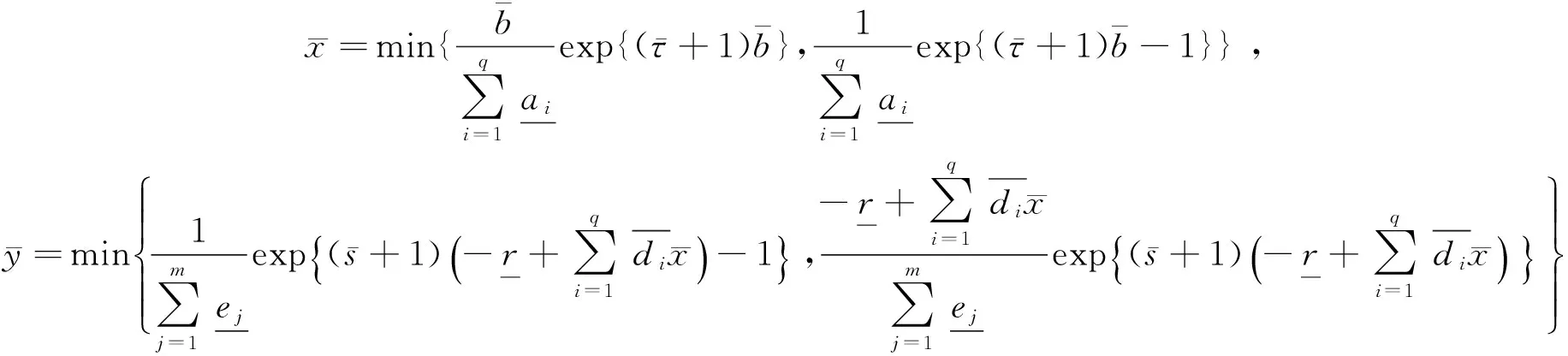
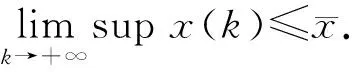
分2种情况讨论.

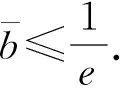
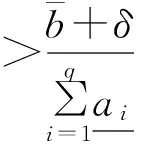
(8)

并且

(9)

(10)

成立．进一步,由式(5)和(10)可以得到:

这与式(9)矛盾．因而,有

(11)

因此,由式(5)和(11),得到

(12)

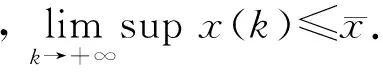
下面将证明

也分2种情况讨论:

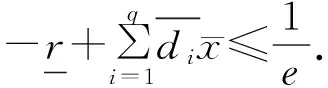
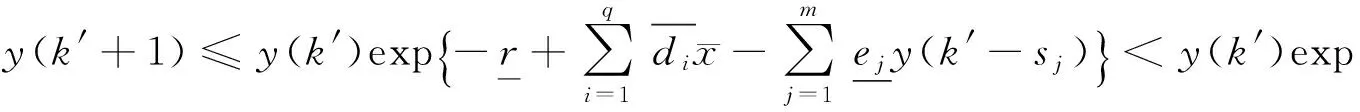
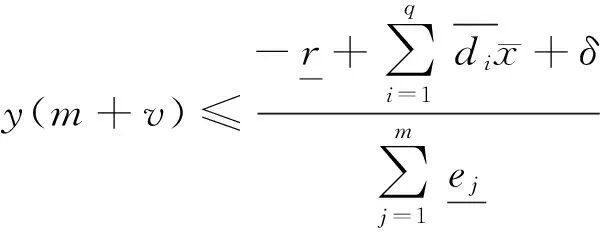
(13)

且

(14)

另一方面,由式(13)和(14),可以证得,当0≤k≤m时,有

(15)

进而,由式(5)和(15)可得

这与式(14)相矛盾．由此可以得到:

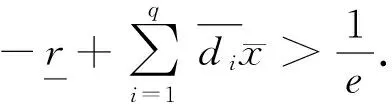
(16)

进而,由式(5)和(16)可以得到:

因而,有

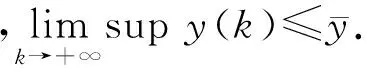
其中：

(17)

证明 由定理1知,对于充分大的K可以得到

(18)

由式(5)和(18)可以得到

即

或

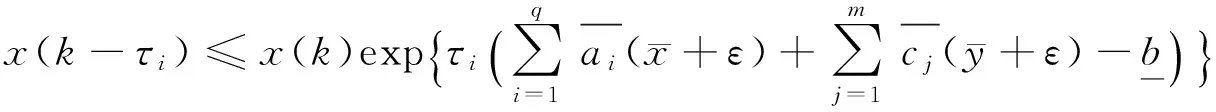
(19)

由式(19)与(5)可以得到，当k充分大时，有

由引理1及上述不等式可以得到:

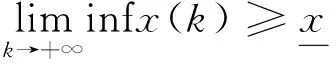
类似于上述证明过程,可以得到:

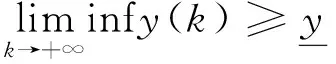
定理3 假定(H1),(H2)成立,则满足条件(6)的系统(5)是持久的．

[1] Saito Y, Ma W, Hara T. A necessary and sufficient condition for permanence of a Lotka-Volterra discrete system with delays[J].Math Anal Appl, 2001,256:162-174.

[2] Zeng Xiaoyun, Shi Bao, Gai Mingjiu. A discrete periodic Lotka-Volterra system with delays[J].Computers & Mathematics with Applications, 2004,47:491-500.

[3] Mackey M C, Glass L.Oscillation and chaos in physiological control system [J]. Science,1997, 197: 287-289.

[4] Kocic V L, Ladas G.Global Behavior of Nonlinear Difference Equations of Higher Order with Applications[M]. Dordrecht: Kluwer Academic Publishers,1993:35-125.

[5] Lakshmikantham V, Trigiante D.Theory of Difference Equations, Numerical Methods and Applications, Mathematics in Science and Engineering [M].Boston: Academic Press, 1988:57-225.

[6] Hutson V, Schmitt K. Permanence and the dynamics of biological systems[J]. Mathematical Biosciences,1992,111(1): 1-71.

[7] Freedman H I, Ruan S G.Uniform persistence in functional-differential equations[J].Journal of Differential Equations,1995,115(1):173-192.

[8] Muroya Y. Global attractivity for discrete models of nonautonomous logistic equations[J]. Computers & Mathematics with Applications,2007, 53(7):1059-1073.

[9] Takeuchi Y, Cui J, Miyazaki R, et al. Permanence of delayed population model with dispersal loss[J].Mathematical Biosciences,2006,201(1-2):143-156.

[10] Saito Y.Permanence and global stability for general Lotka-Volterra predator-prey systems with distributed delays[J].Nonlinear Anal,2001,47:6157-6168.

[11] Xu Rui.Persistence and global stability for a delayed nonautonomous predator-prey system without dominating instantaneous negative feedback, distributed delays[J].Math Anal Appl,2001,262:50-61.

[12] Cushing J M. Periodic time-dependent predator-prey system [J]. SIAM J Appl Math,1997,32:82-95.

[13] Saker S H, Agarwal S. Oscillation and global attractivity in a nonlinear delay periodic model of respiratory dynamics [J]. Comput Math Appl,2002, 44(5-6):623-632.

[14] Bainov D, Simeonov P.Impulsive Differential Equations: Periodic Solutions and Applications [M]. Harlow:Longman Scientific & Technical,1993:95-255.

[15] Yang Xitao.Uniform persistence and periodic solution for a discrete predator-prey system with delays[J].Math Anal Appl,2006, 316:161-177.

(责任编辑 李春梅)

Permanence for a Discrete Periodic Lotka-Volterra System with Delays

ZENG Xiao-yun

(Institute of Applied Mathematics, Naval Aeronautical Engineering Academy, Yantai 264001, China)

Saito et al investigate the permanence of a discrete periodic Lotka-Volterra competition predator-prey system with delay and get some good results, by focusing on the case of single delay. In this paper, we investigate a discrete periodic Lotka-Volterra competition predator-prey system with delays. The sufficient and realistic results are obtained for the permanence for the above system.

periodicity; discrete Lotka-Volterra system; permanence

1004-8820(2015)04-0239-07

10.13951/j.cnki.37-1213/n.2015.04.002

2015-02-10

曾晓云(1969- ),女,陕西礼泉人,副教授,硕士,研究方向:时滞微分与差分方程的稳定性理论.

O175

A