Cocycle Perturbation on Banach Algebras

(1.Department of Mathematics,Tianjin Polytechnic University,Tianjin,300160)

(2.Basic Department,Tianjin Vocational Institute,Tianjin,300410)

Cocycle Perturbation on Banach Algebras

SHI LUO-YI1AND WU YU-JING2

(1.Department of Mathematics,Tianjin Polytechnic University,Tianjin,300160)

(2.Basic Department,Tianjin Vocational Institute,Tianjin,300410)

Communicated by Ji You-qing

Let α be a fl ow on a Banach algebra B,and ta continuous function fromRinto the group of invertible elements of B such that usαs(ut)=us+t,s,t∈R. Then βt=Adut◦αt,t∈Ris also a fl ow on B,where Adut(B)for any B∈β is said to be a cocycle perturbation of α.We show that if α,β are two fl ows on a nest algebra(or quasi-triangular algebra),then β is a cocycle perturbation of α.And the fl ows on a nest algebra(or quasi-triangular algebra)are all uniformly continuous.

cocycle perturbation,inner perturbation,nest algebra,quasi-triangular algebra

1 Introduction

In the quantum mechanics of particle systems with an in fi nite number of degrees of freedom, an important problem is to study the di ff erential equation

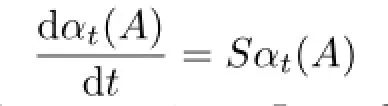
under variety of circumstances and assumptions.In each instance the A corresponds to an observable,or state,of the system and is represented by an element of some suitable Banach algebra B.S is an operator on B,and{αt}t∈Ris a group of bounded automorphisms on B.The function

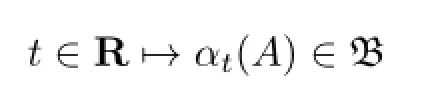
describes the motion of A.The dynamics are given by solutions of the di ff erential equation subject to certain supplementary conditions of continuity.Thus it is worth to study the group of bounded automorphisms on B.For more details see[1].

A fl ow α on B is a group homomorphism of the real line R into the group of bounded automorphisms on B(i.e.,tαt)such that

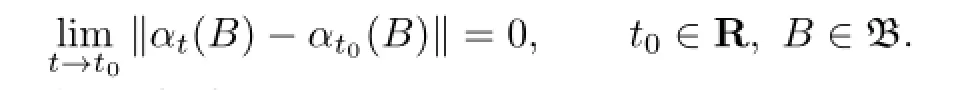
If there exists an h∈B such that

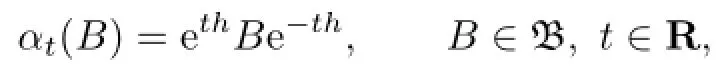
then we call α an inner fl ow.We say that a fl ow α is uniformly continuous if

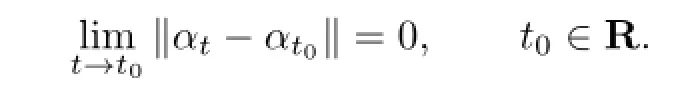
If α is a fl ow on B and if u is a continuous map of R into the group of invertible elements G(B)of B such that

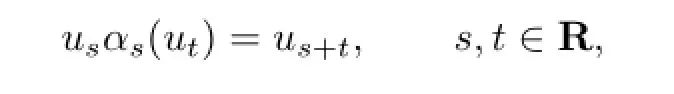
then we call u=(ut)t∈Ran α-cocycle for(B,R,α).Let

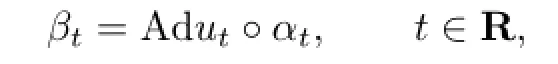
where

i.e.,

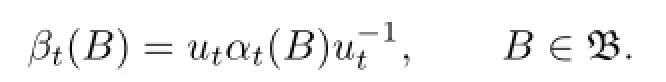
Then β is also a fl ow on B,and is said to be a cocycle perturbation of α.

If α is a fl ow on B,let D(δα)be composed of those B∈B for which there exists an A∈B with the property that

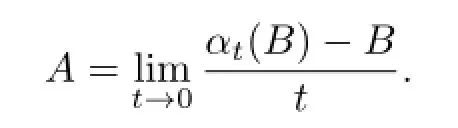
Then δαis a linear operator on D(δα)de fi ned by

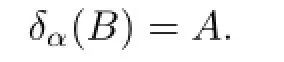
We call δαthe in fi nitesimal generator of α.By Proposition 3.1.6 of[1],δαis a closed derivation,i.e.,the domain D(δα)is a dense subalgebra of B and δαis closed as a linear operator on D(δα)and satis fi es

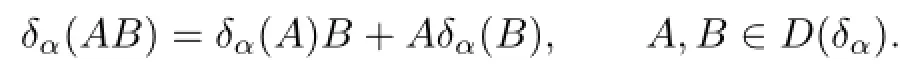
We call β an inner perturbation of α if α,β are two fl ows on B,

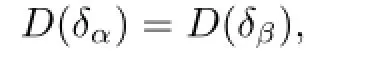
and there exists an h∈B such that

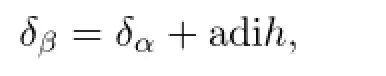
where i is the imaginary unit,and

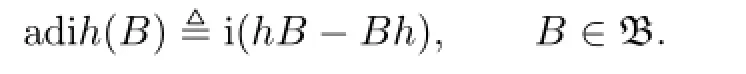
Moreover,

if and only if α is uniformly continuous.For more details see[1–2].

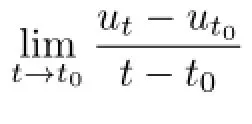
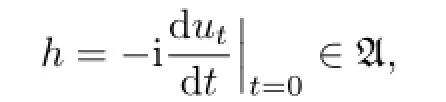
then the in fi nitesimal generator of the fl ow

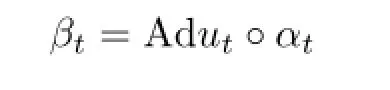
is given by

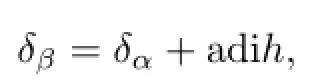
i.e.,β is an inner perturbation of α.Moreover,for any α-cocycle u=(ut)t∈R,there is a w∈G(A)and a di ff erentiable α-cocycle v=(vt)t∈R,i.e.,vtis an α-cocycle and di ff erentiable such that

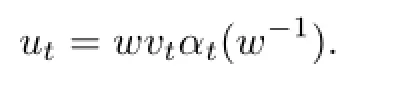
In Section 2,we consider the cocycle of fl ows on Banach algebras and obtain some similar results to[3].

It is well-known that a fl ow α on B may not be uniformly continuous even if B is a C∗-algebra(see[1–2]).In Section 3,we study the fl ows on a nest algebra τ(N)and the quasi-triangular algebra

(see[11]).We recall that a nest N is a chain of closed subspaces of a Hilbert space H containing{0}and H which is,in addition,closed under taking arbitrary intersections and closed spans.The nest algebra T(N)associated with N is the set of all T∈B(H)which leave each element of the nest invariant.For instance,if H is separable with orthonormal basisand Hn=span{e1,···,en},then exists for any t0∈R,and

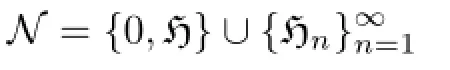
is a nest.In this case,T(N)is simply the set of all operators whose matrix representation with respect to this basis is upper triangular.It is obvious that τ(N)and Qτ(N)are typical Banach algebras.We obtain that all of the fl ows on τ(N)(or Qτ(N))are uniformly continuous.Moreover,all of the fl ows are cocycle perturbation to each other.

2 Cocycle Perturbations

Let B be a Banach algebra,α be a fl ow on B and u be an α-cocycle.Then

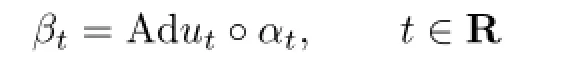
is a cocycle perturbation of α.In this section,we obtain that β is an inner perturbation of α if and only if u is di ff erentiable(see Theorem 2.1).Moreover,for any α-cocycle u,there is a di ff erentiable α-cocycle v and an inventible element w in B such that

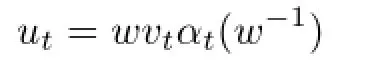
(see Theorem 2.2).

The following lemmas are useful for this paper.

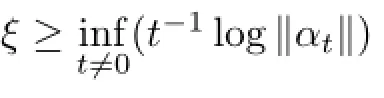
Lemma 2.2([1],Proposition 3.1.33)Letαbe a fl ow on a Banach algebraBwith in fi nitesimal generatorδα.For eachP∈Bde fi ne the bounded derivationδPby

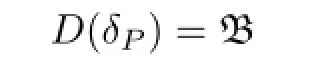
and

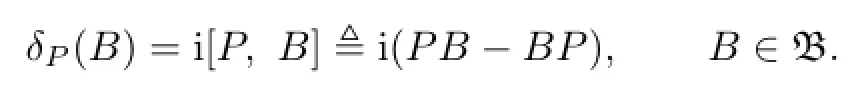
Thenδ+δPgenerates a fl ow onBgiven by

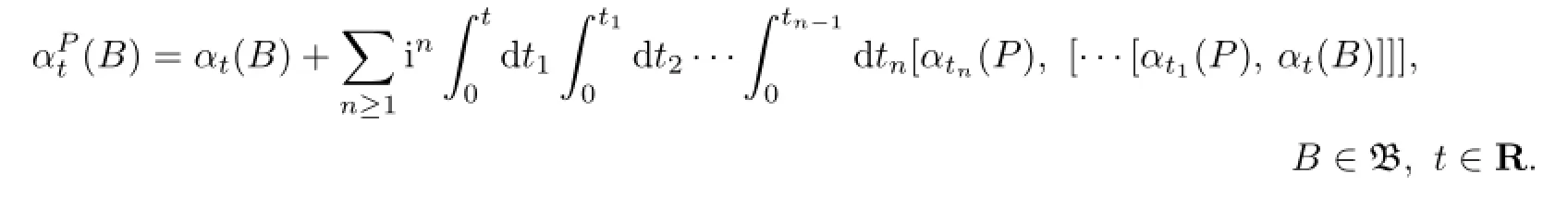
Lemma 2.3LetBbe a Banach algebra with unit1,αbe a fl ow onBandδdenote the in fi nitesimal generator ofα.Furthermore,for eachP∈B,de fi neδPas in Lemma2.2. Thenδ+δPgenerates a fl ow onBgiven by

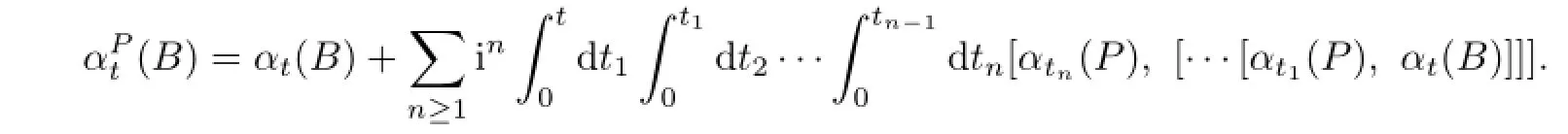
Moreover,

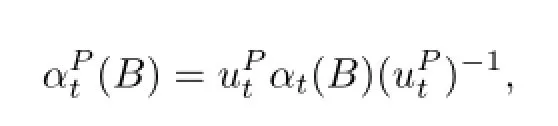
whereis a one-parameter family of invertible elements,determined by

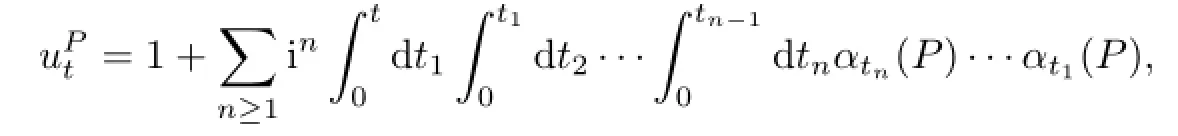
which satis fi es theα-cocycle relation

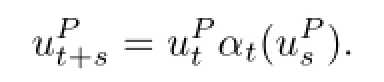
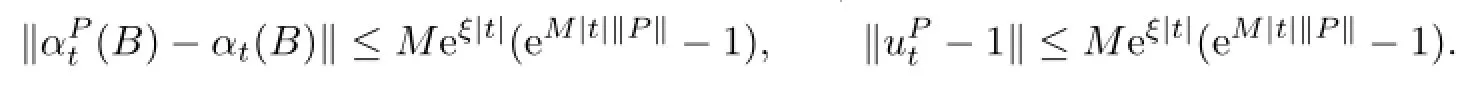
Proof.The fi rst statement of the lemma can be obtained from Lemma 2.2.We just give the proof of the last statement of this lemma.

We consider uPtde fi ned by the series.

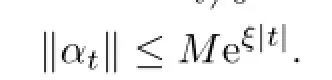
Let

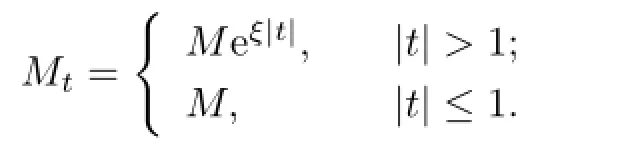
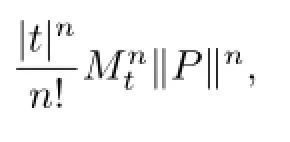
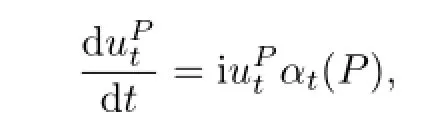
and

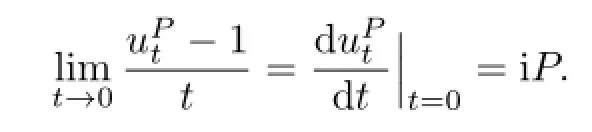
Hence,

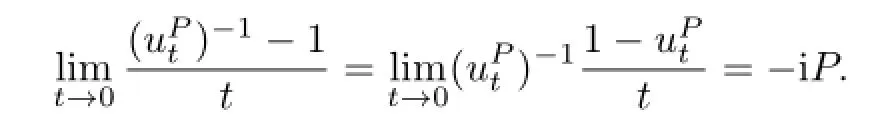
To establish the α-cocycle relation,we fi rst note that

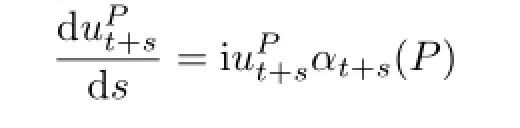
and

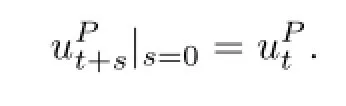
Since

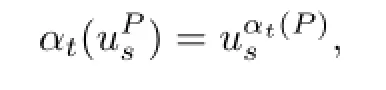
we can get

Moreover,

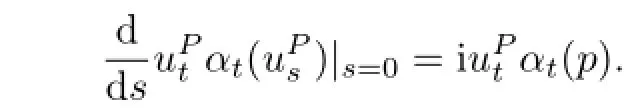
Hence,

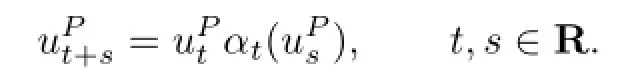
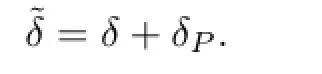
Choosing A∈D(δ+δP),one has

Similarly,if A∈D(˜δ),we obtain that

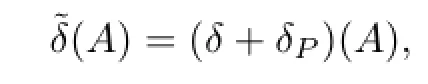
and then

Thus,by Theorem 3.1.26 in[1],one must have

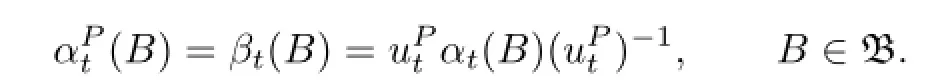
Theorem 2.1Letαbe a fl ow onB,(ut)t∈Rbe anα-cocycle,andβt=Adut◦αt.Thenβis an inner perturbation ofαif and only ifutis di ff erentiable.

Proof.Sufficiency.It follows immediately from Lemma 2.3.

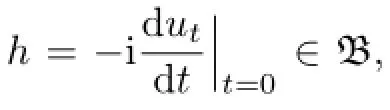
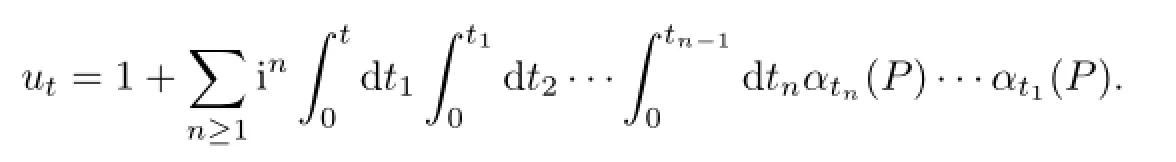
Hence,β is an inner perturbation of α by Lemma 2.3.

Corollary 2.1Adopt the assumptions of Lemma2.3and also assume thatαtis an inner fl ow,i.e.,there exists anh∈Bsuch that

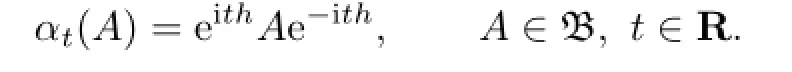
Then

whereis de fi ned as in Lemma2.3,and

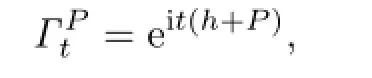
i.e.,is an inner fl ow.

Proof.If

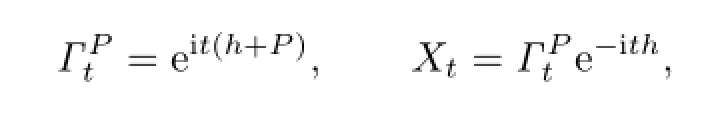
then

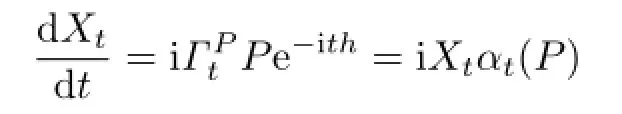
and X0=1.Thus,Xtis the unique solution of the integral equation

This solution can be obtained by iteration and one fi nds Xt=whereis de fi ned as in Lemma 2.3,and

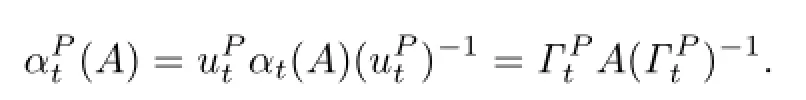
The proof is completed.

In the following we show that every α-cocycle is similar to a di ff erentiable α-cocycle.

De fi nition 2.1Letαbe a fl ow onB.A∈Bis called an analytic element forαif there exists an analytic functionf:C→Bsuch that

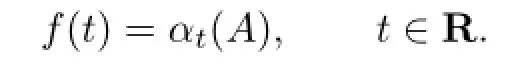
Lemma 2.4Letαbe a fl ow on the Banach algebraB,andM,ξbe constants such that

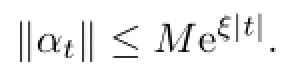
ForA∈B,de fi ne

Then eachAnis an entire analytic element forαt,and there exists anNsuch that

‖An‖≤2M‖A‖, n≥N,andAn→Ain the weak topology asn→∞.In particular,theαanalytic elements form a normal-dense subspace ofB.

Proof.Since

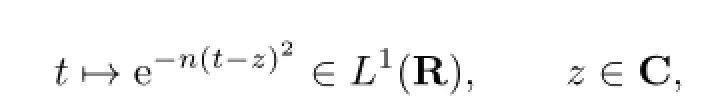
we know that

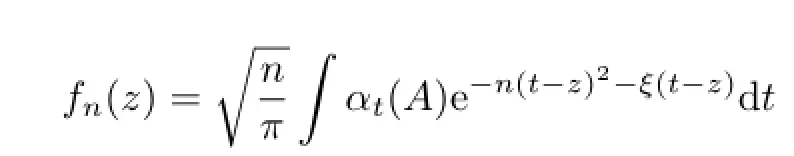
is well de fi ned for all z∈C.For z=s∈R,we have

But for η∈B∗we have

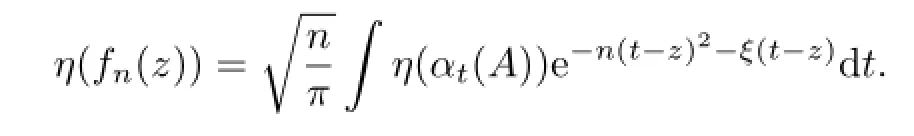
Since

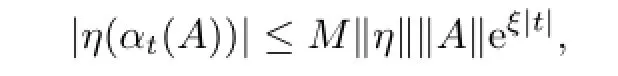
i

t follows from the Lebesgue dominated convergence theorem that tη(fn(z))is analytic.

Hence each Anis analytic for αt(A).Furthermore,we can derive the estimate

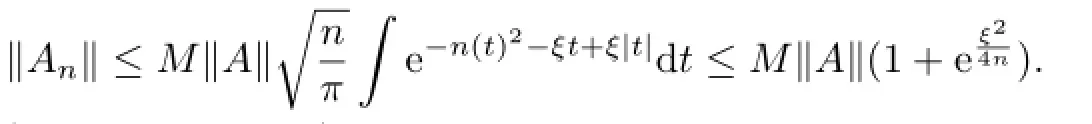
Hence,there exists an N such that

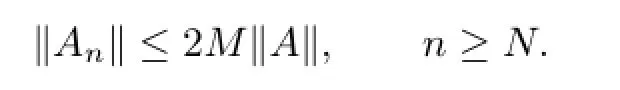
Noting that

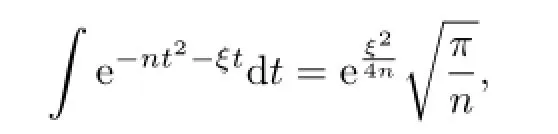
one has

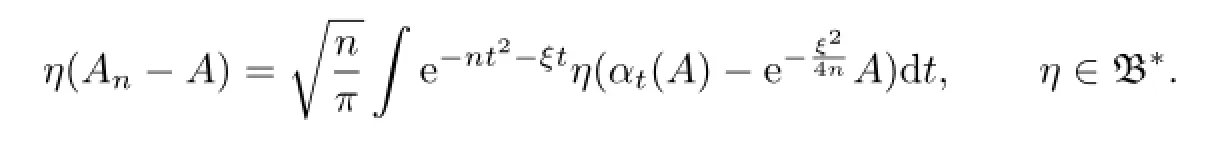
For any ε＞0 we may choose a δ＞0 such that|t|＜δ implies

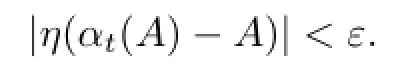
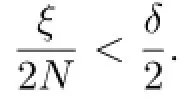
On the other hand,

√

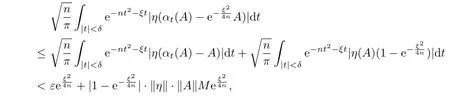
and

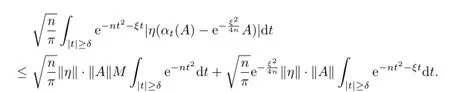
So,for any η∈B∗,

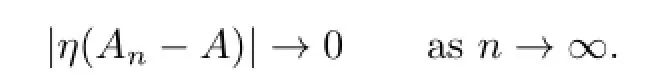
Finally,note that the norm closure and the weak closure of a convex set are the same,so the α analytic elements form a normal-dense subspace of B.

Theorem 2.2Ifuis anα-cocycle forB,then for a givenε＞0there exist a di ff erentiableα-cocyclevand aw∈G(B)such that

Proof.The proof is similar to that of Lemma 1.1 in[3]and is omitted.

For given two fl ows α and β on a unitary Banach algebra B,we say that β is a conjugate to α if there exists a bounded automorphism σ of B such that

Conjugate,cocycle perturbation and inner perturbation de fi ne three equivalence relations. We say that β is cocycle-conjugate to α if there exists a bounded automorphism σ of B such that β is a cocycle perturbation of σασ−1.This also de fi nes an equivalence relation among the fl ows.We say that α is approximately inner if there is a sequence{hn}in B such that

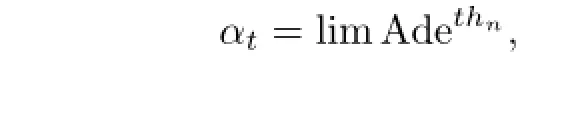
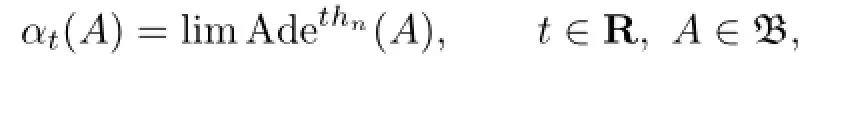
i.e.,or equivalently,uniformly continuous in t on every compact subset of R and every A∈B. A fl ow on a Banach algebra B is said to be asymptotically inner if there is a continuous function h of R+into B such that

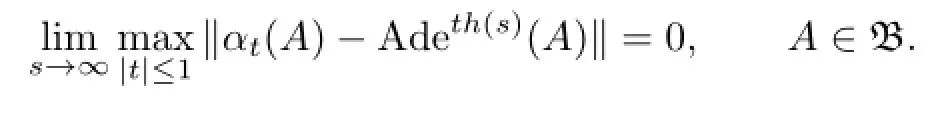
Corollary 2.2Letαandβbe two fl ows on Banach algebraB.Then the following conditions are equivalent:

(i)βis cocycle-conjugate toα;

(ii)βis an inner perturbation ofσασ−1for some automorphismσofB,whereσασ−1is the actiont

If one of above conditions is satis fi ed andαis inner(approximately or asymptotically inner), then so isβ.

Proof.The fi rst statement of the proposition can be obtained from Corollary 1.3 of[3].We just give the proof of the last statement of the corollary.

First we prove that if β is an inner perturbation of α,i.e.,

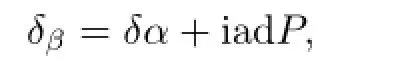
then it follows that if α is inner(approximately or asymptotically inner),so is β.

(a)If α is inner,then so is β by Corollary 2.1.

(b)If α is approximately inner,then there is a sequence{hn}in B such that

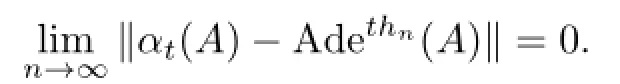
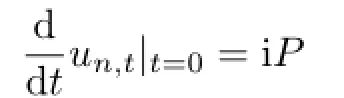
by means of Lemma 2.3.Since βt=Adut◦αtand un,t→ut,we obtain that

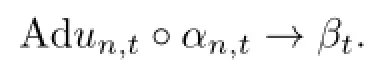
Besides,Adun,t◦αn,tis inner by Corollary 2.1.Then β is approximately inner.

(c)If α is asymptotically inner,then there is a continuous function h of R+into B such that

by means of Lemma 2.3.Since βt=Adut◦αtand us,t→ut,we obtain that

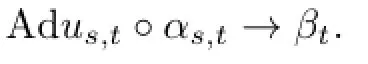
by Corollary 2.1.Then β is approximately inner.The proof is completed.

In the following we prove that if β is conjugate to α,i.e.,there is a bounded automorphism σ of B such that

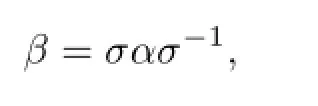
then,if α is inner(approximately or asymptotically inner),then so is β.

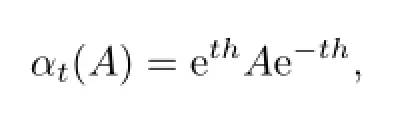
then

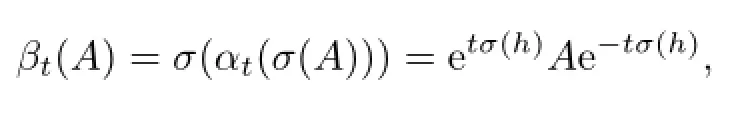
i.e.,β is inner.

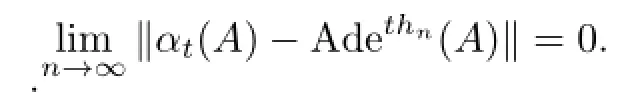
Because β is conjugate to α,i.e.,

σ is the bounded automorphism of A,so there exists an M＞0 such that

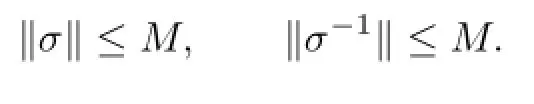
Therefore,

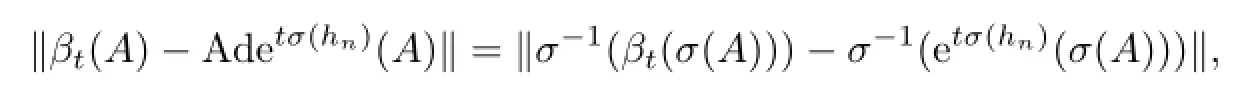
i.e.,β is asymptotically inner.

Finally,if β is cocycle-conjugate to α,then there is a bounded automorphism σ of B such that β is a cocycle perturbation of σασ−1.If α is inner(approximately or asymptotically inner),then so is σασ−1.Then so is β.

[1]Bratteli O,Robinson D W.Operator Algebras and Quantum Statistical Mechanics I.Berlin-Heidelberg-New York:Springer-Verlag,1979.

[2]Sakai S.Operator Algebras in Dynamical Systems.Cambridge:Cambridge Univ.Press,1991.

[3]Kishimoto A.Locally representable one-parameter automorphism groups of AF algebras and KMS states.Rep.Math.Phys.,2000,45:333–356.

[4]Kishimoto A.UHF fl ows and the fl ip automorphism.Rev.Math.Phys.,2001,13(9):1163–1181.

[5]Kishimoto A.Examples of one-parameter automorphism groups of UHF algebra.Comm.Math. Phys.,2001,216:395–428.

[6]Kishimoto A.Approximately inner fl ows on seperable C∗-algebras.Rev.Math.Phys.,2002,14:1065–1094.

[7]Kishimoto A.Approximate AF fl ows.J.Evolution Equation,2005,5:153–184.

[8]Kishimoto A.The one-cocycle property for shifts.Ergodic.Theory Dynam.Systems,2005,25: 823–859.

[9]Kishimoto A.Multiplier cocycles of a fl ow on a C∗-algebra.J.Funct.Anal.,2006,235:271–296.

[10]Kishimoto A.Lifting of an asymptotically inner fl ow for a separable C∗-algebra.in:Bratteli O, Neshveyev S,Skau C.The Abel Symposium 2004 Operator Algebras.Berlin:Springer,2006: 233–247.

[11]Davidson K R.Nest Algebras.Essex:Longman Group UK limited,1988.

tion:47D03,46H99,46K50,46L57

A

1674-5647(2014)01-0001-10

Received date:Sept.17,2010.

Foundation item:The NSF(11226125,10971079,11301379)of China.

E-mail address:shiluoyi@aliyun.com(Shi L Y).