Easy profit maximization method for open-pit mining

C. Dinis da Gama

IST - Technical University of Lisbon, Avenida Rovisco Pais 1, 1049-001 Lisboa, Portugal

1. Basic concepts

Mining is essentially governed by the knowledge obtained from three scientific disciplines: geology, mining engineering and economics.

Only through an intensive program of research and field studies can a successful mining project be implemented. This program must be carefully coordinated through a succession of pre-designed stages. Since the ultimate goal to achieve is obtaining an economic benefit upon the invested capital, that benefit is justified due to the uncertainty and risk that are involved in all those stages.

Initial studies are geological in nature, based on pre-existing information (publications, technical reports, satellite images, etc.)and usually complemented with data from exploration activities on the ground, involving studies of geology, geophysics and geochemistry. Representative sample acquisition follows from field investigation including open-pits, shallow trenches, as well as drilling campaigns. These samples are then sent to specialized laboratories for the physico-chemical characterization of minerals,allowing the creation of a database that, after appropriate computational processing, may describe the main characteristics of the deposit. Analyses of technical and economic feasibility of the project are thus required, initially with reduced available data and subsequently with the greater volumes of information in order to provide reliable evaluations of project feasibility.

Questions on the possibility of ensuring future revenues from the sale of the final product to the market, which should be higher than the investments required in all steps of the previous study,must be answered. Moreover, it is expected that these revenues will cover the necessary capital for the mining project with yields that should be higher than those of alternative financial applications.

In essence, there is always a strong interaction between the four variables represented in Fig. 1.

It is obviously necessary to involve components of engineering and economics to answer these questions.

Gama (1986) listed the following tasks required to develop this

approach:

(1) Determine the geometric shape of the mineralization, by defining the spatial orientation of the ore to extract (including the distribution of its contents along the mineralized volume) as well as the barren rocky material that must be removed.

(2) Design the mining method appropriate for extracting the deposit, including the mechanical equipment to be used in operations of excavation, loading and transportation of both ore and waste.

(3) The techniques of washing/processing that will be recommended for the product concentration, in conditions of supplying the consumer market.

(4) The general costs necessary to implement the project, from investments in exploration and recognition of the deposit, to feasibility studies and engineering design of the mine, not to mention the corresponding indirect costs of production (of fices,business administration, social services, etc.)

(5) The funds to be spent as payments of taxes, miscellaneous fees and compliance with environmental protection rules will apply.

Fig. 1. The four main variables influencing the economic feasibility of a mining project, according to Gentry and O’Neil (1984).

There are several criteria to quantify all the variables listed above, being more used that proposed by Plewman (1970), Barnes(1980), and Hustrulid and Kuchta (2006).

It consists in defining the hourly profit function L ($/h) by the following relation:where P (t/h) is the hourly production of the mine, which is sent to the mill; T (%) is the average grade of ore that is mined; U (%)is the metal recovery rate obtained in the concentration plant; V($/t) is the sales price per ton of metal (or useful substance) out of the plant; M ($/t) is the cost of mining per ton of excavated ore;E ($/t) is the cost of removal per ton of waste material; R (t/t) is the instantaneous waste/ore stripping ratio; B ($/t) is the cost of processing per ton of ore that enters the plant; and F ($/h) is the hourly incidence of fixed costs, capital, taxes and other indirect charges.

Fig. 2 schematically represents those variables.

The profit function can also be formulated in unit values (per ton or cubic meter of ore), by assigning a unit profit function L′($/h),which is given by

In all these variables, there are two geological characteristics,which have great importance: the stripping ratio R and the grade of ore T. It is therefore essential to determine what their extreme values are which may lead to a zero profit.

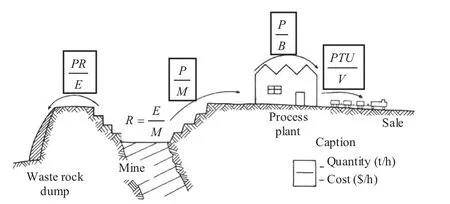
Fig. 2. Flowchart of production in an open-pit mine, with its main attributes.

Fig. 3. Typical variations of exploitable reserves (Q + W) and stripping ratio (R) as a function of the cut-off grade (TCM).

Thus, the minimum allowable cut-off grade is provided by the expression:meaning the grade content that separates the exploitable material(ore) from the one which is sent to the heap (waste).

In the same way, the maximum allowable stripping ratio is given by representing the largest amount of overburden material that can be removed in order to extract one ton of ore with an average grade T, above the level of TCM.

In practice, the operating conditions of any mine should be set away from those where profit can either be zero or negative, so that TCMand Rmaxare limit values not to be exceeded, considering that the other variables are unchanged.

It is worth noting the instant relationships that must exist between the quantities of ore, waste rock and the actual cut-off grade. Fig. 3 shows this typical variation, by means of introducing the concept of geological reserve Q + W, which is defined as the sum of the quantities of ore and waste, as well as showing a typical variation of the stripping ratio with cut-off grade.

Another relevant concept is the allowable stripping ratio Rad,which is defined upon the consideration of a minimum profit (Lmin)that must always exist, and it is given by

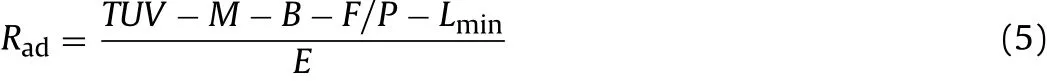
Therefore, the economic control of mining should be conducted in a continuous way and it must be based on the assessment of the levels of the ore grade T and stripping ratio R, which should satisfy the following conditions:

2. Correlations between ore body model and profit function

The ability to formulate three-dimensional block models for any mineral deposit can be used to evaluate the conditions of its exploitability.

For this purpose, it is important to correlate mathematically the relevant variables in the profit function and its dependence on the cut-off grade TC. In particular, there are three variables that can betreated alike: the ore reserve Q, the average content of the ore grade T, and the stripping ratio R, by means of three functions such as:

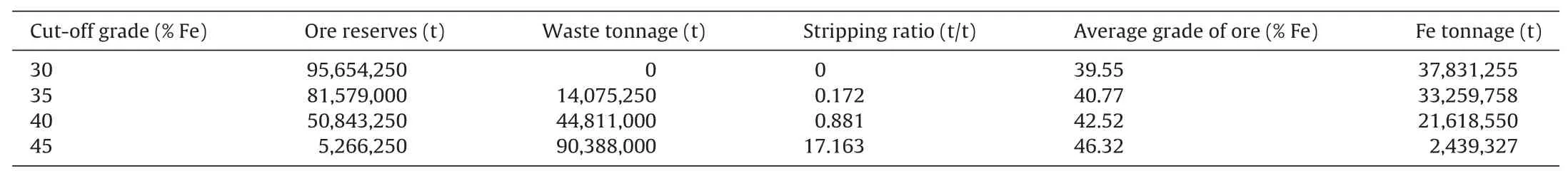
Table 1Summary of reserves calculated for the Mua ore body, for various levels of cut-off grade.

Substituting these variables in the pro fit function formula, a direct relationship between pro fit and cut-off grade is obtained in order to allow a continuous monitoring of the mining operation economics.

One can thus determine relevant characteristics of the project,such as the optimal cut-off grade (de fined as one that maximizes pro fit), the adequate stripping ratio and the maximum overall

pro fit.

The above can be illustrated with simple mathematical functions, of the following type:

where a0, a1, b0, b1, c0, and c1are constant coefficients.

It is then possible to establish equations linking operational pro fit with the cut-off grade and then find the cut-off grade value that leads to that maximum pro fit.

In this way, the total pro fit would be given by the expression:

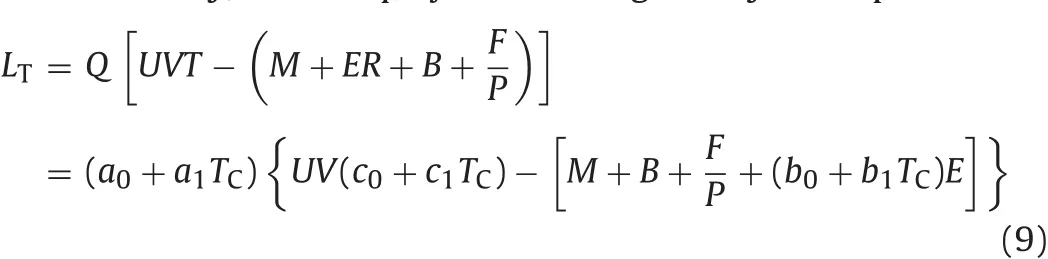
To determine the maximum of this function, it can be derived with respect to TC, and afterwards making it equal to zero in order to determine the optimal value of cut-off grade TOP, which is given by

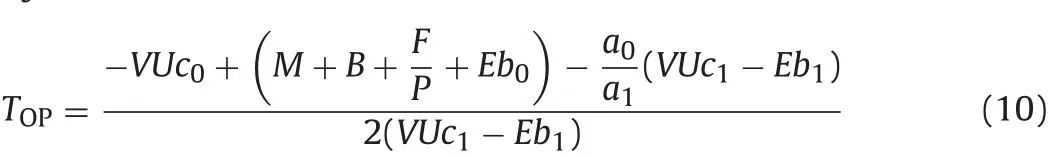
This important expression is of great interest, for it involves the four main variables included in Fig. 1 and may determine any instantaneous balance between them, as well as providing advantageous solutions for mine production planning.

Table 3Data set of Q, R and T as function of cut-off grade, subjected to statistical regression analysis (as all correlation coefficients are greater than 0.88, there is no need to use polynomials with a degree higher than 1).

Table 2Application example: numerical values of variables involved in the pro fit function.

aAnother source (Index Mundi) in August 2011 indicates the value (177.66 $/t)of iron ore concentrate with 62% Fe imported into China (CFR Tianjin Port).

3. Application of real geological data

Table 1 contains the main results of the geological characterization of the iron ore deposit of Cabec¸ o de Mua (Portugal), before applying economic considerations.

In addition, a set of economic parameters were determined upon feasibility studies on the project, which are shown in Table 2.

From these values it follows that the unit pro fit function is given by the relation:

resulting in (for the case of R = 0) a minimum cut-off grade of 27.17%Fe.

As indicated in Table 1, the average grade of the entire deposit is 39.55% Fe, a value that is well above the minimum cut-off grade,thus indicating favorable conditions of exploitability.

Other parameters of interest can be calculated in order to seek more pro fitable opportunities.

In effect, on the basis of data presented in Table 1, it is possible to quantify the three functions mentioned above (Q, R and T), as Table 3 shows.

It must be emphasized that R may not assume negative values,so in a situation where TC＜ -b0/b1, the values of b0= b1= 0 must be used.

Substituting the numerical data of Table 3 in the expression for calculating the optimal cut-off grade, we obtain TOP= 25.17% Fe,leading to a maximum total pro fit of:

Fig. 4. Sensitivity analysis of effects of selling price variations.

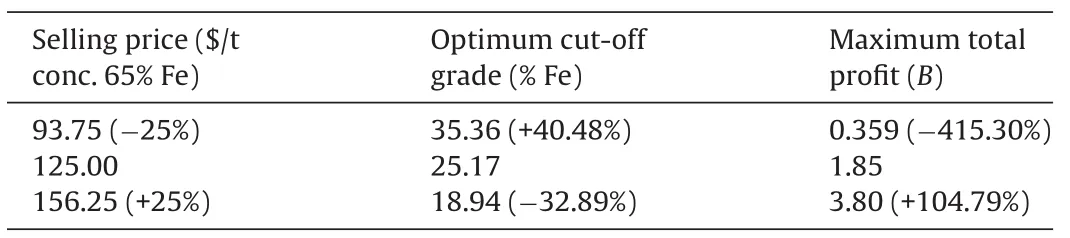
Table 4Values of the optimal cut-off grade and the maximum total profit for a range of iron ore concentrate selling prices of ±25% the basic value of 125.00 $/t.

The method opens the possibility of performing sensitivity analyses on different project variables, for example, by varying the iron ore concentrate selling price, which is the most important exogenous variable.

Assuming values of ±25% on the price shown in Table 2, a set of results may be gathered, as listed in Table 4, which provides a range of solutions that can contribute to adequate decision-making on project management. Such results are depicted in Fig. 4.

It may be observed that the changes in terms of optimal cut-off grade and the maximum total profit fluctuations are not proportional to the selling price variations, showing in particular a great variability for the maximum total profit.

The situation is particularly difficult for the lowest selling price, which requires a much greater optimum cut-off grade, thus decreasing the volume of exploitable reserves and leading to higher production costs due to the need for larger quantities of waste removal.

4. Conclusions

It was sought to describe the usefulness of an expedite approach to permanently control surface mining projects, with the aim of determining the optimum values of the main variables that influence project profitability.

Given a multitude of situations that might be encountered during the life of any mine operation, its managers must possess essential tools for rapid decision-making criteria and adjustment to reality. Such methods do not invalidate the applicability of more complex methodologies, such as integrated computational models,although these will lead to many difficulties in providing precise input data that sometimes are not available in mining companies.

Barnes MP. Computer-assisted mineral appraisal and feasibility. New York: Society for Mining Metallurgy; 1980.

Gama CD. Methodology for the control of mining profits. IPT Publication No. 1661.Brazil: Institute of Technological Research of São Paulo; 1986.

Gentry DW, O’Neil TJ. Mine investment analysis. New York: Society of Mining Engineers; 1984.

Hustrulid W, Kuchta M. Open pit mine planning & design. 2nd ed. London: Taylor &Francis; 2006.

Plewman RP. The basic economics of open pit mining. In: Symposium on planning open pit mines; 1970.