一类非线性抛物方程Cauchy问题解的存在性条件
陈美珍,潘佳庆
(集美大学理学院,福建厦门 361021)
一类非线性抛物方程Cauchy问题解的存在性条件
陈美珍,潘佳庆
(集美大学理学院,福建厦门 361021)
研究了带源项的非线性抛物方程Cauchy问题解存在的必要条件以及解所应具有的性质,通过把文献中的线性算子推广到形式较一般的带源项的非线性抛物算子,利用其中处理线性问题的方法来处理非线性问题.
Cauchy问题;必要条件;L1可积
1 引言


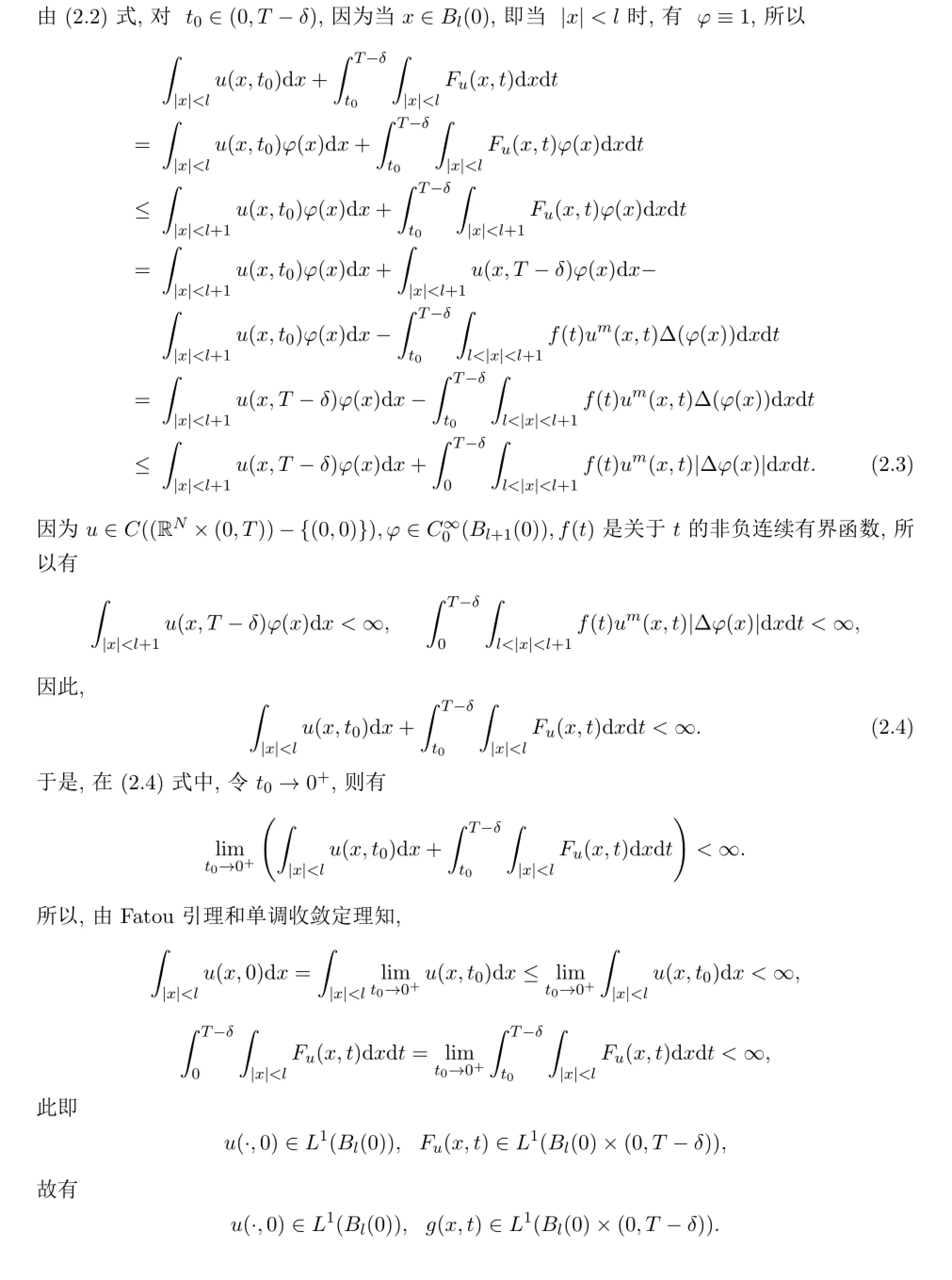
2 定理及其证明


参考文献
[1]张剑文,赵俊宁.非定常边界层问题整体解的存在唯一性[J].中国科学:A辑,2006,36(8):870-900.
[2]王培林,谭忠.具有Neumann边界条件及临界Sobolev指数的半线性抛物方程整体解的渐近性及Lq估计[J].厦门大学学报:自然科学版,2004,43(1):17-20.
[3]金春花,尹景学.具非线性源的非散度型扩散方程的临界指标[J].数学年刊:A辑,2009,30(4):525-538.
[4]孙法国,薛应珍.一类非线性抛物型方程组解的整体存在及爆破[J].纯粹数学与应用数学,2007,23(3):304-310.
[5]容跃堂,樊彩虹.一类带有非局部源的退化反应扩散方程组解的整体存在与爆破[J].纯粹数学与应用数学, 2009,25(1):39-46.
[6]屈改珠,朱春蓉.(3+1)维带有源项的反应扩散方程的不变集和精确解[J].纯粹数学与应用数学,2009,25(3):580-585.
[7]Daskalopoulos P,Pino M D.On nonlinear parabolic equations of very fast difusion[J].Arch.Rational Mech. Anal.,1997,137:363-380.
[8]Kubo M,Lu Q Q.Nonlinear degenerate parabolic equations with Neumann boundary condition[J].J.Math. Anal.Appl.,2005,307:232-244.
[9]Lair A V,Oxley M E.A necessary and sufcient condition for global existence for a degenerate parabolic boundary value problem[J].Journal of Mathematical Analysis and Applications,1998,221:338-348.
[10]Taliaferro S D.Isolated singularities of nonlinear parabolic inequalities[J].Math.Ann.,2007,338:555-586.
[11]谷超豪,李大潜,陈恕行,等.数学物理方程[M].2版.北京:高等教育出版社,2002.
[12]Vazquez J L.An Introduction to the Mathematical Theory of the Porous Medium Equation[M]//Shape Optimization and Free Boundaries.Dordrecht:Kluwer Academic,1992.
[13]Mizoguchi N,Quiros F,Vazquez J L.Multiple blow-up for a porous medium equation with reaction[J]. Mathematische Annalen,2011,350:801-827.
The existence conditions of solutions of a class of nonlinear parabolic equation
Chen Meizhen,Pan Jiaqing
(School of Science,Jimei University,Xiamen361021,China)
This paper discusses the necessary conditions of solution to a class of nonlinear parabolic equation and the nature of the solution.By extending the linear operator to a more general form of nonlinear parabolic operator with the source term,we use the method in document which was used to handle the linear problem to handle the nonlinear problem.
the Cauchy problem,the necessary conditions,L1integrable
O175.26
A
1008-5513(2013)06-0654-07
10.3969/j.issn.1008-5513.2013.06.016
2013-11-01.
陈美珍(1986-),硕士生.研究方向:非线性数学物理.
潘佳庆(1957-),博士,教授.研究方向:偏微分方程,流体力学.
2010 MSC:35K10,35K15,35K55

