伪黎曼流形上的Simons型不等式
杨慧章,穆 凤
(红河学院数学学院,云南 蒙自 6661100)
伪黎曼流形上的Simons型不等式
杨慧章,穆 凤
(红河学院数学学院,云南 蒙自 6661100)
是n维黎曼流形到n+p维伪黎曼流形的等距浸入.通过计算Ricci张量长度平方的拉普拉斯算子,得到了伪黎曼流形上的一个Simons型积分不等式.
伪黎曼流形;Ricci张量;拉普拉斯算子
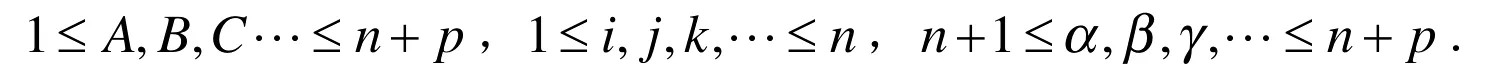


限制在M上有
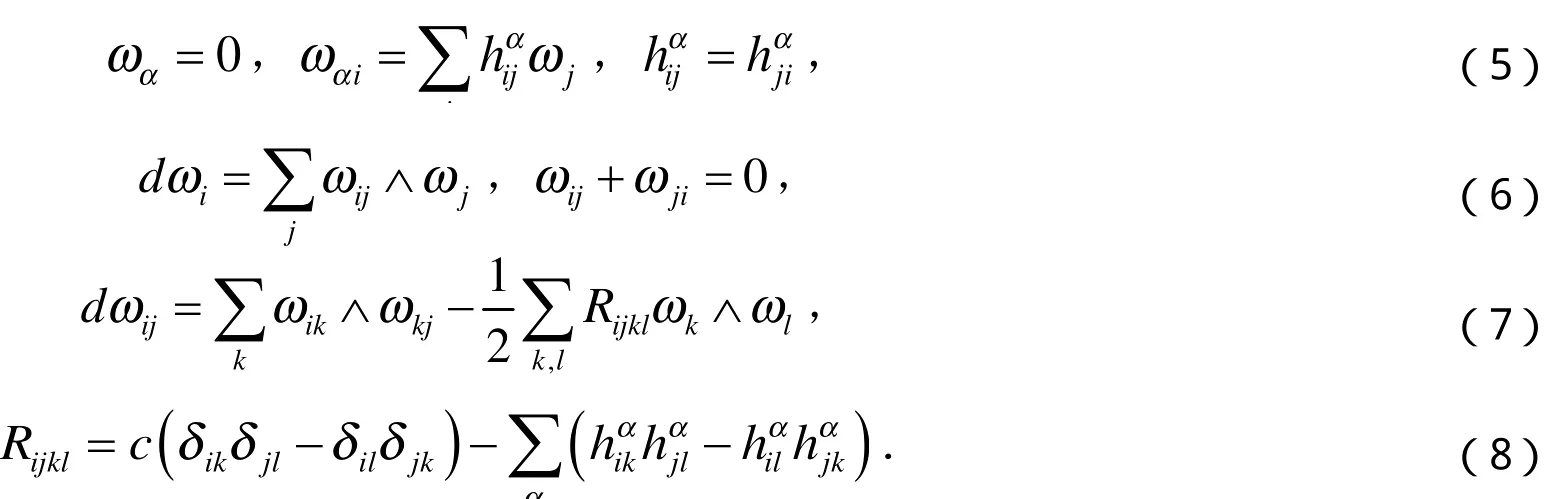
还有
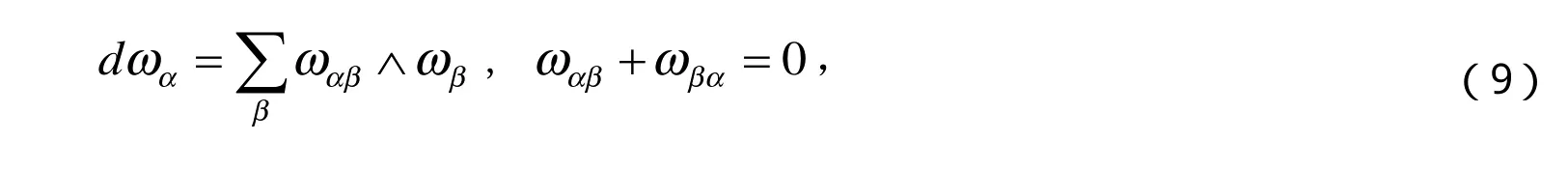
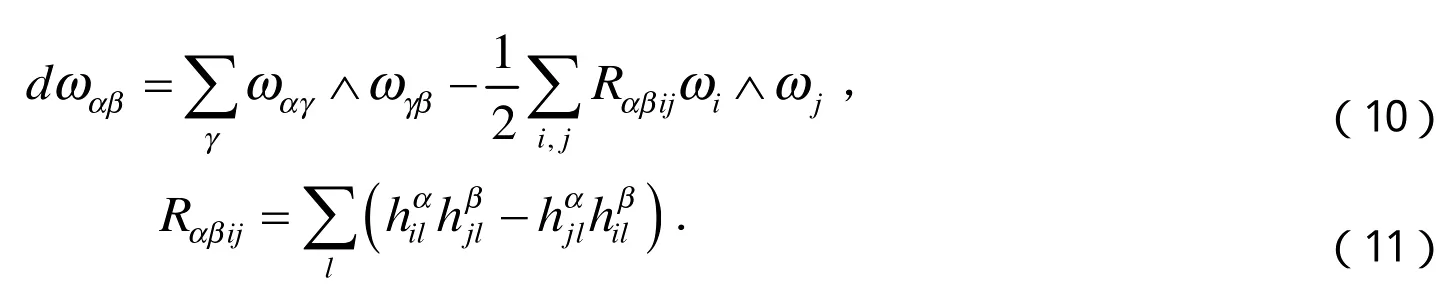
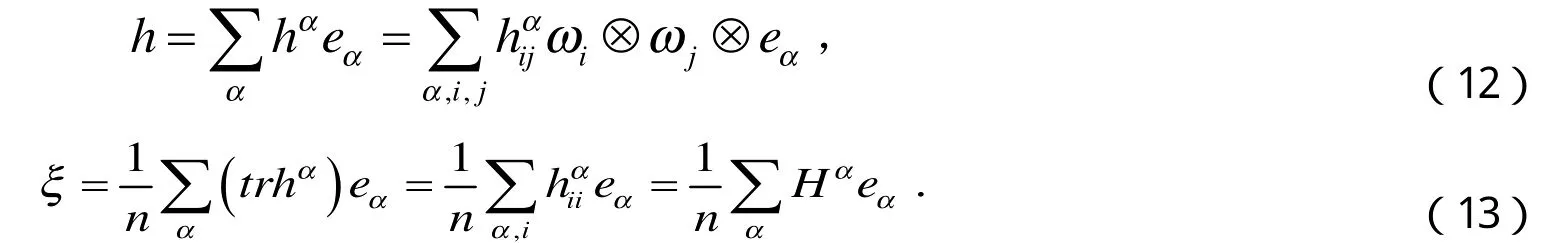
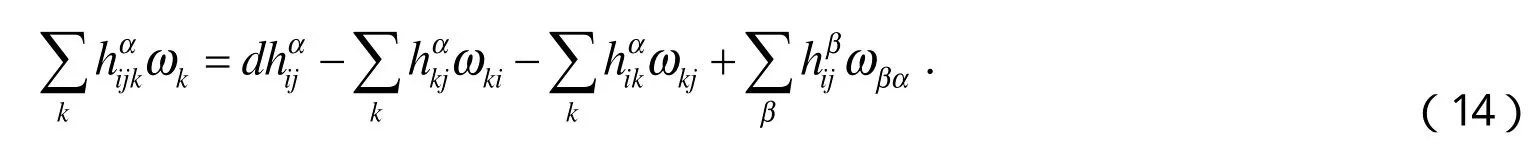
Codazzi方程为

Ricci恒等式为

由(8)式得,M的Ricci曲率为

定义Ricci张量的共变导数如下:

由于Mn为中的极大类空子流形,由(17)、(18)式及M的极大性得
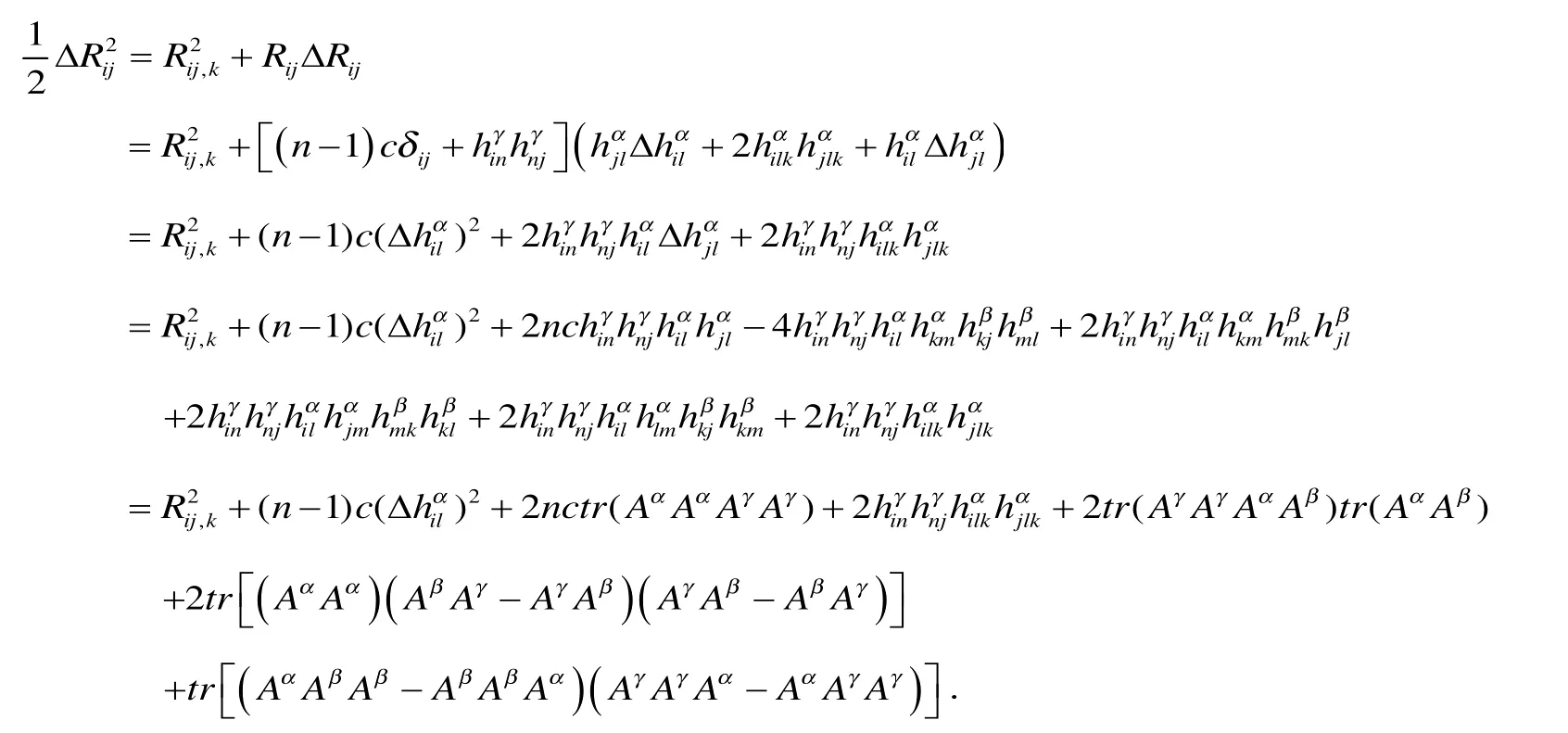
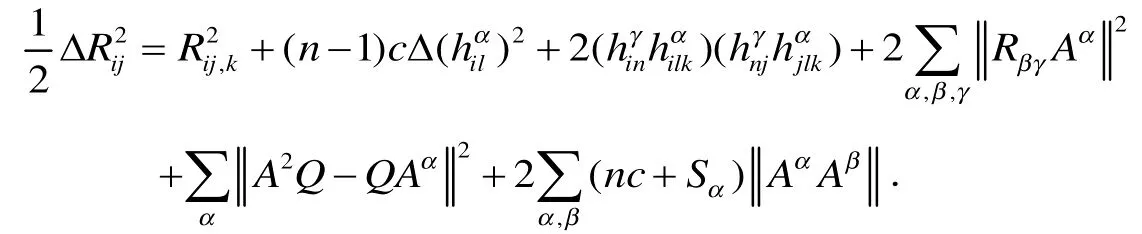
由于M为紧致的极大类空子流形,对上式两边积分有

由此易得下面的定理:
定理 若M为紧致的极大类空子流形,则有
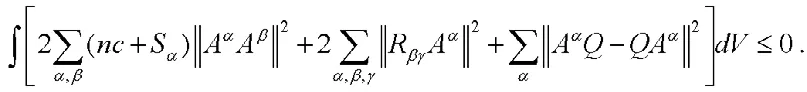
文中通过计算Ricci张量Laplaian算子,从内蕴量的角度给出了伪黎曼流形中紧致极大类空子流形的一个Simons型积分不等式.关于此不等式在伪黎曼流形中的应用,还需进一步的研究.
[1] Chern S.S., Do Carmo M, Kobayashi S.Minimal submanifolds of a sphere with second fundamental form of constant length[J].Functional Analysis and Related Field.Berlin, Springer-verlag.1970, 59-75.
[2] Xu Hongwei.On closed minimal submanifolds in p inched Riemannian manifolds[J].TransAmerMath Soc, 1995, 347 (5) :1743~1752.
[3] Takabashi T.Minimal immersions of Riemannian manifolds[J].Math.Soc.Japan,1960, 18:380-385.
[4] SONG Weidong , ZHANG Liang.An integral inequality of Simons’type[J] .Journal of Anhui Normal University:Natural Science Edition ,2003 ,26 (3) :205 - 208.
[5] 陈六新,郭震,李同柱.一个新的Simons 型不等式[J].西南师范大学学报,2003,28(4):533-535.
A Simons’ Type Inequality in Pseudo-Riemannian Manifold
YANG Hui-zhang,MU Feng
(Department of Mathematics, Honghe University, Mengzi 661100,China)
Letbe a n-dimensional Riemannian manifold isometrically immersed into (n+p)-dimensional pseudo-Riemannian manifold.By calculat ing the Laplacian of the square of the length of Ricci curvature, a new Simons’ integral inequality is obtained in the pseudo-Riemannian manifold.
pseudo-Riemannian manifold; Ricci curvature; Laplacian
O186.13
A
1008-9128(2012)02-0029-03
2011-10-19
红河学院科研基金(10XJY121)
杨慧章(1982—),女,昆明人,硕士,讲师.研究方向:微分几何.
[责任编辑 张灿邦]

