四氯合锌酸正九烷铵的晶体结构及热化学性质

卢冬飞 邸友莹 何东华

(聊城大学化学化工学院,山东聊城252059)

四氯合锌酸正九烷铵的晶体结构及热化学性质

卢冬飞 邸友莹*何东华

(聊城大学化学化工学院,山东聊城252059)

合成了四氯合锌酸正九烷铵复合物(C9H19NH3)2ZnCl4(s)(C9Zn(s)),并使用X射线单晶衍射、化学分析以及元素分析确定了其晶体结构和化学组成.利用其晶体学数据推导了C9Zn(s)的晶格能UPOT=952.94 kJ·mol-1.在298.15 K下,利用恒温环境溶解-反应热量计测定了C9Zn(s)在不同质量摩尔浓度下的摩尔溶解焓.在Pitzer电解质溶液理论基础上确定了C9Zn(s)的无限稀释摩尔溶解焓ΔsΗm∞=20.09 kJ·mol-1,以及Pitzer焓参数组合的值.

四氯合锌酸正九烷铵;晶格能;无限稀释摩尔溶解焓;Pitzer参数;Pitzer理论

1 Introduction

Energy source is the most basic driving force for society development and economic growth,and energy source is also the basis of human survival.Nowadays,with the development of society,the demand for energy sources is over-growing,but the supply of the energy sources always falls short of the demand. Therefore,to develop a new green energy,improve the utilization of energy sources in the modern world,and reduce the waste of energy sources have attracted much attention.For these purposes,phase change materials(PCMs),especially solid-solid phase change materials(SSPCMs),have become a hot spot in the fields of energy and material sciences.

As a kind of solid-solid phase change materials,plenty of studies1-7about the complexes(n-CnH2n+1NH3)2MX4are reported, in which M is a divalent transition metal ion(M=Zn2+,Cu2+, Mn2+,Cd2+,Co2+,etc.),X is a halogen and n varies between 8 and 18.However,the title complex,(C9H19NH3)2ZnCl4(s) (C9Zn(s)),has never been synthesized until now,and its crystal structure,lattice potential energy,and some basic thermochemical data have never been reported.It is needed to develop its new application fields and to carry out relevant theoretical research.In this paper,C9Zn(s)is synthesized,the crystal structure is determined by X-ray crystallography,lattice potential energy is calculated,and the molar enthalpy of dissolution of C9Zn(s)at infinite dilution and the sums of Pitzer′s parametersare obtained.

2 Experimental

2.1 Synthesis of the title complex

n-Nonylamine(99%,mass fraction),hydrochloric acid (37%,mass fraction),and zinc chloride(99%,mass fraction) were chosen as a reactants and anhydrous methanol(analytical grade)was used as the solvent.The reactants were accurately weighed at a molar ratio of n(C9H19NH3):n(HCl):n(ZnCl2)=2:2: 1 and slowly dissolved into anhydrous methanol under sufficient stirring.The mixture was heated and stirred under refluxing for 6 h.After solution was boiled and refluxed for 6 h,the mixture was condensed by boiling off some anhydrous methanol until crystal membrane emerged from the solution surface. The final solution was naturally cooled to room temperature, filtered,and colorless transparent crystal was obtained.The product was recrystallized for three times with anhydrous methanol and colorless crystal was gained.Finally,the sample was placed in a vacuum desiccator at T=303.15 K to dry in vacuum for 12 h,the final product was placed in a weighing bottle and preserved in a desiccator.The purities(mass fraction)of the Zn and Cl in the complex were determined by chemical analyses, and the contents of C,H,and N were determined by element analyses(model:PE-2400,Perkin Elmer,USA).The contents of Zn,Cl,C,H,and N in the complex have been measured to be 13.17%,28.62%,43.57%,8.97%,and 5.63%,respectively. Theoretical contents of Zn,Cl,C,H,and N in the complex have been calculated to be 13.19%,28.60%,43.61%,8.95%, and 5.65%,respectively.This showed that the purity of the sample prepared was higher than 99.64%(mass fraction).

2.2 X-ray crystallographic analysis

A single crystal suitable for X-ray analysis was glued to a fine glass fiber and was then mounted on the Bruker Smart-1000 CCD diffractometer with Mo-Kαradiation,λ=0.071073 nm.The intensity data was collected at(298±2)K.The structure was solved by direct methods and expanded using Fourier techniques with SHELXL-97 program.8The non-hydrogen atoms were refined anisotropically by full-matrix least-squares calculations on F2.The hydrogen atoms were added theoretically,riding on the concerned atoms,and not refined.Crystallographic data for the structural analysis of C9Zn(s)has been deposited with the Cambridge Crystallographic Data Centre,and we have applied for a CCDC No.808503.Crystal data and refinement details are summarized in Table 1.

2.3 Isoperibol solution-reaction calorimetry

The isoperibol solution-reaction calorimeter consisted primarily of a precision temperature controlling system,an electric energy calibration system,a calorimetric body,an electric stirring system,a thermostatic bath made from transparent silicate glass,a precision temperature measuring system,and a data acquisition system.The principle and structure of the calorimeter were described in detail elsewhere.4Experiments have demonstrated that the precision of temperature controlling of this system can reach±1×10-3K,and measurements9-11indicate that the precision of the temperature measurement system can reach±1×10-4K at least and the time constant of the calorimeter was about 3 s.

The reliability of the calorimeter was verified previously by measuring dissolution enthalpy of KCl(calorimetrically primary standard)in double distilled water.According to the molar ratio of KCl to water,nKCl:nH2O≈1:1110,a certain amount of KCl was dissolved in 100 cm3double distilled water at T=(298.150±0.001)K.The average enthalpy of dissolution of KCl was(17547±13)J·mol-1,which was compared with corresponding published data(17536.0±3.4)J·mol-1under the same experimental conditions.12This showed that the relative deviation between the literature value and the measuring value was within±0.3%.

Table 1 Crystal data and structure refinement for the complex C9Zn(s)

3 Results and discussion

3.1 Description of crystal structure

The crystal parameters,data collection,and refinement results for the complex are listed in Table 1.It is found from Table 1 that crystal system of the complex is orthorhombic,space group is Pnma,unit cell dimensions are a=1.03231(12)nm,b= 0.74250(8)nm,c=3.5130(3)nm;α=β=γ=90°,and Z=4.The calculated density of the complex is 1.223 g·cm-3and the volume of formula unit is 269.27(5)nm3.Selected bond lengths and bond angles are listed in Table 2.The geometries of the hydrogen bonding are listed in Table 3.The molecular structure of C9Zn(s)is shown in Fig.1,and the packing of the cell structure is plotted in Fig.2.

From Fig.1 and Table 2,we can see that the title complex was formed by two(C9H19NH3)+cations and one[ZnCl4]2-anion.As shown in Fig.2 and Table 3,the anions and cations are connected via electrostatic interaction and hydrogen bonds NH…X.Hydrogen bonds N－H…X together with the van der Waals forces exist in the crystals to stabilize the structures.All hydrogen atoms are placed in geometrically idealized positions and constrained to ride on their parent atoms.

3.2 Lattice potential energy of the complex

Table 2 Selected bond lengths(L)and bond angles(θ)for the complex C9Zn(s)

Table 3 Hydrogen bond lengths and bond angles for the complex C9Zn(s)

Equation(1)is used to estimate lattice potential energy for the general type of the salts MpXq: where z+and z-are the respective charges on the cation and anion of the complex,p and q are the number of the cation and anion per molecule of the general type of the salts MpXq,respectively,v is the number of ions per molecule and is equal to (p+q).For the case of salts of formula M2X with charge ratio (1:2),as for the title complex C9Zn(s),z+=1,z-=2,p=2,q=1,v= 3,α′=165.3 kJ·mol-1·nm,β′=-29.8 kJ·mol-1,where α′and β′are constants13,and Vmis molecular(formula unit)volume in units of nm3,14,15

Fig.2 Packing structure of the complex C9Zn(s)

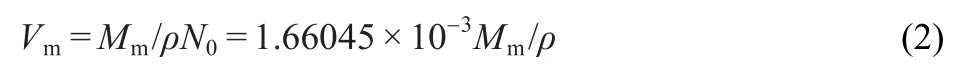
where N0is the Avogadro′s constant,6.02245×1023molecules· mol-1.Thus,the formula(1)is changed to:3

where the values of the constants for M2X(1:2)are:γ=8375.6 kJ·mol-1·cm and δ=-178.8 kJ×mol-1;3The density ρ(ρ=1.223 g·cm-3)and molar mass Mm(Mm=495.72 g·mol-1)are obtained from crystal structure data of the title complex in Table 1.Vmis calculated to be 0.6730 nm3according to equation(2), consequently lattice potential energy of C9Zn(s)is derived to be UPOT=952.94 kJ·mol-1.

3.3 Molar enthalpy of dissolution at infinite dilution and sum of Pitzer′s parameters

C9Zn(s)can be dissolved in double-distilled water.The experimental values of enthalpy of dissolution(ΔsHm)of C9Zn(s) in double-distilled water are given in Table 4,and the curvilinear relationship of ΔsHm(in kJ·mol-1)with molality m(in mol· kg-1)is shown in Fig.3.

The dissolution process of C9Zn(s)may be expressed as:

where W is the number of kilograms of solvent.Since C9Zn(s) is dissociated to 2C9H19NH+3(aq),Zn2+(aq),and 4Cl-(aq)at infinite dilution,the excess Gibbs energy(Gex)can be expressed by Pitzerʹs unsymmetrical mixing electrolyte solution theory.16-18

The general form of Gexof electrolytes16-21is given by

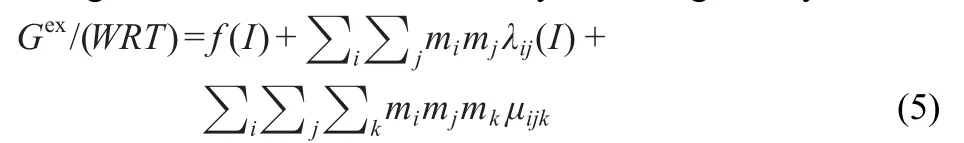
i,j,and k refer to different ions in the solution.The quantities λij(I)is the second virial coefficient for pairwise interaction between ions i and j,and a function of I(the ionic strength),m is the molality of the solute.The ionic strength dependence may be neglected for the third virial coefficientμijk,which represents the triple ion interactions and is important only at high concentrations.R is the molar gas constant,8.3145 J·mol-1· K-1.f(I)is a general function of the ionic strength I which is related to the electrical forces.The expression for f(I)is given by16-21

where b is a parameter having the value 1.2 kg1/2·mol-1/2for all solutions andAϕis the Debye-Hückel coefficient for the osmotic coefficient given by

where D is the dielectric constant or relative permittivity,dwis the density of the water or other solvents,e is the electronic charge,ε0is the permittivity of free space,N0is the Avogadro constant,and k is the Boltzmann constant.

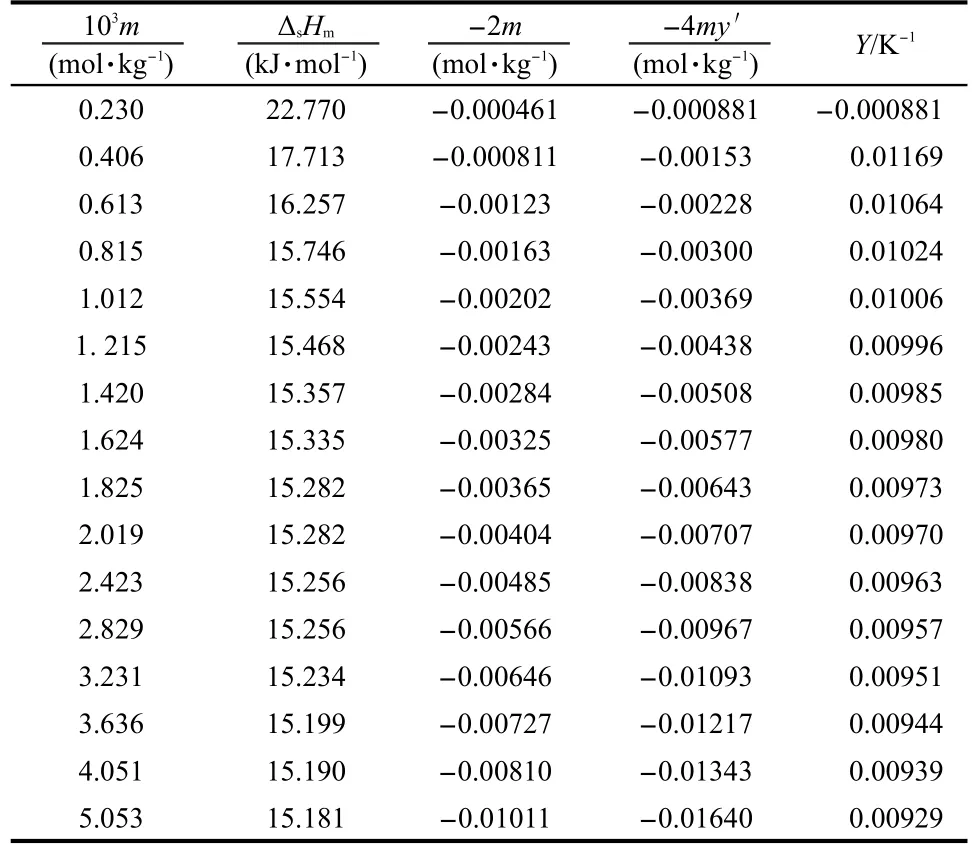
Table 4 Values of thermodynamical parameters of the complex C9Zn(s)at various molalities at T=298.15 K

The virial coefficients depend on the short-range forces between solute particles.The individual coefficient for ions cannot be measured.Therefore,measurable coefficients of electrically neutral combinations are defined by

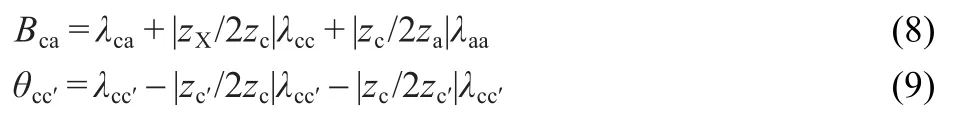
which c and c′are ions of the same sign,and a is an ion of the opposite signs.The B terms can be evaluated from the pure electrolyte data;the θ terms arise only for mixtures and can be evaluated from the common-ion mixtures.22Since the λ depends on the ionic strength,so do B and θ.The following empirical expressions22have been found to represent the ionic dependence of B and θ satisfactorily:

where the value of α is normally equal to 2.0 kg1/2·mol-1/2,andare characteristic parameters of electro-lyte.We substitute Eqs.(8-11)into Eq.(5)and obtain the following equation:16-21

Fig.3 Plot of measured dissolution enthalpy ΔsHmof the complex C9Zn(s)as a function of molality m at T=298.15 K

Since C9Zn(s)may be dissociated to(aq)+Zn2+(aq)+4Cl-(aq)}in water and the molality of the sample in dissolution experiment is relatively low,the electrolyte solution may be regarded as a unsymmetrical mixed electrolytes solution: 2C9H19NH3Cl-ZnCl2andm[C9H19NH3]+=2m,mZn2+=m,andmCl-= 4m.Equation(12)can be rewritten as follows:

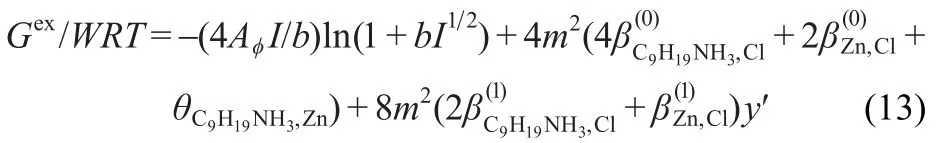
whereyʹ=[1-(1+2I12)exp(-2I1/2)]/2I.The equations for the excess enthalpyL=Hexobtained from temperature differentiation22of Eq.(13)can be expressed as,

where AHis the Debye-Hückel parameters for enthalpy,23AH= 1986 J·mol-1at T=298.15 K.

From the above equations and the excess enthalpyL=Hex,a working equation24to determine the Pitzerʹs parameter is shown as,

where Y is the extrapolation function,-2m and-4my′are the variables of the fitting process,ΔsHmis the molar enthalpy of dissolution of the complex,α0=ΔsH∞m/(2RT2).The values of Y,-2m,and-4myʹare given in Table 4,respectively.The three dimensional(3D)chart of Y against-2m and-4my′is plotted in Fig.4.Regression of Y against-2m and-4my′is made by the least squares to obtain α0=0.01359,=-8.150,and=5.237. The standard deviation of fitting is 9.00×10-4.The molar enthalpy of dissolution for C9Zn(s)at infinite dilution is determined to be0.09 kJ·mol-1.

Fig.4 3D chart of Y against-2m and-4my′for the complex C9Zn(s)

The degree of chaos or disorder of the system is increased when C9Zn(s)is dissolved in double-distilled water.It is concluded that the entropy change of dissolution reaction is greater than zero according to the statistical explanation of the entropy in statistical thermodynamics.From the values of enthalpy of dissolution,we deduce that the dissolution of C9Zn(s)in water is a typical endothermic process,25which indicates that it should be an entropy-driven process instead of enthalpy-driven process.

4 Conclusions

Bis(n-nonylammonium)tetrachlorozincate C9Zn(s)was synthesized,and the crystal structure of the complex has been determined by X-ray crystallography.The lattice potential energy was obtained from the crystallographic data.The molar enthalpy of dissolution of C9Zn(s)at infinite dilution was determined to beΔsΗ∞m=20.09 kJ·mol-1and the sums of Pitzerʹs parameters were obtained through Pitzerʹs electrolyte solution theory. The result indicated that it was an endothermic reaction when C9Zn(s)was dissolved in double-distilled water,which indicated that it should only be an entropy-driven process.

(1) Hawes,D.W.;Banu,D.;Feldman,D.Solar Energy Mater. 1990,21,61.

(2) Lee,K.W.;Lee,C.E.;Kim,J.;Kang,J.K.Solid State Commun.2002,124,185.

(3)Kong,Y.X.;Di,Y.Y.;Yang,W.W.;Lü,Y.F.;Tan,Z.C.Chin. J.Chem.2010,28,521.

(4) Kang,J.K.;Choy,J.H.;Madeleine,R.L.J.Phys.Chem. Solids 1993,54(11),1567.

(5) Li,W.P.;Zhang,D.S.;Zhang,T.P.;Wang,T.Z.;Ruan,D.S.; Xing,D.Q.;Li,H.B.Thermochim.Acta 1999,326,183.

(6) Wu,K.Z.;Zhang,J.J.;Liu,X.D.Thermochim.Acta 2009,483, 55.

(7)Arend,H.;Huber,W.J.Cryst.Growth 1978,43,213.

(8)Yang,W.W.;Di,Y.Y.;Li,J.;Kong,Y.X.J.Chem.Thermodyn. 2009,41,945.

(9)Di,Y.Y.;Yu,H.G.;Tan,Z.C.;Gao,S.L.;Liu,Y.;Sun,L.X. J.Chem.Thermodyn.2003,35,885.

(10)Di,Y.Y.;Tan,Z.C.;Gao,S.L.;Wang,S.X.J.Chem.Eng. Data 2004,49,956.

(11) Yu,H.G.;Liu,Y.;Tan,Z.C.;Dong,J.X.;Zou,T.J.;Huang,X. M.;Qu,S.S.Thermochim.Acta 2003,401,217.

(12) Rychly,R.;Pekarek,V.J.Chem.Thermodyn.1977,9,391.

(13) Glasser,L.;Jenkins,H.D.B.J.Am.Chem.Soc.2000,122,632.

(14) Jenkins,H.D.B.;Glasser,L.Inorg.Chem.2002,41,4378.

(15) Glasser,L.Thermochim.Acta 2004,421,87.

(16) Yang,J.Z.;Pitzer,K.S.J.Solut.Chem.1988,17,909.

(17) Pitzer,K.S.J.Solut.Chem.1975,4,3.

(18) Bradley,D.J.;Pitzer,K.S.J.Phys.Chem.1979,83,12.

(19)Ali,A.;Sabir,S.;Shahjahan;Hyder,S.Acta Phys.-Chim.Sin. 2007,23,1007.[Ali,A.;Sabir,S.;Shahjahan;Hyder,S.物理化学学报,2007,23,1007.]

(20) Pitter,K.S.J.Phys.Chem.1973,77,2.

(21) Sllvester,L.F.;Pltrer,K.S.J.Phys.Chem.1977,81,19.

(22) Phutela,R.C.;Pitzer,K.S.J.Solut.Chem.1986,5,649.

(23) Pitzer,K.S.Activity Coefficients in Electrolyte Solutions,2nd ed.;CRC:Boca Raton,1991;p 75.

(24) Pitzer,K.S.J.Phys.Chem.1983,87,2360.

(25)Yang,W.W.;Di,Y.Y.;Kong,Y.X.;Tan,Z.C.Chin.Phys.B 2010,19,060517.

January 26,2011;Revised:March 23,2011;Published on Web:April 13,2011.

Crystal Structure and Thermochemical Properties of Bis(n-Nonylammonium)Tetrachlorozincate

LU Dong-Fei DI You-Ying*HE Dong-Hua
(College of Chemistry and Chemical Engineering,Liaocheng University,Liaocheng 252059,Shandong Province,P.R.China)

We synthesized crystalline bis(n-nonylammonium)tetrachlorozincate(C9H19NH3)2ZnCl4(s) (C9Zn(s)).X-ray single crystal diffraction,chemical analysis,and elemental analysis were used to determine the crystal structure and composition of the complex.The lattice potential energy UPOTwas calculated to be 952.94 kJ·mol-1from crystallographic data.The molar enthalpies of C9Zn(s)dissolution using various molalities were measured in double-distilled water by an isoperibol solution-reaction calorimeter at 298.15 K.According to Pitzerʹs electrolyte solution theory the molar enthalpy of dissolution of C9Zn(s)at infinite dilution(ΔsΗm∞)was calculated to be 20.09 kJ·mol-1and the sums of Pitzerʹs parameterswere obtained.

Bis(n-nonylammonium)tetrachlorozincate;Lattice potential energy;Molar enthalpy of dissolution at infinite dilution; Pitzerʹs parameter; Pitzerʹs theory

O642

*Corresponding author.Email:diyouying@126.com,diouying@lcu.edu.cn;Tel:+86-635-8230645.

The project was supported by the National Natural Science Foundation of China(20673050,20973089).国家自然科学基金(20673050,20973089)资助项目