Generalized PP and Zip Subrings of Matrix Rings∗

LIU ZHONG-KUI AND QIAO HU-SHENG

(Department of Mathematics,Northwest Normal University,Lanzhou,730070)

Generalized PP and Zip Subrings of Matrix Rings∗

LIU ZHONG-KUI AND QIAO HU-SHENG

(Department of Mathematics,Northwest Normal University,Lanzhou,730070)

LetRbe an abelian ring.We consider a special subringAn,relative to α2,···,αn∈REnd(R),of the matrix ringMn(R)over a ringR.It is shown that the ringAnis a generalized right PP-ring(right zip ring)if and only if the ringR is a generalized right PP-ring(right zip ring).Our results yield more examples of generalized right PP-rings and right zip rings.

generalized right PP-ring,PP-ring,right zip ring

1 Introduction

All rings considered here are associative with identity.

A ring R is called a generalized right PP-ring if for any x∈R the right ideal xnR is projective for some positive integer n,depending on x,or equivalently,if for any x∈R the right annihilator of xnis generated by an idempotent for some positive integer n,depending on x.Left cases may be de fi ned analogously.A ring R is called a generalized PP-ring if it is both generalized right and left PP-ring.Right PP-rings are generalized right PP obviously. But the converse is not true(see[1]).

Let R be a ring.Denote by REnd(R)the set of all ring homomorphisms from R to R. De fi ne a subring of matrix ring Mn(R)over R as

By Proposition 3 of[1],if R is an abelian ring,then the ring Snis a generalized right PP-ring if and only if the ring R is a generalized right PP-ring.In Theorem 5 of[2],it was shownthat for a ring R,Snis a right(left)zip ring if and only if R is a right(left)zip ring.In this paper we consider the subring

where α2,···,αn∈REnd(R)are compatible ring homomorphisms from R to R.We show that if R is an abelian ring,then the ring Anis a generalized right PP-ring if and only if the ring R is a generalized right PP-ring,and for any ring R,Anis a right(left)zip ring if and only if R is a right(left)zip ring.Our results yield more examples of generalized right PP-rings and zip rings.

2 Main Results

Let R be a ring,and α be a ring endomorphism of R.According to[3]or[4],α is called compatible if for each a,b∈R,we have

For more information,examples,and other applications of this concept,we refer the reader to[3]and[4].

According to[5],α is called a rigid endomorphism if rα(r)=0 implies r=0 for r∈R. A ring R is said to be α-rigid if there exists a rigid endomorphism α of R.Clearly,any rigid endomorphism is a monomorphism and any α-rigid ring is reduced.Rigid endomorphisms were discussed in[5–7].In[8],a ring endomorphism α of R is called a weakly rigid endomorphism if α is a monomorphism and if there exist a,b∈R such that ab=0 then aα(b)=α(a)b=0.Clearly the identity map of R is weakly rigid.Every monomorphism of rings without non-zero zero-divisors is weakly rigid.

Let α be a rigid endomorphism of R.It was shown in[6]that if ab=0 then aαn(b)= αn(a)b=0 for any positive integer n.Thus any rigid endomorphism is weakly rigid.But the converse is not true(see,for example,[8]).By Proposition 3 of[8],α is rigid if and only if α is weakly rigid and R is reduced.Further examples of weakly rigid endomorphisms of rings can be found in[8]and[9].

Clearly,all weakly rigid endomorphisms and so,all rigid endomorphisms are compatible. Thus there exist many examples of compatible endomorphisms as shown in[5]–[9].

Lemma 2.1Letαbe compatible.Then

(1)αis a monomorphism,and

(2)for anye2=e∈R,α(e)=e.

Proof.Clearly,α is a monomorphism.Suppose that e2=e∈R.Then

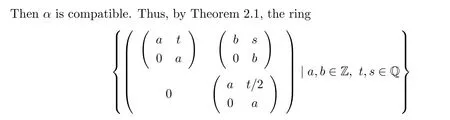
By Lemma 2.1 and by analogy with the proof of Lemma 2 in[1],we have the following more general result needed in Example 2.1.

Lemma 2.2LetR⊆Sbe an extension of rings such that every idempotent ofRcommutes with any element ofS.Suppose thatα2,···,αn∈REnd(R)are compatible.Then every idempotent in the ring

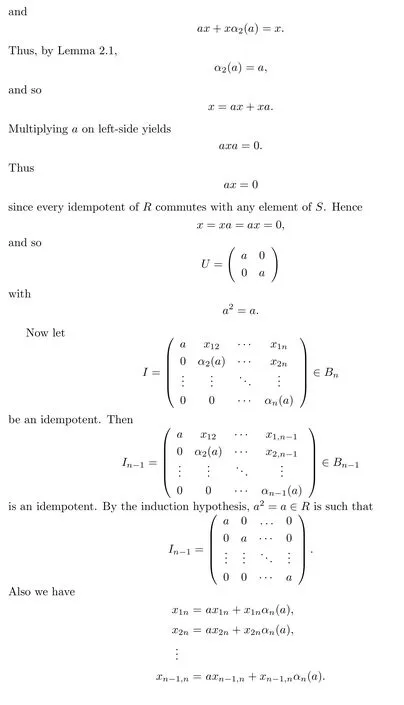
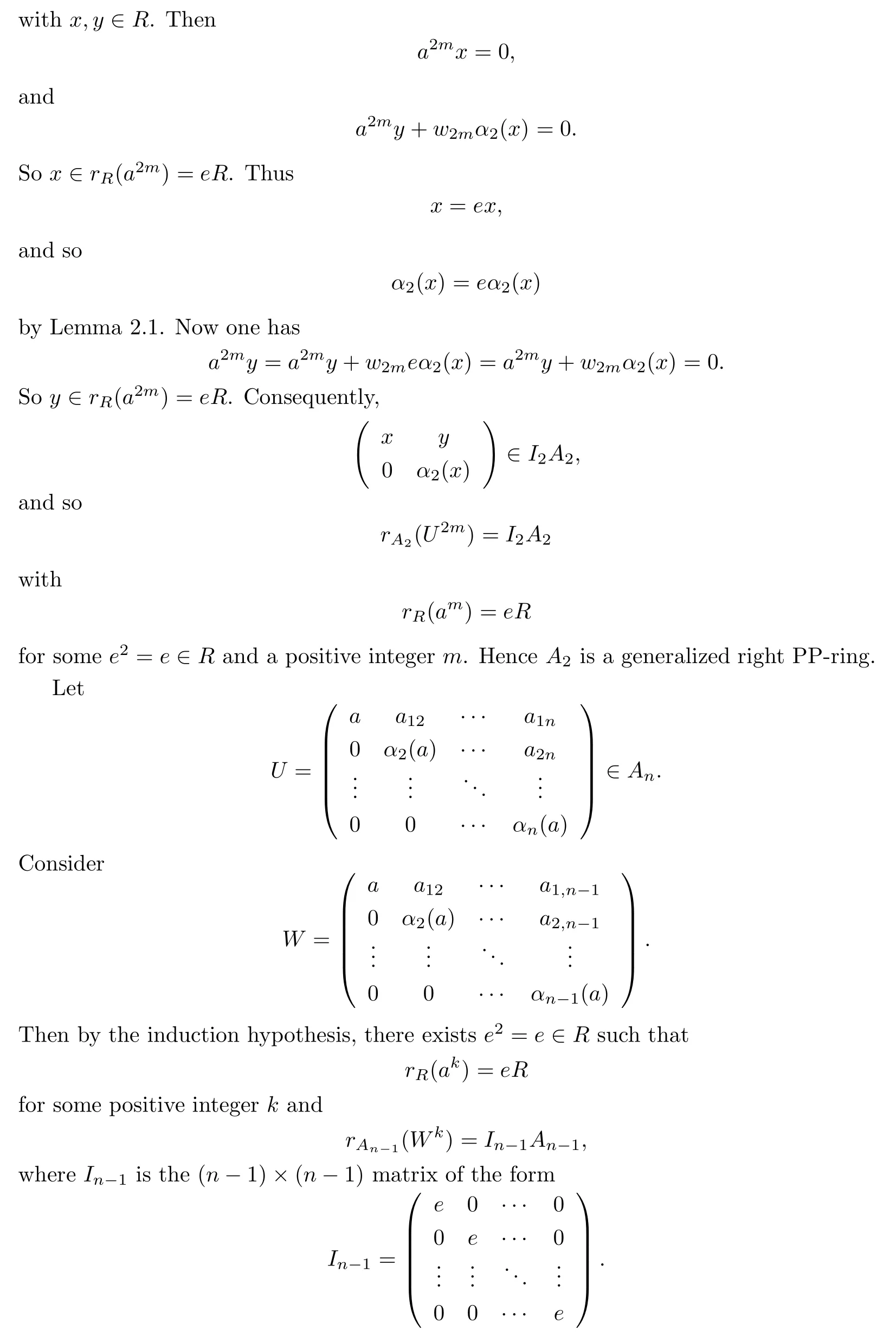
by Lemma 2.1.Thus xn−1,n∈rR(a2k)=eR.Multiplying the right hand side of the third equation by e yields

is a generalized right PP-ring.

A ring R is called right zip provided that if the right annihilator rR(X)of a subset X of R is zero then

for a finite subset Y⊆X.Examples and results of zip rings can be found in[2]and[11].In Theorem 5 of[2],it was shown that for a ring R,Snis a right(left)zip ring if and only if R is a right(left)zip ring.By analogy with the proof of Theorem 2.1 we have the following result.

Theorem 2.2LetRbe a ring andα2,···,αn∈REnd(R)be compatible.ThenRis a right(left)zip ring if and only ifAnis a right(left)zip ring.

[1]Hong,C.Y.,Kim,N.K.and Lee,Y.,p.p.-rings and generalized p.p.-rings,J.Pure Appl. Algebra,167(2002),37-52.

[2]Hong,C.Y.,Kim,N.K.,Kwak,T.K.and Lee,Y.,Extensions of zip rings,J.Pure Appl. Algebra,195(2005),231-242.

[3]Annin,S.,Associated and Attached Primes over Noncommutative Rings,Ph.D.Dissertation, University of California,Berkeley,2002,pp.129.

[4]Annin,S.,Associated prime over Ore polynomial rings,J.Algebra Appl.,3(2004),193-205.

[5]Krempa,J.,Some examples of reduced rings,Algebra Colloq.,3(1996),289-300.

[6]Hong,C.Y.,Kim,N.K.and Kwak,T.K.,Ore extensions of Baer and P.P.-rings,J.Pure Appl.Algebra,151(2000),215-226.

[7]Hong,C.Y.,Kim,N.K.and Kwak,T.K.,On skew Armendariz rings,Comm.Algebra, 31(2003),103-122.

[8]Liu,Z.K.and Fan,W.L.,Principal quasi-Baerness of skew power series rings,J.Math.Res. Exp.,25(2005),197-203.

[9]Liu,Z.K.,Triangular matrix representations of rings of generalized power series,Acta Math. Sin.,Engl.Ser.,22(2006),989-998.

[10]Ohori,M.,On noncommutative generalized p.p.rings,Math.J.Okayama Univ.,25(1984), 157-167.

[11]Faith,C.,Rings with zero intersection property on annihilators:zip rings,Publ.Mat., 33(1989),329-332.

Communicated by Du Xian-kun

16D15,16D40,16D25

A

1674-5647(2010)03-0193-10

date:Jan.19,2008.

The NSF(10961021)of China,TRAPOYT and NWNU-KJCXGC212.