展开方法及Fitzhugh-Nagumo方程新的孤波解
周建荣
(佛山科学技术学院 理学院数学系, 广东 佛山 528000)
周建荣
(佛山科学技术学院 理学院数学系, 广东 佛山 528000)
应用展开方法导出了Fitzhugh-Nagumo非线性方程新的孤波解.
孤波解;展开方法; Fitzhugh-Nagumo方程
近年来, 寻求非线性方程的行波解已引起人们的极大兴趣. 目前, 求解非线性方程比较成功的方法有齐次平衡法[5], 逆散射方法[2], Hopf-Cole变换法[12], Exp函数展开法[13], 双曲正切函数展开法[3,11], Sine-Cosine方法[15], 辅助方程法[14], 双曲函数法[4], 第一积分法[1,9]和双线性法[6], 等等.

新的孤波解, 其中δ为实常数.

这里的求导是关于波变量ξ.


应用方程(4), 从(3)式可得

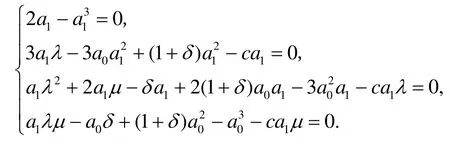
应用数学软件Maple解此方程组得三组解:
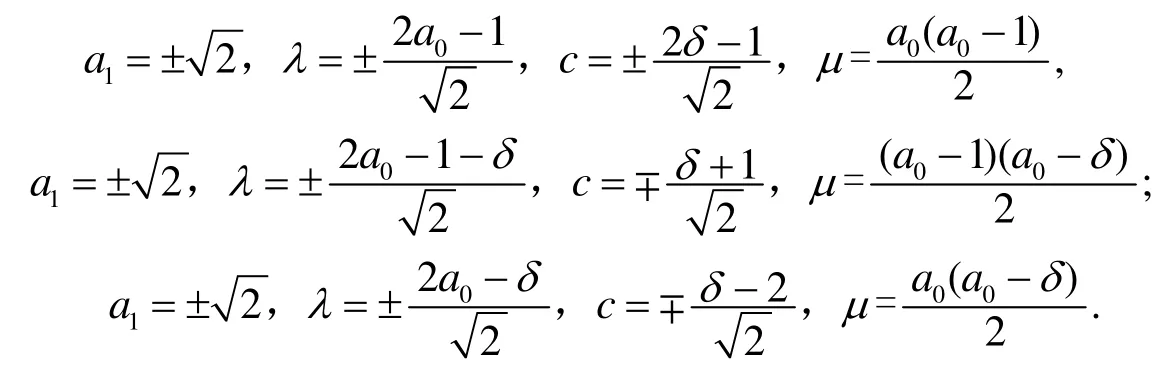



(6)~(13)式是在文[11]中通过Tanh-Coth方法已经得到的孤波解.
[1] S. Abbasbandy, A. Shirzadi.The first integral method for modified Benjamin–Bona–Mahony equation[J]. Commun Nonlinear Sci Numer Simulat 2010, (15): 1759~1764
[2] Ablowitz MJ, Clarkson PA.Solitons,nonlinear evolution equations and inverse scattering transfor[M]. Cambridge: Cambridge University Press, 1990
[3] Bekir A.Applications of the extended tanh method for coupled nonlinear evolution equations[J]. Commun Nonlinear Sci Numer Simulat 2008, 13(9): 1748~1757
[4] Chaofa Deng.New exact solutions to the Zakharov–Kuznetsov equation and its generalized form[J]. Commun Nonlinear Sci Numer Simulat 2010, 15: 857~868
[5] Fan E, Zhang H.A note on the homogeneous balance method[J]. Phys. Lett. A 1998, (246): 403~406
[6] Hirota R.Direct method of finding exact solutions of nonlinear evolution equations[J]. In: R. Bullough , P. Caudrey(Eds.), Backlund Transformations, Springer, Berlin 1980: 1157~1175
[7] Hai-Ling Lü, Xi-Qiang Liu, Lei Niu.A generalized-expansion method and its applications to nonlinear evolution equations[J].Applied Mathematics
and Computation 2010, (215): 3811~3816
[8] F. Tascan, A. Bekir.Travelling wave solutions of the Cahn-Allen equation by using first integral method[J]. Applied Mathematics and Computation 2009, 207: 279~282
[9] F. Tascan, A. Bekir, M. Koparan.Travelling wave solutions of nonlinear evolution equations by using the first integral method[J]. Communications in Nonlinear Science and Numerical Simulation 2009, 14: 1810~1815
[10] Wang ML, Li X, Zhang J.The-expansion method and traveling wave solutions of nonlinear evolution equations in mathematical physics[J]. Phys. Lett. A 2008, 372(4): 417~423
[11] Wazwaz AM.The tanh-coth method for solitons and kink solutions for nonlinear parabolic equations[J]. Appl. Math. Coptut. 2007, 188: 1467~1475
[12] Wazwaz AM.Multiple soliton solutions for a (2 + 1)-dimensional integrable KdV6 equation[J]. Commun Nonlinear Sci Numer Simulat 2010, 15: 1466~1472
[13] Xu F.Application of Exp-function method to symmetric regularized long wave (SRLW) equation[J]. Phys. Lett. A 2008, 372(3): 252~257
[14] Yunxi Guo, Shaoyong Lai.New exact solutions for an (N+1)-dimensional generalized Boussinesq equation[J]. Nonlinear Analysis 2010, 72: 2863~2873
[15] Yusufoglu E, Bekir A, A, Alp M.Periodic and solitary wave solutions of Kawahara and modified Kawahara equations by using sine-cosine method[J]. Chaos, Solitons & Fractals 2008, 37(4): 1193~1197
ZHOU Jian-rong
(Department of Mathematics, Foshan University, Foshan 528000, China)
The-expansion method is used to construct new solitary wave solutions of Fitzhugh-Nagumo equation.
solitary wave solutions;-expansion method; Fitzhugh-Nagumo equation
O175.29
A
1672-5298(2010)03-0021-03
2010-01-15
广东高校优秀青年创新人才培育项目(LYM08101)
周建荣(1978- ), 男, 湖南永州人, 博士, 佛山科学技术学院理学院数学系讲师. 主要研究方向: 渐近分析及非线性问题
———理学院

