Position Group Contribution Method for Predicting the Normal Boiling Point of Organic Compounds
WANG Qiang (王强), MA Peisheng (马沛生), WANG Chang (王昶) and XIA Shuqian (夏淑倩)
Position Group Contribution Method for Predicting the Normal Boiling Point of Organic Compounds
WANG Qiang (王强)1,2, MA Peisheng (马沛生)2, WANG Chang (王昶)1and XIA Shuqian (夏淑倩)2
1School of Material Science and Chemical Engineering, Tianjin University of Science and Technology, Tianjin 300457, China2School of Chemical Engineering, Tianjin University, Tianjin 300072, China
A new position group contribution model is proposed for the estimation of normal boiling data of organic compounds involving a carbon chain from C2to C18. The characteristic of this method is the use of position distribution function. It could distinguish most of isomers that include- or-structure from organic compounds. Contributions for hydrocarbons and hydrocarbon derivatives containing oxygen, nitrogen, chlorine, bromine and sulfur, are given. Compared with the predictions, results made use of the most common existing group contribution methods, the overall average absolute difference of boiling point predictions of 417 organic compounds is 4.2 K; and the average absolute percent derivation is 1.0%, which is compared with 12.3 K and 3.2% with the method of Joback, 12.1 K and 3.1% with the method of Constantinou-Gani. This new position contribution groups method is not only much more accurate but also has the advantages of simplicity and stability.
normal boiling point, prediction, position group contribution
1 Introduction
Boiling point is one of the most important thermal properties. Almost other thermo chemical properties are predictable from boiling point and critical constants [1-5], so the precise forecast of boiling point is much needed. Joback and Reid [6] proposed a group contribution method that gives an approximate value of the boiling point of aliphatic and aromatic hydrocarbons. The boiling point is estimated with the sum of contributions of all structural groups found in the molecule. Joback tested this method on 353 compounds. The average error was 16.8 K or 5.0% with standard a deviation of 17.9 K.
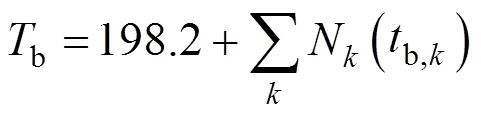
wherebis normal boiling point, K;Nis number ofgroups in molecule, (b,k) is Joback contribution of grouptob. Boiling point is used in the prediction of many other properties such as critical temperature. The errors inbwill be propagated to other properties calculated with it. It is recommended to get true value for this very important and fundamental property. Devotta and Pendyala [7] modified the Joback method to treatbof halogenated compounds much more accurately. They report that the average percent deviations for refrigerants and other substances was 12% in the original method.
Constantinou and Gani [8, 9] developed an advanced group contribution method based on the UNIFAC groups but were enhanced by allowing more sophisticated functions of the desired properties and by providing contributions at a ‘‘Second order’’ level forb, the C-G equations are

Here,b1,k,b2,jare contribution of groupandtob.
The first order results are generally good at boiling but not at melting. The second order contributions improve the agreement for all butfpfor the dimethylpentanes, where the correction goes in the wrong direction.
Marrero-Morejon and Pardillo-Fontdevila [10] give two equations for estimatingb. They call their preferred method a group interaction contribution technique. It can also be considered as a method of bond contributions. They tabulate contributions from 167 pairs of atoms alone or with hydrogen attached. Forb, their basic equation is

whereWis the molecular weight, andNis the number of atoms of typewith contributionsbbk.

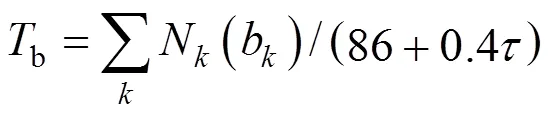
Table 1 Summary of comparison of estimation methods for Tb [14]
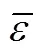
The advantages of group contribution methods are simple and general. Since organic compounds used in chemical industry consist about only 100 groups, group contribution methods can be applied to a great real number of substances. However, most of group contribution methods have serious problems that they cannot distinguish from structural isomers because the isomers have the same number and kind of groups so that the calculated results are inevitably the same.
This work proposes a new method for estimating the physical properties such as normal boiling point, and defining and utilizing the position parameter of group in organics structure that the most accurate existing method used for predicting the normal boiling point parameters of formation.
2 Experimental data
Total of 417 compounds containing carbon, hydrogen, oxygen, nitrogen, chlorine, bromine, and sulfur were used for the determination of group contributions. The complete list is given in the following table and includes linear and branched alkanes (146), cycloalkanes (26), alkenes (32), aromatics (26), alcohols (27), aldehydes and ketones (31), acids (12), phenols and ether oxides(14), esters (22), chloro and bromoalkanes (19), amines (27), nitriles (9), pyridines (10), thiols (14), and thioethers (17). The experiment data from the TRC Thermodynamic Tables [15] priority are used in calculation,which give the critical properties, normal boiling points, and melting point for a large number of hydrocarbons and derivatives; Ma and Poling’s handbooks [14, 16] provide a abundance of properties data about organic compound.
3 Computations
3.1 Calculated method
The first step consists testing correlations to represent the properties. Only one-parameter contribution was considered for each group. The normal boiling point function is constructed by all groups’ contribution, as well as position correction. Benson’s second order groups [17] and a few of third order groups [14] were applied into these works. Here, normal boiling point is expressed as follows [18-20]
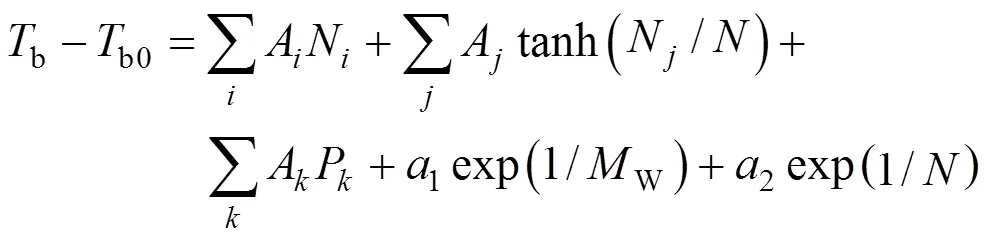
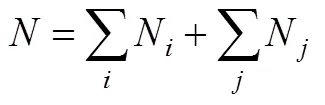
ParameterAorArevealsorgroup contributions, and the set of contributions that allowed minimizing the residual estimation error were then computed by regressive simulation.Nrepresents the number of groups that carbon element forms the center of the group,Nrepresents the number of groups that noncarbon element forms the center.is total number of groups.Pcharacterizes position factor [18].b0is 4775.5907 K,Wis molecular weight.
Not only were group contributions considered here but also corrections used by position to take into account longer distance interactions were considered. Some corrections for interactions through benzene or pyridine rings were obtained, resulting in a better distinction between series of chain-branched aromatic isomers. Parameters for five-membered and six-membered saturated hydrocarbon rings were also determined. We retained the correlations that were found to statistically give the best estimations.
3.2 Application samples
The examples ofbcomputation for the organics is given as follows.
Example 1 Estimation of the boiling point of 2,3,4,4-tetramethylhexane:
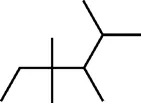
This compound is decomposed in position groups as follows:


Molecular weight: 142.285
From the contributions in Table 2, the normal boiling point is estimated by Eq. (5):

Hence, the calculated result is 430.4 K, whereas the experimental boiling point is 434.75 K.
Example 2 Estimation of the boiling point of 3-heptanol:
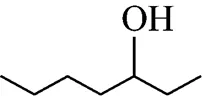
This compound is decomposed in position groups as follows:

The position of (OH) group: the compensated factor is 3
Molecular weight: 116.203
From the contributions in Table 2, the normal boiling point is calculated by Eq. (5):

Hence, the calculated result is 431.95 K, whereas the experimental boiling point is 425.15 K.
Example 3 Estimation of the boiling point of-1,3-dimethylcyclohexane

This compound could be decomposed in position groups as follows:
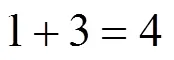
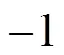
1 cyclohexane correction
From the contributions in Table 1, the critical temperature is estimated by Eq. (5):
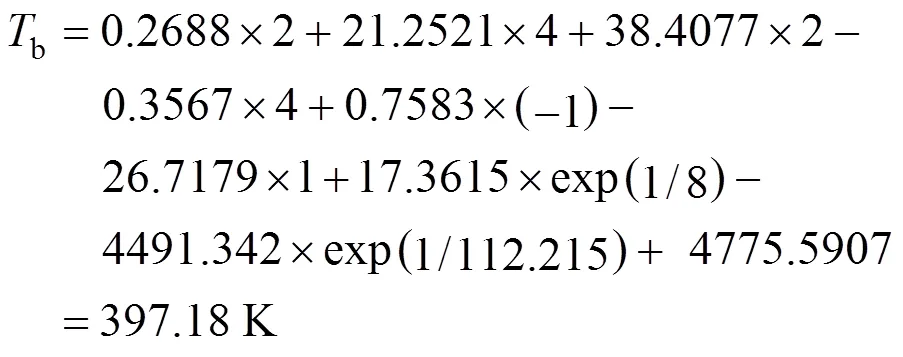
Hence, the calculated result is 397.18 K, whereas the experimental boiling point is 397.61 K.
4 Results and discussion

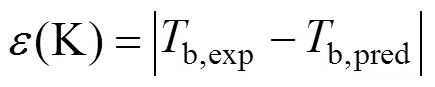



The excellent results were obtained for all the chemical families that were under investigation. The mean estimation error being of 4.2 K12.3 K obtained the use of Joback’s correlation. This confirms the greater precision of methods based on second order Benson’s groups for thermochemical data prediction. The second order method of Constantinou and Gani resulted in a mean error of 12.1 K, slightly higher than the accuracy claimed by these authors. It should also be pointed out that Constantinou’sbestimation method gives higher discrepancies than Joback’s for the pyridine derivatives, the amines, and the nitriles, indicating that some contributions need to be revised.
The prediction value in Table 3 indicates that the normal boiling temperature can be described by Eq. (5) very well. Its relation coefficient is 0.9935, and then, we can obtain confident level from incomplete beta function, which can be calculated from incomplete Gamma function. The confidence level is as high as 0.9990, which is the most significance level.
One complementary sets of position group contributions were developed for the predictive estimation of normal boiling point of organic compounds. The second order groups is defined by Benson, whose ideas have been recognized for a long time as more accurate than first order groups for thermochemical predictions, which were used for this purpose. Position factor could distinguish most isomers that include- or-structure of organic compounds for their properties. Contributions for compounds containing carbon, hydrogen, oxygen, nitrogen, sulfur, chlorine, and bromine were reported, and results were evaluated with regard to predictive methods developed previously. Normal boiling point predictions exhibit an averagedeviation of about 4.2 K, less than three times of the well known first order method of Joback and Reid and more than three times of the more precise second order method of Constantinou and Gani.
This method also can be applied for estimation of critical properties of organics effectively [18-20].

Table 2 Position group contributions for the prediction of Tb
①- and-corrections consider interactions between alkyl chains through a benzene ring.
②Corrections for pyridines:,, andpyridine corrections take into account alkyl ligands in position,, andwith respect to the N element, respectively.

Table 3 Comparison of Tb predicted with our method and with the methods of Joback and Constantinou
5 Conclusions
It is proposed that a quantitative relationship for normal boiling point based on position group contribution method and that position distribution function has been developed, which could distinguish most isomers including- or-structure of organic compounds for their thermodynamics properties. The estimated results for 417 different types of organics show that the position group contribution method is more precise than an existing method that uses first or second order groups.
1 Sanghvi, R., Yalkowsky, S.H., “Estimation of normal boiling point of organic compounds”,...., 45, 2856-2861 (2006).
2 Tadeusz, H., “Prediction of thermodynamic properties of the systems formed by-alkanes, aliphatic monoethers, and I-chloralkanes using a cell-hole group contribution model”,..., 108, 2383-2397 (2004).
3 Rebelo, L.P., Canongia, J.N., Esperanca, J.M., Filipe, E., “On the critical temperature normal boiling point and vapor pressure of ionic liquids”,..., 109, 6040-6043 (2005).
4 Valderrama, J.D., Robles, P.A., “Critical properties, normal boiling temperature and acentric factors of fifty ionic liquids”,...., 46, 1338-1344 (2007).
5 Dearden, J.C., “Quantitative structure-property relationships for prediction of boiling point, vapor pressure and melting point”,..., 22, 1696 (2003).
6 Joback, K.G., Reid, R., “Estimation of pure-component properties from group-contributions”,..., 57, 233-243 (1987).
7 Davotta, S., Pendyala, V.R., “Modified Joback group contribution method for normal boiling point of aliphatic halogenated compounds”,...., 31, 2042-2046 (1992).
8 Constantinou, L., Pricken, S.E., Mavrovouniotis, M.L., “Estimation of thermodynamic and physical properties of acyclic hydrocarbons using the ABC approach and conjugation operators”,...., 32, 1734-1742 (1993).
9 Constantinou, L., Gani, R., “A new group contribution method for estimation of properties of pure compounds”,., 40, 1697-1710 (1994).
10 Marrero-Morejon, J., Pardillo-Fontdevila, E., “Estimation of pure compound properties using group-interaction contributions”,., 45, 615-621 (1999).
11 Krzyzaniak, J.F., Myrdal, P., Simamora, P., Yalkowsky, S.H., “Boiling point and melting point prediction for alphatic nonhydrogen bonding compounds”,...., 34, 2530-2535 (1995).
12 Yalkowsky, S.H., Danmenfelser, P., Myrdal, P., Simamora, P., “Unified physical property estimation relationships”,, 28, 1657 (1994).
13 Zhao, L., Yalkowsky, S.H., “A combined group contribution and molecular geometry approach for predicting melting point of alphatic compounds”,...., 38, 3581 (1999).
14 Poling, E.B., Prausnitz, J.M., O’Connell, P.J., “The Properties of Gases and Liquids, 5th ed.., McGraw-Hill, Inc., New York (2001).
15 Frenkel, M., Gadalla, N.M., Hall, K.R., Hong, X., Marsh, K.N., Wilhoit, R.C., TRC Thermodynamic Tables: Hydrocarbon; Non-Hydrocarbon, Thermodynamic Research Center, The Texas A&M University System (1997).
16 Ma, P.S., Handbook of Property Data of Organic Compound, Chemical Industry Press, Beijing (2006).
17 Benson, S.W., Thermochemical Kinetics, 2nd ed., John Wiley and Sons, New York (1976).
18 Wang, Q., Ma, P.S., “Position group contribution method for the prediction of critical temperatures of organic compounds”,..., 53, 1103-1109 (2008).
19 Wang, Q., Ma, P.S., “Position group contribution method for the prediction of critical pressure of organic compounds”,..., 53, 1877-1885 (2008).
20 Jia, Q.Z., Wang, Q., Ma, P.S., “Position group contribution method for the prediction of critical volume of organic compounds”,..., 53, 2606-2612 (2008).
2008-09-23,
2008-12-13.
* To whom correspondence should be addressed. E-mail: wang_q@tust.edu.cn
 Chinese Journal of Chemical Engineering2009年2期
Chinese Journal of Chemical Engineering2009年2期
- Chinese Journal of Chemical Engineering的其它文章
- Kinetics of Reactive Extraction of Nd from Nd2O3 with TBP-HNO3Complex in Supercritical Carbon Dioxide*
- Activity Coefficient Models to Describe Vapor-Liquid Equilibrium in Ternary Hydro-Alcoholic Solutions*
- Experimental Study on the Initial Position Distribution of Taylor Bubbles in Cryogenic Upward Inclined Tubes*
- Enhancement of Proton Exchange Membrane Fuel Cell Performance Using a Novel Tapered Gas Channel*
- Tert-butylation of Toluene with Tert-butyl Alcohol over Realuminated H-mordenite Zeolite*
- A Fully Flexible Potential Model for Carbon Dioxide*
