一类双曲守恒律方程组退化Goursat问题整体光滑解的存在性

赵佳敏　肖伟

摘要： 针对一类双曲守恒律方程组退化Goursat问题， 研究其整体光滑解的存在性. 首先， 引入特征角α，β， 建立α，β和压力P的特征分解; 其次， 利用α，β的特征分解得到不变区域， 进而得到特征角的最大模估计； 最后， 通过压力P的特征分解以及连续性方法建立解的梯度估计， 从而证明退化Goursat问题解的存在性.

关键词： 双曲守恒律方程组； 特征分解； 退化Goursat问题； 平面稀疏波

中图分类号： O175.2文献标志码： A文章编号： 1671-5489（2024）02-0197-08

Existence of  Global Smooth Solutions for Degenerate Goursat Problem of a Class of Hyperbolic Conservation Law Systems

ZHAO Jiamin， XIAO Wei

（School of Sciences， Changan University， Xian 710064， China）

Abstract： We studied the existence of the global smooth solutions for degenerate Gourset problem of a class of hyperbolic conversation law systems. Firstly， we introduced  characteristic angles α，β， and established characteristic decompositions for α，β and pressure P. Secondly， the characteristic decompositions of  α，β were used to obtain the invariant region， and then the maximum norm estimate of the characteristic angles were obtained. Finally， the gradient estimates of the solution were established by the characteristic decomposition of pressure P and continuity method， which proved the existence of the solutions to the degenerate Gourset problem.

Keywords：  hyperbolic conservation law system; characteristic decomposition; degenerate Goursat problem; planar rarefaction wave

1 引言與预备知识

非线性双曲守恒律方程组来源于物理和力学等中的许多自然现象. 其中二维Riemann问题的研究在理论和实际中均具有重要意义. 对于Euler方程的二维Riemann问题， 文献［1］基于广义特征分析法和数值实验提出了一系列猜想. 但跨音速结构的存在以及小尺度结构［2-3］使得该类问题的解更复杂. 文献［4-5］根据对称性避免了这些结构， 得到了一些有意义的结果. 对于Goursat问题， Barthwal等［6］为得到对应二维Riemann问题直到真空边界的全局解的存在性， 考虑Euler方程组Goursat型边值问题， 并在文献［7］中通过特征分解和自举法的思想得到了简单波和平面稀疏波相互作用相应的二维Riemann问题在真空边界处解的整体存在性； Dai等［8］考虑气体动力学压差方程的Goursat问题， 证明了退化真空Goursat问题整体光滑解的存在性； Hu等［9］利用文献［8］的方法将非线性波动方程局部解延拓为整体解.

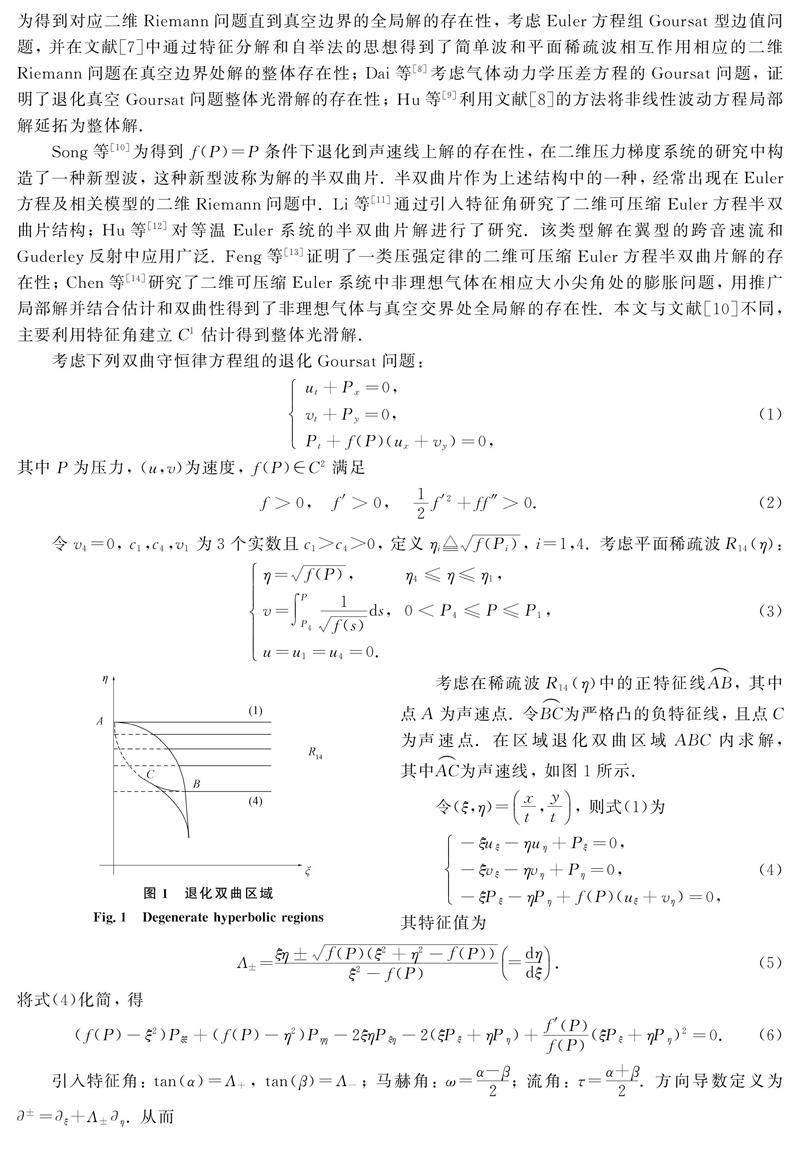
Song等［10］为得到f（P）=P条件下退化到声速线上解的存在性， 在二维压力梯度系统的研究中构造了一种新型波， 这种新型波称为解的半双曲片. 半双曲片作为上述结构中的一种， 经常出现在Euler方程及相关模型的二维Riemann问题中. Li等［11］通过引入特征角研究了二维可压缩Euler方程半双曲片结构; Hu等［12］对等温Euler系统的半双曲片解进行了研究. 该类型解在翼型的跨音速流和Guderley反射中应用广泛. Feng等［13］证明了一类压强定律的二维可压缩Euler方程半双曲片解的存在性； Chen等［14］研究了二维可压缩Euler系统中非理想气体在相应大小尖角处的膨胀问题， 用推广局部解并结合估计和双曲性得到了非理想气体与真空交界处全局解的存在性. 本文与文献［10］不同， 主要利用特征角建立C1估计得到整体光滑解.

参考文献

［1］ZHANG T， ZHENG Y X. Conjecture on the Structure of Solutions of the Riemann Problem for Two-Dimensional Gas Dynamics Systems ［J］. SIAM J Math Anal， 1990， 21（3）： 593-630.

［2］GLIMM J， JI X M， LI J Q， et al. Transonic Shock Formation in a Rarefaction Riemann Problem for the 2D Compressible Euler Equations ［J］. SIAM J Appl Math， 2008， 69（3）： 720-742.

［3］LAX P D， LIU X D. Solution of Two-Dimensional Riemann Problems of Gas Dynamics by Positive Schemes ［J］. SIAM J Sci Comput， 1998， 19（2）： 319-340.

［4］LI J Q. On the Two-Dimensional Gas Expansion for Compressible Euler Equations ［J］. SIAM J Math Anal， 2001， 62（3）： 831-852.

［5］ZHANG T， ZHENG Y X. Axisymmetric Solutions of the Euler Equations for Polytropic Gases ［J］. Arch Rational Mech Anal， 1998， 142（3）： 253-279.

［6］BARTHWAL R， RAJA SEKHAR T. On the Existence and Regularity of Solutions of Semihyperbolic Patches to 2-D Euler Equations with van Der Waals Gas ［J］. Stud Appl Math， 2022， 148（2）： 543-576.

［7］BARTHWAL R， SEKHAR T R. Existence of Solutions to Gas Expansion Problem through a Sharp Corner for 2-D Euler Equations with General Equation of State ［J］. Stud Appl Math， 2023， 151（1）： 141-170.

［8］DAI Z H， ZHANG T. Existence of a Global Smooth Solution for a Degenerate Goursat Problem of Gas Dynamics ［J］. Arch Rational Mech Anal， 2000， 155（4）： 277-298.

［9］HU Y B， WANG G D. Semi-hyperbolic Patches of Solutions to the Two-Dimensional Nonlinear Wave System for Chaplygin Gases ［J］. J Differential Equations， 2014， 257（5）： 1567-1590.

［10］SONG K， ZHENG Y X. Semi-hyperbolic Patches of Solutions of the Pressure Gradient System ［J］. Discrete Contin Dyn Syst （Ser A）， 2009， 24（4）： 1365-1380.

［11］LI M J， ZHENG Y X. Semi-hyperbolic Patches of Solutions to the Two-Dimensional Euler Equations ［J］. Arch Rational Mech Anal， 2011， 201（3）： 1069-1096.

［12］HU Y B， LI J Q， SHENG W C. Degenerate Goursat-Type Boundary Value Problems Arising from the Study of Two-Dimensional Isothermal Euler Equations ［J］. Z Angew Math Phys， 2012， 63（6）： 1021-1046.

［13］FENG M， XIAO W. On the Existence of Solutions of Semi-hyperbolic Patches to Two-Dimensional Euler Equations for a Class of Pressure Laws ［J］. Anal Math Phys， 2022， 12（4）： 98-1-98-18.

［14］CHEN J J， SHEN Z M， YIN G. The Expansion of a Non-ideal Gas Around a Sharp Corner for 2-D Compressible Euler System ［J］. Math Methods Appl Sci， 2023， 46（2）： 2023-2041.

［15］COURANT R， FRIEDRICHS K O. Supersonic Flow and Shock Waves ［M］. New York： Interscience Publishers， Inc， 1948： 399-401.

［16］王柔懷， 伍卓群. 二自变量准线性双曲型方程组的若干定解问题解的存在与唯一性 ［J］. 吉林大学自然科学学报， 1963（2）： 459-502. （WANG R H， WU Z Q. On Mixed Initial Boundary Value Problem for Quasi-linear Hyperbolic System of Partial Differential Equations in Two Independent Variables ［J］. Acta Scientiarum Naturalium Universitatis Jilinensis， 1963（2）： 459-502.）

（责任编辑： 赵立芹）

收稿日期： 2023-06-27.

第一作者简介： 赵佳敏（1998—）， 女， 汉族， 硕士研究生， 从事偏微分方程的研究， E-mail： 1362531542@qq.com.

通信作者简介： 肖 伟（1983—）， 男， 汉族， 博士， 副教授， 从事偏微分方程的研究， E-mail： xiaowei1802002@chd.edu.cn.

基金项目： 陕西省自然科学基础研究计划项目（批准号： 2018JQ1084）、 陕西省重点研发计划项目（批准号： 2019GY202）和长安大学中央高校基本科研业务费专项基金（批准号： 300102121101）.