Residual stress modeling of mitigated fused silica damage sites with CO2 laser annealing
Chuanchao Zhang(张传超), Wei Liao(廖威), Lijuan Zhang(张丽娟),Xiaolong Jiang(蒋晓龙), Zhenhua Fang(方振华), and Xiaodong Jiang(蒋晓东)
Laser Fusion Research Center,China Academy of Engineering Physics,Mianyang 621900,China
Keywords: fused silica,damage mitigation,residual stress,laser annealing
1.Introduction
High-quality fused silica can be produced at large scales and is widely used for the manufacture of large and expensive optical components such as windows,lenses and gratings suitable for high-power laser systems.Over the past decades,despite significant progress having been made in increasing the damage resistance of fused silica optics operating at UV wavelengths,laser-induced surface damage of fused silica optics is still a major issue for high-power laser systems.[1-3]Although the surface damage sites initially have a diameter of the order of tens of microns,the size of such sites increases exponentially with subsequent high-fluence nanosecond UV laser shots, which limits the durability of optical components for high-power laser systems.[4,5]To avoid growth of the damage site,localized CO2laser melting of fused silica has been used to mitigate surface damage sites on fused silica optics.[6-9]However, thermal quenching following the melting of damage sites can accumulate a significant amount of residual stress due to the finite relaxation times near the glass transition of fused silica,which may cause critical fracture to relieve stress throughout the heat-treated region.[10]CO2laser annealing by a linear ramp-down of laser power after damage mitigation has been demonstrated and applied to avoid rapid cooling down,which minimizes the residual stress to an acceptable level,avoiding critical fracture.[11-13]
Accurate description of residual stress of mitigated fused silica damage sites for quenched and laser-annealed cases is of major interest for optimization of the damage mitigation process.The nondestructive photoelastic method is generally used to characterize stress in transparent materials.The measured retardance value is associated with the stress level to be evaluated.But the residual stress of mitigated damage sites is nonuniform, and there is no direct proportionality between the retardance and the stress level of a mitigated damage site.Thus, the stress distribution and quantitative values of stress levels of a mitigated fused silica damage site cannot be directly evaluated by the photoelastic method.[14]In order to assess the residual stress distribution of mitigated fused silica damage sites, various numerical models of CO2laser interaction with fused silica, which take into account multiphysics processes and temperature-dependent physical properties in the whole thermal history during localized CO2laser treatment of fused silica, have been developed to quantify the residual stress of mitigated damage sites.Gallaiset al.regarded the residual stress of mitigated damage sites as the imprinted thermal stress induced by temperatures below a limit between 1100°C and 1600°C after heating.[14]Matthewset al.indicated that the magnitude of the final residual tensile stress of mitigated damage sites is nearly equal to the maximum compressive stress prior to cool-down.[12]Vigneset alpresented a multiphysics finite-element model to analyze the residual stress of mitigated damage sites considering viscous flowing,quenching of the material and structural relaxation.[15]These reported numerical models have been successfully used to evaluate the residual stress distribution of quench-cooled mitigated damage sites.For CO2laser annealing, however, the mitigated damage site shows a more complex thermal history, meaning that the reported numerical models inaccurately predict the residual stress distribution of laser-annealed mitigated damage sites.Matthewset al.reported that the calculated value for the maximum hoop stress of mitigated damage sites with CO2laser annealing is 41%larger than the value from critical fracture experiments, while the deviation between the calculated and experimental values for the maximum hoop stress of quench-cooled mitigated damage sites is only 6%.[12]Hence,the ability to accurately assess the residual stresses of laserannealed mitigated damage sites is still a challenge.
In this study, a numerical model based on the measured fictive temperature distribution of the mitigated damage sites is explored to evaluate the distribution and quantitative values of the residual stress of CO2laser-annealed mitigated fused silica damage sites.The fictive temperature of a glass,which is defined as the temperature of the equilibrium melt whose structure is equivalent to that of the cooled glass, is a convenient tool in describing glass structural relaxation phenomena.[16]The proposed model assumes that the initial frozen-in state of fused silica is the zero-point of residual stresses in the melt zone, and the generation of residual stresses results from the differences in thermoelastic contraction of fused silica with different fictive temperatures from the initial frozen-in temperature to ambient temperature, and has succeeded in evaluating the nonuniform residual stress fields of quench-cooled mitigated damage sites.[17]Spatially resolved fictive temperatures of mitigated damage sites for quenched and CO2laserannealed cases have been widely characterized using confocal Raman microscopy.[12,18]Based on the reported fictive temperature distribution,the residual stress fields of the CO2laserannealed mitigated damage sites were systematically analyzed by the proposed model in this study, and the calculated maximum hoop stress agrees well with the reported experimental result,demonstrating the suitability of the proposed model for describing the residual stresses of mitigated fused silica damage sites for CO2laser annealing.
2.Residual stress model based on fictive temperature
During the localized CO2laser melting of a fused silica damage site the melted fused silica is soft, and thermally induced stresses are released by structural relaxation.The melt zone persists in a zero-stress state until the laser is shut off,and rapid thermal quenching ensues.Due to the high cooling rate for the quenched case,the melt zone of the mitigated site is frozen-in at higher fictive temperatures with greater density.After collective cooling of fused silica, the melt zone undergoes intense residual tensile stresses,while CO2laser annealing by a linear ramp-down of laser power following damage mitigation can effectively suppress rapid cool-down and allow the melt zone to more fully relax.Thus, the mitigated damage site for the CO2laser-annealed case is frozen-in at a lower fictive temperature than for the quenched case, and therefore the residual stress is effectively minimized.[11-13]The axial fictive temperature distributions of mitigated damage sites for quenched and CO2laser-annealed cases have both been widely characterized.[12,18]
According to the reported data for mitigated damage sites quench-cooled at a peak temperature of 2300 K and mitigated damage sites laser-annealed by linear ramp from a maximum of 2300 K to ambient 300 K,[12]depth profiles of fictive temperatures on the axis of mitigated damage sites can be fitted as logistic curves
whereTfquenched(z)on-axis(in K) is the depth profile of on-axis fictive temperatures of mitigated damage sites quench-cooled at 2300 K peak temperature,Tfannealed(z)on-axis(in K) is the depth profile of on-axis fictive temperatures of mitigated damage sites laser-annealed by linear ramping from a maximum of 2300 K to ambient 300 K;zis measured in microns.
The two-dimensional (2D) axisymmetric fictive temperature distributions of mitigated damage sites result from isotropic thermal diffusion of the thermodynamic temperature field, and show an axisymmetric characteristic of a spherical cap.[17]Thus, the fictive temperature distributions can be mapped by the fictive temperature profiles along the axial depth.According to Eqs.(1) and (2) and the distribution characteristics of fictive temperatures for the mitigated damage sites in Ref.[17],the 2D axisymmetric fictive temperature distributions of mitigated damage sites can be described as
whereTfquenched(r,z) is the 2D axisymmetric fictive temperature distribution of mitigated damage sites quench-cooled at 2300 K peak temperature,Tfannealed(r,z) is the 2D axisymmetric fictive temperature distributions of mitigated damage sites laser-annealed by linear ramping from a maximum of 2300 K to ambient 300 K.The typical fictive temperature distributions of mitigated damage sites for both quenched and laser-annealed cases were artificially reconstructed according to Eqs.(3) and (4), and are shown in Fig.1, where it can be seen that CO2laser annealing can effectively abate the local fictive temperature modification of mitigated damage sites.
Before damage mitigation, the fictive temperature of the fused silica substrate is uniform with a value of 1315 K.After damage mitigation, the fictive temperature of the local melt zone increases, as shown in Fig.1, resulting in local densification and induction of tensile stresses on the surface of mitigated damage sites.The key of the proposed model for evaluating the residual stress of mitigated damage sites is that the relationship between residual strain and fictive temperature can be determined.According to the proposed model of residual stress fields analyzed from fictive temperature distributions for quench-cooled mitigated damage sites,[17]the difference between specific volume changes of fictive temperatureincreased fused silica and the substrate from the initial frozenin temperatures to the ambient temperature can be regarded as the residual thermal volume displacement as the body collectively cools.Therefore the residual thermal volume strain of fused silica with fictive temperatureTfis expressed as

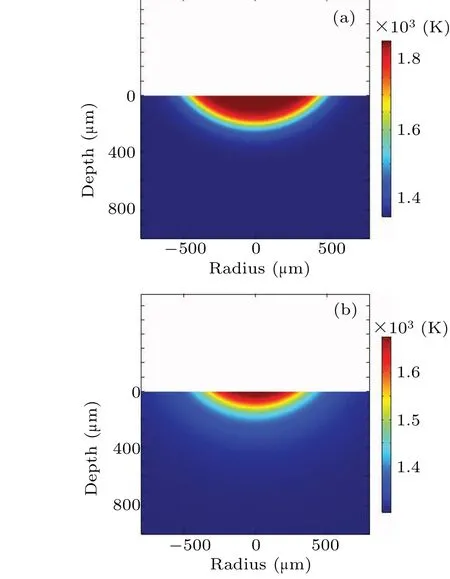
Fig.1.The artificial reconstruction of typical fictive temperature distributions of mitigated damage sites in Ref.[12] for mitigated damage sites quench-cooled at a peak temperature of 2300 K(a)and mitigated damage sites laser-annealed by linear ramping from a maximum of 2300 K to ambient 300 K(b).
According to the symmetry of this study, a 2D axisymmetric geometry is used to describe the residual stress as a function of the residual thermal strain of fictive temperatureincreased fused silica.Making use of the cylindrical coordinate system (r,θ,z) for axially symmetric problems,[19]the constitutive equations for the axisymmetric fictive temperature modification zone of mitigated damage sites are as follows:
where theεvalues are components of the strain,Eis Young’s modulus,Gis shear modulus andνis Poisson’s ratio.
Combining the equilibrium equations and constitutive equations, the residual stresses of mitigated damage sites for laser annealing can be obtained by commercial finite element analysis(FEA)software.The physical properties of fused silica used in the model are summarized in Table 1.
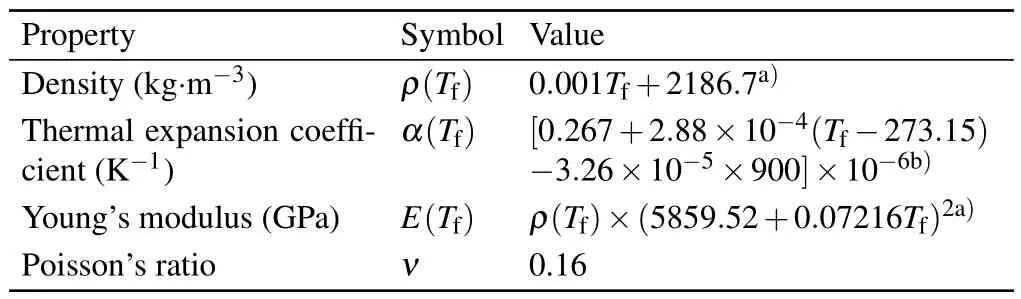
Table 1.Physical properties of fused silica used in the calculations.
3.Results and discussion
From Eqs.(3)-(6) and the equations of equilibrium for axially symmetric problems
where theσare components of the stress, the residual stress field of a mitigated damage site can be obtained by solving elastic equilibrium conditions and stress-strain relations.
The calculated hoop stress fields of mitigated damage sites for quenched and CO2laser-annealed cases based on fictive temperature distributions are shown in Fig.2,which indicates that the local fictive temperature modification zones of mitigated damage sites suffer intensive tensile stress.For the mitigated damage sites quench-cooled at a peak temperature of 2300 K, the hoop stresses remain almost constant on the melt surface.The hoop stress at the surface centerr=0 is 21.8 MPa and increases slowly to a maximum of 23.7 MPa atr=335 µm; with the radius further increasing, the hoop stress decreases rapidly and reaches a minimum of-2.4 MPa atr=660µm.For the mitigated damage sites laser-annealed by linear ramping from a maximum of 2300 K to ambient 300 K, the hoop stresses are almost constant at the melt surface center, and the hoop stress at the surface centerr=0 is 16.8 MPa and just slowly increases to a maximum of 17.2 MPa atr=150 µm; with the radius further increasing, the hoop stress gradually decreases to 0 atr=650µm.In comparison with the calculated hoop stresses at the melt surface center for quenched and CO2laser-annealed cases,CO2laser annealing can effectively suppress the maximum surface hoop stress.
On the axis, the tensile hoop stresses of quench-cooled mitigated damage sites dramatically increase from 21.8 MPa atz=0 to a maximum of 28.4 MPa atz=110µm,and then rapidly decrease to-4.5 MPa atz=330 µm.The axial tensile hoop stresses of laser-annealed mitigated damage sites increase from 16.8 MPa atz=0 to a maximum of 18.1 MPa atz=35 µm, and then gradually decrease to 0 atz=280 µm.It is interesting to note that the maximum hoop stresses of quench-cooled mitigated damage sites do not occur atz=0 on-axis, but in fact reside in regions below the surface atz=110µm,which is consistent with the results in Ref.[12].The maximum hoop stress of quench-cooled mitigated damage sites is 57% larger than that of laser-annealed mitigated damage sites.
The depth profiles of fictive temperatures and calculated hoop stresses on the axis of mitigated damage sites for quenched and CO2laser-annealed cases are plotted in Fig.3 for comparison.The quenched and laser-annealed cases both display a characteristic sigmoidal shape with nearly constantTfat the surface, and a mostly monotonic decrease into the bulk toTfini.It is obvious that the hoop stresses increase to the maximum value with depth of constantTfincreasing,and then decrease with the decrease of fictive temperatures.The slopes of hoop stresses are consistent with those of the corresponding fictive temperatures for quenched and CO2laser-annealed cases.According to the reported depth profiles of fictive temperatures for thermally quenched silica sites at peak temperatures of 1700 K and 2300 K in Ref.[12],the maximum hoop stresses for the mitigated damage sites quench-cooled at peak temperatures of 1700 K and 2300 K are also calculated by the proposed model.The calculated maximum hoop stresses in this study and the calculated and measured maximum hoop stresses in Ref.[12] are summarized in Table 2.The second column indicates maximum hoop stresses estimated from critical fracture experiments in Ref.[12].The third column indicates maximum hoop stresses simulated by the finite element analysis of the model in Ref.[12].The fourth column indicates calculated maximum hoop stresses using the proposed model in this work.
For the quenched case at a peak temperature of 1700 K,the maximum hoop stress 16.8 MPa calculated by the proposed model in this study is consistent with the experimental result of<18.7 MPa in Ref.[12], while the calculated maximum hoop stress of 26.7 MPa in Ref.[12]is much larger than the experimental result.For the quenched case at a peak temperature of 2300 K, both the simulated maximum hoop stresses in Ref.[12] and in this work are close to the experimental results, which indicates that the models used in Ref.[12] and in this work can both effectively evaluate the residual stress fields of mitigated damage sites quench-cooled at a peak temperature of 2300 K.Compared with the maximum hoop stresses for thermally quenched mitigated damage sites at 1700 K,2300 K and 3000 K calculated using the proposed model, it is obvious that the maximum hoop stresses increase with increasing quenched peak temperature, which agrees with the general experimental results.
For the laser-annealed case, the calculated maximum hoop stress in Ref.[12] is 41% larger than the experimental result,while the calculated maximum hoop stress in this study is just 5% less than the experimental result.Thus, the proposed model in this study can accurately evaluate the residual stress fields of mitigated damage sites for the laser-annealed case.The residual stress generation of mitigated damage sites is a multiphysics process involving viscous flowing, quenching of the material and structural relaxation.In addition, the physical properties of fused silica are temperature dependent.Thus, it is extremely difficult to precisely assess the residual stress directly from the thermal history of the localized CO2laser treatment of fused silica.The proposed model is just dependent on the measured fictive temperature distribution,and avoids complex thermodynamic processes.Therefore,the proposed model can accurately evaluate the residual stress fields of laser-annealed mitigated damage sites with a more complex thermal history.
The calculated radial stress fields of mitigated damage sites for quenched and CO2laser-annealed cases based on fictive temperature distributions are shown in Fig.4.The distributions of radial stresses are significantly different from those of hoop stresses.The tensile radial stresses on the surface extend far beyond the local fictive temperature modification zone.For the mitigated damage sites quench-cooled at a peak temperature of 2300 K, the surface radial stresses rapidly increase from 21.8 MPa atr=0 to a maximum of 35.8 MPa atr=335µm,and then gradually decrease to zero at radii larger than 2 mm.For the mitigated damage sites laser-annealed by linear ramping from a maximum of 2300 K to ambient 300 K,the radial stresses just slowly increase from 16.8 MPa atr=0 to a maximum of 19.5 MPa atr=245µm,and then gradually decrease to zero at radii larger than 2 mm.Compared with the fictive temperature distributions in Fig.1,the maximum residual radial stresses reside in the border regions of local fictive temperature modification zones,where the slope of the fictive temperature profiles is larger.The maximum radial stress of quench-cooled mitigated damage sites is 84%larger than that of laser-annealed mitigated damage sites, which shows that CO2laser annealing can effectively suppress the maximum radial stress.
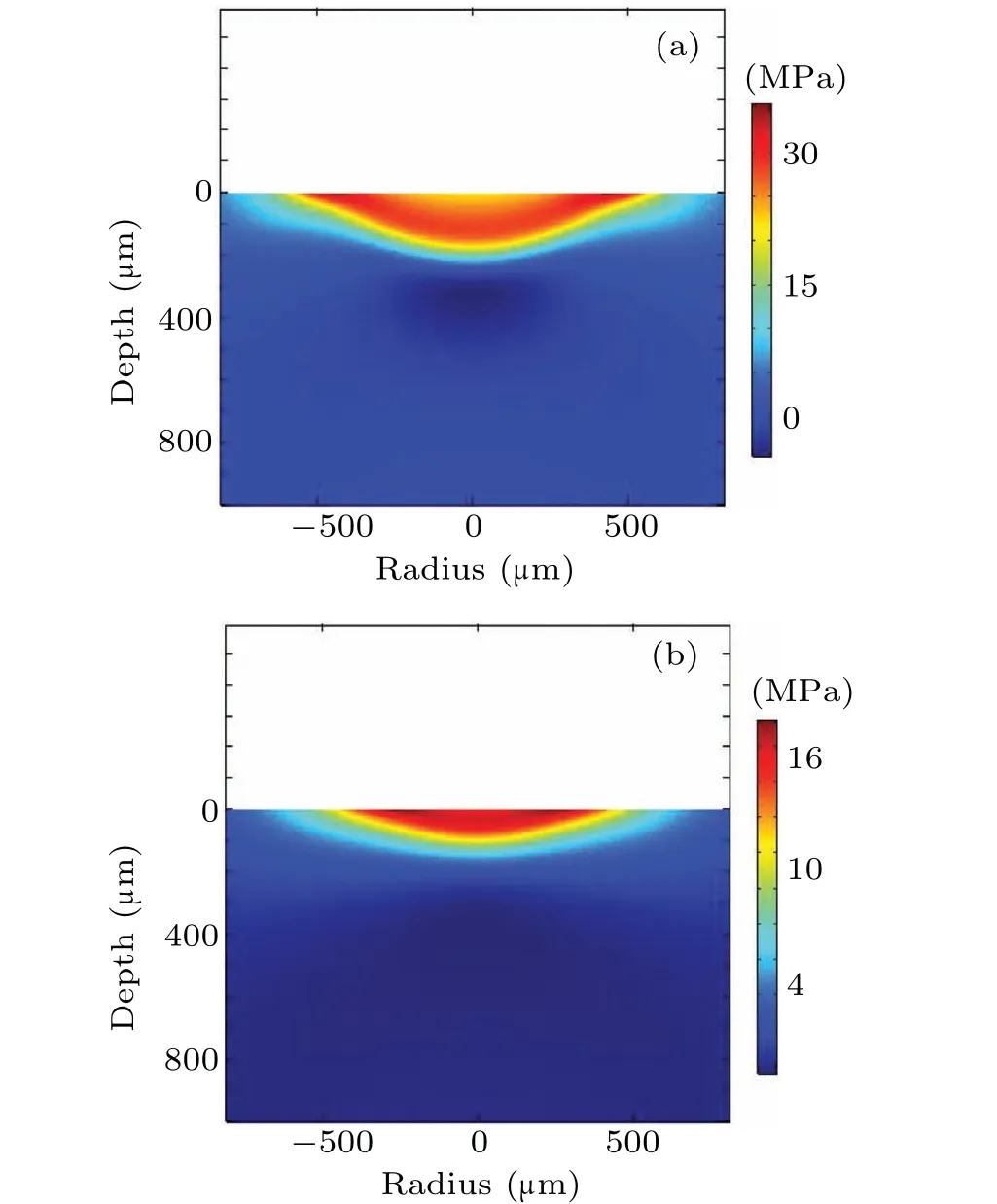
Fig.4.Calculated radial stresses σrr of mitigated damage sites for mitigated damage sites quench-cooled at a peak temperature of 2300 K(a) and mitigated damage sites laser-annealed by linear ramping from a maximum of 2300 K to ambient 300 K (b).A positive value for the stress indicates tension.
According to the residual radial and hoop stress fields,it is convenient to assess the optical retardance caused by stressinduced birefringence.The calculated residual radial and hoop stress fields have an azimuthal symmetry.The directions of principal stresses are parallel or orthogonal to the radius.The relative optical retardation between waves polarized in the radial direction and in the tangential direction can be obtained by the integrated principal stress difference along thezdirection in this study,and can be calculated by[17,22]
whereKis the stress-optic coefficient of fused silica and is equal to 35 nm·cm-1·MPa-1, anddis the thickness of fused silica sample.
Figure 5 shows the radial profiles of optical retardance for a light beam traveling through the thickness of mitigated damage sites for quenched and laser annealed cases,which are calculated from the simulated residual hoop and radial stress fields in Figs.2 and 4.The radial characteristic shape of the optical retardance for quench-cooled mitigated damage sites is in agreement with the reported measurement.[23]CO2laser annealing can effectively reduce the optical retardance of mitigated damage sites.The slope of the radial optical retardance profile of the laser-annealed mitigated damage sites dramatically decreases compared with that of quench-cooled mitigated damage sites.

Fig.5.The calculated radial profiles of optical retardance for a light beam traveling through the thickness of mitigated damage sites induced by the simulated residual radial and hoop stress fields for quenched and laser-annealed cases.
4.Conclusion
A numerical model based on the measured fictive temperature distribution of mitigated damage sites is explored to evaluate the quantitative values of the residual stress fields of CO2laser-annealed mitigated fused silica damage sites.The proposed model extracts the residual strain from the differences in thermoelastic contraction of fused silica with different fictive temperatures from initial frozen-in temperatures to the ambient temperature,and the residual stress fields of mitigated damage sites for the quenched and CO2laser-annealed cases are obtained by a finite element analysis of equilibrium equations and constitutive equations.The simulated results indicate that the proposed model can accurately evaluate the residual stress fields of laser-annealed mitigated damage sites with a more complex thermal history.The calculated maximum hoop stress is in good agreement with reported experimental results.The maximum hoop stress of quench-cooled mitigated damage sites is 57%larger than that of laser-annealed mitigated damage sites.The maximum radial stress of quench-cooled mitigated damage sites is 84%larger than that of laser-annealed mitigated damage sites.CO2laser annealing can effectively suppress the maximum residual stresses.The estimated optical retardance profiles from the calculated radial and hoop stress fields are consistent with photoelastic measurements.These results provide sufficient evidence to demonstrate the suitability of the proposed model to describe the residual stresses of mitigated fused silica damage sites for CO2laser annealing.
Acknowledgement
Project supported by the National Natural Science Foundation of China(Grant No.62275235).
- Chinese Physics B的其它文章
- Does the Hartman effect exist in triangular barriers
- Quantum geometric tensor and the topological characterization of the extended Su–Schrieffer–Heeger model
- A lightweight symmetric image encryption cryptosystem in wavelet domain based on an improved sine map
- Effects of drive imbalance on the particle emission from a Bose–Einstein condensate in a one-dimensional lattice
- A new quantum key distribution resource allocation and routing optimization scheme
- Coexistence behavior of asymmetric attractors in hyperbolic-type memristive Hopfield neural network and its application in image encryption