Relations between SMBH Parameters and Jet Generation and Efficiency in Blazars
H.Zhang and H.J.Zhang
Department of Physics, Yunnan Normal University, Kunming 650092, China; kmzhanghj@163.com
Abstract We analyzed the relationship between several basic parameters describing supermassive black holes such as jet power, black hole spin, accretion disk magnetic field, black hole mass, etc.We found that there is a general correlation between these parameters, such as jet power is significantly positively correlated with black hole spin,while black hole mass is significantly negatively correlated with black hole spin.To apprehend these relationships,we consider the Blandford–Znajek model to be superior to the Blandford–Payne model.It is also found that the intrinsic gamma luminosity of the FSRQs has a positive correlation with the accretion disk magnetic field, while the intrinsic gamma luminosity of the BL Lacs has a negative correlation with the accretion disk magnetic field.A feedback effect may exist between accretion disk accretion rate and magnetic field, which may be the key to the evolution between BL Lacs and FSRQs.There is no significant difference in the jet power and jet generation efficiency of FSRQs and BL Lacs, which suggests that the jets are generated by the same mechanism.The contribution rate of accretion rate to jet generation efficiency is high,while the contribution rate of accretion rate to jet power is very low.
Key words: galaxies: evolution – galaxies: fundamental parameters – galaxies: jets
1.Introduction
Active galaxies are a special kind of galaxy that has very violent activities and extreme physical processes, such as generating more energy than what nuclear reaction inside the star provides, generating relativistic high-energy particles and gamma-rays, thus forming non-thermal continuous radiation.Current research believes that these phenomena and processes of active galaxies are mainly produced or triggered by the core of a galaxy.Therefore,the core of an active galaxy is called an active galactic nucleus(AGN).AGNs can be classified as type 1 or type 2 depending on the obscuration of the luminous nucleus.AGNs can also be classified by the angle between the relativistic jet and the line of sight, and the relativistic jets of blazars are believed to be closely aligned to our line of sight.It is found from observations that blazars can be classified into flat-spectrum radio quasars (FSRQs) and BL Lacertae objects(BL Lacs), and while the angle between the jet and the line of sight cannot be used to distinguish them, the accretion rate or accretion model may be the key to their difference.Urry &Padovani (1995) divided blazars into two categories based on the equivalent width (EW) of their emission lines such that objects with EW>5 Å are FSRQs,and objects with EW<5 Å are BL Lacs (Urry & Padovani 1995).Xiong & Zhang (2014)and Sbarrato et al.(2012) divided blazars by the relation between Lγ/LEddand LBLR/LEddwith the dividing line LBLR/LEdd∼5×10-4; FSRQs are greater than the dividing line while BL Lacs are less than the dividing line, and Ghisellini (2010) dividedwith the dividing line Ldisk/LEdd∼10-2.The blazars selected in this paper have almost the same dividing line as that of Xiong and Sbarrato.In this paper,an interesting result is obtained,that is,the jet power and accretion disk magnetic field of FSRQs are positively correlated while the jet power and accretion disk magnetic field of BL Lacs are negatively correlated.These results may have important consequences for the evolution of blazars.
The bolometric luminosity is one of the critical parameters controlling the observational properties of AGNs.It can be used to study the sequence of AGNs (Fossati et al.1997;Ghisellini & Tavecchio 2008; Ghisellini 2010, 2016), the evolution of AGNs (Xie et al.2004, 2006) and the connection between jet and accretion disk or black hole (Yu et al.2015;Xue et al.2016).The bolometric luminosity can be estimated by broad-line region (BLR) luminosity Lbol=10LBLRor by X-ray luminosity Lbol=kLX(2–10 keV).The bolometric luminosity is also likely to be related to the beam power, where the beam power of the outflow is defined as the energy per unit time, Lj=dE/dt (Donato et al.2001; Giommi et al.2012;Calderone et al.2013; Daly et al.2018; Chen et al.2021).There are two obvious peaks in the spectral energy distributions(SEDs)of blazars;the low-energy peak is interpreted as caused by synchrotron radiation, and the high energy peak is interpreted as produced by inverse Compton (IC) scattering.Regarding γ-rays for different energy levels, Mannheim &Biermann (1992) reported that γ-rays of lower-power blazars are produced by the synchrotron-self Compton process and γrays of higher-power blazars are produced by the external Compton(EC)process(Sikora et al.1994).Fossati et al.(1997)calculate the bolometric luminosity by using Lγ∝Lsyn,peak,where Lsyn,peakis the luminosity of the synchrotron radiation peak.In this paper, we use the method of integrating the SED to calculate the bolometric luminosity and compare it with Lbol=kLX(2–10 keV), finding that the former of BL Lacs is greater than the latter, but we think it has little effect on the correlation analysis.
In regards to black hole systems with powerful jets, the energy of jets may be partly derived from the black hole spin(Blandford & Znajek 1977; Begelman et al.1984;Meier 1999, 2001; Blandford et al.2019).Jets may also be related to other parameters,and it is important to understand the relationship between the intrinsic physical variables.Many authors have identified relevant relationships (Rawlings &Saunders 1991; Celotti & Fabian 1993; Maraschi & Tavecchio 2003; Celotti & Ghisellini 2008; Ghisellini et al.2010;Sbarrato et al.2012; Ghisellini et al.2014; Xiong &Zhang 2014; Chen et al.2015).The intrinsic γ-rays in this paper have a significant correlation with the black hole spin,and we believe that the Blandford–Znajek(1977,hereafter BZ)mechanism may dominate over the Blandford–Payne (1982,hereafter BP) mechanism.In the BZ model, the generation of jets is related to the black hole mass,black hole spin,accretion rate and accretion disk magnetic field, while the BP model is independent of the black hole spin.In a hybrid model(Meier 1999, 2001), because of the existence of a magnetic switch, two distinct slow and fast jets will be produced.For high enough spin,black holes trigger the magnetic switch,and powerful jets will be produced.The lower spin black holes also have jets, but the energy of the jets is dominated by magnetic energy.For the samples in this paper, almost all blazars have sufficiently high spin and highly relativistic jets,but only a few BL Lacs have lower spin.Chen et al.(2021) studied the relationships between the jet powers and accretion rate of rotating supermassive black holes (SMBHs), finding that the contribution of the black hole mass and accretion rate to jet power is more than 95%.The samples in this paper have a similar result.
There may be some unknown relationships between various parameters of blazars.In order to reveal the relationship between them, we have collected 168 data on blazars and the corresponding observation times are in the Space Science Data Center SED Builder (https://tools.ssdc.asi.it/SED/) (Stratta et al.2011).We used these data to calculate the bolometric luminosity, accretion disk magnetic field, etc., and analyze the relationship between them.The methods are introduced in Section 2; the results and discussions are in Section 3; the summary and conclusions are in Section 4.The cosmological parameters H0=69.6 kms-1Mpc-1, Ωm=0.286 and ΩΛ=0.714 have been adopted in this work.
2.The Methods
2.1.The Samples
The black hole masses are from Ghisellini et al.(2014),Xiong & Zhang (2014), Chen et al.(2021).All samples are blazars, and there are 168 blazars in total (including 125 FSRQs and 43 BL Lacs).The virial masses (Shaw et al.2012)of blazars in our sample have been calculated by assuming the size of the BLR scales,assuming the BLR clouds are bound by the gravity of the central black hole,with the square root of the ionizing disk luminosity as indicated by reverberation mapping, with an average uncertainty of 0.5 dex (Peterson &Wandel 2000;McLure&Dunlop 2004).Nemmen et al.(2012)computed the intrinsic γ-ray luminosity as L=fbLiso, where fbis the opening angle or beaming correction factor.For blazar objects, fbis computed as 1 -cos (1Γ), where Γ is the bulk Lorentz factor.Part of the sources’ Γ is from Ghisellini et al.(2014), and part of the sources’fbis obtained from Chen et al.(2021).The rest of the sources’ intrinsic γ-ray luminosity is obtained from Xiong & Zhang (2014).To get the Lorentz factor we first have to acquire the Doppler factor.For the Doppler factors, the excess frequency of very-long-baseline interferometry (VLBI) can generally be interpreted as being caused by Doppler boosting by comparing the X-rays obtained from VLBI data with those obtained from observations.The Doppler factors can also be obtained using the relationship between the observed brightness temperature and the intrinsic bright temperature, and this method requires significant bursts in the light curve.Once we get the apparent velocity we can calculate the Lorentz factor(Hovatta et al.2009).The accretion disk luminosities are also from Ghisellini et al.(2014), Xiong & Zhang (2014), Chen et al.(2021), with an average uncertainty of 0.3 dex.Nemmen et al.(2012)estimated bolometric luminosity asbut we calculate bolometric luminosity by integrating the SED in this paper.We estimated the power of the jet by using an empirical relation between the average jet power and the radio luminosity, with a scatter of 0.47 dex (Chen et al.2021).The values of the other parameters will be described below.
2.2.Eddington Luminosity
The accretion of massive objects and the associated release of the binding gravitational energy are important sources of radiation in astrophysics.The process depends on the geometry and can be performed via various routes (Netzer 2013).In particular,spherical and nonspherical systems can behave very differently.We assume a black hole with mass M, monochromatic luminosity Lν,total luminosity L and fully ionized gas at a distance r from the black hole.The radiation pressure force on a particle is
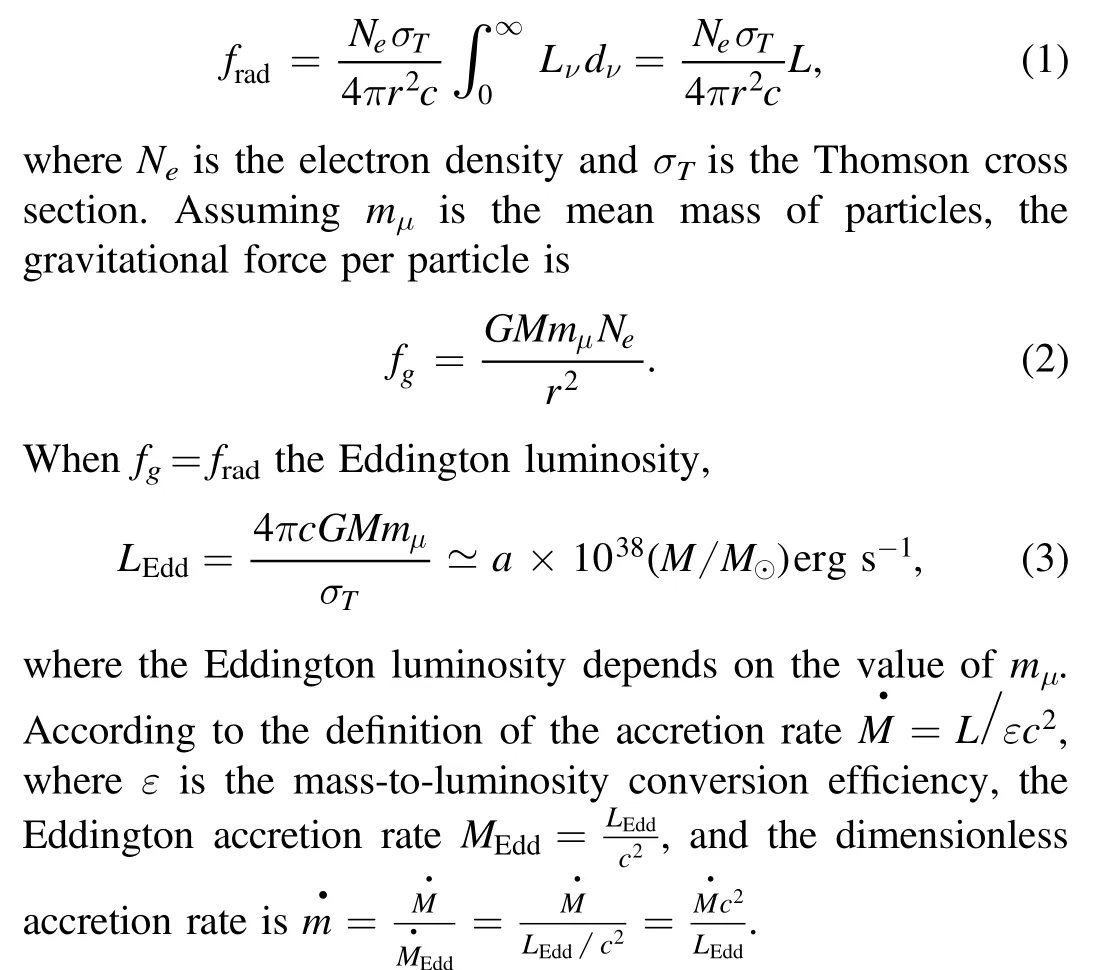
2.3.Accretion Rate
We can estimate the accretion rate from the part of the SED that shows Lν∝ν1/3(Frank et al.2002; Netzer 2013; Wang et al.2014).The equation gives the monochromatic luminosity at long wavelengths,
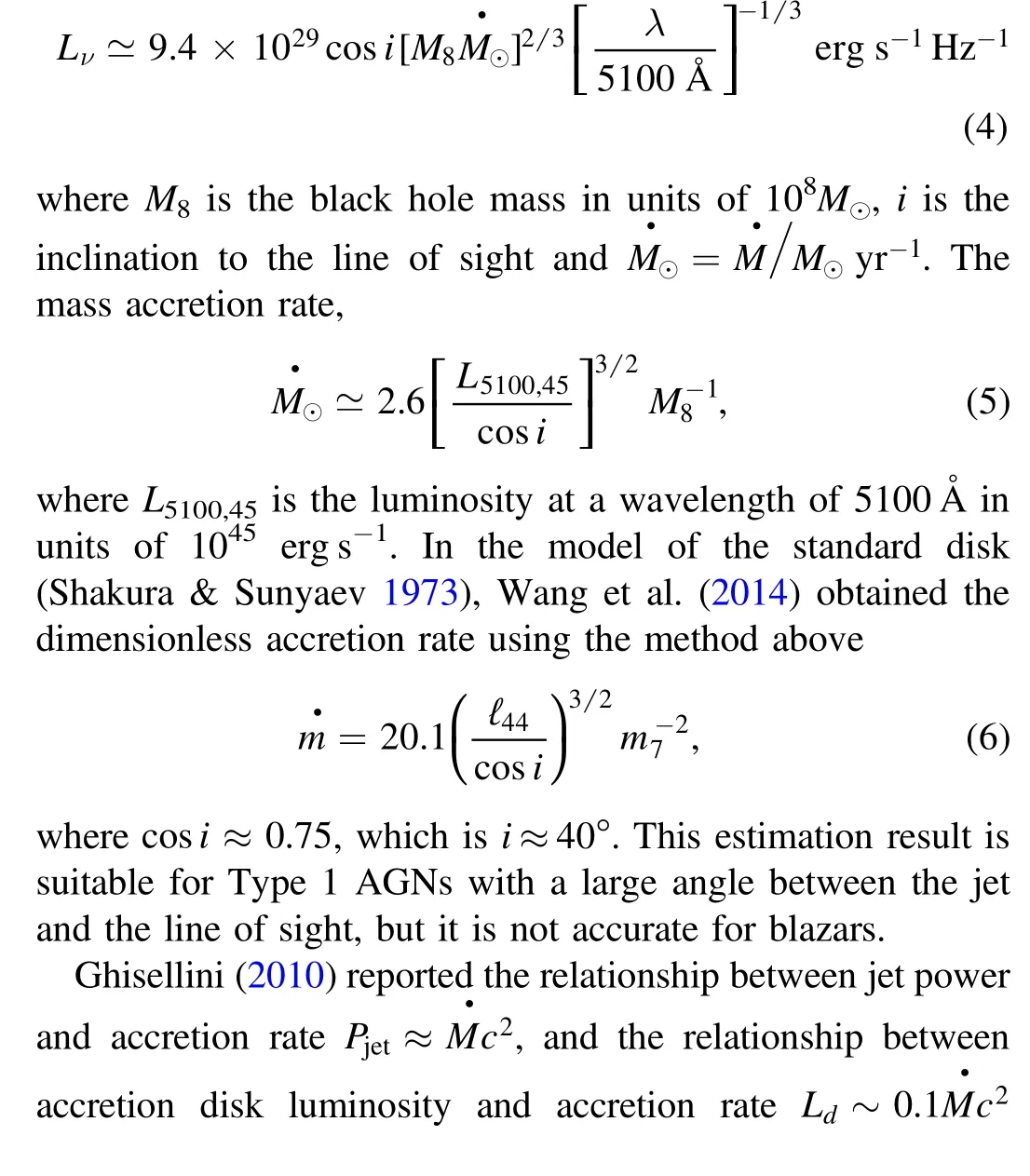

2.4.Bolometric Luminosity

Due to the different ranges of synchrotron radiation peaks,the upper and lower limits of the integration should be modified.For low synchrotron peak (LSP) and intermediate synchrotron peak (ISP) objects, the integration range of the synchrotron radiation peak can be set as[7,17],while for high synchrotron peak (HSP) cases the integration range of the synchrotron radiation peak can be changed to [7, 21].The integration range of the IC peak can be uniformly set to[17, 27].
2.5.The Spin of Black Holes
The relativistic effects become obvious where the orbits become unstable very close to the black hole.Far from the black hole, where the gravitational field is well approximated by Newton’s law of gravity, material follows stable circular orbits.The location of this transition is the innermost stable circular orbit(ISCO).Due to frame dragging effects,the size of the radius of an ISCO is affected by the spin of the black hole,and we can obtain black hole spin by measuring the gravitational redshifts of atomic features in the X-ray spectrum.This is called the X-ray reaction method(Reynolds 2019).The technique is employed most extensively for measuring black hole spin.However, it is very difficult to measure large numbers of samples with this technique, so other methods are considered.Chen et al.(2021) find that the spin of GX 339-4 obtained by the X-ray reaction method is 0.94±0.02,which is consistent with the result 0.92±0.06 using the method of Daly et al.(2018).
Daly et al.(2018) estimated the black hole spin using the radiation properties of the accretion disk and the properties of the radio source associated with the collimated outflow of the black hole.Daly reported the relationships between bolometric luminosity and beam power for 97 sources,

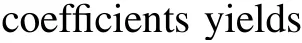

2.6.Magnetic Field of Accretion Disk
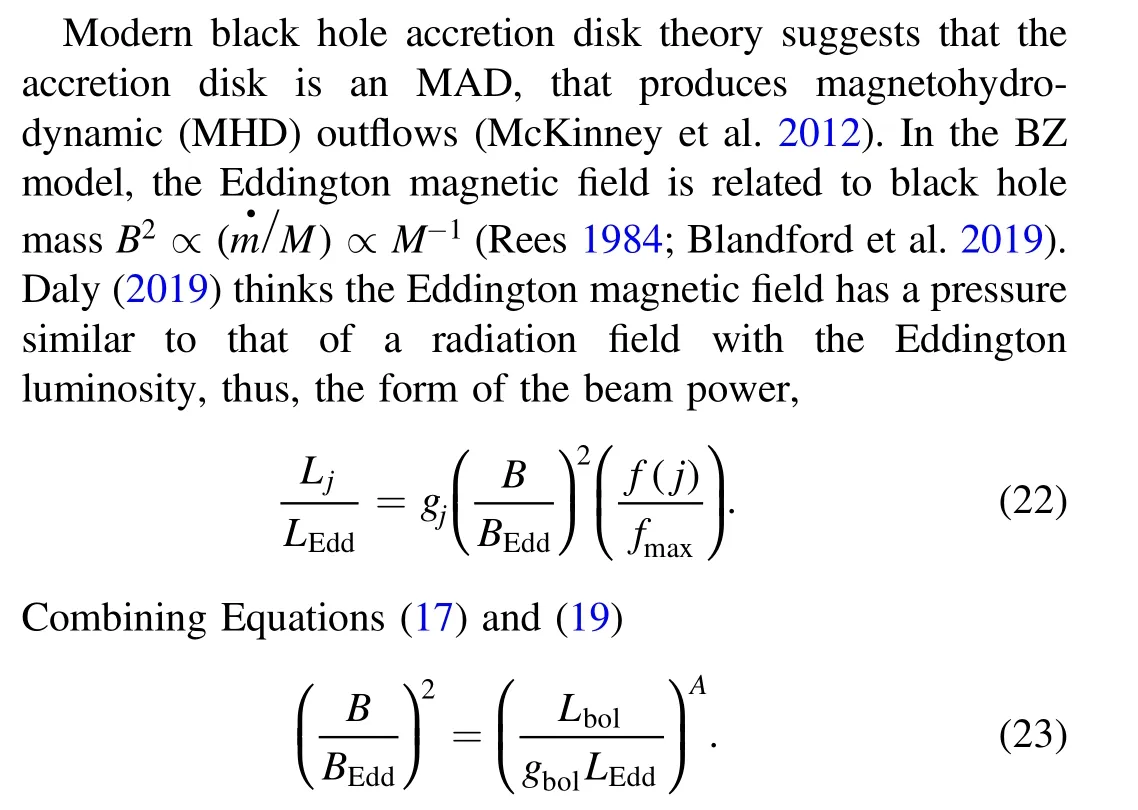

Table 1 The Samples
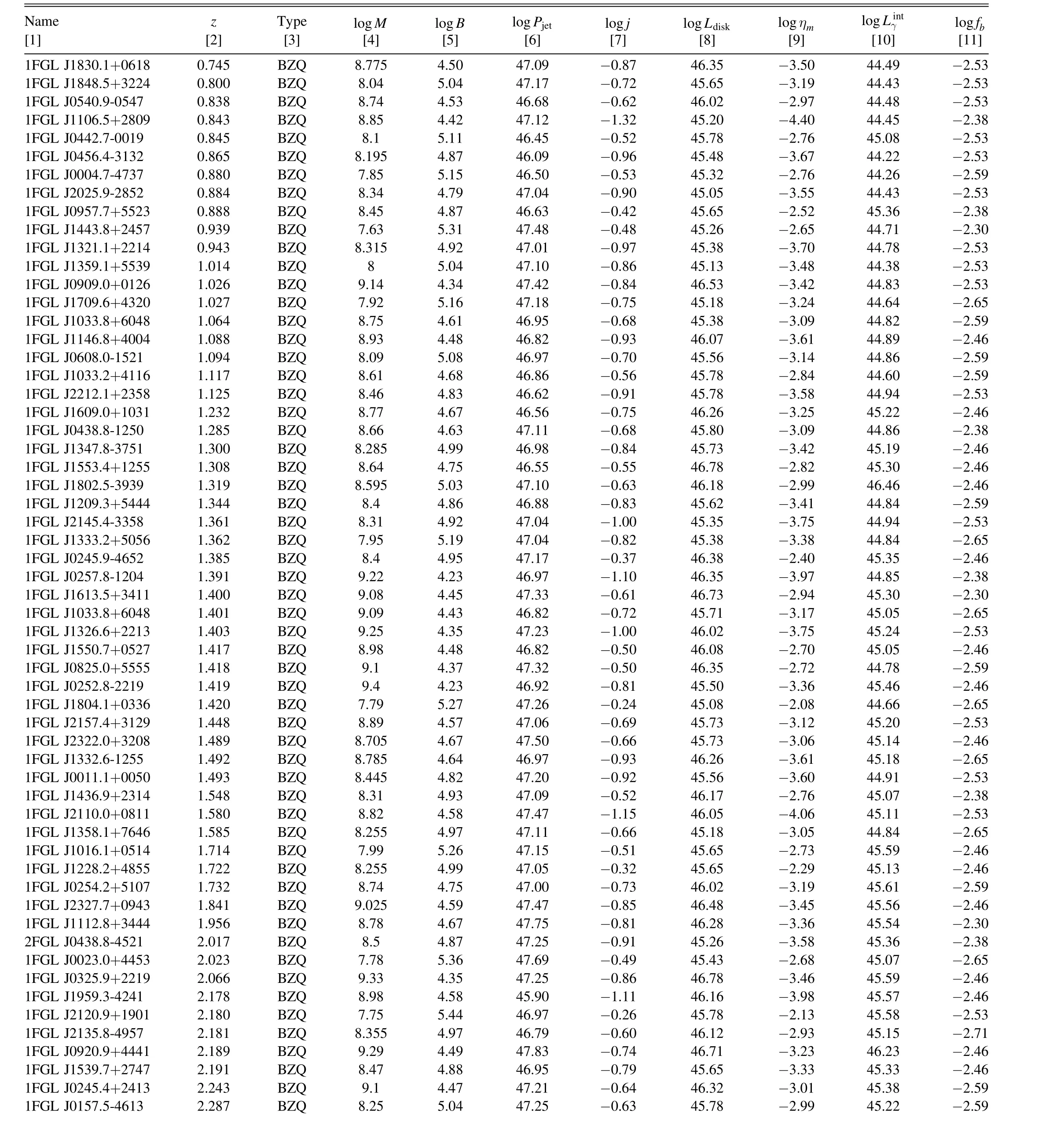
Table 1(Continued)

Table 1(Continued)
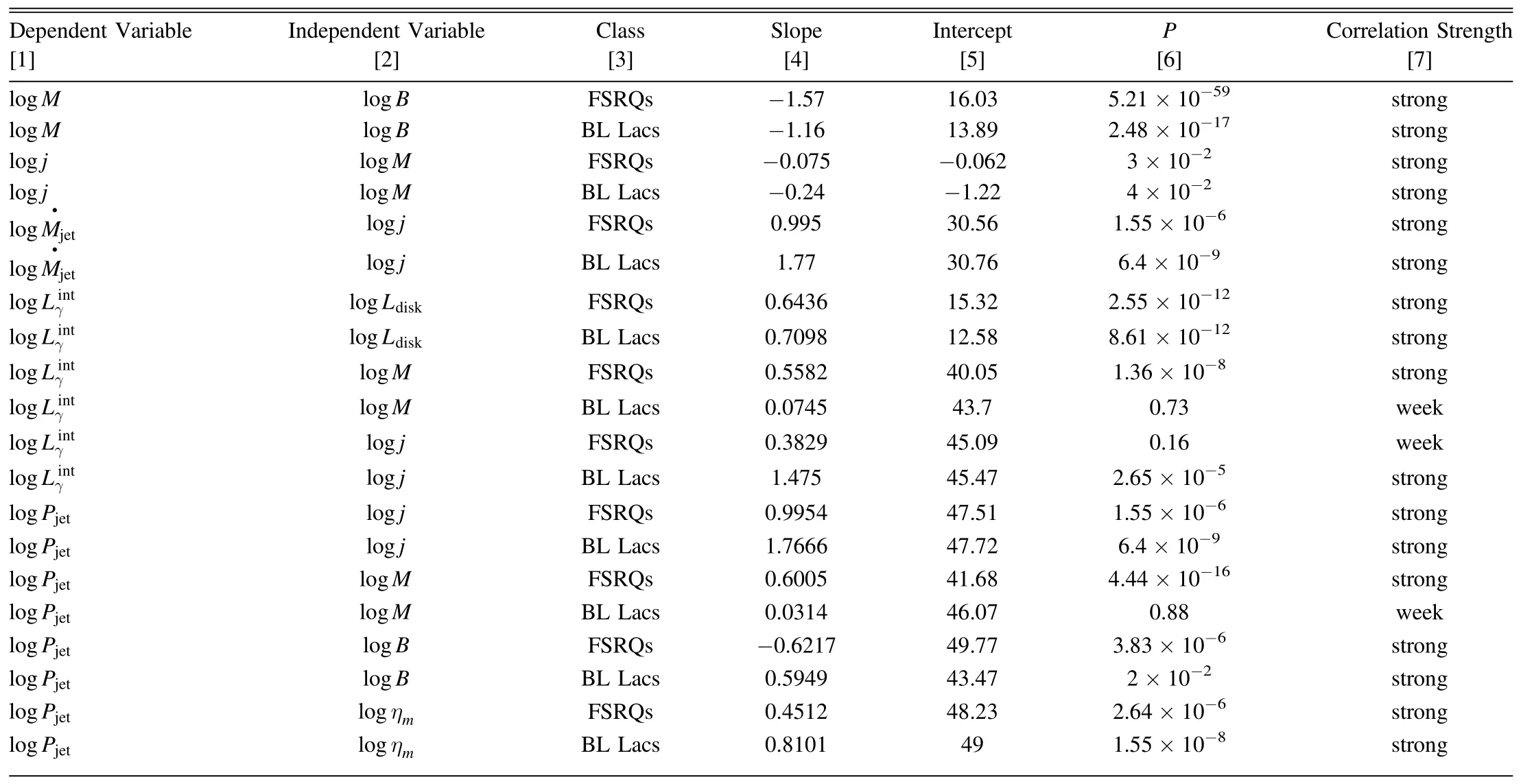
Table 2 The Samples
In addition, from what is discussed in Daly (2019), the magnetic field can be written as

2.7.Jet Power and Beam Power
Cavagnolo et al.(2010) reported that the relationship between average jet power and jet spectral flux at radio
We get the radio luminosity by integrating the radio flux over the 10–5000 MHz frequency band (Machacek et al.2006).
For the beam power, Willott et al.(1999) and Chen et al.(2021)reported the relationship between beam power and radio luminosity
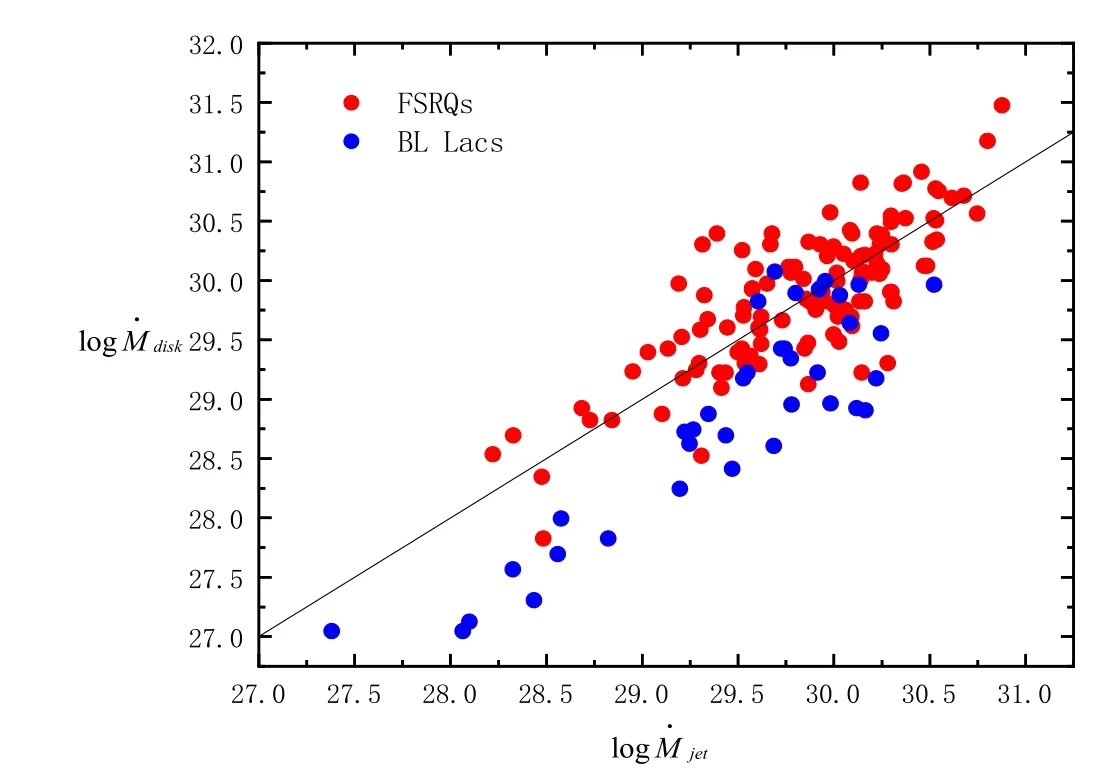
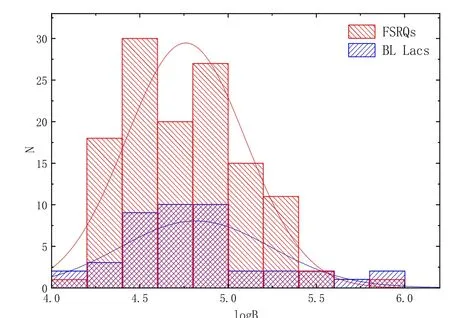
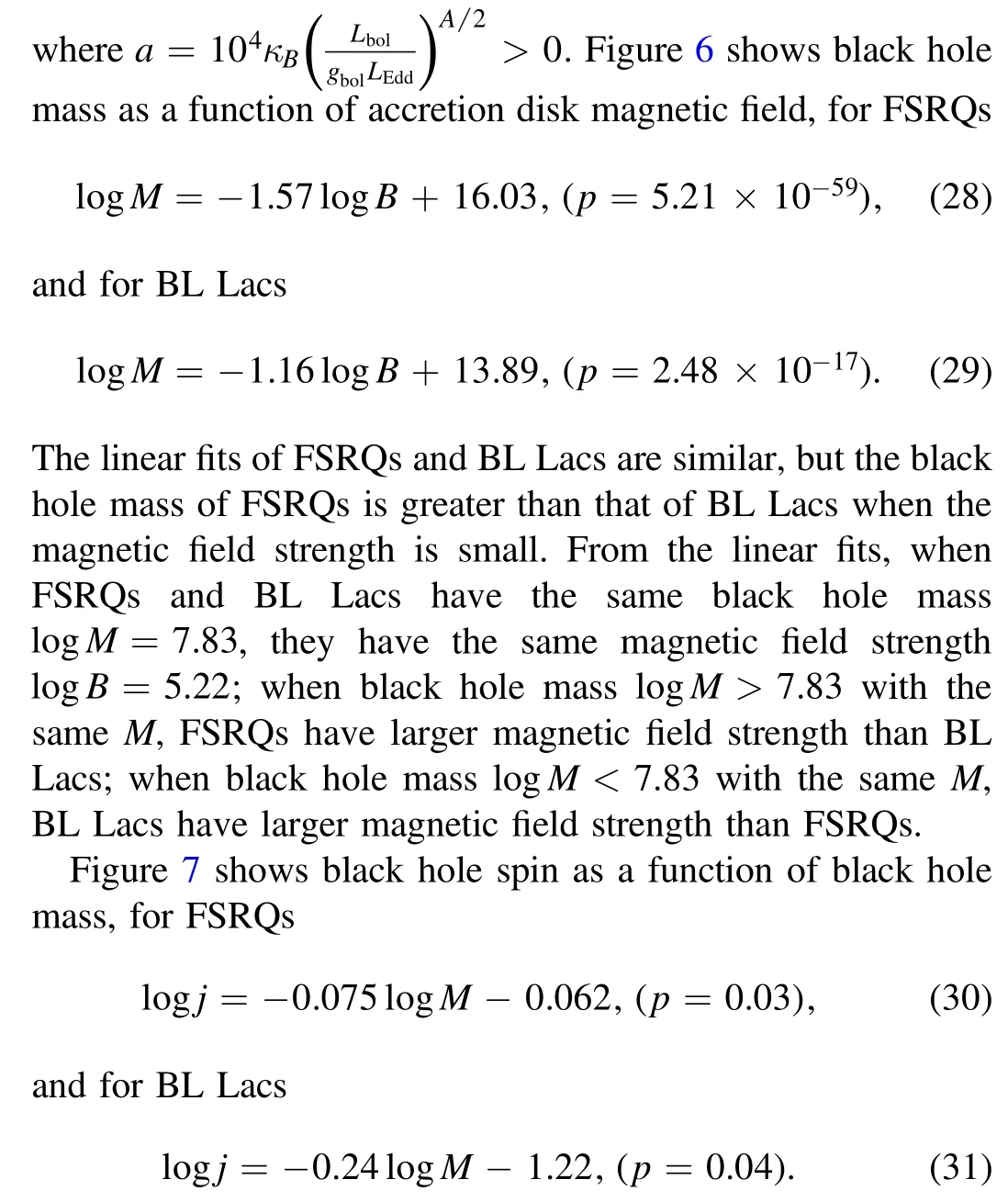
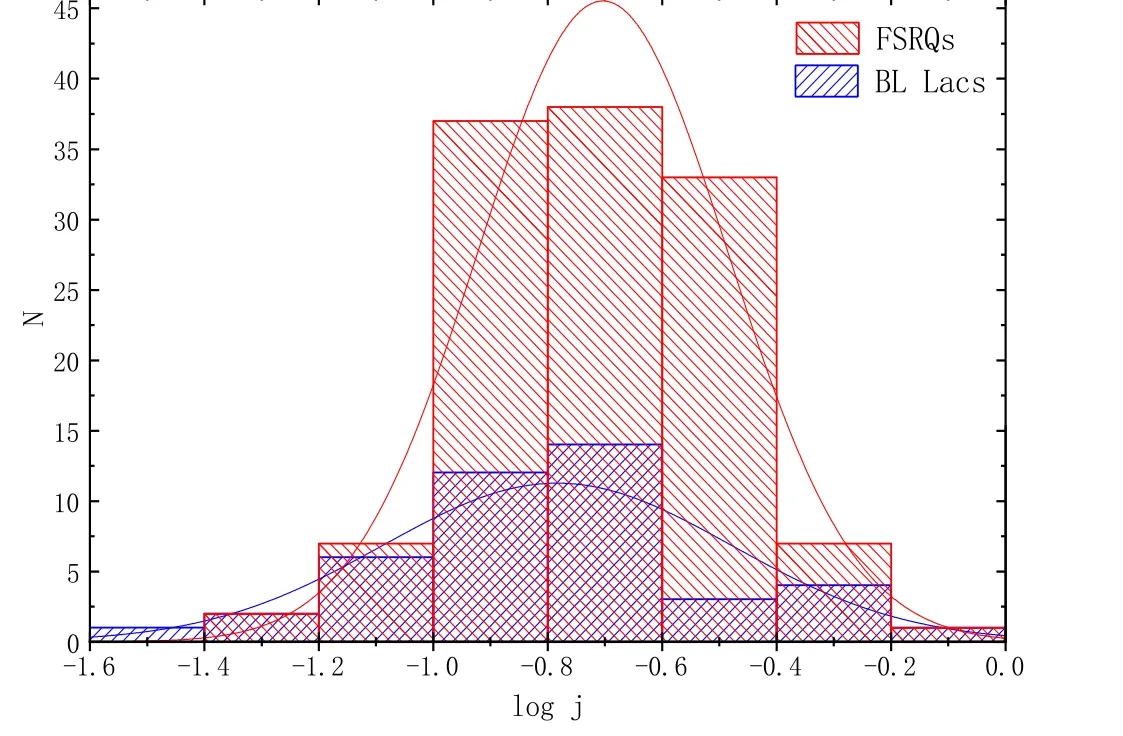
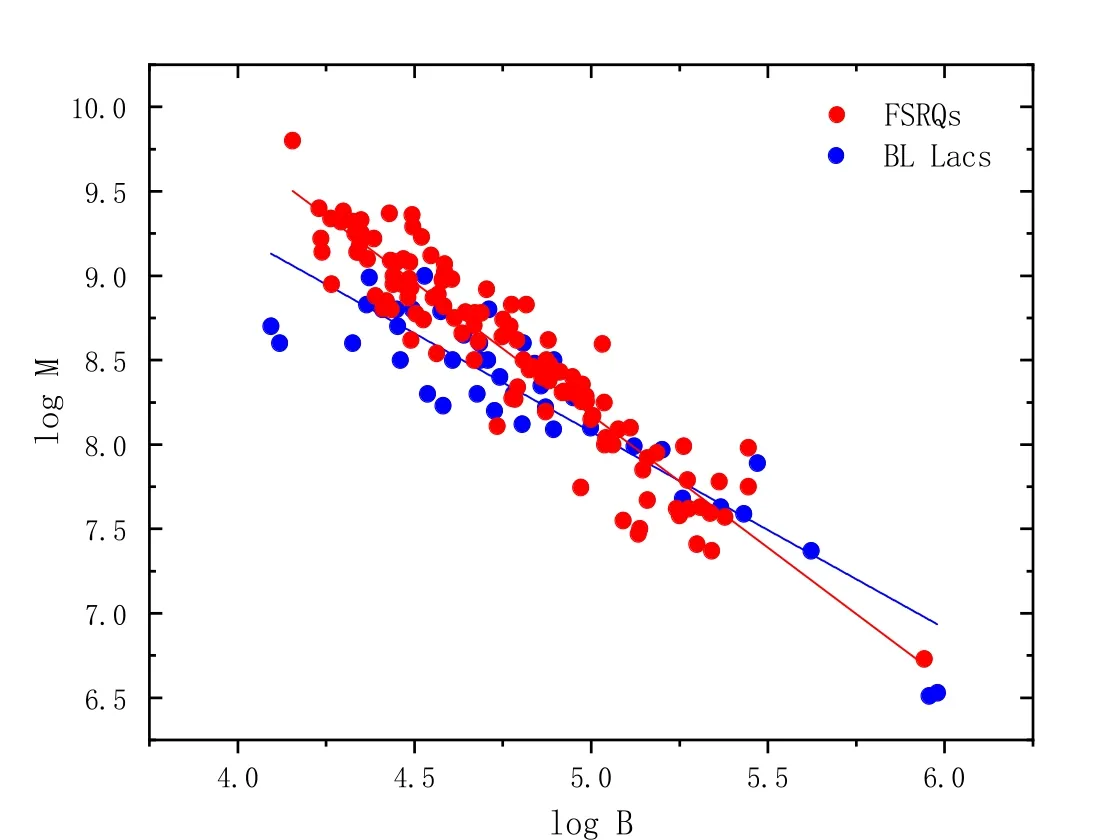
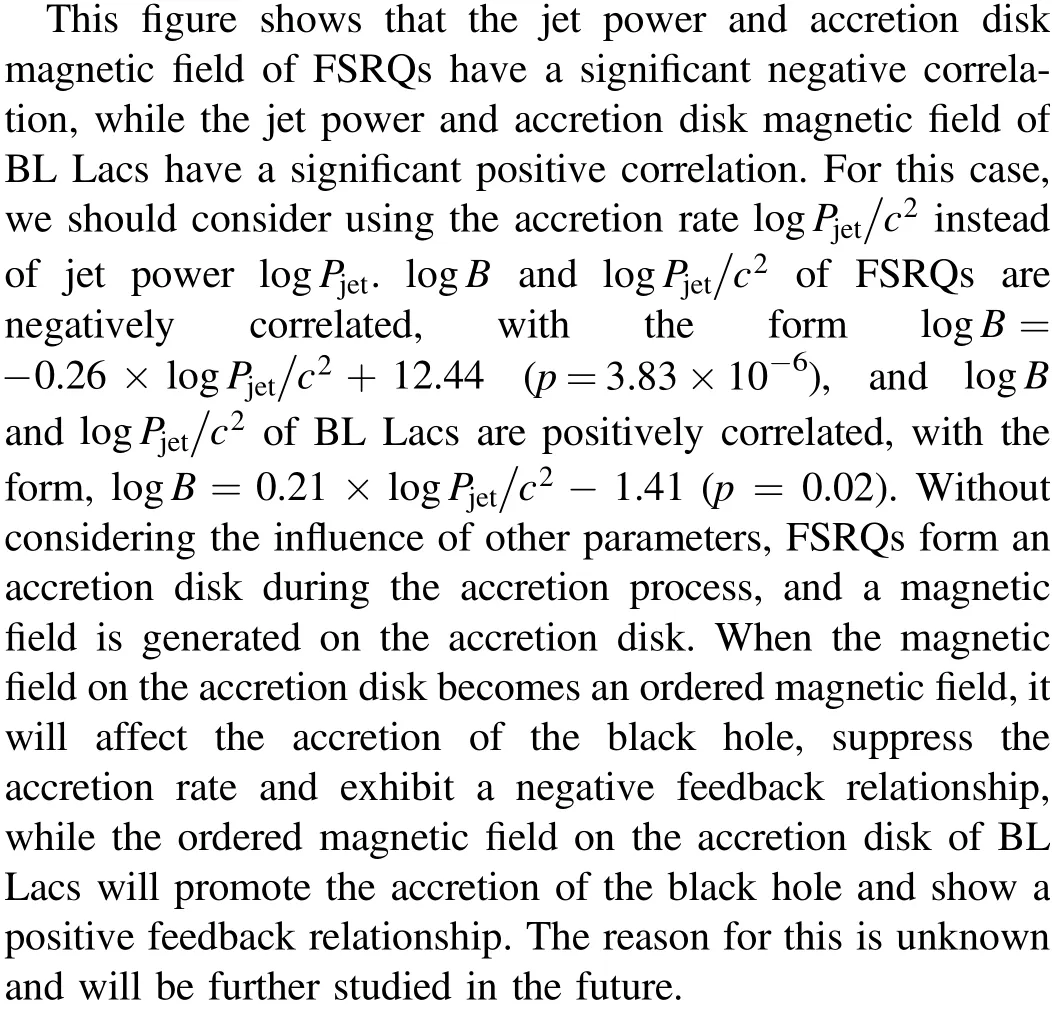
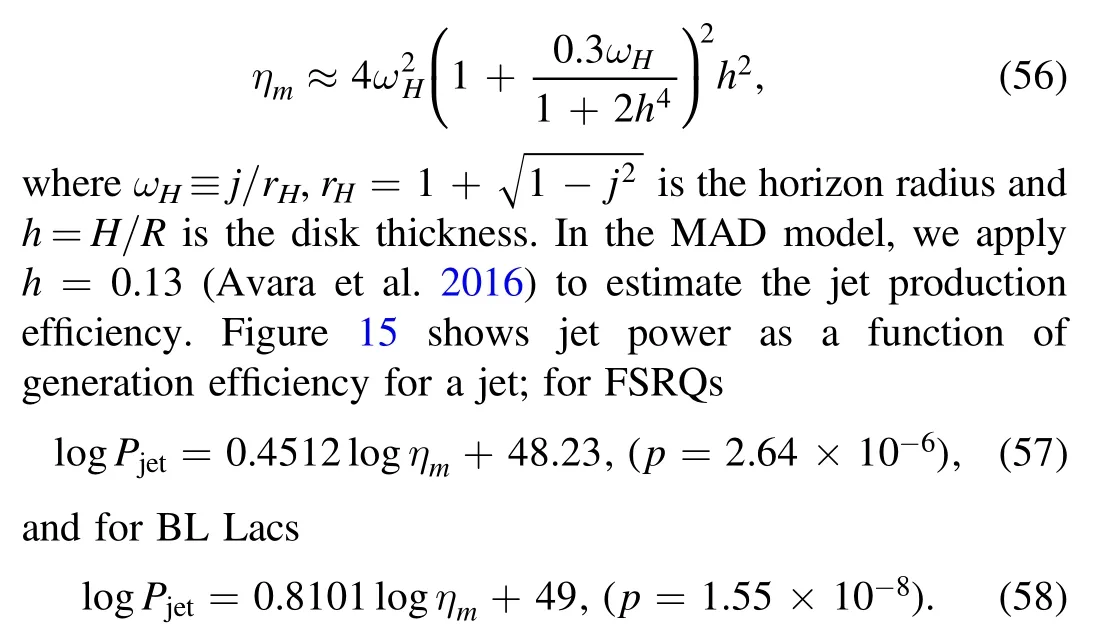
and where 1 Figure 2 shows the distributions of redshift.The distributions of FSRQs and BL Lacs are 0.189 Figure 1. Md •isk =L d 0.1c2vs. M j•et = Pj et c2for all samples.The black line stands for the accretion rate calculated by the two methods being the same.FSRQs are near the black line and BL Lac are below the black line. Mj•et is greater than Md•isk for most BL Lacs, but this aspect has little effect on the correlation analysis. Figure 4 shows the distributions of accretion disk magnetic field, and the associated distributions of FSRQs and BL Lacs are 4.15 < logB< 5.94and 4.09 < logB< 5.98, respectively.The means of FSRQs and BL Lacs arelogB=4.76and logB=4.81, respectively, and the medians of FSRQs and BL Lacs arelogB=4.73 logB=4.78, respectively.Neither FSRQs nor BL Lacs fit a normal distribution (for FSRQs,p = 0.016, for BL Lacs p = 0.021), and the distributions of FSRQs and BL Lacs are very similar.Accretion disk magnetic field is computed using Equation (20) derived by Daly (2019)through empirical relationships, because an accurate accretion disk magnetic field is difficult to obtain. Figure 5 shows the distributions of black hole spin, and the associated distributions of FSRQs and BL Lacs are- 1.32 < logj< - 0.04and - 1.48 < logj< - 0.13, respectively.The means of FSRQs and BL Lacs arelogj= -0.7and logj= - 0.78,respectively,and the medians of FSRQs and BL Lacs arelogj= -0.69andlogj= - 0.75, respectively.Both FSRQs and BL Lacs are normally distributed (for FSRQs and BL Lacs p=0.2),FSRQs and BL Lacs have exactly the same distribution, and j is computed by Equation (18). Equation (21) indicates that Figure 4.Distributions of accretion disk magnetic field; the meanings of lines are the same as in Figure 2. Both FSRQs and BL Lacs are negatively correlated, that is,when the black hole mass is larger, the black hole spin is slower,and this situation is described in King et al.(2008)and Chen et al.(2021).Possible reasons for the result are isotropic chaotic accretion of black holes or the merging of smaller black holes(Volonteri et al.2005;Nelson et al.2014;Fiacconi et al.2018). Figure 5.Distributions of accretion disk magnetic field; the meanings of lines are the same as in Figure 2. Figure 6.The red line is the linear fit of FSRQs,and the blue line is the linear fit of BL Lacs. Figure 8 plots black hole mass as a function of accretion disk magnetic field, for FSRQs Both FSRQs and BL Lacs are positively correlated, and when accretion disk luminosity and black hole spin are considered,there is a similar relationship.Chen et al.(2021) reported that the third-order fitting is better betweenlogPjetand j, and the numerical simulations in Nemmen et al.(2007) have a similar result.These relationships all indicate that the black hole spin has a promoting effect on the black hole accretion rate. Figure 7.The red line is the linear fit of FSRQs,and the blue line is the linear fit of BL Lacs. We get the radio luminosity by integrating the radio flux over the 10–5000 MHz frequency band, and γ-ray luminosity by integrating the γ-ray flux over 0.1–100 GHz(Nemmen et al.2007).γ-rays are produced upstream by IC scattering,however radio is produced by synchrotron emission from the extended jet (Ghisellini & Tavecchio 2009, 2010).γ-ray luminosity is a good indicator of jet power.In order to avoid the beaming effect we use intrinsic γ-ray luminosity,Lintγ.Figure 9 shows intrinsic γ-ray luminosity as a function of accretion disk luminosity for FSRQs Figure 8.The red line is the linear fit of FSRQs,and the blue line is the linear fit of BL Lacs. Figure 9.The red line is the linear fit of FSRQs,and the blue line is the linear fit of BL Lacs. and for BL Lacs The intrinsic γ-ray luminosity of FSRQs has a significant positive correlation with the black hole mass, but has no significant correlation with the black hole spin, while the intrinsic γ-ray luminosity of BL Lacs has a significant positive correlation with the black hole spin, but has no significant correlation with black hole mass.These relationships indicate that the jet power is related to the mass,spin and accretion rate of the black hole,but there are differences between FSRQs and BL Lacs. Figure 10.The red line is the linear fit of FSRQs,and the blue line is the linear fit of BL Lacs. In the BP model (Blandford & Payne 1982), the magnetic field lines leave the accretion disk and extend far away,and the angle between the polar component of the magnetic field and the surface of the disk is less than 60 deg;in a hot magnetically dominated corona where the flow is driven by gas pressure, a centrifugally driven outflow of matter from the disk is possible.In the BZ model (Blandford & Znajek 1977), when the magnetic field lines supported by the external current flowing in the equatorial disk pass through the rotating black hole, an electric potential difference is induced.If the field strength is large enough, the vacuum is unstable, and the cascade of electron-positron pairs will create a surrounding force-free magnetic layer.In these cases, that energy and angular momentum will be extracted electromagnetically.Further results show that the charge will never make a significant contribution to the geometry of the rotating black hole. Figure 12 shows the relationship between jet power and black hole spin, for FSRQs From the significant correlation between the jet power and the black hole spin,we think that the BZ model may dominate over the BP model for the samples selected in this paper,indicating that the jet generation extracts the energy of the black hole spin.In addition, jet power is greater than the accretion disk luminosity for all samples,also tending to favor the BZ model. Figure 11.The red line is the linear fit of FSRQs,and the blue line is the linear fit of BL Lacs. Figure 12.The red line is the linear fit of FSRQs,and the blue line is the linear fit of BL Lacs. Figure 13 displays the relationship between jet power and black hole mass, for FSRQs The jet power and black hole mass of FSRQs have a significant positive correlation, but not the BL Lacs, which is consistent with the relationship of intrinsic γ-ray luminosity. Figure 13.The red line is the linear fit of FSRQs,and the blue line is the linear fit of BL Lacs. Figure 14 shows the relationship between jet power and magnetic field strength, for FSRQs In the BZ model, the accretion disk magnetic field plays an important role in the generation of jets, which is considered in the multiple linear regression; the multiple linear regression of the jet power of FSRQs is Figure 14.The red line is the linear fit of FSRQs,and the blue line is the linear fit of BL Lacs. with p ≈0, and the standardized coefficients of black holemass, accretion disk luminosity, black hole spin and magneticfield strength are 1.797, 0.118, 0.364 and 1.264, respectively.The contribution rates of each parameter are: For BL Lacs With p ≈0, the standardized coefficients of black hole mass,accretion disk luminosity, black hole spin and magnetic field strength are 1.702, 0.045, 0.378 and 1.669, respectively.The contribution rates of each parameter are: From the point of view of contribution rate, there is no significant difference between FSRQs and BL Lacs.The contribution rate of the accretion rate of FSRQs and BL Lacs to the jet power is almost zero,while the contribution rates of the accretion disk magnetic field to the jet power of FSRQs and BL Lacs are as high as 35.68% and 43.99%, respectively.The relationships betweenlogBandlogM•jetabove affirm that the accretion rate and the accretion disk magnetic field have a significant correlation.It can be considered that the accretion disk magnetic field is the product of the accretion process.It is the accretion disk magnetic field that directly affects the jet during the generation of the jet,but the accretion rate indirectly affects the jet, so the contribution rate of the accretion disk magnetic field can be regarded as the contribution rate of the accretion rate.The black hole masses of FSRQs and BL Lacs have a great contribution rate to the jet,which are 50.72%and 44.86%, respectively, and the contribution rates of the black hole spin of FSRQs and BL Lacs are 10.27% and 9.96%,respectively.The spacetime outside the horizon of an SMBH will be curved, and a rotating black hole has a drag effect on the spacetime outside the horizon; the jet will extract the rotational kinetic energy of the black hole.Obviously,the mass of the black hole has a greater contribution rate. The effect of general relativity is obvious where the inside of the accretion disk is close to the black hole.We assumed that all samples can produce a jet and extract the black hole rotation energy, which is not necessarily accurate.Assuming the accretion disk can be modeled as fluids, in the BZ model, for the production of the jet, strong and dynamic magnetic fields are necessary.Since accretion is a long process,it is important to perform multidimensional general relativistic magnetohydrodynamics (GRMHD) simulations.Nakamura et al.(2018)performed extensive inspections of the M87 jet by using twodimensional (2D) GRMHD simulations and the steady axisymmetric force-free electrodynamic (FFE) solution.The result suggests that the M87 jet is likely powered by a rotating black hole.Mościbrodzka et al.(2016) performed threedimensional (3D) GRMHD simulations of M87, and the result suggests that the edge-brightening is related to black hole spin.Avara et al.(2016)performed fully 3D GRMHD simulations of thin accretion disks with high radiation efficiency.In the longterm (t ∼70000rg/c) evolution simulation, the quasi-steadystate MAD radius is extended to t ∼35rg, and the radiation efficiency of the accretion disk is 15%.Combining more than 25 different thicker MAD models, Avara et al.found the jet production efficiency in the thin MAD model, Figure 15.The red line is the linear fit of FSRQs,and the blue line is the linear fit of BL Lacs. Both FSRQs and BL Lacs have significant positive correlations between jet power and jet production efficiency.That is, our samples conform to the numerical simulation performed by Avara et al.(2016).The contribution rate of other parameters will be very small after considering black hole spin.Because the jet generation efficiency is computed by black hole spin, we do not consider the black hole spin in the multiple linear regression of the jet production efficiency.The multiple linear regression of the jet generation efficiency of FSRQs is as follows: with p=4.05×10-11.The standardized coefficients of black hole mass, accretion disk luminosity and magnetic field strength are -0.258, 0.633 and 0.415, respectively.The contribution rates of each parameter are: The contribution rate of the black hole mass to the jet generation efficiency is negative, about 20%.That is, without considering other parameters,the larger the black hole mass is,the lower the jet generation efficiency, while the contribution rates of the accretion rate and the accretion disk magnetic field are positive, and the combined contribution rate is about 80%.This result is taken for granted.The relationship between black hole spin and black hole mass above shows that a black hole with higher spin has smaller mass.Under the premise that the black hole spin has a significant contribution to the jet generation, the smaller the black hole mass is, the higher the jet generation efficiency.The relationship between black hole spin and accretion rate in the previous section shows that the black hole spin has a promoting effect on the accretion rate,but as the black hole(long-term isotropic chaotic)accretion process proceeds, the black hole spin will slow down.For this, it is necessary to examine the contribution rate of the black hole mass and spin to the accretion rate.We used a multiple linear regression betweenlogLdisk,logMandlogjto calculate the contribution rate.The contributions of the black hole mass and black hole spin of FSRQs to the accretion rate are 69.2% and 30.8%, respectively, and the contributions of the black hole mass and black hole spin of BL Lacs to the accretion rate are 36.4% and 63.6%, respectively.Combining what is discussed above, a possible explanation is that BL Lacs and FSRQs are objects from the same class, but BL Lacs occur in the early stage of FSRQs.At this time, the BL Lacs have relatively smaller mass with relatively higher spin.Also, the spacetime drag effect caused by the black hole spin causes strong magnetic reconnection at the inner radius of the accretion disk to generate a strong magnetic field, so that BL Lacs can generate powerful jets.The accretion of material at this time is dominated by the black hole spin,and as the accretion process proceeds, the mass increases and the spin becomes slower.Then, the accretion model may change.BL Lacs gradually become FSRQs.At this time, the spacetime drag effect caused by the spin of the black hole is relatively weak,but there is still a strong magnetic field to generate jets, and the accretion of material at this time is dominated by the black hole mass. In Figure 6, the data points are basically concentrated in the arealogB<5.22, where the black hole mass of FSRQs is generally greater than that of BL Lacs.From this, it can be considered that BL Lacs may occur earlier than FSRQs.With the evolution of the accretion process, the accretion disk magnetic field becomes stronger.When the magnetic field reaches a critical value,the magnetic field of the accretion disk gradually weakens.This evolutionary process results in FSRQs and BL Lacs that may have the same accretion disk magnetic field but FSRQs have a larger black hole mass, where the area logB>5.22can be considered as the intermediate state of BL Lacs to FSRQs. For FSRQs or BL Lacs,as the black hole mass increases,the black hole spin becomes slower, i.e., logj∝-logM.The possible reason is long-term isotropic chaotic accretion.The angular momentum of the black hole is conserved.As the isotropic chaotic accretion proceeds,the mass of the black hole increases and the spin slows down.It may also be the result of merging with a small-mass black hole, which is similar to reverse accretion.The angular momentum of a small-mass black hole is opposite to the direction of the black hole spin,which causes the black hole spin to slow.When the black hole spin becomes slower, the spacetime drag effect caused by the black hole spin becomes weaker, and the accretion disk magnetic field weakens, i.e., logj∝logB.At the same time,the mass of the black hole increases, i.e., logB∝-logM. Xiong& Zhang (2014)foundthedividingline LBLR/LEdd∼5×10-4 betweenFSRQs andBLLacsfrom the relationship between logLBLRLEddandlogL γLEdd.Sbarratoetal.(2012)obtainedthe same result,and thesimilar dividingline inthispaper is LBLR/LEdd∼5×10-2, whichis differentfrom the results ofXiongand Sbarrato.Ghisellini(2010)obtained the dividingline Ldisk/LEdd∼10-2between FSRQs and BL Lacs•from the relationship between logLdiskLEddandlogM MEdd.Our samples have almost exactly the same dividing line.The magnitude of the accretion rate affects the magnitude of Ldiskand LBLR, so it can be considered that Xiong and Zhang,Sbarrato et al.and Ghisellini indirectly used the accretion rate to classify blazars.In the process of black hole accretion evolution, the accretion disk will generate an ordered magnetic field and affect the accretion rate and jet power.Therefore, the contribution of the accretion disk magnetic field to the accretion rate and jet flow is considered.From the multiple linear regression, we find that there is no significant difference in dimensionless accretion rate between FSRQs and BL Lacs, and the magnetic field contributes the most.The generation of jets is also affected by the accretion disk magnetic field.From the linear fitting of logPjetandlogBin Figure 14,it can be seen that the jet power of FSRQs is inhibited by the magnetic field of the accretion disk, while for BL Lacs the situation is the opposite.The possible reason for this is that the accretion disk magnetic field continues to increase during the evolution of BL Lacs into FSRQs until the accretion disk magnetic field of BL Lacs reaches the critical value.If there are factors that inhibit the accretion disk magnetic field of BL Lacs, the magnetic field strength will not increase to a very high level.Data show that the accretion rate and accretion disk magnetic field of BL Lacs are mutually reinforcing, so that the accretion disk magnetic field can increase to a high value.When the magnetic field reaches the critical value,and the accretion rate also reaches the maximum, the accretion model may change.At this time, the accretion rate and the accretion disk magnetic field inhibit each other, so that the accretion disk magnetic field gradually becomes weaker, which may be the reason for Figure 6.Here we analyze the evolution of BL Lacs from the perspective of increasing black hole mass.BL Lacs with smaller mass evolve into FSRQs.We notice that the mean mass of BL Lacs is smaller than that of FSRQs,but the black hole spin of BL Lacs is slower than that of FSRQs.If analyzed from the perspective of black hole spin,it is not impossible that FSRQs with higher spin evolve into BL Lacs.If both of these scenarios are possible, the continuous evolution of blazars would be possible. The intrinsic γ-ray luminosity of FSRQs has a significant positive correlation with the accretion disk luminosity and black hole mass, but no significant correlation with the black hole spin,while the intrinsic γ-ray luminosity of BL Lacs has a significant positive correlation with the accretion disk luminosity and black hole spin, but no correlation with black hole mass.This situation is not necessarily consistent with the evolution, because the jet power of FSRQs has a significant correlation with the black hole spin.A possible reason is that a considerable part of the γ-ray photons of FSRQs is produced by the EC mechanism. Figure 12 shows that the samples are completely consistent with the BZ model.The jet generation efficiency and jet power of FSRQs and BL Lacs are almost the same,indicating that the jet generation mechanism of FSRQs and BL Lacs may be the same.Jet power is greatly affected by the black hole mass(the contribution rate is over 44%),and is very little affected by the accretion rate (the contribution rate is about 3%), while the jet generation efficiency is relatively weakly affected by the black hole mass(the contribution rate is about 20%),and is relatively greatly affected by the accretion rate (the contribution rate is about 50%).Black hole spin energy is governed by black hole mass and rotational speed.In the BZ model, jet power is directly related to the black hole spin and mass.Part of the contribution rate of the accretion rate is transferred to the contribution rate of magnetic field.The jet power is indirectly related to the accretion rate,resulting in a very low contribution rate.While the generation efficiency of jets is also related to the black hole mass,part of the contribution rate of black hole mass is transferred to the contribution rate of accretion rate.This results in a lower contribution rate of the black hole mass and a higher contribution rate of the accretion rate.The contribution rate of the magnetic field to the jet power is about 40% (for FSRQs, 35.68%, for BL Lacs, 43.99%).The contribution rate of the magnetic field to the jet generation efficiency is about 30%.The jet power is slightly more affected by the magnetic field, but the difference is not obvious.The difference is that the effect of the magnetic field on the jet power is during the BZ process, while the effect of the magnetic field on the jet generation efficiency is before the BZ process.In the hybrid model,Meier(1999)thinks that the generation of a jet is related to the magnitude of the magnetic field, such that for high enough spin, the black hole triggers the magnetic switch,producing relativistic jets.The samples selected have strong accretion disk magnetic field, high enough spin and can generate powerful jets, which are consistent with Meier’s theory. The basic parameters mentioned in the article have uncertainties, some of them even exceeding 0.5 dex, and we have to admit that these interpretations are just a bold attempt.In addition,our work can give others an idea to understand the relationship between the fundamental parameters of black holes. Acknowledgments This work is supported by the National Natural Science Foundation of China (NSFC, grant No.11063004).We thank the experts for their advice. ORCID iDs H.Zhang https://orcid.org/0000-0001-6741-11523.The Results and Discussions
3.1.Distribution of Parameters
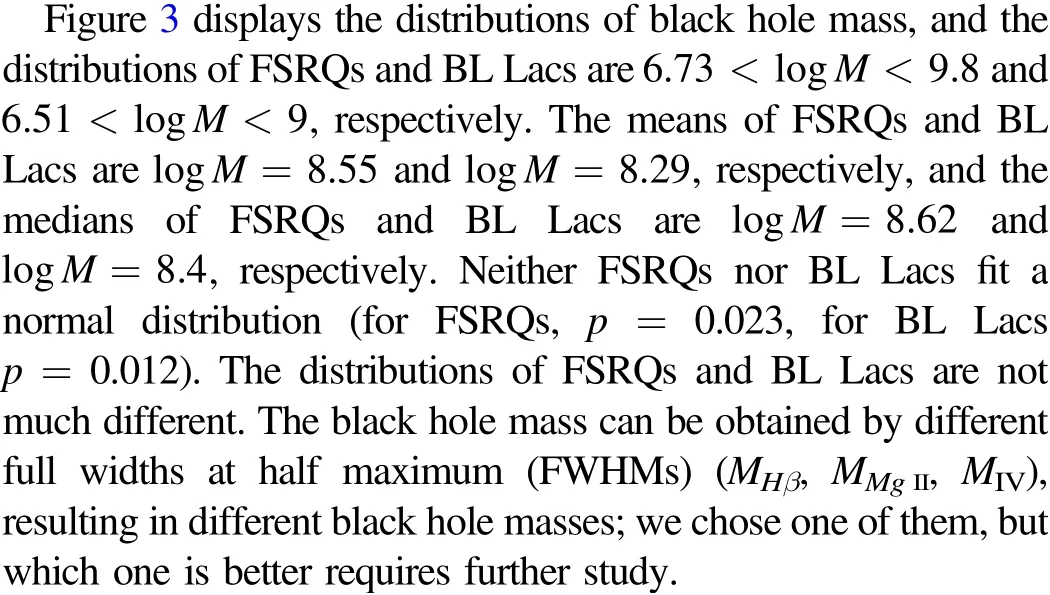
3.2.Black Hole Mass versus Magnetic Field and Black Hole Spin
3.3.Accretion Rate versus Black Hole Spin


3.4.Intrinsic γ-Ray Luminosity
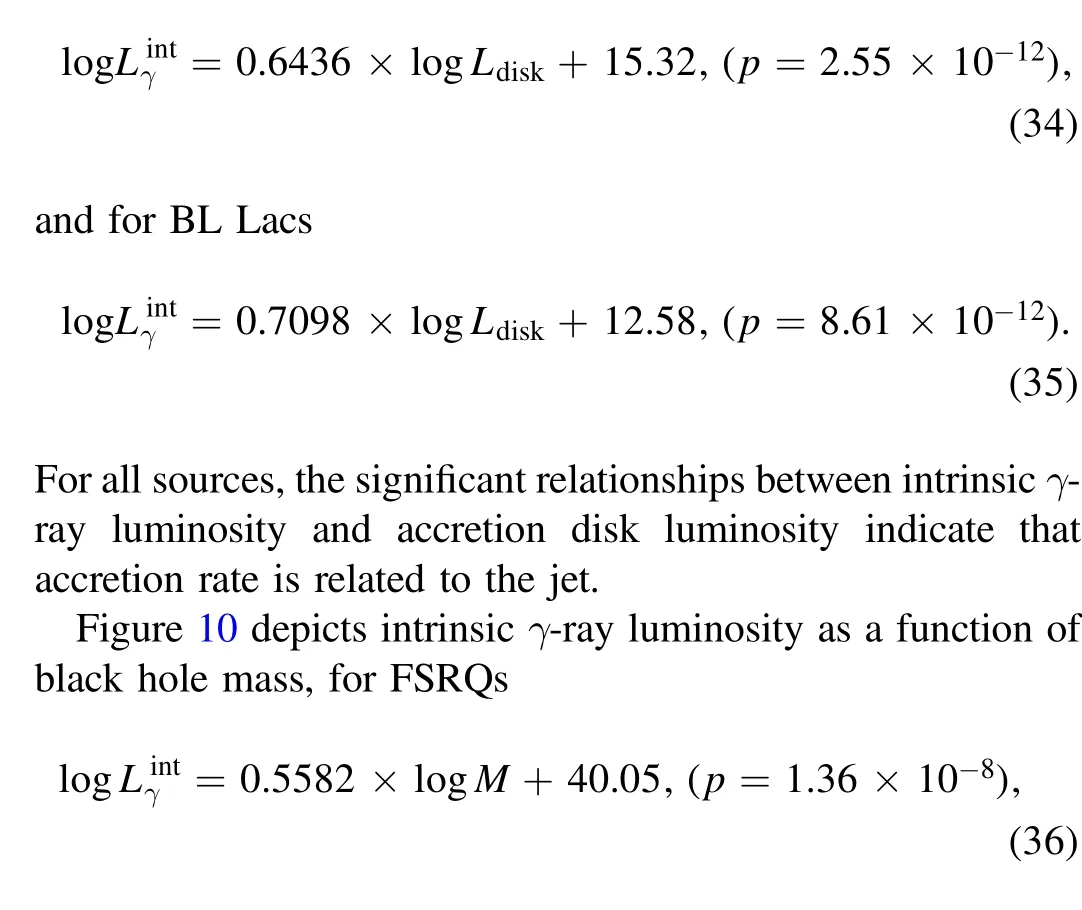




3.5.Jet Power

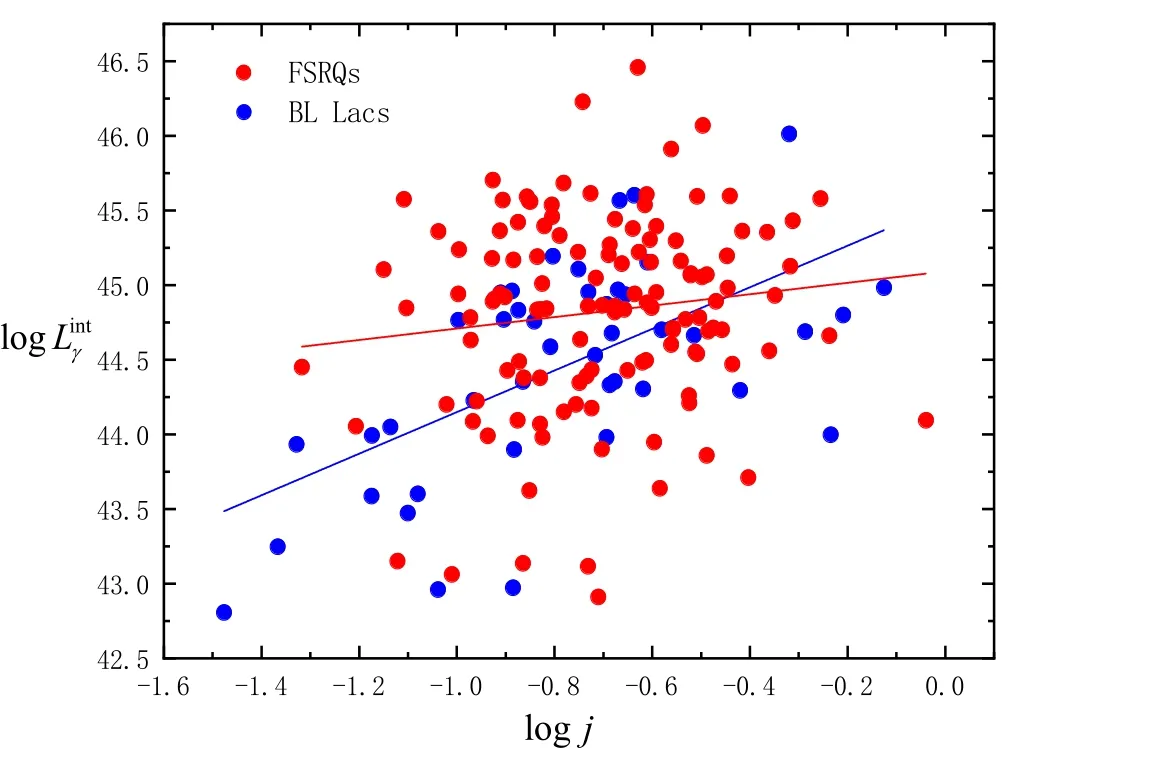
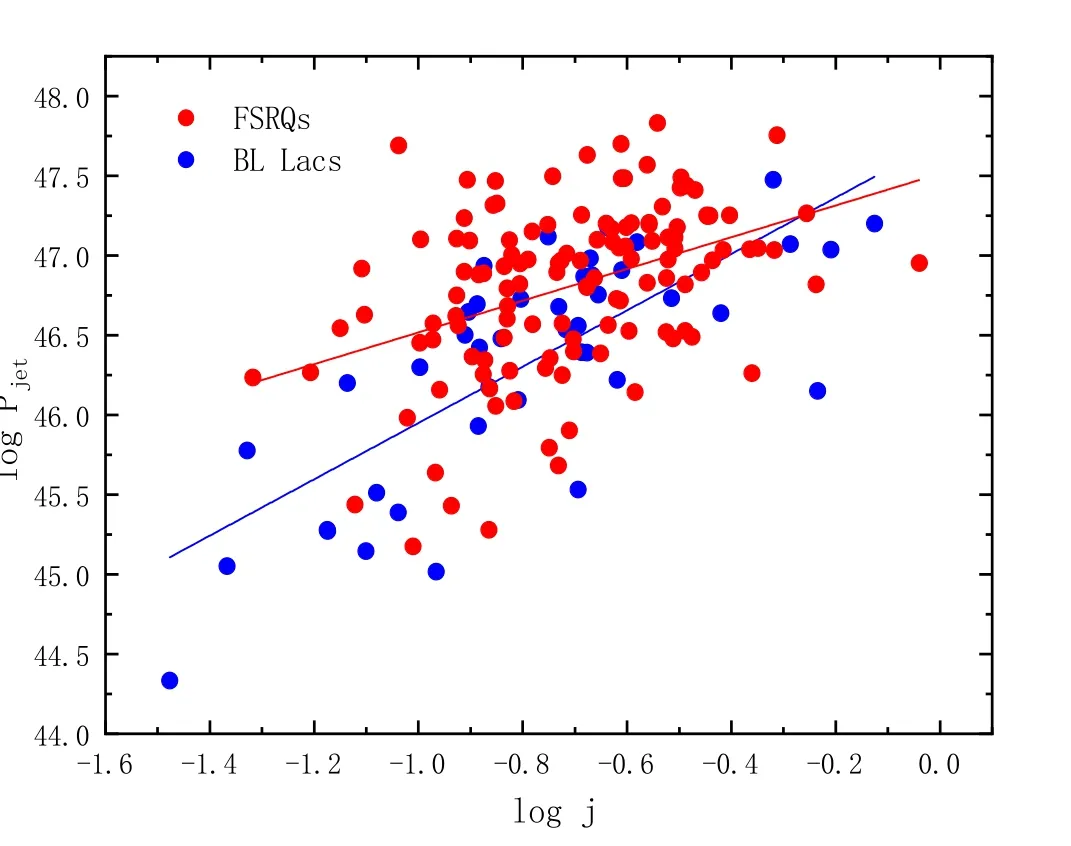

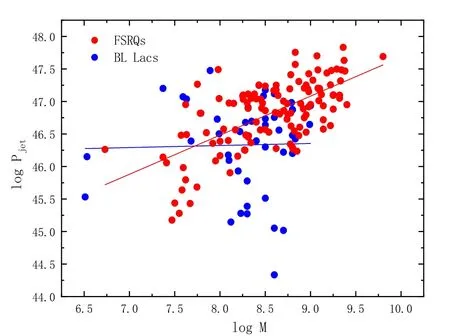
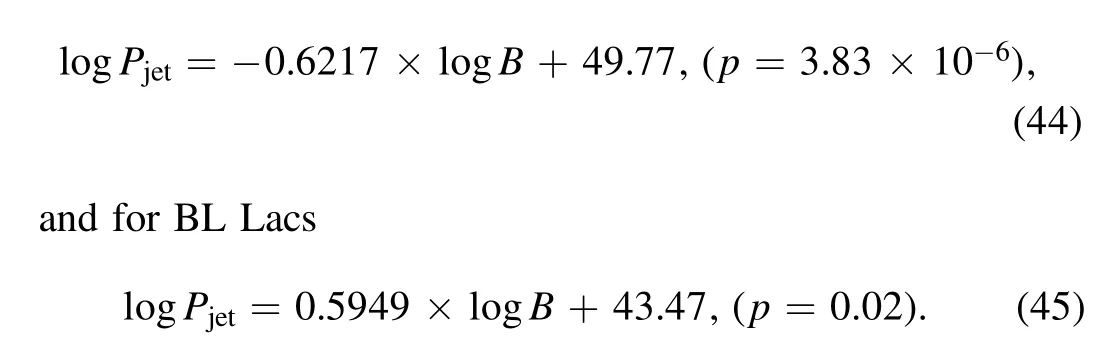

3.6.Generation Efficiency of Jet

4.Summary and Conclusions
 Research in Astronomy and Astrophysics2023年11期
Research in Astronomy and Astrophysics2023年11期
- Research in Astronomy and Astrophysics的其它文章
- Photometric and Spectroscopic Study of Two Low Mass Ratio Contact Binary Systems: CRTS J225828.7-121122 and CRTSJ030053.5+230139
- Injection Spectra of Different Species of Cosmic Rays from AMS-02, ACECRIS and Voyager-1
- The AIMS Site Survey
- A High-Temperature Superconducting Wideband Bandpass Filter at the L Band for Radio Astronomy
- Modified Masses and Parallaxes of Close Binary Systems: HD 39438
- Detecting HI Galaxies with Deep Neural Networks in the Presence of Radio Frequency Interference
