Research and experimental analysis of precision degradation method based on the ball screw mechanism①
KONG Deshun(孔德顺),GAO Xiangsheng∗,JIANG Huizeng②
Abstract As a key transmission component in computer numerical control (CNC) machine tools,the ball screw mechanism (BSM) is usually investigated under working load conditions.Its accuracy degradation process is relatively long,which is not conducive to the design and development of new products.In this paper,the normal wear depth of the BSM nut raceway is calculated under the variable speed operation condition using the fractal wear analysis method and the BSM's accelerated degradation proportional wear model.Parameters of the acceleration degradation model of the double-nut preloaded ball screw pair are calculated based on the physical simulation results.The accelerated degradation test platform of the BSM is designed and manufactured to calculate the raceway wear model when the lubrication condition is broken under the variable-speed inertial load and the boundary lubrication condition under the uniform speed state.Three load forces and two samples are selected for the accelerated degradation test of the BSM.The measured friction torque of the BSM is employed as the evaluation index of the accuracy degradation test.In addition,the life cycle of the accuracy retention is accurately calculated by employing the parameters of the physical simulation model of the BSM.The calculations mentioned above can be used to estimate BSM's accuracy performance degradation law under normal operating conditions.The application of the proposed model provides a new research method for researching the precision retention of the BSM.
Key words: ball screw mechanism (BSM),fractal theory,wear model,accelerated degradation method,friction torque,precision retention
0 Introduction
As a key part of the numerical control machine tool,the precision degradation process of the ball screw mechanism (BSM) under a normal working load is relatively long,which is not conducive to the design and development of new products.Most researchers use experimental methods to shorten the test cycle of precision degradation of BSM and explore BSM's failure mode or accuracy variation characteristics.The surface pitting corrosion,structural failure mode,and fatigue life of the BSM were experimentally studied by Shimoda et al.[1].Zhang et al.[2]explored the fast wear of the screw raceway based on the wear situation of the screw raceway and nut raceway by combining the mathematical modeling of lubrication and elastic contact stress.Fleischer et al.[3]used the adaptive lubrication method to improve BSM wear.Zhen and An[4]analyzed the influence law of ball dimensional tolerance,axial load,radial load,and dimensional ball accuracy on the service life of the BSM.Li et al.[5]proposed a systematic method of BSM prediction that can achieve fault diagnosis,early diagnosis,health assessment,and life prediction.Deng et al.[6]proposed the hybrid gated recurrent unit-particle filter (GRU-PF) method to achieve the life prediction of the BSM.These investigations are only qualitative research on the precision degradation characteristics of ball screw.
According to the structural parameters,performance degradation characteristics,and working conditions of the BSM,the performance evaluation of BSM was achieved by combining qualitative analysis and quantitative calculation[7-8].Shen[9]proposed a precision degradation model of BSM based on the contact deformation and motion law between the ball and the raceway of a double-nut preloaded BSM.Moreover,the author combined the model with the adhesive wear law and the quantitative relationship between working conditions,structural parameters,and precision degradation of the BSM.Chen et al.[10]analyzed the contact stress and the roller-slip relationship between the ball and the raceway.The test verified that the speed and the load greatly affect the relationship mentioned above.It was found that friction and wear were the main reasons for the precision decline of BSM.Zhou et al.[11]established the load distribution and velocity change models that can accurately predict the performance and life of the double-nut BSM.Liu et al.[12]used fractal geometry theory to analyze the microscopic morphology representation of raceway and ball surfaces.The authors combined the microscopic morphology representation of threaded groove surface and a multi-scale contact mechanics model.Lastly,the authors determined that the accuracy degree decline increased with the axial load and rotational speed.Zhao et al.[13]established a wear model to analyze the change law of wear depth.Moreover,the author combined the proposed model with Archard theory and the iterative interpolation algorithm and investigated the precision life degradation law of a double-nut ball screw.Similarly,Zhang et al.[14]proposed a BSM degradation model by using the wear amount,load,stroke,and experimental data.These investigations are based on detailed theoretical models to quantitatively study the precision life degradation mechanism of BSM,but it is difficult to accurately predict the precision degradation law of BSM.
The preload and friction torque are typically used as the evaluation indexes of the BSM's precision degradation.BSM wear will reduce the preload and friction torque.Firstly,the parameters of the accelerated degradation model are estimated via the maximum likelihood estimation method using the fractal adhesive contact wear model.The Weibull distribution function of accuracy evaluation under different stress levels was ensured to be consistent by employing the proportional wear model of the accelerated degradation of BSM[15-16].Secondly,the calculation method of raceway wear depth under ball screw transmission conditions is considered.Finally,the accelerated degradation method of the BSM is verified by using the accelerated degradation BSM test bench,providing a new method for the research on BSM accuracy retention.
1 BSM wear model
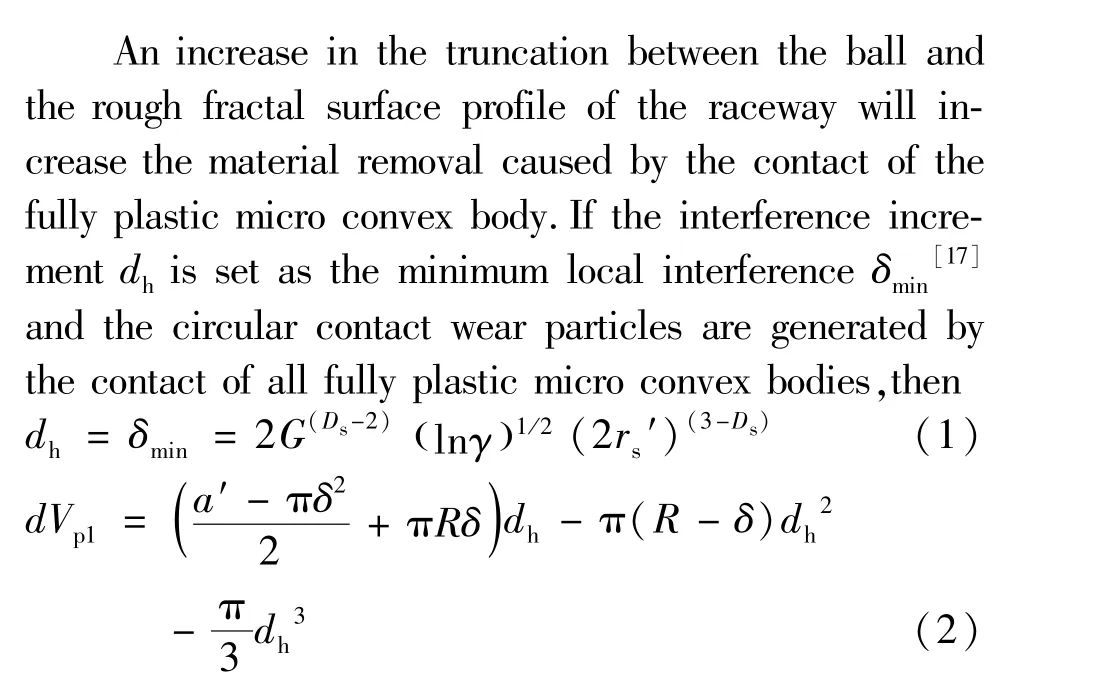
where,rs′is the truncation radius of the minimum micro-convex body contact,Ris the radius of the sphere of the removed table,a′is the area of the larger bottom surface,dhis the height from the larger to the smaller bottom surface,andδis the distance from the larger bottom surface of the table to the top of the entire ball.Therefore,the wear volume of a single micro-convex body can be approximated by the volume of the table as follows.

Without considering the influence of time, the wear amount of single contact on the entire contact surface can be calculated by integrating the following expression:


The raceway contact area wear is calculated by the semi-analytical method by considering the relative sliding of the ball and the raceway contact area meshing.It is assumed that the relative sliding velocity of the contact surface isvs,and the normal interference of the maximum micro convex body to be worn off isδc.According to Ref.[18], the number of the same micro bumps being worn off per unit of time (i.e.,the wear frequency of micro bumps) can be expressed as follows.
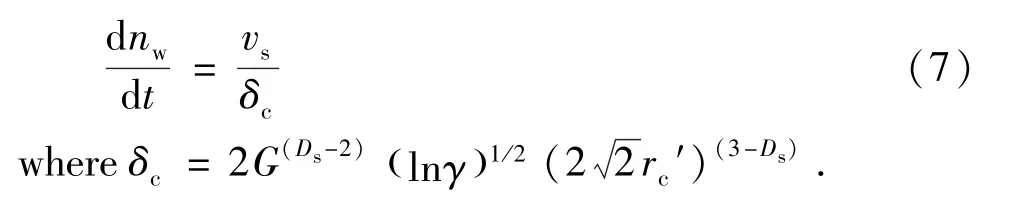

The wear volume of a single micro convex body per unit of time can be written as
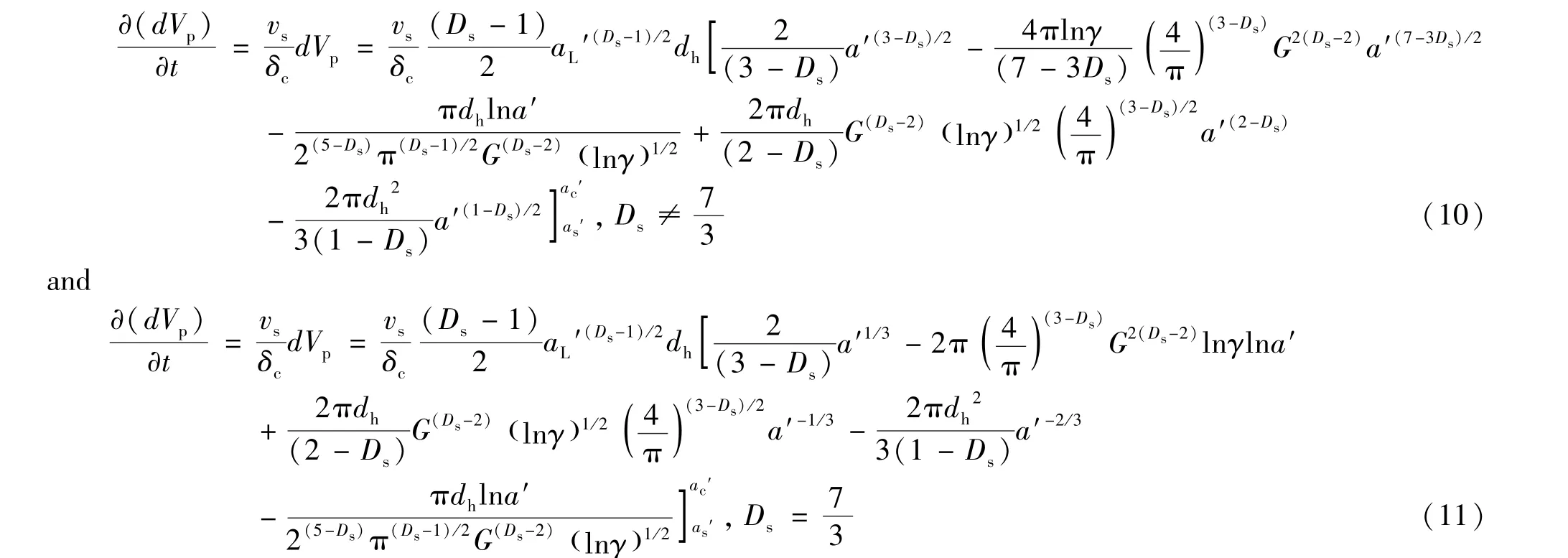
Therefore,the wear rate per unit area and per unit time can be expressed as
The motion between the ball and the raceway is compound motion characterized by five motion modes on the macro level[19].There are three relative angular velocity components(ωx,ωy,ωz) and two relative linear velocity components(vx,vy).The angular velocity between the ball and the raceway isωxandωy.The relative sliding linear velocity component between the ball and the raceway is denoted as (vx,vy) ,and the angular velocity relative to the spin is denoted asωz.Therefore,the relative sliding velocity of any point in the contact area can be expressed as

wherenbis the number of the working ball,tis the running time,Ss(j) is the linear length of the raceway,anddyis the grid spacing in the circular direction of the raceway section.
2 Stress analysis of oil film contact
The boundary lubrication state exists between the ball and the raceway during BSM operation,and the oil film and micro-convex body jointly affect the normal load in the contact area.The lubricating oil below the mean heighthmof the fractal surface does not flow,and the lubricating oil above the mean heighthmof the fractal surface is in the elastic fluid state.The discretized oil film thickness distribution in the contact area between the ball and the raceway can be expressed according to the isothermal point contact elastohydrodynamic lubrication theory[20].

Moreover,the normal elastic deformation of any point on the grid node in the contact region can be expressed as

whereη0is the dynamic viscosity value of the lubricating oil,ueis the coiling speed of the lubricating oil,αis the viscosity coefficient of the lubricating oil.Then,
The distribution value of oil film thickness in the contact area between the ball and the screw raceway under load can be obtained via Eqs (17) -(23).The total normal loadQAbetween the ball and the lead screw raceway is shared by the oil film loadQymand the micro-convexQwt.Then,
3 BSM wear analysis
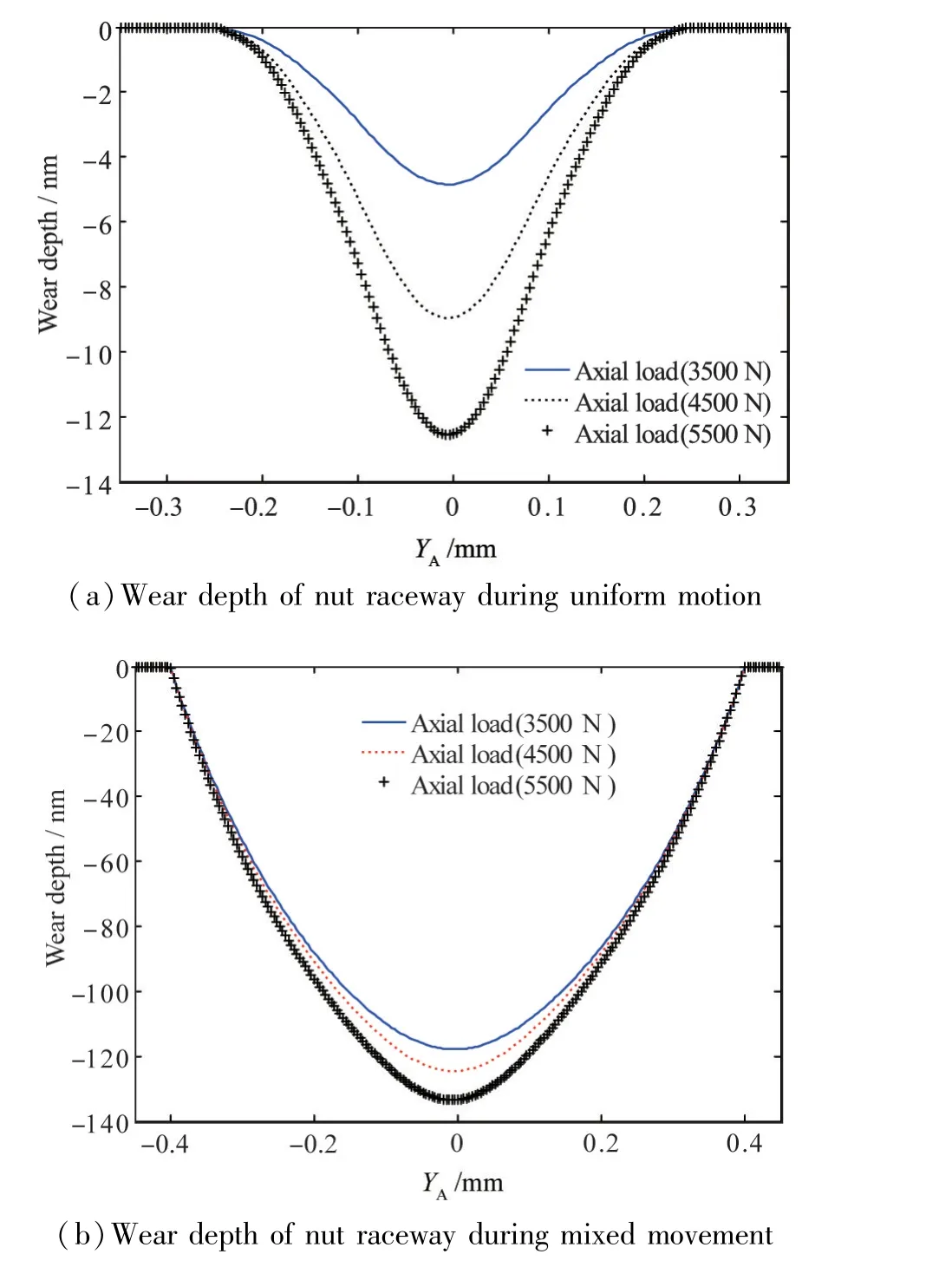
The motion forms of the ball screw can be divided into accelerated,uniform,and deceleration.The force of the contact point between the ball and the raceway is unbalanced during the acceleration and deceleration of the ball screw,and the lubrication is insufficient,intensifying the wear of the ball and the raceway.The raceway wear depth calculated according to Eq.(16) when the lead screw runs for 1 h under dry friction is shown in Fig.1.The raceway wear depth when the lead screw runs for 1 h under oil film lubrication is shown in Fig.2.According to Fig.1,when the contact between the ball and the raceway is characterized as dry friction,the wear depth significantly increases with the screw rotation speed.According to Fig.2,the nut wear depth increases with the screw speed.However,the nut raceway wear depth decreases when the screw speed increases.The main reason for this phenomenon is that the centrifugal force effect increases the oil film thickness,and the oil film jointly bears the normal load of the raceway.Hence,nut raceway wear is reduced due to the sufficient lubrication between the ball and the raceway.Moreover,the analysis of Figs 1 and 2 shows that the raceway wear's contact surface is narrow during film contact lubrication and wider during dry friction contact.The phenomena above can be attributed to the reduction of contact friction and wear between the ball and the raceway due to the oil film.
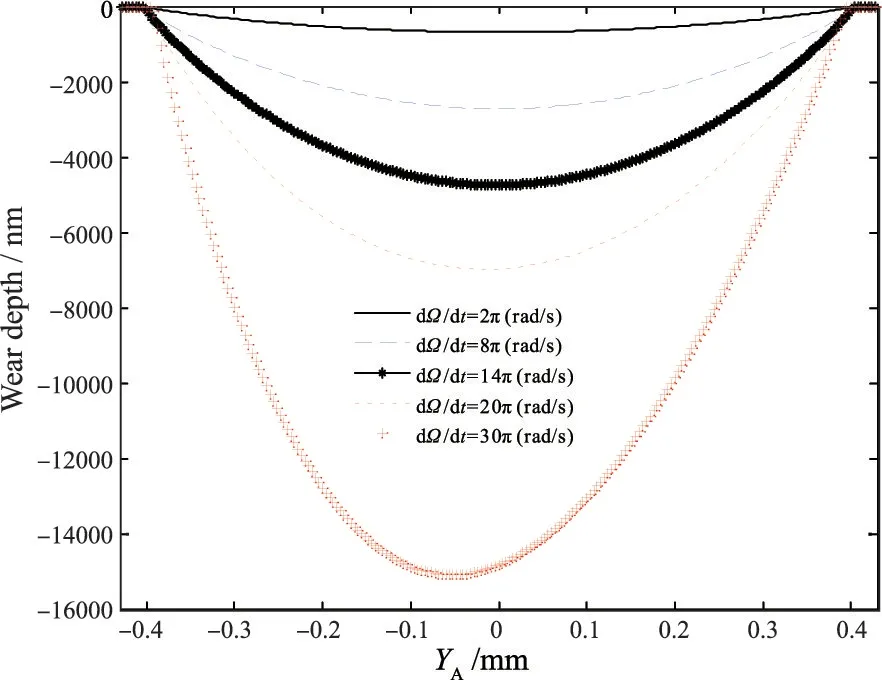
Fig.1 Distribution of the wear depth along the normal crosssection of the raceway in the same stroke(1 h)

Fig.2 Distribution of the wear depth along the normal crosssection of the raceway under lubrication condition in the same stroke(1 h)
The test bench for the ball screw pair was designed and processed to investigate the accuracy degradation law of the acceleration test for the ball screw pair,as shown in Fig.3.Moreover,the accuracy degradation law of the ball screw pair was explored under the following three working conditions.
(1)Uniform rotation (800 r/min) during the entire motion of the lead screw (under ideal conditions).
(2)The screw accelerated during the initial stage.When the speed increases to 800 r/min,the screw runs at a constant speed.Uniform deceleration movement is carried out before the end of the nut stroke.Lastly,the acceleration and deceleration values are all equal to 5 m/s2.
(3)When the nut runs to the middle position of the lead screw stroke,it starts to deaccelerate uniformly.The nut's acceleration and deceleration value is equal to 5 m/s2during the entire movement.
The raceway wear depth of the nut in the course of 1 km under the above three working conditions is shown in Fig.4.It can be seen that the raceway wear depth reaches the maximum value when the nut moves at variable speed throughout the entire running stroke.The raceway wear depth is minimal when the nut moves uniformly throughout the stroke.The main reason for this phenomenon is the unbalanced force and insufficient lubrication of the contact point between the ball and the raceway.
4 Design of BSM's accelerated degradation test
A composite exponential accelerated degradation model[15-16]was established to analyze the accuracy degradation characteristics of the ball screw.The test samples are double-nut preloaded BSM,whose parameters are shown in Table 1.The initial preload value is 1500 N,the number of test samples isn= 2,and the number of acceleration test load levels ish= 3.The maximum load value applied in the test does not exceed 4500 N(three times the preload) to ensure that the precision degradation mechanism of BSM does not change.Moreover,the load value applied in the test is 2000 N,3000 N,and 4000 N.According to the machining errors and installation and debugging differences of BSM,it can be concluded that the parameter variation of the ball screw is normally distributedN(0.5%).According to Ref.[15],the P-fractional precision life of the ball screw under normal working conditions is approximately equal toτp= 1.4246×107R.

Parameters Value Unit(I)Geometry parameters of the ball screw Screw pitch cycle radius r 16 mm Pitch L 10 mm Contact angle β 40.2600 o Helix angle α 5.6833 o Screw inner radius of screw rCS 16.182 mm Nut inner radius of screw rCN 15.818 mm Radius of curvature of the screw raceway rS 3.215 mm Radius of curvature of the nut raceway rN 3.215 mm Ball's radius rb 2.9765 mm Preload FWS 2562.8 N Number of balls nb 206 Density of the ball material ρ 7.8 g/cm3 Young's modulus E 211.1 GPa Poisson's ratio v 0.3(II) The experimental conditions Room temperature 20 ℃
Raceway wear degradation was calculated according to the compound exponential accelerated degradation model of BSM,as shown in Fig.5.For the same ball screw load,raceway wear increases nonlinearly with an increase in revolutions.Similarly,raceway wear increases with load under the same number of revolutions.The simulated friction torque degradation curve that considers the errorε ~ N( 0,0.052)distribution of the structural parameters of the ball screw is shown in Fig.6.
Statistical analysis method and least square method were used to carry out data fitting of BSM simulation results for the accelerated stepping degradation, as shown in Fig.7.Moreover,the accelerated degradation model of a compound exponential BSM was used for parameter estimation,and the estimated values ofβi,jandαare shown in Table 2.The friction torque rapidly decreases in the initial and final stages of BSM precision degradation for the following reasons.
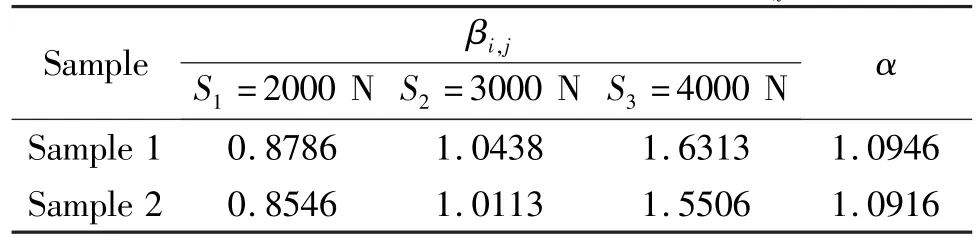
Sample βi,j S1 =2000 N S2 =3000 N S3 =4000 N α Sample 1 0.8786 1.0438 1.6313 1.0946 Sample 2 0.8546 1.0113 1.5506 1.0916
(1) There are burrs in the ball and the raceway in the early stage,which can be easily removed once the screw starts to run.

(2) The BSM preload degrades in the final stage,and the impact force of the ball movement in the raceway increases,aggravating the raceway wear.
Therefore,under the same operating conditions,the friction torque value of BSM in the initial stage,as opposed to the final stage,is greatly reduced.
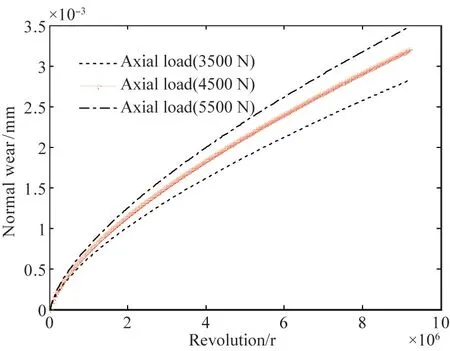
Fig.5 The average degradation curve of raceway wear

Fig.6 Degradation simulation value of friction

Fig.7 Fitting results of the degradation curve of friction torque
According to theβ-1i~Weibull(ηi,m)model andβi,jvalue[15],the maximum likelihood estimation method is used to estimate parametersηiandmi,as shown in Table 3.BSM accelerated degradation model parametersbB0andbB1are calculated using the least square method according to the value ofηiand the formulamk0=αmB,as shown in Table 4.According to the distribution parameterηiand the stress functionSi,buildaiand function of load are shown in Fig.8.

Fig.8 The relationship between ηi and Si

Parameter S1 =2000 N S2 =3000 N S3 =4000 N mi ηi 1.5535 1.48947 0.62570 3.52201

bB 0 bB 1-1.2327 0.9294
5 Analysis of the accelerated degradation test results of BSM
The test adopts the timing truncation loading mode to validate the BSM's compound exponential accelerated degradation model.The truncation (load holding)times for the three stages of the load level are 3.07×106R,3.07× 106R,and 3.07× 106R.The friction torque value was tested when the BSM ran at 2.9568×105Rto accurately verify the accuracy degradation law of BSM.During the test,the chamber temperature was 15 -40 ℃,oil lubrication model No.32 was used,and the BSM test sample model was GD3210-P3.The acquisition process of the friction torque of the BSM is shown in Fig.9.A constant screw rotation speed of 100 r/min was employed to accurately obtain the friction torque values of BSM at different stages of the precision degradation process.The variation curves of the friction torque at different stages are shown in Fig.10.According to the analysis in Fig.10,due to the existence of machining errors of ball screw,the friction torque value in the initial stage has a significant decline trend,but its change trend cannot truly reflect the accuracy degradation characteristics of ball screw.In the middle stage,the friction torque value of ball screw tends to decline gently and presents nonlinear changes.In the later stage,due to the wear of the ball screw,this causes the downward trend of the friction torque value of the ball screw to fluctuate greatly.

Fig.9 Friction torque test diagram of BSM

Fig.10 The degradation curve of friction torque
The precision degradation model of BSM[16]was used to fit the friction torque value according to the statistical analysis method of stepping accelerated degradation test data of BSM.The obtained degradation law of BSM friction torque is shown in Fig.11.It can be observed that the friction torque degradation curve of BSM can be used to accurately estimate its accuracy degradation characteristics,which is consistent with the predicted value of the simulation model.

Fig.11 Fitting results of the degradation curve of friction torque
The least square method was used to estimateβi,jandαparameters in the degradation model shown in Fig.11.The estimated values are shown in Table 5.

Sample βi,j S1 =2000 N S2 =3000 N S3 =4000 N α Sample 1 1.0610 1.3752 2.1608 0.4514 Sample 2 0.9125 0.9979 1.1034 0.3397


η1 η2 η3 m 0.9465 1.1343 0.8561 3.0451
Moreover, the maximum likelihood estimation method is used to estimate parametersbB0andbB1according to theβ-1i ~Weibull(ηi,m) model andηivalue[15],as shown in Table 7.

bB 0 bB 1-0.1059 0.0412
A double-nut preloaded BSM was used as a sample in the accelerated degradation test.The allowable value was set to 5 μm to control the reverse nut difference within the allowable range.The maximum allowable degradation of the friction torque of the BSM can be set to approximately 30% of the initial value.Moreover,the BSM operates under the condition that the external load value does not exceed 2500 N.According to the BSM and friction torque test values,the BSM normally operates under a load value ofS0= 1500 N.The friction moment degradation of the ball screw pair can be estimated according to Weibull distribution forηZ,0=1.7742×107R.The confidence interval of BSM samples is set to 90%,and the quantile asPfractile=0.1.Then,thePquantile time of BSM accuracy retention under normal operating conditions is obtained asτP= 1.341×107Rrevolutions.The BSM precision retention test life cycle error is 5.7% compared with the simulation value.Thus,the accuracy life cycle value of the BSM is acceptable according to the normal use of the ball screw.It can be concluded that the compound exponential accuracy degradation model of the ball screw pair selected in this paper can accurately reflect its accuracy degradation law.
6 Conclusions
In this paper,the BSM's compound exponential accelerated degradation model is established,and the model parameters are optimized.The maximum likelihood estimation method is used to estimate the accelerated degradation model parameters,ensuring the BSM accuracy under different stress levels and improving the accuracy of model parameters.
Firstly,the fractal wear analysis method is used to analyze the variation law of wear depth on the arc of the normal section of the BSM raceway under different operating conditions.The unbalanced force and insufficient lubrication of BSM under variable speed conditions are considered within the method.Moreover,the accelerated inertia load is accounted.Lastly,the lubrication failure and the boundary lubrication condition of uniform and variable speed are accounted.
An accelerated degradation test platform for ball screw accuracy retention is established.Three levels and two samples are used for the accelerated degradation test.The comparison between theoretical result and experimental result demonstrates that the friction moment of BSM is used as the evaluation index of ball screw accuracy degradation,providing a new research method for BSM accuracy retention investigations.
 High Technology Letters2023年3期
High Technology Letters2023年3期
- High Technology Letters的其它文章
- End-to-end aspect category sentiment analysis based on type graph convolutional networks①
- A wireless geophone based on STM32①
- Research on system combination of machine translation based on Transformer①
- The relationship between extra connectivity and t/k-diagnosability under the PMC model①
- Deep reinforcement learning based task offloading in blockchain enabled smart city①
- Design of two-dimensional spatially coupled LDPC codes for combating burst erasures①
