碳纤维增强环氧树脂复合板材的制备及其各向异性传热和弹性变形行为

江金鱼 何浩宇 黄磊 吴振鹏 董博闻

摘 要：采用模压成型技术制备了太阳能电池阵用碳纤维增强环氧树脂（CF/EP）复合板材，并通过光学显微镜、SEM及XRD对其宏观、微观结构进行表征。基于多物理场耦合计算软件Comsol建立了CF/EP复合板的固体传热数值模型，借助曲线坐标系转换算法揭示了热量于复合板碳纤维中优先沿纤维轴向传导，进而解释了碳纤维对CF/EP复合板导热性能提升的作用机制。并基于有限元计算软件Abaqus，建立了CF/EP的轴向应力-应变模型。研究发现在CF/EP的弹性变形过程中，沿平行于应力方向排列的碳纤维承担了绝大部分应力，且当应变率升至1%时，轴向碳纤维的内部应力最高可达54.1 MPa。

关键词：太阳能电池阵；碳纤维增强环氧树脂（CF/EP）；曲线坐标；固体传热；弹性变形

中图分类号：TB33

文献标志码：A

文章编号：1009-265X（2023）04-0111-08

收稿日期：2022－11－24

网络出版日期：2023－02－23

基金项目：国家自然科学基金项目（52201038），湖北省重点实验室开放课题（2021XY104），省级大学生创新创业项目（S202210920021）

作者简介：江金鱼（1983—），女，安徽池州人，副教授，硕士，主要从事非金属材料的性能分析方面的研究。

碳纤维增强环氧树脂（CF/EP）是以环氧树脂（EP）作为基体相，以碳纤维（CF）作为增强相复合制备得到的复合材料，具有高比模量、高比强度、低热膨脹系数及优良导电导热性等优异性能，是太阳能电池阵、人造卫星、电子仪表等电子器件中不可缺少的结构材料［1-3］。然而，CF/EP中的碳纤维在导热系数、弹性模量等方面均呈现明显的各向异性，导致其排列方向将显著影响CF/EP复合材料的导热及力学性能［4-6］。目前，相关学者围绕CF/EP复合材料的性能优化开展了大量基础研究［7-8］，分别探究了材料结构（例如增韧相浓度）、材料缺陷（例如孔隙、夹杂及裂纹）、纤维增强方式（例如纤维表面改性）及固化工艺对复合材料力学性能的影响，然而却极少关注CF的各向异性对材料性能的影响机制。同时，受服役条件变化及环境因素影响，CF/EP复合材料的各向异性也将对其传热、承载等性能产生更为显著的影响。因此，研究CF/EP复合材料中CF各向异性对材料性能的影响机制具有十分重要的意义。

鉴于此，本文拟针对实验制备的CF/EP复合板材，基于数值仿真技术进行相关复合板材的热传导及静力学分析，揭示CF在导热系数及弹性模量方面的各向异性对复合板材相关性能的影响机制。

1 实 验

1.1 实验材料

本文涉及的实验材料参数如表1所示。

1.2 实验方法

1.2.1 碳纤维预处理

首先将待使用的T-300型号PAN基碳纤维置于体积比1∶1的乙醇/丙酮溶液中进行回流处理，静置72 h以达到清洁碳纤维表面并脱除碳纤维表层上浆剂的效果；随后将碳纤维取出并用丙酮洗净置于干燥箱中干燥至恒重；进一步将干燥后的碳纤维置于50 ℃的去离子水中进行4 h的超声处理；最后将处理好的碳纤维置于干燥箱中于60 ℃干燥至恒重。

1.2.2 CF/EP复合板材制备

本实验采用正交刷搭配模压成型技术制备CF/EP复合材料，用作太阳能电池阵（见图1（a））的结构材料。将上一步中经过预处理的碳纤维平纹布剪裁成200 mm×150 mm尺寸并置于干燥箱中于60 ℃烘干并称重。以碳纤维：环氧树脂质量比3∶2的比例称取环氧树脂，并将树脂通过丙酮稀释至40%后向溶液中加入质量分数为12%的苯二胺，通过磁力搅拌器将溶液搅拌均匀。将以上配置好的树脂胶溶液通过正交刷涂的方式均匀涂覆到碳纤维平纹布表面，并将平纹布叠层排布（共4层）。将铺展好的碳纤维平纹布放置于平面模压模具中在变温条件下静置：室温（4～6 h）80 ℃（3 h）120 ℃（3 h）180 ℃（3 h），随后于加热器中自然冷却至25 ℃。静置固化过程中需每1.5 h进行一次加压，将产品最终厚度控制为2 mm。

1.3 CF/EP宏观、微观结构表征

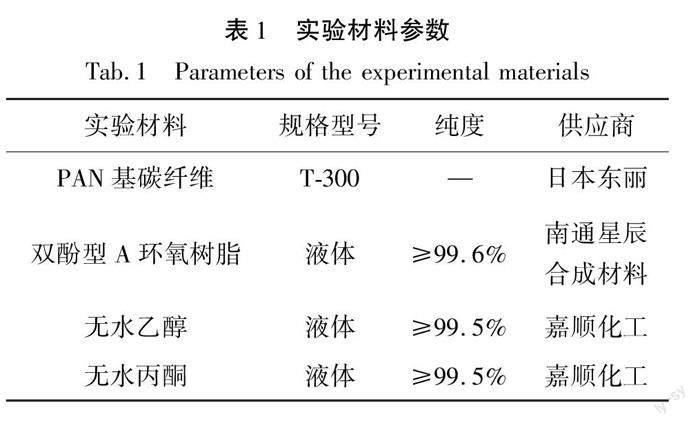
首先通过线切割对上一步中制备的CF/EP复合材料（见图1（b））进行取样，通过MEF-4A徕卡光学显微镜（Optical microscope， OM）对样品进行宏观结构表征；通过X射线衍射仪（XRD-6000）对CF/EP复合材料进行物相分析，扫描速率为2 （°）/min，扫描角度范围设置为20～70 ℃，随后通过MDI JADE 6晶体分析软件对材料进行物相分析（见图1（c））；最后通过ZEISS场发射扫描电子显微镜对碳纤维进行二次电子成像。

2 结果及分析

2.1 CF/EP复合板各向异性传热分析

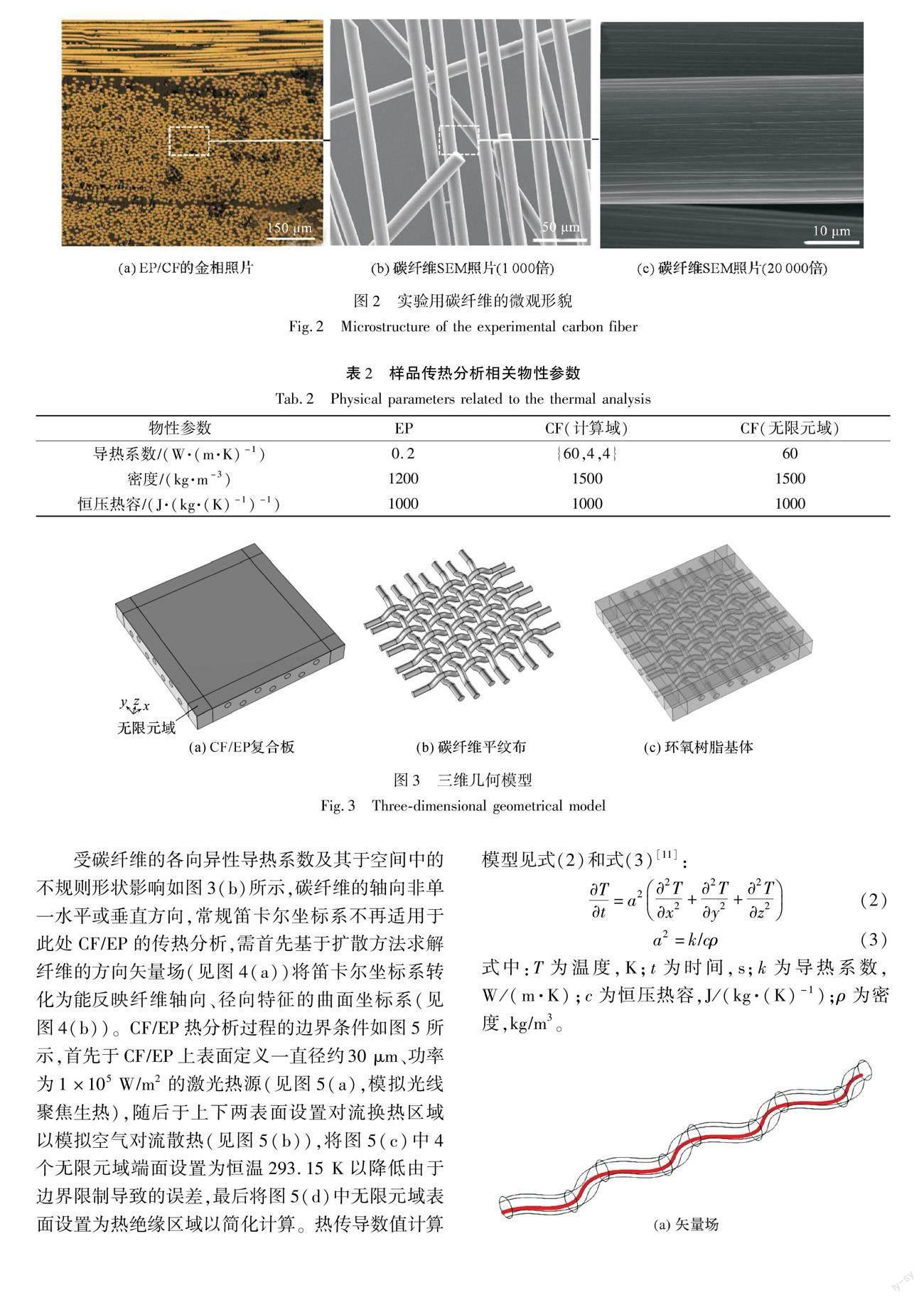
基于图2中CF/EP的宏观、微观结构表征结果，通过三维建模软件CREO构建如图2所示的单层碳纤维对应的CF/EP复合材料三维几何模型，并将其带入到后续有限元计算软件中，模型中碳纤维直径为15 μm，长度约300 μm，CF/EP复合板尺寸为300 μm×300 μm×40 μm。同时，由于所构建的三维模型尺度远小于实际CF/EP复合板尺寸，为减小模型边界造成的计算误差及与实际情况的偏差，需在传热计算前对模型边界区域划分无限元域，如图2（a）所示。

各样品的三维几何模型如图3所示，将图3中的单层碳纤维及环氧树脂基体模型合并后代入有限元计算软件Comsol中，基于固体传热数值模型进行传热分析。各样品传热分析模型相关物性参数如表2所示［10］，由于碳纤维的导热系数呈明显的各向异性趋势，即沿纤维轴向的传热系数远高于径向，因此其各向异性导热系数k需用式（1）中的二阶张量表示［9］：

k=kxxkxykxzkyxkyykyzkzxkzykzz=6000040004（1）

受碳纤维的各向异性导热系数及其于空间中的不规则形状影响如图3（b）所示，碳纤维的轴向非单一水平或垂直方向，常规笛卡尔坐标系不再适用于此处CF/EP的传热分析，需首先基于扩散方法求解纤维的方向矢量场（见图4（a））将笛卡尔坐标系转化为能反映纤维轴向、径向特征的曲面坐标系（见图4（b））。CF/EP热分析过程的边界条件如图5所示，首先于CF/EP上表面定义一直径约30 μm、功率为1×105 W/m2的激光热源（见图5（a），模拟光线聚焦生热），随后于上下两表面设置对流换热区域以模拟空气对流散热（见图5（b）），将图5（c）中4个无限元域端面设置为恒温293.15 K以降低由于边界限制导致的误差，最后将图5（d）中无限元域表面设置为热绝缘区域以简化计算。热传导数值计算模型见式（2）和式（3）［11］：

Tt=a22Tx2+2Ty2+2Tz2（2）

a2=k/cρ（3）

式中：T为温度，K；t为时间，s；k为导热系数，W/（m·K）；c为恒压热容，J/（kg·（K）-1）；ρ为密度，kg/m3。

CF/EP复合板在局部光源加热条件下的传热过程计算结果如图6所示，由图6（a）可见复合板上表面首先于光斑聚焦区域升温（光斑直径约为30 μm），上表面局部最高温度高于380 K，而远离光斑区域温度接近室温（293.15 K，初始值）。如图6（b）所示

为碳纤维内部温度场计算结果，可见热量由表层光斑区域传至碳纤维，进而沿碳纤维轴向优先传递，导致碳纤维轴向区域升温效果强于径向，即证明受碳纤维各向异性热传导系数影响，碳纤维中的热量会优先沿轴向传导。图6（c）－（d）所示为CF/EP复合板中间横截面温度场场及全局温度等值面计算结果，可见高温区域（图6（c）中黄色区域）主要沿着靠近光斑处碳纤维轴向分布。

碳纤维平纹布内部热通量如图7所示，从图7中可明显观测到热量优先沿着碳纤维的轴向方向传递，其径向方向热通量较低（与轴向热通量数值相差较大，因此未显示），这进一步证明了CF/EP复合板在局部受热条件下热量会优先沿碳纤维轴向方向传递至远离热源的低温区域，即揭示了碳纤维对于CF/EP导热性能提升方面的作用机制。

2.2 CF/EP复合板弹性变形分析

将2.1中建立的CF/EP复合板几何模型进一步代入到有限元计算软件Abaqus中进行静力学分析。采用C3D10四面体网格对相关几何模型进行网格划分，如图8为网格划分结果，随后于复合板一端面设置固定边界以模拟实际拉伸实验中的固定端，同时在与固定端面平行面设置模型边长1%的轴向应变率以模拟CF/EP复合板的实际拉伸实验。

如图9所示分别为不同应变率下EP基体和CF增强相中的应力分布计算结果，可以发现随着应变率增加，EP基体和CF增强相中均出现局部应力集中现象，但两者应力最大值相差较大，EP基体由于弹性模量低（约1 GPa），其上应力峰值小于0.7 MPa。而对于弹性模量较高的CF（T-300的拉伸模量约为240 GPa，压缩及剪切模量约8 GPa），在拉伸过程中的应力峰值高于50 MPa，且应力集中区域（即主要承担应力的部分）主要位于轴向与拉伸方向平行的CF上，而轴向与拉伸方向垂直的CF增强相承担的应力较低。由此可见，碳纤维的各相异性对CF/EP的导热及力学性能均会造成显著影响。

3 结 论

本文首先采用模压成型技术制备了太阳能电池阵用碳纤维增强环氧树脂CF/EP复合板材，并基于相关宏观、微观结构表征结果建立了可用于传热分析和静力学分析的数值仿真模型，通过传热分析发现在局部加热条件下CF/EP复合板材中的热量会优先沿CF的轴向方向由高温区域传导至远离热源

的低温区域，从而实现材料散热并提升复合材料的导热性能。进一步基于靜力学计算发现在复合板材弹性变形过程中，应力集中优先发生于与拉伸方向平行的CF中，且当应变率升至1%时，轴向碳纤维的内部应力最高可达54.1 MPa，由此证明CF能显著提升复合板材的力学性能。综上所述，本文揭示了碳纤维导热系数及弹性模量的各向异性对CF/EP复合板材的影响机制。

参考文献：

［1］胡丹丽，付大鹏，张越，等.配副材料对碳纤维增强环氧树脂复合材料摩擦学性能的影响［J］. 润滑与密封， 2022， 47（7）： 97-103.

HU Danli， FU Dapeng， ZHANG Yue， et al. Effect of tribopair matrials on tribological properties of carbon fiber reinforced epoxy composites［J］. Lubrication Engineering， 2022， 47（7）：97-103.

［2］刘熠晨，李颖杰，曹锦锋，等.碳纤维织物增强环氧树脂/石墨烯微片复合材料的导热及摩擦性能［J］.塑料，2022，51（4）：52-55，59.

LIU Yichen，LI Yingjie， CAO Jinfeng， et al. Preparation and performance research of epoxy resin/graphene microsheet/carbon fiber cloth composite［J］. Plastics， 2022， 51（4）： 52-55，59.

［3］李琴.废旧再生碳纤维增强环氧树脂复合材料力学性能及界面性能研究［J］.塑料科技，2022，50（2）：19-22.

LI Qin. Research on mechanical properties and interface properties of waste recycled carbon fiber reinforced epoxy resin composites［J］. Plastics Science and Technology， 2022， 50（2）：19-22.

［4］SCHOLLE P， SINAPIUS M. Influence of the electrical anisotropy of unidirectional carbon fiber reinforced plastics on their self strain sensing properties［J］. Composites Part C： Open Access， 2022， 8： 100256.

［5］KHUDIAKOVA A， BERER M， NIEDERMAIR S， et al. Systematic analysis of the mechanical anisotropy of fibre-reinforced polymer specimens produced by laser sintering［J］. Additive Manufacturing， 2020， 36：101671.

［6］張杰，宁荣昌，李红，等.碳纤维增强环氧树脂基复合材料的性能研究［J］.中国胶粘剂，2009，18（3）：21-25.

ZHANG Jie， NING Rongchang， LI Hong， et al. Study on performance of composite material based on epoxy resin reinforced by carbon fiber［J］. China Adhesives， 2009， 18（3）：21-25.

［7］罗锐祺，刘勇琼，廖英强，等.碳纤维增强环氧树脂复合材料力学性能影响因素的研究进展［J］.材料导报，2021，35（S2）：558-563.

LUO Ruiqi， LIU Yongqiong， LIAO Yinqiang， et al. Research progress on the influencing factors of mechanical properties of carbon fiber reinforced epoxy resin composites［J］. Materials Reports， 2021， 35（S2）：558-563.

［8］黄远，何芳，万怡灶，等.碳纤维增强环氧树脂基复合材料湿热残余应力的微Raman光谱测试表征［J］.复合材料学报，2009，26（4）：22-28.

HUANG Yuan， HE Fang， WAN Yizhao， et al. Testing and characterization of hygrothermal stresses in carbon-fibers reinforced epoxy composites using Raman spectroscopy［J］. Acta Materiae Compositae Sinica， 2009， 26（4）：22-28.

［9］WANG G N， GAO M Y， YANG B， et al. The morphological effect of carbon fibers on the thermal conductive composites［J］. International Journal of Heat and Mass Transfer， 2020， 152：119477.

［10］MAYBOUDI L S. COMSOL Heat Transfer Models［M］. Dulles， Virginia： Mercury Learning and Information， 2020.

［11］LEBON G， MATHIEU P. A numerical calculation of nonlinear transient heat conduction in the fuel elements of a nuclear reactor［J］. International Journal of Heat and Mass Transfer， 1979， 22（8）：1187-1198.

Preparation， anisotropic heat transfer and elastic deformation of CF/EP composite plates

JIANG Jinyu1a，1b， HE Haoyu1a， HUANG Lei1a， WU Zhenpeng1a， DONG Bowen1a，2

（1a.School of Mechanical and Electrical Engineering; 1b. Key Laboratory of Intelligent Transportation Technology and

Equipment of Hubei Province， Hubei Polytechnic University， Huangshi 435000， China; 2.School of Materials Science

and Engineering， Dalian University of Technology， Dalian 116000， China）

Abstract：

In this paper， carbon fiber reinforced epoxy （CF/EP） composite plates for solar arrays were prepared by molding technology， and the macrostructure and microstructure were characterized by optical and scanning electron microscopy. Based on the above characterization results， a three-dimensional geometric model of CF/EP composite plates was constructed and substituted into the subsequent heat transfer and mechanical numerical model for solution. Firstly， based on the multi-physics field coupling calculation software Comsol， the solid heat transfer numerical model of CF/EP composite plates was established to reveal the mechanism of the influence of carbon fibers′ anisotropy on the heat transfer behavior of the composite plates. Through the curve coordinate system conversion algorithm， the relevant geometric model was converted from the three-dimensional Cartesian coordinate system to a curve coordinate system which is more suitable to describe the axial/longitudinal properties of carbon fibers， and can simplify the calculation. The actual heat transfer behavior of CF/EP was simulated with the boundary conditions such as surficial heat source region and surface air convection area. A constant temperature area and a thermal insulation area were set to reduce the difficulty of the solution. The above mathematical models were further solved based on the finite element method. Based on the calculated temperature field diagram and the heat vector in CF/EP composite plates， it is found that heat was transmitted preferentially along the axial direction of carbon fibers during heating. However， the thermal conductivity of the fiber in the radial direction was low. The mechanism of carbon fibers in improving the thermal conductivity of CF/EP composite plates was thus revealed.

Further， the CF/EP three-dimensional geometric modelwas substituted into the mechanical numerical model， and the axial stress-strain model of CF/EP was established based on the finite element calculation software Abaqus. The research shows that， in the uniaxial tensile test along the fiber axis， the stress concentration occurs preferentially at the bonding interface between the carbon fiber and the resin matrix. The stress-strain relationship of CF/EP composite plates is calculated. It is found that during the initial elastic deformation of CF/EP， the carbon fibers arranged along the direction parallel to the stress bear most of the stress. When the strain rate rises to 1%， the internal stress of the axial carbon fibers can reach 54.1 MPa at most. It can be seen that the strengthening effect of carbon fiber reinforcement on the mechanical properties also presents obvious anisotropy.

In conclusion， based on the three-dimensional geometric model of the actual CF/EP composite plate， the anisotropic heat transfer and elastic deformation behavior model of CF/EP was constructed by combining the physical properties of carbon fibers and epoxy resins. The finite element method was used to solve the above models. The strengthening mechanism of carbon fibers on the heat transfer and mechanical properties of the epoxy resin matrix was revealed. This work can serve as a positive theoretical significance for the subsequent production， preparation and performance optimization of high-performance CF/EP composites and other carbon fiber-reinforced composites.

Keywords：

solar array; CF/EP; curve coordinate; solid heat transfer; elastic deformation