一些图类的复合图的几何算数指标

阿斯牙·米吉提

一些图类的复合图的几何算数指标

阿斯牙·米吉提

（喀什大学 数学与统计学院，新疆 喀什 844800）

给出了２条路，２个圈以及１条路和１个圈的复合图的第一几何算数指标和第二几何算数指标的精确表达式．

复合图；第一几何算数指标；第二几何算数指标

1 引言及预备知识

拓扑指标是源于相应分子图的分子结构的数值描述．拓扑指标在化学和药理学等学科中发挥着重要的作用[1]．许多拓扑描述已经在理论化学中得到了应用，特别是在QSPR/QSAR研究中[2-3]．

本文讨论２条路，２个圈以及１条路和１个圈的复合图的第一几何算数指标和第二几何算数指标，得到了第一几何算数指标和第二几何算数指标的精确表达式．

2 主要结果及证明

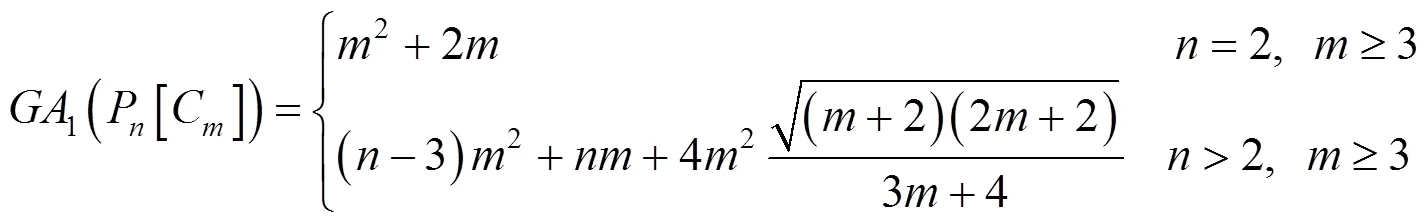
证毕．

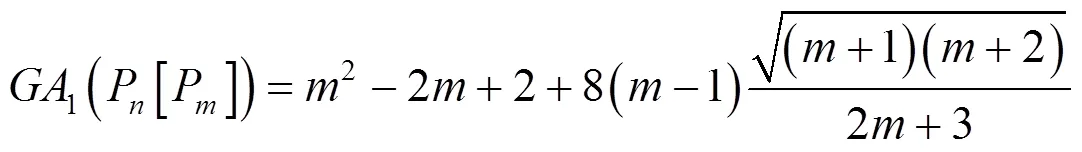
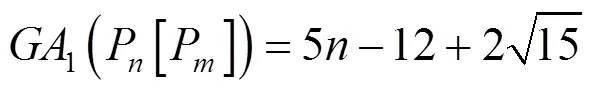
证毕．

证毕．

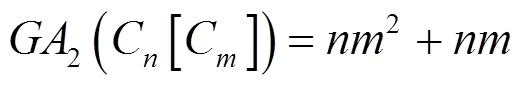
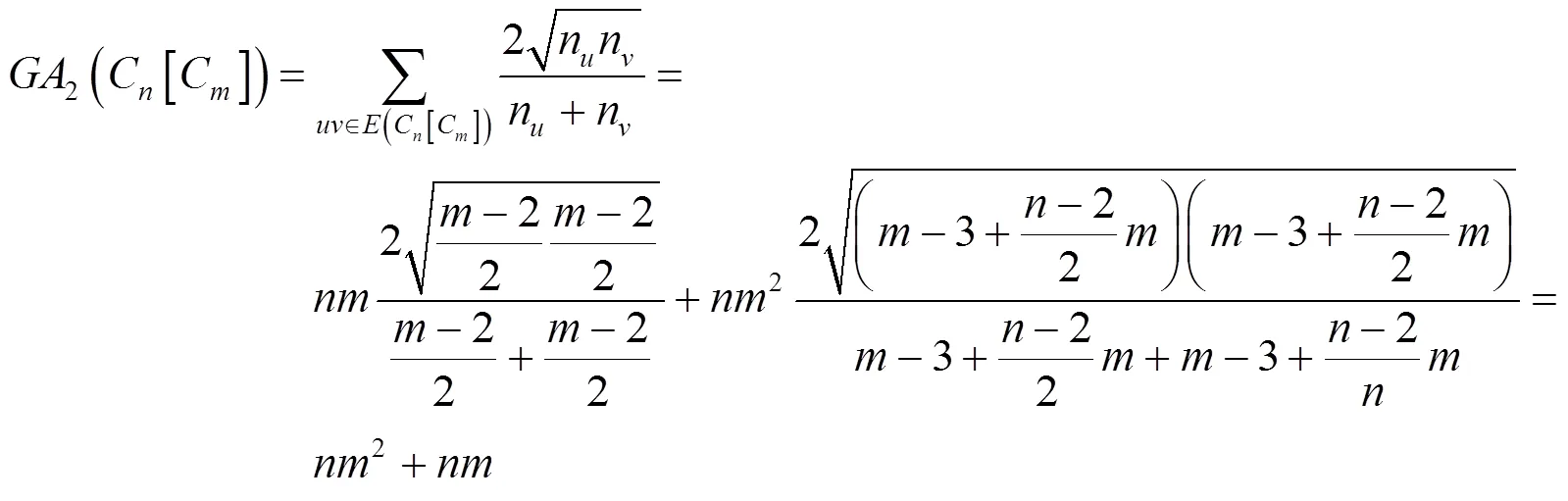
证毕．

3 结语

本文主要讨论了２条路，２个圈以及１条路和１个圈的复合图的第一几何算数指标和第二几何算数指标，并得到了这些图类的复合图的第一几何算数指标和第二几何算数指标的精确表达式．但关于一般的２个图的复合图的第一几何算数指标和第二几何算数指标的确定值还是一个有待解决的问题．希望能在将来开展更深入的研究，得到这个问题的解答．

[1] Todeschini R，Consonni V．Handbook of Molecular Descriptors[M]．Wiley-VCH：Weinheim，2000．

[2] Khadikar P V，Karmarkar S，Agrawal V K．A Novel PI Index and Its Applications to QSPR/QSAR Studies[J]．Journal of Chemical Information and Modeling，2001，41：934-949．

[3] Pogliani L．From molecular connectivity indices to semiempirical connectivity terms：recent trends in graph theoretical descriptors[J]．ChemInform，2000，31（51）：3827-3858．

[4] Vukievi D，Furtula B．Topological index based on the ratios of geometrical and arithmetical means of end-vertex degrees of edges[J]．Journal of Mathematical Chemistry，2009，46（4）：1369-1376．

[5] Fathtabar G，Furtula B，Gutman I．A new geometric arithmetic index[J]．Journal of Mathematical Chemistry，2010，47（1）： 477-486．

Geometric-arithmetic index of composition graphs of some graphs

ASIYA Mijiti

（School of Mathematics and Statistics，Kashgar University，Kashgar 844800，China）

The exact expressions was given for the first and second geometric-arithmetic indices of the composition graph with two paths，two cycles and one paths and one cycle．

composition graph；first geometric-arithmetic index；second geometric-arithmetic index

1007-9831（2022）10-0016-05

O157.5

A

10.3969/j.issn.1007-9831.2022.10.005

2022-04-10

新疆自治区自然科学基金项目（2022D01A14）；喀什大学校级科研项目（20212745）

阿斯牙·米吉提（1994-），女，新疆喀什人，助教，硕士，从事图论研究．E-mail：1779812132@qq.com