Electromagnetic wave absorption properties of Ba(CoTi)xFe12−2xO19@BiFeO3 in hundreds of megahertz band

Zhi-Biao Xu(徐志彪) Zhao-Hui Qi(齐照辉) Guo-Wu Wang(王国武) Chang Liu(刘畅)Jing-Hao Cui(崔晶浩) Wen-Liang Li(李文梁) and Tao Wang(王涛)

1Key Laboratory of Magnetism and Magnetic Materials of the Ministry of Education,Lanzhou University,Lanzhou 730000,China

2The Institute of Effectiveness Evaluation of Flying Vehicle,Beijing 100089,China

Keywords: microwave absorption,M-type ferrite,high permeability,impedance matching

1. Introduction

Nowadays, the rapid development of microwave technology and the widespread popularity of electronic communication equipment have brought convenience to human life.[1]The leakage from EM waves has also introduced excessive pollution to the environment, which threatens the physical and mental health of human.[2]Various EM wave absorption materials with excellent properties have been developed to eliminate the adverse effects of excessive EM radiation on human life.[3–6]These materials include dielectric materials[7–11]such as carbon materials,[12,13]nonmagnetic sulfides,[14]conjugated polymers[15]and magnetic materials such as ferrites,[16–19]magnetic metals,[20–22]and rare earth alloys.[23,24]However, most studies have mainly focused on EM wave absorption materials in the gigahertz range,whereas studies on absorption materials working in the hundreds of megahertz range are relatively rare.[25–27]This means that serious problems of EM pollution in the hundreds of megahertz band have not been well solved.[28]In this frequency range,effective absorption is difficult due to the long wavelength and insufficient dielectric or magnetic loss.[29–31]It is a serious challenge for researchers to explore materials with excellent absorption properties in the hundreds of megahertz bands.

Ferrite materials have attracted much attention of researchers as good EM wave absorption materials.[32]Among them, M-type barium ferrites (BaFe12O19) have been deeply investigated as an absorption material working in the gigahertz range because they have suitable permittivity, high stability, low density, and low cost.[33]However, the high resonance frequency and low permeability of M-type ferrites are not conducive to their absorption properties in the hundreds of megahertz band.[34]Related reports have indicated that co–substitution of trivalent iron ions in M-type ferrites by divalent(Co2+,Mn2+,Zn2+,Ni2+)and tetravalent(Ti4+,Sn4+,Zr4+,Ir4+)cations is a proven method for increasing the permeability and decreasing the resonance frequency.[35]Among these,Co2+–Ti4+co-substitution is considered as the most effective method for adjusting the absorption properties because that the substitution of Co2+and Ti4+for Fe3+in barium ferrite can not only dramatically increase the permeability but also increase the magnetic loss in range of hundreds of megahertz.[36]

In this work,Ba(CoTi)xFe12−2xO19with high permeability in hundreds of megahertz was obtained by substituting Fe3+distributed in five different lattice sites in BaFe12O19with Co2+and Ti4+. The permittivity was optimized by the BiFeO3dielectric phase formed by doping with Bi2O3, thus obtaining superb impedance matching. The EM wave absorption properties for Ba(CoTi)xFe12−2xO19@BiFeO3were studied in detail and its EM wave absorption mechanism was deeply discussed in hundreds of megahertz range.

2. Experiment

2.1. Fabrication

The target Ba(CoTi)xFe12−2xO19ferrite ceramics withx= 0.9, 1.0, 1.1, 1.2, 1.3, and 1.4 were prepared by the conventional solid-state reaction method. Analytical grade BaCO3(AR grade,≥99%),Co2O3(AR grade,≥99%),TiO2(AR grade,≥99%), and Fe2O3(AR grade,≥99%), were mixed and ball milled in a planetary ball mill for 12 h. Then,the powders after ball milled were desiccated and pre-sintered at 1100◦C for 6 h in a tube furnace under the air atmosphere.The pre-sintered powders were secondly ball milled for 12 h with 5 wt%Bi2O3in the same mill grinder. After drying,the powders were granulated by using 10.0 wt%polyvinyl alcohol(PVA)adhesive. Finally,the granulated particles were pressed at 1000 MPa for 10 min to form ring samples with an inner diameter of 3.04 mm,an outer diameter of 7 mm,and a thickness of 2–3 mm. The ring samples were sintered in the same furnace at 935◦C for 6 h to form bulk samples.

2.2. Characterization

The crystal structure of the bulk samples was characterized by x-ray diffraction (XRD) with a Cu-Kαradiation source. The surface morphologies of bulk samples were characterized by using a scanning electron microscopy(SEM).The hysteresis loops of the bulk samples at room temperature were measured by using a vibrating sample magnetometer(VSM)to characterize the static magnetic properties of the material.The EM parameters of the bulk samples were measured at room temperature in a frequency range from 1 MHz to 1 GHz by using an impedance analyzer.

3. Results and discussion

3.1. Structural characterization

The XRD patterns of Ba(CoTi)xFe12−2xO19bulk samples with variousx(x=0.9, 1.1, 1.2, 1.3, and 1.4) are shown in Fig. 1(a). The results indicate that the crystal structure does not change significantly with the variation of Co2+–Ti4+content. The standard PDF cards and the crystal face index of the diffraction peaks are shown in Fig.1(b). It can be clearly seen that the XRD patterns of bulk samples with various Co2+–Ti4+content show diffraction peaks that belong to two different phases. Some strong diffraction peaks are the M-type barium ferrite Ba(CoTi)xFe12−2xO19with hexagonal ferrite structure (PDF#39-1433), and the other diffraction peaks marked with red symbols are the BiFeO3with a typical rhombohedral perovskite structure (PDF#20-0169). Except for the two phases,no other impurity phases are detected in the XRD patterns of all bulk samples. This indicates that the addition of Bi2O3does not affect the formation of Ba(CoTi)xFe12−2xO19phase,[37]and the Bi2O3just combines with Fe3+to form Bi–Fe compound(BiFeO3).[38]Then, the BiFeO3enters into the interstice between the Ba(CoTi)xFe12−2xO19particles.[39]

The lattice parameters of the bulk samples change with the substitution of Fe3+by Co2+–Ti4+. Among them, lattice constantsaandcof the bulk samples are calculated using different diffraction peaks in the XRD patterns. The formula is as follows:

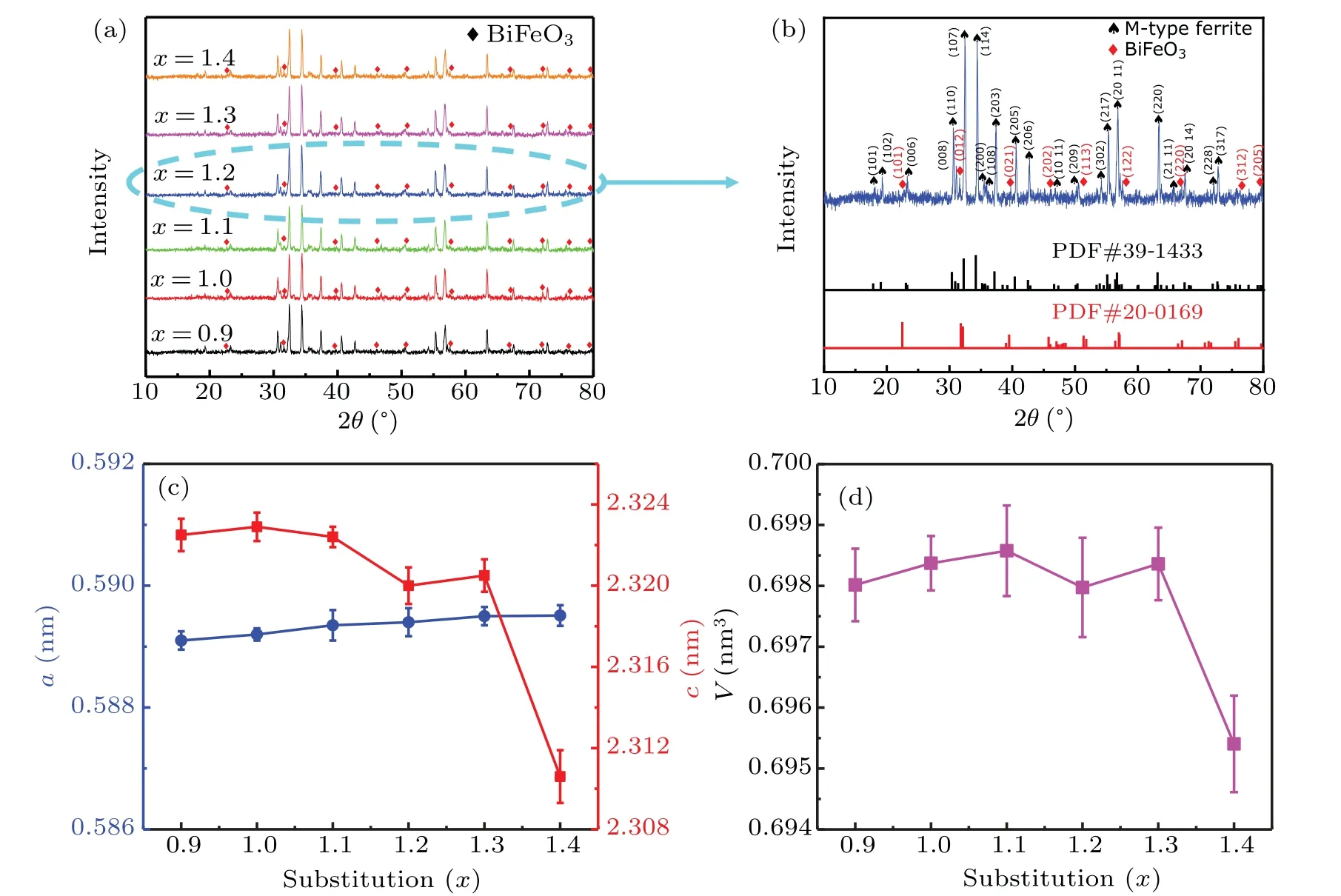
Fig.1. (a)XRD patterns of the bulk samples with various x(x=0.9,1.0,1.1,1.2,1.3,1.4). (b)The standard cards and the crystal face index of the diffraction peaks. (c)Lattice constants a and c for the bulk samples with various x. (d)Cell volume V for the bulk samples.

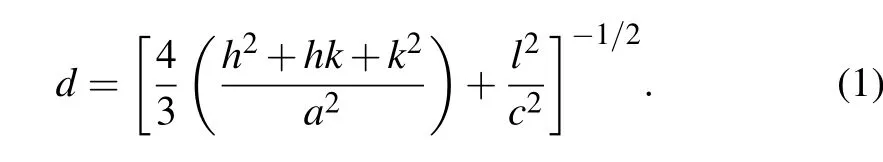
Figure 1(c)shows the variation of lattice constantsaandcas a function of Co–Ti contentx.It can be seen that there is a slight and steady increase of the lattice constantawith Co–Ti substitution from 0.5891 nm to 0.5895 nm. The lattice constantschas an overall decreasing trend with increasingx. The cell volumeValso has an overall decreasing trend,asxis changed from 0.9 to 1.4, and the result is in good agreement with researches conducted by Liet al.[45]

3.2. Morphological properties

Figures 2(a)–2(f) show the cross-sectional SEM images of bulk samples with various Co2+–Ti4+content. The SEM images show that the particle size of Ba(CoTi)xFe12−2xO19@BiFeO3does not change significantly with the increase of Co2+–Ti4+content. The particle size is approximately 500 nm–2 µm in all bulk samples. The small particle size can be attributed to the liquid phase effect induced by the addition of excess Bi2O3during the sintering process.[40,41]Figure 2(g) shows the local magnified view of the cross section for the bulk sample, where the part marked by the yellow dotted line is Ba(CoTi)xFe12−2xO19particles and the part marked by the blue dotted is BiFeO3.It can be seen that the Ba(CoTi)xFe12−2xO19particles are coated by the BiFeO3formed during the sintering process.Figures 2(h)–2(i) show the elemental mapping images of Ba(CoTi)xFe12−2xO19@BiFeO3bulk samples. The elements Ba, Fe, Co, and Ti are distributed uniformly inside the bulk samples. The element Bi is observed mainly at the surface of Ba(CoTi)xFe12−2xO19particles. It is further proved that BiFeO3is coated on the surface of Ba(CoTi)xFe12−2xO19particles.

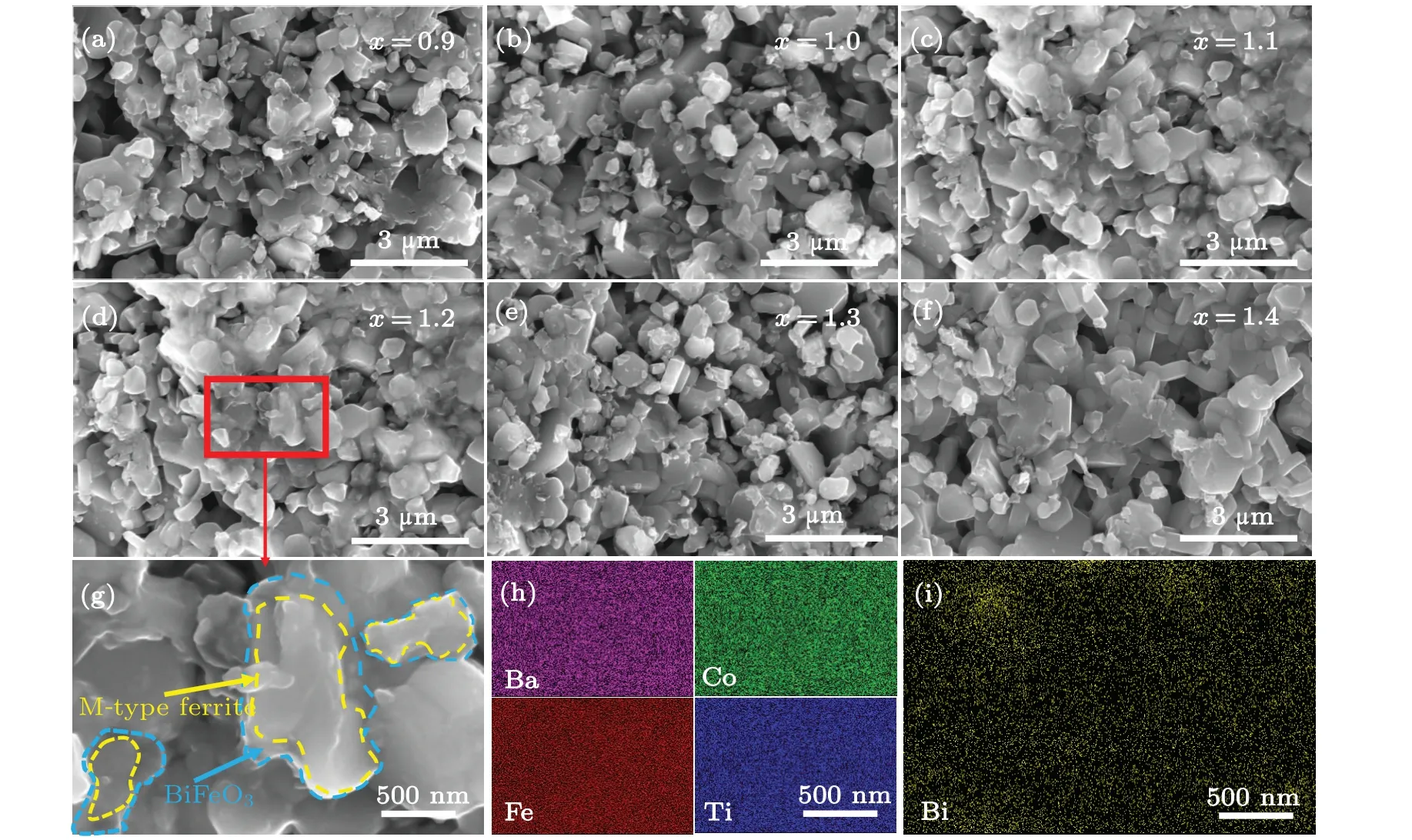
Fig.2. (a)–(f)The SEM images of Ba(CoTi)xFe12−2xO19@BiFeO3: (a)x=0.9,(b)x=1.0,(c)x=1.1,(d)x=1.2,(e)x=1.3,and(f)x=1.4. (g)The local magnified view of the cross section for the bulk sample. (h)–(i)The elemental mapping images of Ba(CoTi)xFe12−2xO19@BiFeO3.

3.3. Static magnetic properties

The hysteresis loops of the Ba(CoTi)xFe12−2xO19@BiFeO3bulk samples with variousxare shown in Fig.3(a).The results show that bulk samples exhibit typical soft-magnetic properties with the variation ofxvalues. The variation of saturation magnetization (Ms) and coercivity (Hc) with differentxare shown in Fig. 3(b).Msdecreases slightly with the increase ofx.Hcdecreases rapidly with increasingxfrom 0.9 to 1.1.Asxcontinues to increase,Hcalso decreases slowly. The static magnetic properties of Ba(CoTi)xFe12−2xO19@BiFeO3mainly depend on the magnetic phase Ba(CoTi)xFe12−2xO19.In the basic structure of Ba(CoTi)xFe12−2xO19, Co2+ions first preferentially occupy the 2aand 2blattice sites and Ti4+ions occupy 2aand 4f2lattice sites.[42]The ions at the 2aand 2blattice sites have an upward spin direction,while the ions at the 4f2lattice sites have a downward spin direction.[43]Msis determined by the magnetic moment of each metal cation and the amount of magnetic ions in each lattice site.[44]Therefore, asxincreases from 0.9 to 1.4,Msof the Ba(CoTi)xFe12−2xO19@BiFeO3bulk sample decreases slightly. In addition, based on the S–W theory,Hc≈2K1/µ0Ms.[45]When the value ofxis low, Co2+–Ti4+ions first preferentially occupy the 2a,2b,and 4f2.This causes the magnetocrystalline anisotropy constant (K1) value to decrease rapidly, thenHcdecreases rapidly. Asxcontinues to increase, more Co2+–Ti4+ions occupy the 2aand 4f1sites,which leads to a slight decrease inK1value and a corresponding slow decrease inHc.

Fig.3. (a)Magnetic hysteresis loops of Ba(CoTi)xFe12−2xO19@BiFeO3 with various x values. (b)Effects of Co2+–Ti4+ content on Ms and Hc of Ba(CoTi)xFe12−2xO19@BiFeO3.

3.4. Complex permittivity and permeability

The dependence of complex permittivity and permeability on frequency of Ba(CoTi)xFe12−2xO19@BiFeO3with variousxare shown in Fig.4.The real parts(ε′)of the permittivity of all samples at 1 MHz–1 GHz are about 20,while the imaginary parts(ε′′)are almost 0.For the samples withx=0.9,1.0,1.1,1.2,1.3,and 1.4,the real parts(µ′)of the permeability at 1 MHz can reach 2.51, 3.53, 15.46, 20.86, 16.38, and 13.49,while the imaginary parts(µ′′)exhibit obvious peak between 100 MHz and 1 GHz for the samples withx=1.1, 1.2, and 1.3. The above measurement results show that the substitution of Co2+–Ti4+does not have a significant effect on the permittivity of Ba(CoTi)xFe12−2xO19@BiFeO3, while it has a significant effect on the permeability.[46]Theµ′firstly increases and then decreases with the increase ofx. Notably, the highestµ′of Ba(CoTi)xFe12−2xO19@BiFeO3is about 20.86 whenx=1.2,which is almost equal toε′.

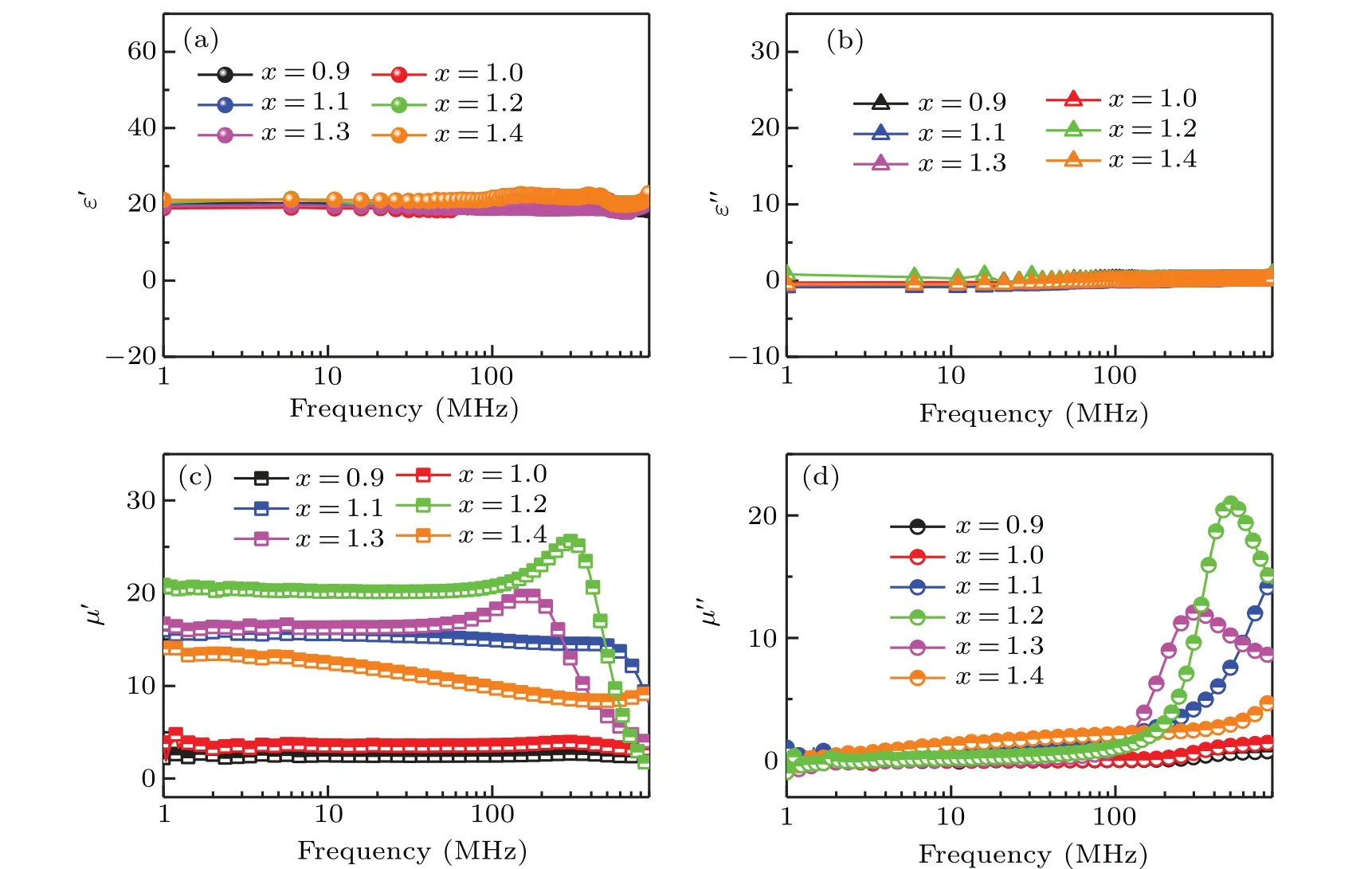
Fig. 4. (a) and (b) The complex permittivity of Ba(CoTi)xFe12−2xO19@BiFeO3 with various x, (c) and (d) the complex permeability of Ba(CoTi)xFe12−2xO19@BiFeO3 with various x.

The complex permeability of Ba(CoTi)xFe12−2xO19@ BiFeO3mainly depends on two types of moment dynamics. One is the domain wall motion, and the other is the spin rotation. To further study the contribution of these two mechanisms to the complex permeability, the measured complex permeability spectrum is fitted by the domain wall motion and spin rotation mechanism

whereχdis the static susceptibility provided by the domain wall motion mechanism,ωdis the angular frequency of the domain wall resonance,βrepresents the damping factor of the domain wall motion mechanism,χsis the static susceptibility of the spin rotation mechanism,ωsis the angular frequency of the natural resonance,αrepresents the damping factor of the spin rotation mechanism,andωis the angular frequency of the applied magnetic field(ω=2π f).

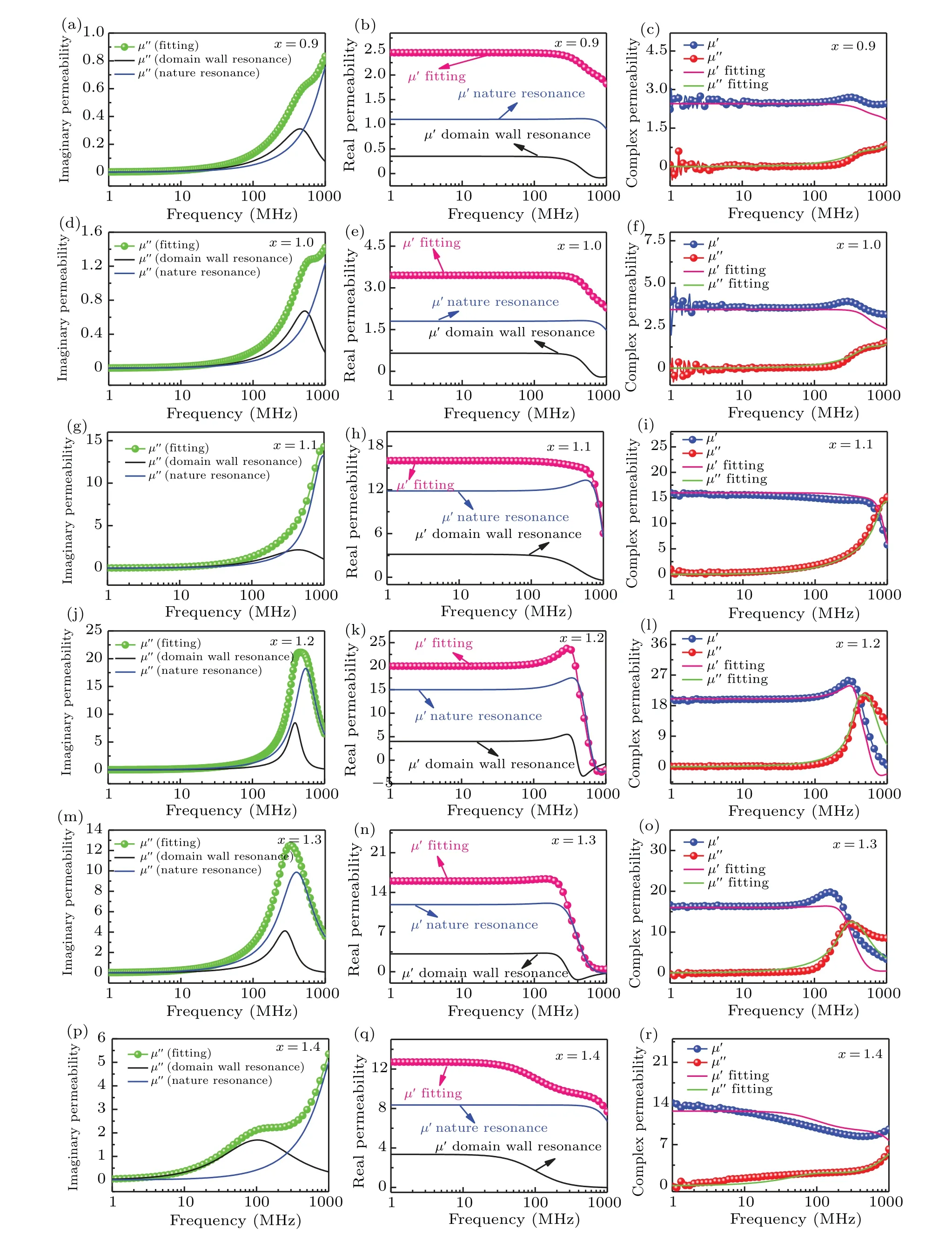
Fig. 5. Theoretical imaginary and real part of the permeability dispersion spectra, and the comparison of theoretical and measured complex permeability dispersion spectra;(a)–(c)x=0.9,(d)–(f)x=1.0,(g)–(i)x=1.1,(j)–(l)x=1.2,(m)–(o)x=1.3,(p)–(r)x=1.4.

Firstly, the above mentioned parameters can be obtained to simulate the measured imaginary part of the permeability spectrum by using Eq. (3). The simulation results are shown in Figs. 5(a), 5(d), 5(g), 5(j), 5(m), and 5(p). These parameters were then substituted into Eq.(2)to obtain the real part of the permeability spectrum, as shown in Figs.5(b), 5(e), 5(h),5(k), 5(n), and 5(q). The measured and simulated curves are shown in Figs.5(c),5(f),5(i),5(l),5(o),and 5(r),respectively.The good overlap between the fitted and measured permeability amply proves that the magnetization process includes domain wall motion and spin rotation mechanisms. According to the fitted results, the spin rotation component significantly affects the permeability dispersion spectra compared to the domain wall movement component.

3.5. EM wave absorption properties

The EM wave absorption properties of Ba(CoTi)xFe12−2xO19@BiFeO3can be characterized by itsRL–fcurves. Based on the transmission line theory,theRL–fcurves with a certain thickness can be calculated from the complex permeability and permittivity by the following equation:[47]

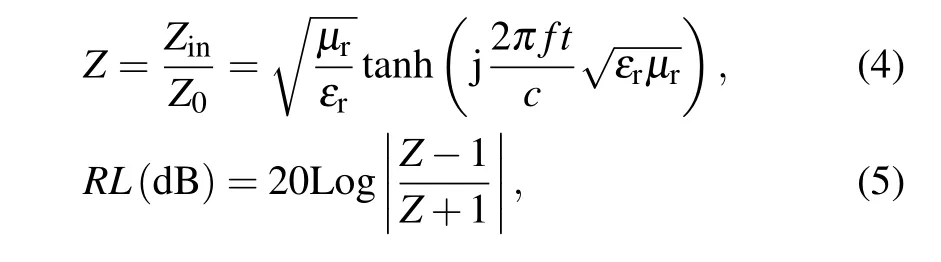
whereZinis the input impedance,Z0is the impedance in free space,µrandεrare the complex permeability and permittivity, respectively,fis the frequency of the EM wave,tis the thickness of the absorber,andcis the velocity of light in free space.

Figures 6(a)–6(f) show the thickness dependence of theRL–fcurves for the Ba(CoTi)xFe12−2xO19@BiFeO3with variousx. As shown in Figs. 6(a) and 6(b), the samples withx= 0.9 and 1.0 display poor EM wave absorption properties with the minimum value of reflection loss (RLmin)above−10 dB when thickness is from 2.0 mm to 4.0 mm.As shown in Fig. 6(c), whenxincreases to 1.1, theRLminreaches−34.43 dB at 955 MHz and the effective bandwidth is 120 MHz (880 MHz–1 GHz) for a thickness of 3.5 mm.The sample withx=1.2 exhibits extremely good EM wave absorption properties, as shown in Fig. 6(d). ItsRLmincan reach−30.42 dB at 617 MHz and the corresponding effective bandwidth reaches 563 MHz in the range of 437 MHz–1 GHz when the thickness is 3.5 mm. The sample withx=1.3 also shows excellent EM wave absorption properties. ItsRLminreaches−40.63 dB at 910 MHz and the effective bandwidth is 190 MHz (810 MHz–1 GHz) for a thickness of 4 mm, as shown in Fig. 6(e). For the sample withx= 1.4, the EM wave absorption properties are slightly decreased. TheRLminreaches−25.41 dB at 990 MHz and the effective bandwidth is 22 MHz(978 MHz–1 GHz)for a thickness of 3 mm,as shown in Fig.6(f).

It is well known that good impedance matching properties are the necessary condition for excellent EM wave absorption properties.[48,49]Figures 7(a)–7(f) show the relative input impedances (|Zin/Z0|) versus frequency of Ba(CoTi)xFe12−2xO19@BiFeO3with a thickness of 2–4 mm.It can be clearly seen that the value of|Zin/Z0| increases with frequency for samples withx=0.9 and 1.0,but is much smaller than 1.0 in the megahertz band. Therefore, their EM wave absorption properties are poor due to the impedance mismatch and the low permeability. For samples withx=1.1,1.2, 1.3 and 1.4, the impedance matching properties are improved due to increased permeability,the|Zin/Z0|value firstly increases and then decreases with frequency,and approach 1.0 at relatively high frequency bands(400 MHz–1 GHz), which means that these samples may obtain better microwave absorption properties in high frequency bands.

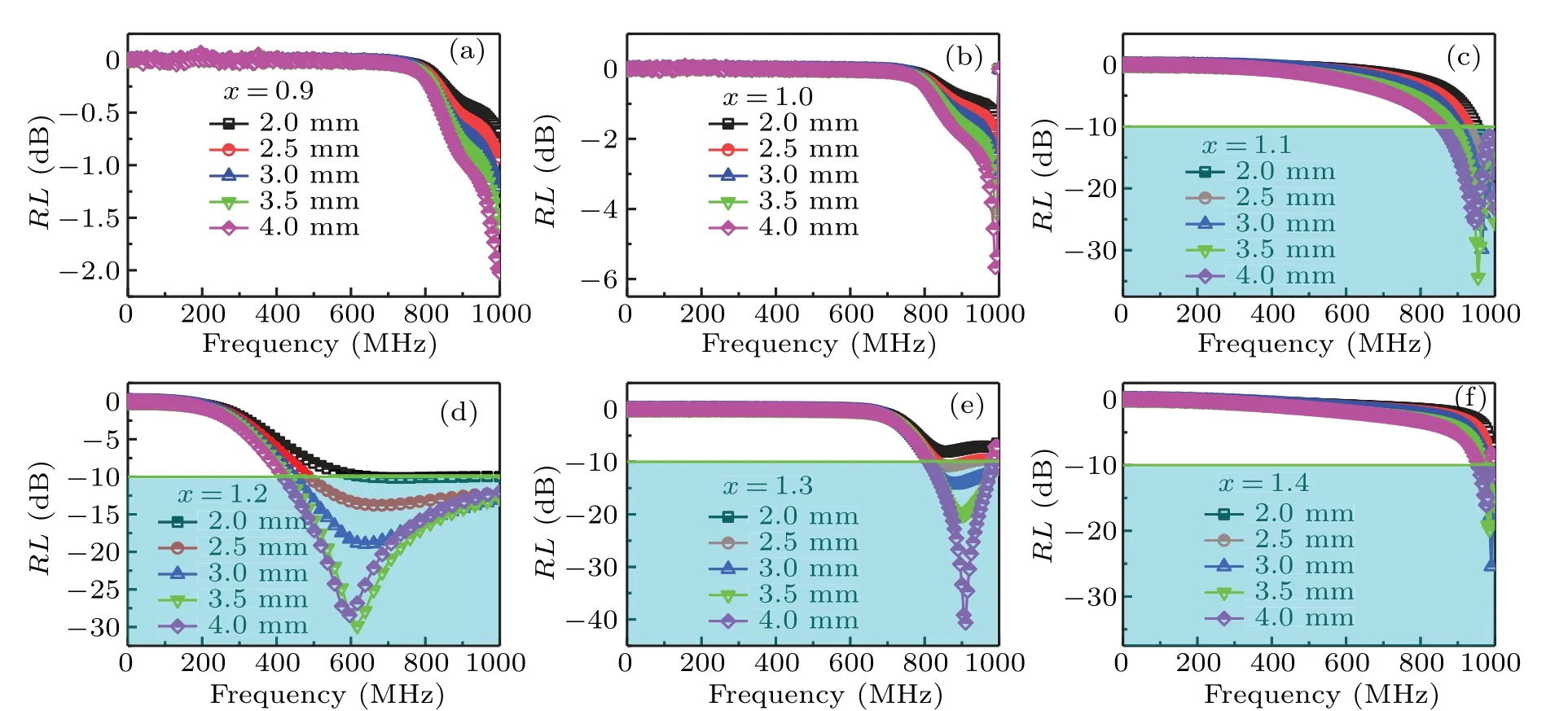
Fig.6. Thickness-dependent RL–f curves of Ba(CoTi)xFe12−2xO19@BiFeO3 absorbers; (a)x=0.9,(b)x=1.0,(c)x=1.1,(d)x=1.2,(e)x=1.3,and(f)x=1.4.

Fig.7. The dependence of the relative input impedance|Zin/Z0|on the frequency for Ba(CoTi)xFe12−2xO19@BiFeO3;(a)x=0.9,(b)x=1.0,(c)x=1.1,(d)x=1.2,(e)x=1.3,(f)x=1.4.

3.6. EM wave absorption mechanism

It can be seen from Fig.6(d)that theRLpeak frequency of the sample withx=1.2 moves from 661 MHz to 596 MHz and the intensity firstly increases then decreases with the absorber thickness increases from 2.5 mm to 4.0 mm.This phenomenon can be explained by the interface cancelation model.[50,51]According to the interface cancelation model

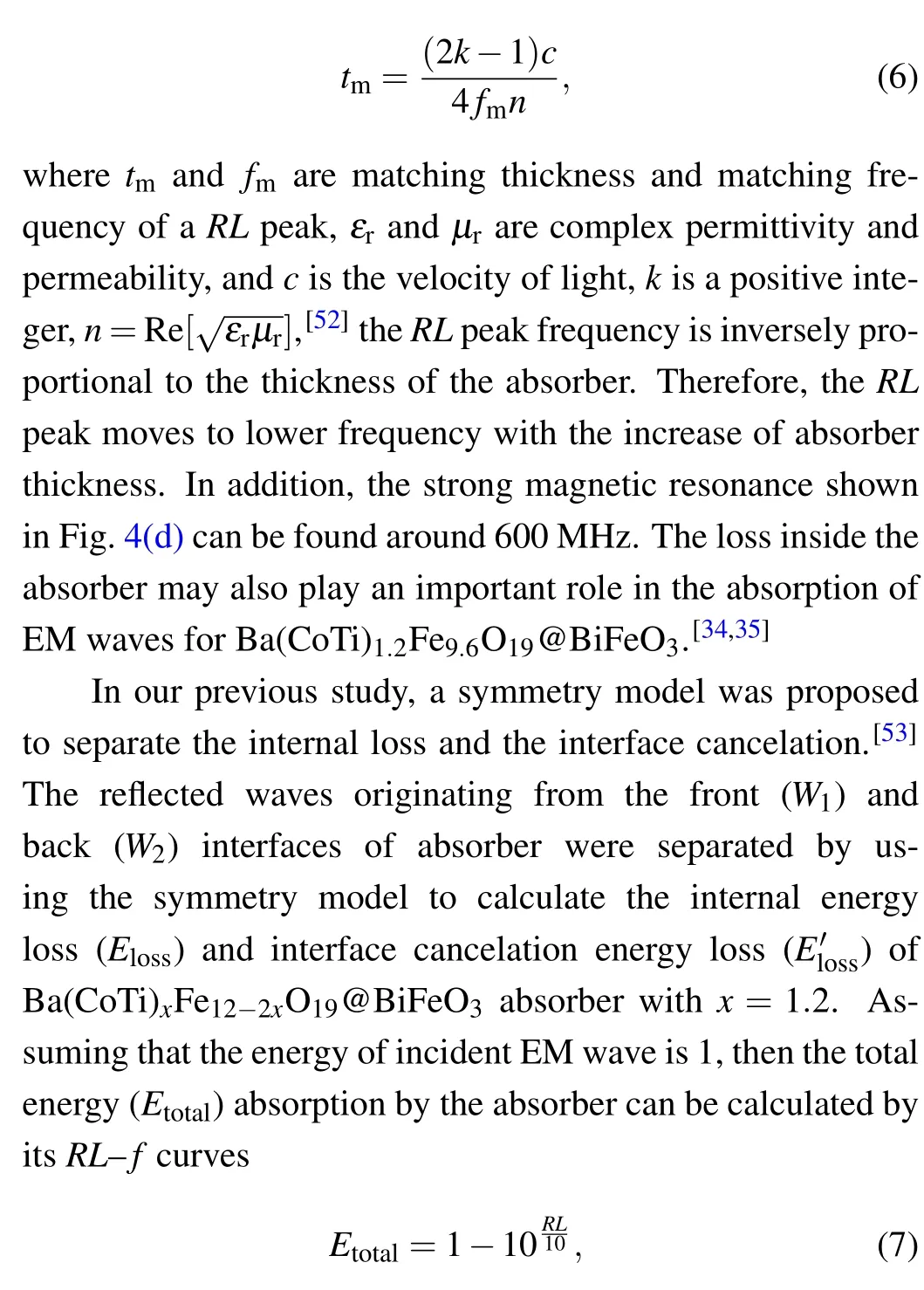
the total energy absorption by the absorber includes the part of interface cancelation and the part of internal loss

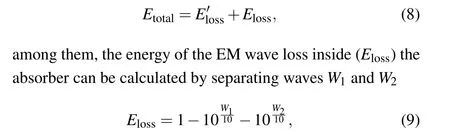
the calculation results are shown in Figs.8(a)–8(d),where the blue solid lines represent the total energy(Etotal)absorbed by the absorbers, the red solid lines represent the attenuated energy(Eloss)of the EM waves inside the absorber,and the solid green lines represent the cancelation energy(E′loss)of reflected waves at the front and back interfaces, and the symbol lines represent theRL–fcurves. It can be seen that the red solid lines are much higher than the green solid lines in the effective absorption frequency band (RL ≤−10 dB). This means that the absorption of EM waves by the absorber mainly originates from the high loss inside the absorber. The internal loss mainly comes from the magnetic loss caused by the natural resonance.[54]As shown in Fig. 8(a), when the thickness of the absorber is 2.5 mm,Elossaccounts for 56%–77% of the incident wave energy, the bandwidth is 514 MHz. TheElossincreases with the increasing thickness of the absorber. When the thickness of the absorber reaches 4 mm,Elossaccounts for 63%–85%of the incident wave energy,and the bandwidth reaches 585 MHz. This indicates that high internal loss can increase the bandwidth of the absorber.

For absorber with a thickness of 3.5 mm,Elossincreases with frequency,and the maximum value at 1 GHz is about 83%(−7.70 dB)of the energy of the incident wave,which does not match with the frequency and intensity of theRLpeak. It indicates that strong absorption peaks cannot be achieved by only internal loss.E′lossfirstly increases and then decreases with frequency,the maximum value ofE′lossmoves to lower frequency with the increase of absorber thickness. TheRL–fcurves exhibit the same trend with the variation of frequency and thickness. This further indicates that theRLpeaks are attributed to interface cancelation. The interface cancelation enhances the absorption properties and generates sharp absorption peaks(−30.42 dB).From above discussions,it can be concluded that the excellent wave absorption properties are based on the combination of high loss inside the absorber and the interface cancelation. The frequency and intensity ofRLpeak are mainly determined by the interface cancelation, and the internal loss plays a crucial role in expanding the bandwidth.

Fig. 8. The total energy loss (blue lines), internal energy loss (red lines), interface cancelation energy loss (green lines) and RL–f curves(symbol lines)of the absorber with various thickness(a)2.5 mm,(b)3.0 mm,(c)3.5 mm,(d)4.0 mm.

4. Conclusion

Herein,the Ba(CoTi)xFe12−2xO19@BiFeO3EM wave absorption material with different Co2+−Ti4+content is successfully prepared, and its low−frequency EM wave absorption properties are investigated. The experimental results show that Ba(CoTi)xFe12−2xO19@BiFeO3exhibits excellent wave absorption properties at different Co2+–Ti4+content.The best comprehensive property is obtained forx= 1.2,in which case, the absorber has the highest permeability,internal loss and the best impedance matching properties,

where theRLminand effective bandwidth are−30.43 dB,617 MHz,respectively.The investigation on absorption mechanism shows that the strong EM wave absorption properties of Ba(CoTi)xFe12−2xO19@BiFeO3are mainly originated from the internal loss caused by natural resonance,and the interface cancelation of the reflected waves from the front and back interfaces further improves the absorption intensity and generates aRLpeak.

Acknowledgements

Project supported by the National Natural Science Foundation of China (Grant No. 11574122) and the Joint Fund of Equipment Pre-Research and Ministry of Education, China(Grant No.6141A02033242).