The existence and blow-up of the radial solutions of a (k1， k2)-Hessian system involving a nonlinear operator and gradient

Guotao WANG(王国涛)Zedong YANG(杨泽栋)

School of Mathematics and Computer Science,Shanai Normal University,Taiyuan 030031，China E-mail: wgt2512@163.com; yangzd1229@163.com.

JiafaXU (徐家发)*

School of Mathematical Sciences，Chongqing Normal University，Chongqing 401331，China E-mail: rujiafa.292@sina.com

Lihong ZHANG(张丽红)

School of Mathematics and Computer Science，Shanai Normal University，Taigyuan 030031，China E-mail: zhanglih149@126.com

1 Introduction

The aim of this paper is to show the positive radial solutions of the following(k1，k2)-Hessian system involving a nonlinear operator and gradient:

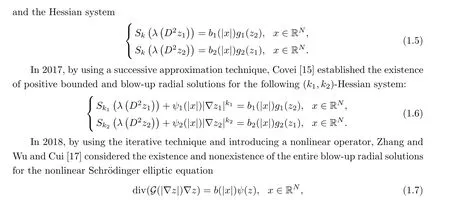
where N ≥2， G is a nonlinear operator on Λ={G ∈C2([0，+∞)，(0，+∞))|， and there exists a constant α ＞0 such that for all 0 ＜l ＜1，G(ls)≤lαG(s)}.

Inspired by the above works， by using the monotone iterative method， we investigate the existence of entire positive bounded and blow-up radial solutions of the nonlinear (k1，k2)-Hessian system (1.1) involving a nonlinear operator. Our results complement the works of many authors([1–15，18–24])，and are also closely related to some recent works by the iterative method ([25–29]) for various differential equations.

2 Main Results

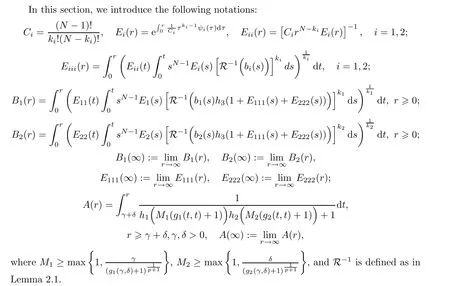
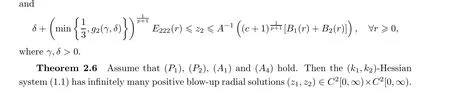
3 Proofs of the Main Results

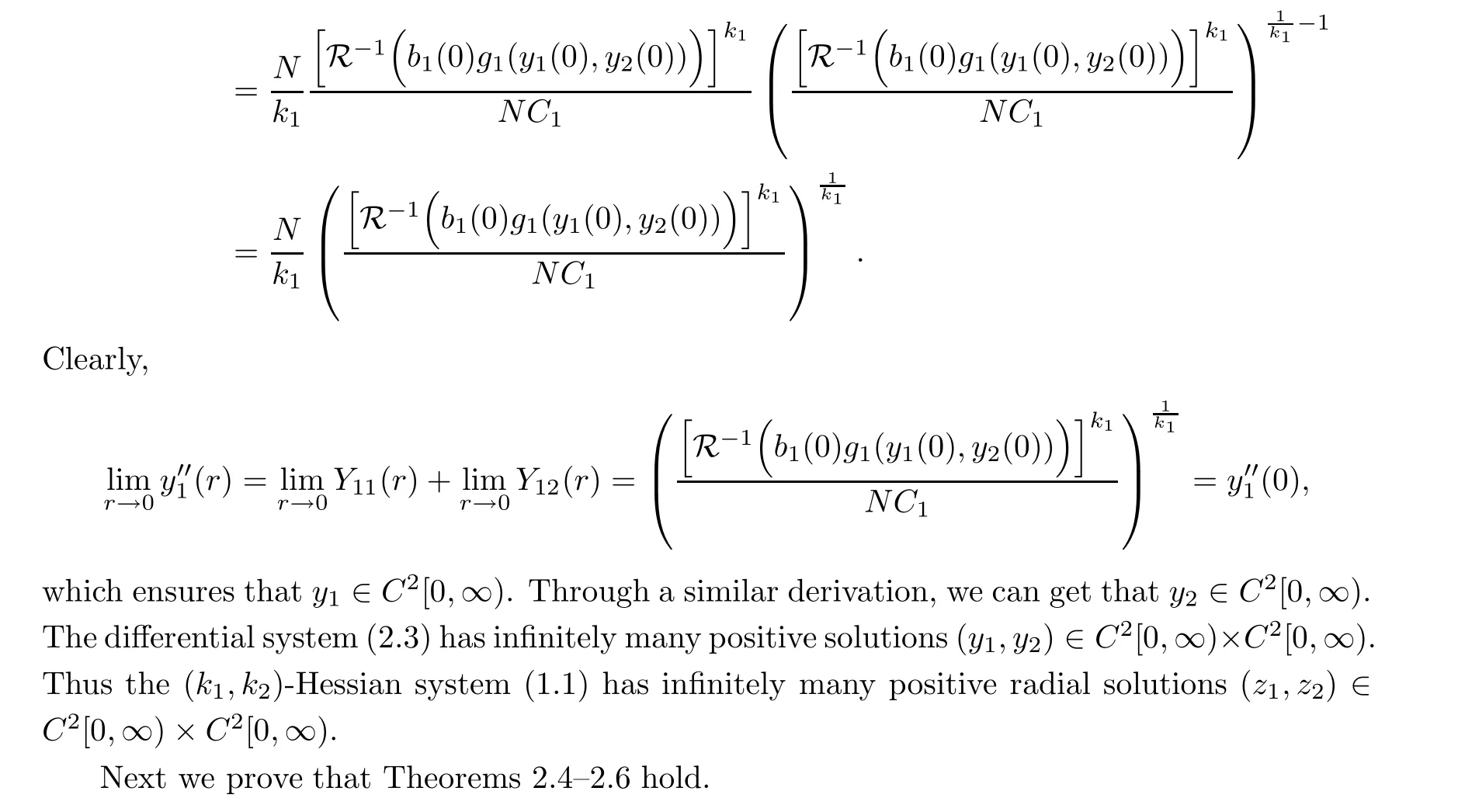
3.1 Proof of Theorem 2.4

3.2 Proof of Theorem 2.5

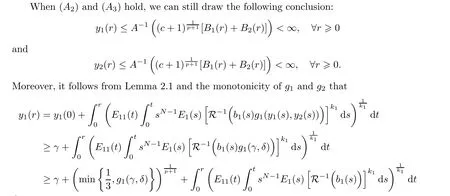
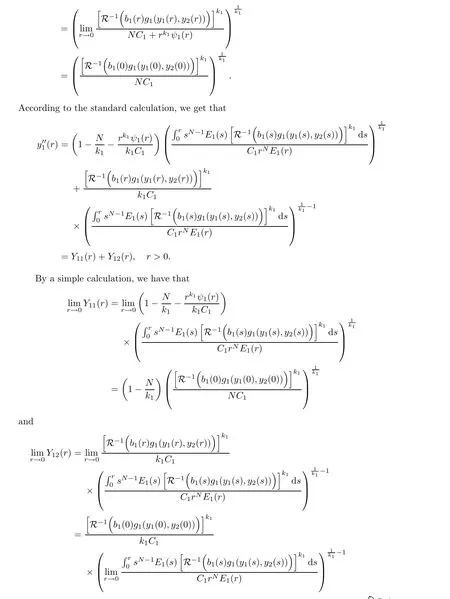
3.3 Proof of Theorem 2.6