Nonlinear dynamical wave structures of Zoomeron equation for population models

Ahmet Bekir and Emad H M Zahran

1Neighbourhood of Akcaglan,Imarli Street,Number: 28/4,26030,Eskisehir,Turkey

2Departments of Mathematical and Physical Engineering,Benha University,Faculty of Engineering,Shubra,Egypt

Keywords: (2+1)-dimensional non-fractional Zoomeron equation, time-fractional biological population model,Paul–Painlev´e approach method,traveling wave solutions

1. Introduction

The biological population process is one of the most important principal pillars on which the population regulation process depends. The principal axes that determine this scheme and play a vital role in the population regulation process are position,time,and density.Two important mathematical models are established to study these population processes,namely (i) the (2+1)-dimensional non-fractional Zoomeron model (NFZM) mentioned in Refs. [1,2], which is a forward stretch for the well-known (1+1)-NFZM “extensively investigated in the literature” that describes special cases of solitons achieved in different branches of physics; (ii) the timefractional biological population model(TFBPM)mentioned in Ref.[3]which is the other well-known model that represents the population processes. Particularly,Jabbariet al.[4]gave a good model to the TFBPM for animals which is a special case of the(2+1)-NFZM.

Many scientists used several methods to study the nonlinear partial differential equations. Most of these methods are included in Refs. [5–21]. In the literature, recent studies have been introduced to discuss these suggested equations.Especially Abazari[22]obtained the periodic and soliton solutions to Zoomeron equation by means of (G′/G)-expansion method, Khanet al.[23]used the (G′/G)-expansion method to find the traveling wave solutions to the (2+1)-dimensional Zoomeron equation. In the relevant subject, there exist other modern articles about solving nonlinear evolution equations that describe the blood flow in arterial vessel that will make revolution in developing the human health and animals. For example Biet al.[24]derived a new model that describes the envelope solitary wave’s propagation of the pulsating flow in the blood and discussed and analyzed the influencing factors of stroke volume which provide a strong theoretical basis for some blood diseases and Wenhuiet al.[25]deduced the(2+1)-dimensional mZK by using multi-scale analysis and reduced perturbations method from the dimensionless equation of (2+1)-dimensional cold dust plasma to describe the propagation law of dust acoustic solitary wave and obtained new model that has high nonlinearity,which can describe the solitary wave on the surface by using the fractional variational method and semi-inverse method.

This work is mainly to realize new impressive regulations for the biological population models through obtaining the exact solution to these two models (which contain some variables) by using the PPAM.[26–28]When these variables take specific values,we will achieve the traveling wave solutions.

2. Technique description of PPAM

Now we first propose the general form of the nonlinear evolution equation We introduceRas a function ofφ(x,t),and its partial derivative is expressed as

According to PPAM[24]the exact solution to the nonlinear ordinary differential equation can be written in the following

3. Exact solution to(2+1)-NFZM equation

In this section,we will use the PPAM as a new technique to realize the exact solution to the(2+1)-NFZM[1]“in terms of some variables”,we can easily obtain the traveling wave solutions when these variables take specific values. According to Refs.[1,2],the(2+1)-NFZM equation can be written as Thus the solution is

In the same manner we can design the other three solutions that are identical to the remaining three results.

4. Some knots about fractional calculus

Before we apply the PPAM mentioned above to the TFBPM,[3]we first give some notes about the fractional calculus.[27]

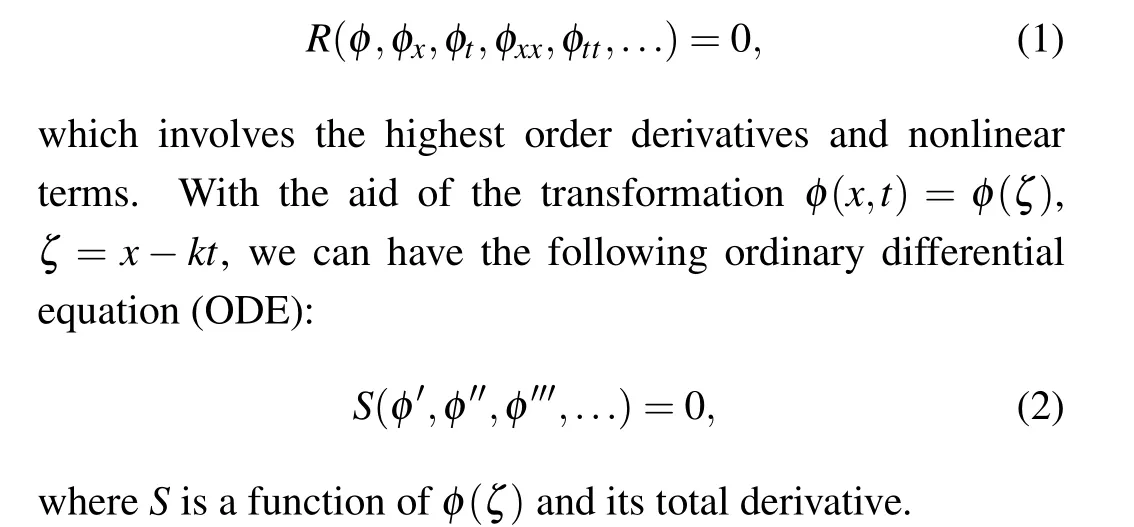
4.1. Caputo’s fractional derivative[28–36]

Although the fractional calculus is a powerful tool to describe the physical phenomena system which has long-term memory and long-range spatial interaction, there exists the distortion between fractional mild solution and strong solution. This confusion is due to the fact that the fractional differential equation has a non-differentiable solution, and this makes solution process difficult, but an approximate continuous solution can be realized if we observe the discontinuous solution.In this section we build preliminary notes and remind some distinctive relations to Riemann–Liouville derivatives.

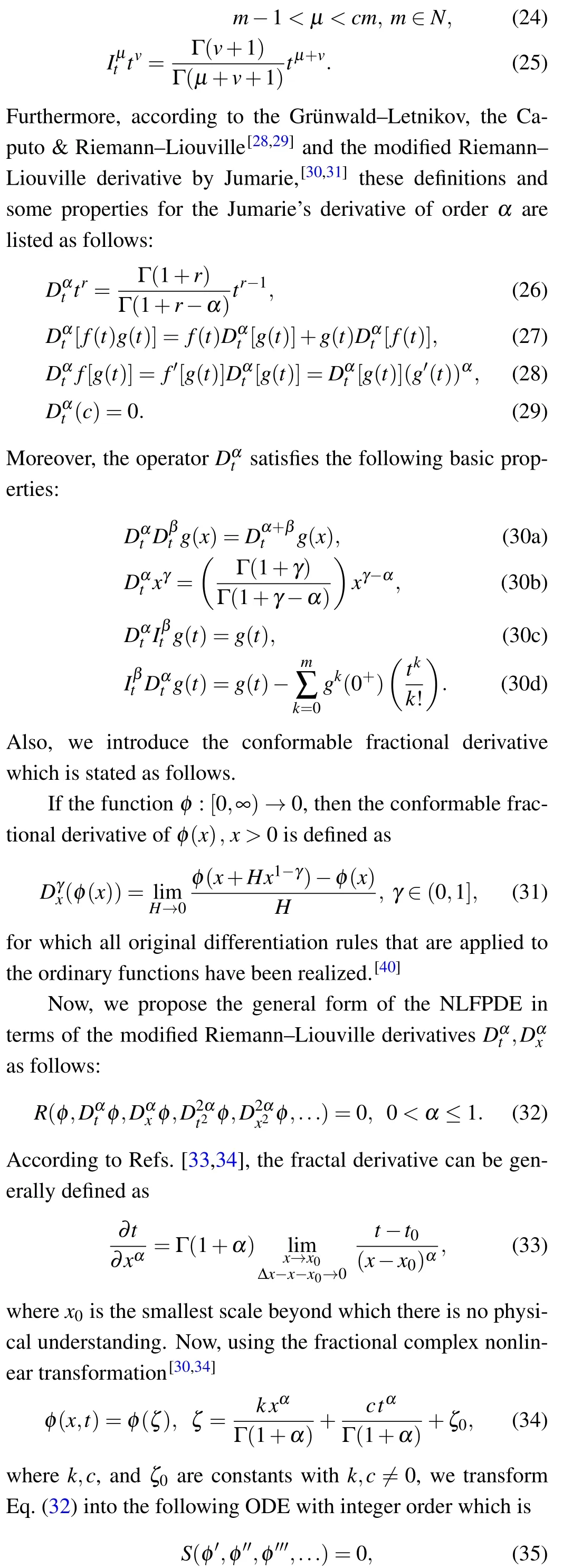
4.2. Exact solution to time-fraction order of biological population model

We apply the constructed approach to the time-fraction order of the biological population model (TFBPM) and consider this complex transformation

where

Remark 1 Many other solutions can be achieved according two different values ofα,λ,andC.

5. Results and discussion

(i) From the point of view of the PPAM, we establish new periodic biological population distribution solutions of the (2+1)-dimensional Zoomeron model. The other achieved solutions for this model indicate that the biological population distribution is symmetric with respect to the axis. These new behaviors are documented via Figs. 1–3. The achieved solutions which were not realized before are new compared with those in Refs. [22,23] where is used the (G′/G)-expansion method that is obtained in Ref. [38] where the exp-function method is used.Furthermore,the simulation of the solitary solutions is achieved as shown in Ref.[39]for the bounded traveling wave solution of this model using the bifurcation method of dynamical system and numerical simulation method. Also some of the obtained solutions are in agreement with the solutions that are achieved in Ref.[40]in some cases but some are in disagreement in other cases. Moreover, the achieved solutions present the periodic behavior in some cases and the isomorphic distribution in other cases for the biological population distribution process.

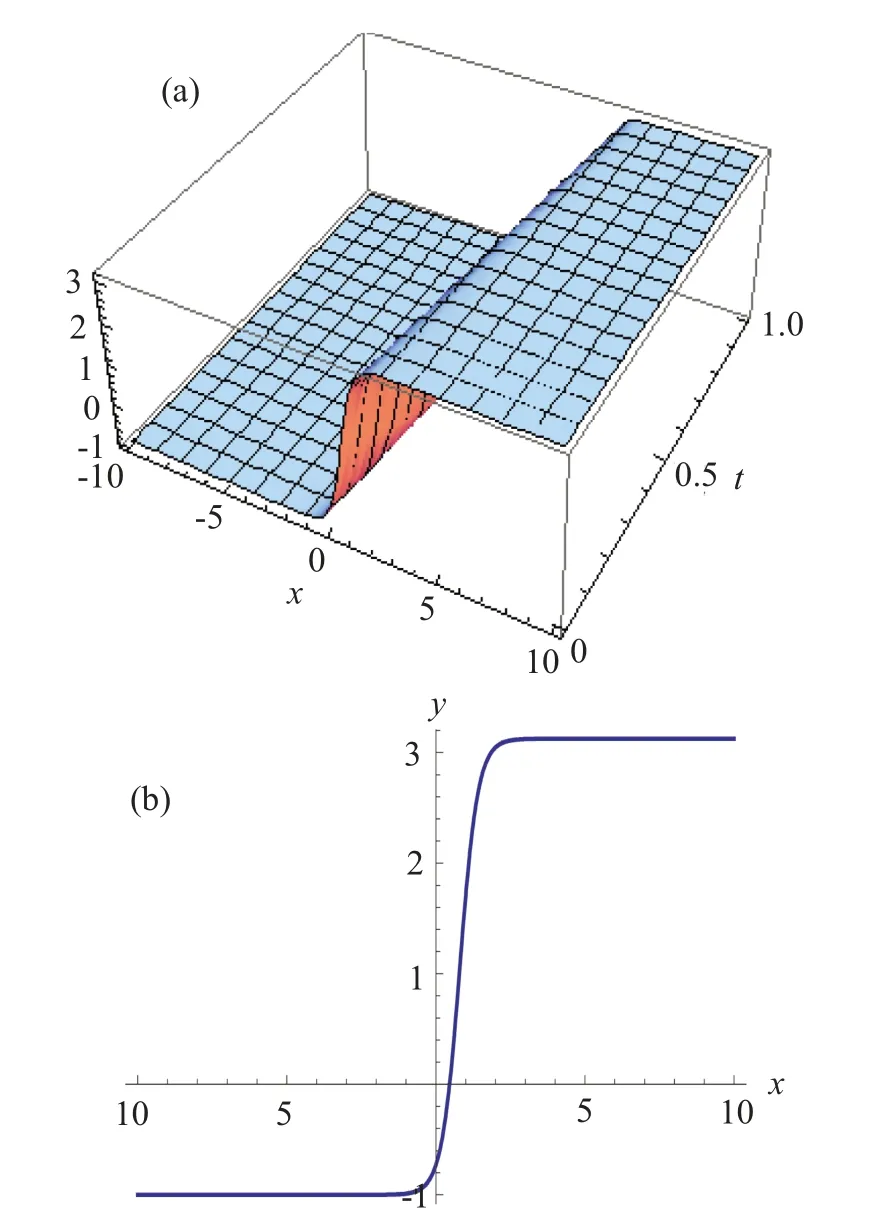
Fig.1. Plot of 2D and 3D Eq.(16)with values: A0=-1,A1=1,A=0.8,N=-3.3,C1=X0=1,k=2,k1=4,t=0.1 for two dimensions.

Fig.2.Plot of real part of 2D and 3D Eq.(19)with values:A0=-i,A1=1,A=0.8,N=-1.6i,C1=X0=1,k=2,k1=4,t=0.1 for two dimensions.

Fig.3. Plot of imaginary part of 2D and 3D Eq.(20)with values: A0=-i,A1 =1, A=0.8, N =-1.6i,C1 =X0 =1, k=2, k1 =4, t =0.1 for two dimensions.

(ii) On the other hand, the achieved solutions for the time fraction population equation in the framework of the suggested technique indicate that the biological population distribution process is regular and symmetric one(see Fig.4)which is new, very accurate compared with the obtained solutions in Refs. [36,37,41], where were used the Homotopy analysis Sumudu transformation method, the Homotopy perturbation method and Homotopy analysis respectivly.

It is clear that all achieved solutions for the fractionalorder biological population models and non-fractional-order biological population models are stable,i.e. for consecutive intervals of time the behaviors of solutions will not change.now, we come to discuss this point. For example for the first solution for two consecutive different intervals of time we find that the behaviors of the solutions in [0–1] are the same as those in Refs.[2,3].

Fig.4. Plot of 2D and 3D Eq.(45) with values: A0 =A1 =c=C=C1 =k=λ =X0=1,A=0.5,N=0.5,α =0.5,t=0.1 for two dimensions.

6. Conclusions

The PPAM is effectively used for the first time to construct new impressive regulations for the biological population models with its different forms, which are the nonfractional-order biological population(2+1)-Zoomeron model(NFBPZM)(see Figs.1–3)and the time-fractional-order biological population model(TFBPM)(see Fig.4). Some of the obtained results agree with the results previously obtained in Refs.[24,25]for the NFBPZM and in Refs.[37–39,41]for the TFBPM in some cases,but some disagree in other cases. The achieved results and corresponding solutions that depend on the fundamental parameters which are the position,time,and density indicate that the population regulation process is symmetric in some cases and periodic in other cases. The satisfying results of the new accurate regulation population process prove this technique to be efficient.