The influence of charge characteristics of suspension droplets on the ion flow field in different temperatures and humidity

Nanxuan SHEN(申南轩),Zihan SU(苏子寒),Yuanhang ZHANG(张远航)and Tiebing LU (卢铁兵)

State Key Laboratory for Alternate Electrical Power System with Renewable Energy Sources,North China Electric Power University, Beijing 102206, People’s Republic of China

Abstract In order to clarify the charging characteristics of suspension droplets in ion flow field under different temperatures and humidity, the effective charging factor used to characterize the charging characteristics of suspension droplets is introduced in this paper, and a calculation method of charging factor is proposed based on the upstream finite element method (FEM).Then, the charging factor under different temperatures and humidity is calculated, and the analytic expression of the charging factor considering the influence of temperature and humidity is obtained by fitting the calculation results.The influence of suspension droplets on the ion flow field is analyzed.The results show that the charging factor is small and increases little with the relative humidity when the relative humidity is less than 60%, and the charging factor is large and increases rapidly with the relative humidity when the relative humidity is more than 60%.At the same relative humidity, the charging factor increases linearly with the temperature.The influence of charged suspension droplets on the ion flow field can be ignored when the relative humidity is less than 60% and must be considered under high temperature and humidity.The calculation method and analytic expression of the charging factor proposed in this paper can be used to model of ion flow field considering the influence of temperature and humidity and provide technical support for the construction of HVDC transmission lines across high temperature and humidity.

Keywords: suspension droplets, charging characteristics, humidity, temperature, ion flow field

1.Introduction

In order to transfer power energy in large capacity and longdistance, several HVDC lines have been completed.Corona discharge occurs during the operation of the HVDC lines,which leads to a problem of ion flow field [1, 2].The surrounding environment of the HVDC line is complex, and the temperature and humidity will affect the ion flow field of HVDC lines [3].Especially in high humidity, suspension droplets will appear in the space and capture the space charge generated by corona discharge.Then the charged suspension droplets will be formed.Charged suspension droplets will cause distortion of the surrounding electric field and complicate the ion flow field.The parameters such as radius and number density of the suspension droplets in space cannot be measured accurately, which makes the charge of suspension droplets difficult to calculate in the ion flow field.Furthermore, the effect of temperature and humidity on the ion flow field is not clear.

Some scholars have studied the charging characteristics of suspension particles in the ion flow field.Dastoori et al used the Faraday cup to measure the charge of dust particles on the bottom of PCB (printed circuit board)[4].The charge of particles in a fluidized bed was measured by Song D with a Faraday tube, and the effect of charged particles on the fluidized bed was analyzed [5].Xu et al measured the concentration and size distribution of particles in flue gas using ELPI (electrical low-pressure impactor), and the average charge of a single-particle was calculated [6].

In the aspect of charge of small particles,Hewitt used the coaxial cylinder mobility analyzer to measure the charge of particles with a diameter of 0.07-0.66 μm.The measured charge was consistent with the charging model considering field charging and diffusion charging, and the correctness of the charging model was verified [7].Luo et al measured the average charge of a single particle under different voltages in DC electric field by using ELPI.The results show that the charging modes of particles with diameters smaller and larger than 0.2 μm are diffusion charging and field charging,respectively [8].The above literature mainly studied the charging characteristics and models of solid particles but did not involve the charging characteristics of suspension droplets in space.

As for the treatment of suspension droplets in the ion flow field,Ma et al measured the ion flow field of HVDC wire under different temperatures and humidity, and the variation of corona voltage with temperature and humidity is considered to be the main reason for the change of ion flow field,so the influence of suspension droplets was not considered[9].Li et al considered that the effect of charged suspension droplets on ion flow field should be considered under any humidity condition, and charged suspension droplets were randomly distributed in space [10].Up to now, the charging characteristics of suspension droplets in the ion flow field under different temperatures and humidity are not clear.It is necessary to study the characterization method of the charging characteristics of suspension droplets in the ion flow field to provide the theoretical basis for calculating the ion flow field of HVDC lines under different temperatures and humidity.

In this paper, firstly, the effective charging factor to characterize the charging characteristics of suspension droplets is introduced.Secondly, the charging factor of suspension droplets under different temperatures and humidity is calculated based on the measurement results of the ion flow field, and the analytic expression of the charging factor considering the influence of temperature and humidity is obtained.Finally, the influence of the charged suspension droplets in the ion flow field is analyzed.The analytic expression can be used to calculate the ion flow field under different temperatures and humidity, which provides a theoretical basis for the construction of HVDC lines.

2.Definition and solution of charging factor

2.1.Definition of charging factor

The charging characteristics of suspension droplets are determined by radius, electrical permittivity, and number density.It is difficult to quantitatively analyze the charging characteristics of suspension droplets because the above parameters are difficult to be measured accurately.In order to calculate the space charge density of suspension droplets,the charging factor is defined.Because the field charging model can be used to calculate the charge of the suspension droplets[10], the expression of effective charging factor is:

where E is the electric field intensity, and ρwis the space charge density of charged suspension droplets.

When the charging factor is determined, the calculation model of the ion flow field considering the influence of suspension droplets is as follows:

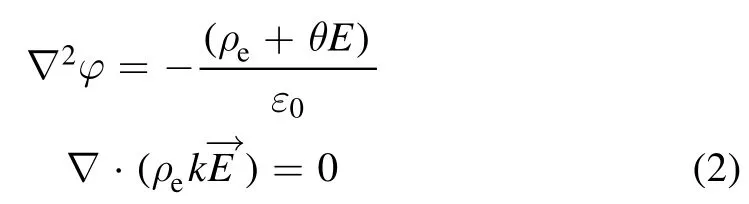
where φ is the electric potential,is the electric field vector with the magnitude E,ρeis the space charge density of ion,k is ion mobility, and ε0is the electrical permittivity of the air.

The boundary condition of equation(2)is that the electric potential on the lines and grounding point are operating voltage and 0, respectively.

According to equation (2), when the charging factor is determined, the ion flow field under the current temperature and humidity can be calculated to realize the prediction of the ion flow field.

2.2.Calculation method of charging factor

The analysis is based on the following assumptions in this work:

(1)The ion mobility and corona inception voltage of the conductor are fixed at a certain temperature, humidity,and air pressure.

(2)It can be considered that the suspension droplets are in a static state in the ion flow field [11].

(3)The electric field on the surfaces of the high voltage conductors is the corona inception electric field after the inception of corona discharge.

(4)The suspension droplets are uniformly distributed in space, and their shape is spherical.

It can be seen from equation(2)that the calculated value of electric field Ecis a function of charging factor θ when the ion mobility,corona inception voltage,and electrode structure are determined.

The error g(Ec)is defined:

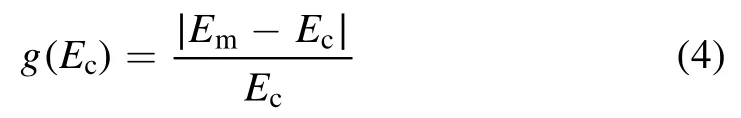
where Emis the measured value of an electric field.

When the error g(Ec)is less than a certain limit, the charging factor can be considered as the charging factor in the current environment.There is no analytical solution for equation (2)because of the introduction of charged suspension droplets.Therefore, the upstream finite element method (FEM)is used to solve equation (2).

According to the above analysis, the calculation process of the charging factor of suspension droplets is as follows:

Step 1.Give the initial values of the charging factor.

Step 2.Give the initial values of charge density.

Step 3.Calculate the space charge density of the suspension droplets and the total space charge density.

Step 4.Calculate the electric field using FEM to solve the Poisson equation.

Step 5.Calculate the space charge density by using the upstream element method to solve the current continuity equation.

Step 6.If the error limiting conditions of space charge density and the electric field in equations (5)and (6)are satisfied,g(Ec)is calculated.Otherwise, the surface charge density value is updated and steps 2-5 are repeated until the error limit condition is satisfied.The error limiting conditions are as follows:

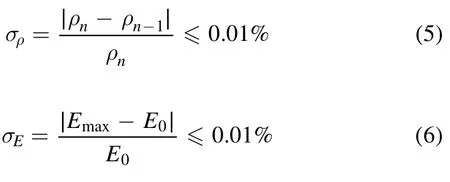
where σρand σEare the space charge density error and the electric field error on the conductor surface, respectively, ρnand ρn−1are the surface charge density of the conductor of the nth and (n−1)th iteration, respectively, Emaxand E0are the maximum electric field and corona inception electric field on the conductor surface, respectively.

Step 7.If g(Ec)≤0.01%,the calculation result is considered to be the charging factor of the suspension droplets in the current environment.Otherwise,update the charging factor value and repeat steps 2-6 until g(Ec)≤0.01%.

In order to consider the influence of the suspension droplets in the calculation, the error limit of g(Ec)should be less than the proportion of the electric field component generated by the charged suspension droplets at the cage surface.Therefore, the error limit of g(Ec)in this paper is 0.01%,which can not only ensure the accuracy of the calculation results,but also ensure the calculation speed and convergence of the calculation.

The calculation flow shows that in order to solve the charging factor, the ion flow field needs to be measured, the corona voltage and ion mobility under different temperatures and humidity are determined.

Figure 1.The experimental platform of the coaxial cylindrical electrode.

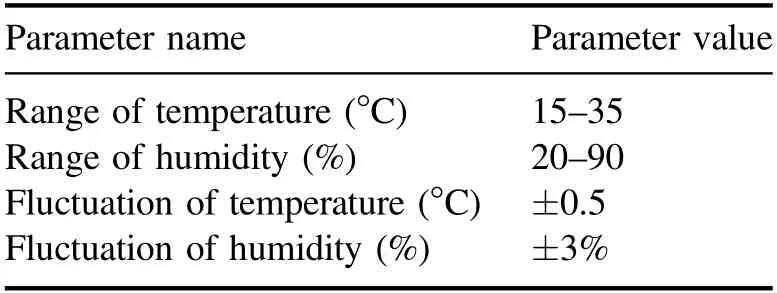
Table 1.Parameters of the artificial climate chamber.

3.Charging factor under different temperatures and humidity

3.1.Measurement of the ion flow field

The artificial climate chamber is used to adjust the temperature and humidity.High-precision temperature and humidity sensors are used to measure the temperature and humidity in the climate chamber.The parameters of the artificial climate chamber are shown in table 1.

The coaxial cylindrical electrode structure is used to generate the corona discharge, as shown in figure 1.The central electrode is a bare wire with a length of 2.8 m and a radius of 1.1 mm.The grounded corona cage is composed of three parts that are electrically insulated from each other.The measuring section is used to measure the ion flow field of HVDC wire, and the shielding section is used to weaken the end-effect.The length of the measuring and shielding sections is 2 m and 0.3 m, respectively, and their diameter is 0.8 m.

The experimental platform was placed in the artificial climate chamber.Under the conditions of temperature T = 30

°C,25°C,and 20°C,the relative humidity RH was adjusted in the range of 30%-90%.It is difficult to clearly reflect the dual impact of temperature and humidity on the measurement and calculation result using the absolute humidity related to both temperature and humidity, so the relative humidity is used in this work.Then,the positive voltage of 0-65 kV was applied to the central electrode by the DC voltage source.The field mill was used to measure the electric field strength, and the ion current plate was used to measure the ion current density.

Figure 2.Measurement of ion flow field under different temperatures and humidity.(a)The total electric field, (b)the ion current density.

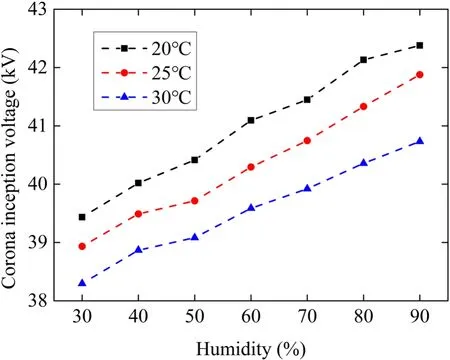
Figure 3.Corona inception voltage of conductor under different temperatures and humidity.

Figure 4.The calculations of positive ion mobility under different temperatures and humidity.

Figure 5.The value of λ is obtained through continuous calculation.

When the applied voltage on the conductor is 50 kV and the corona discharge occurs,the measurement of the ion flow field is shown in figure 2 under different temperatures and humidity.The measurement shows that the total electric field and ion current density decrease with the relative humidity and increase with the temperature.

3.2.Determination of corona inception voltage and ion mobility

Many studies have shown that temperature and humidity have a great influence on corona inception voltage.The influence of temperature on corona inception voltage was consistent,and the corona voltage of the conductor decreases with the increase of temperature[12].The influence of humidity on the corona discharge characteristics of wire has not been consistent[13-16].It is necessary to analyze the corona discharge characteristics of wires under different humidity according to the wire structure.

The corresponding voltage at the inflection point of the relation curve between ion current density and conductor voltage is the corona inception voltage of the conductor[17].

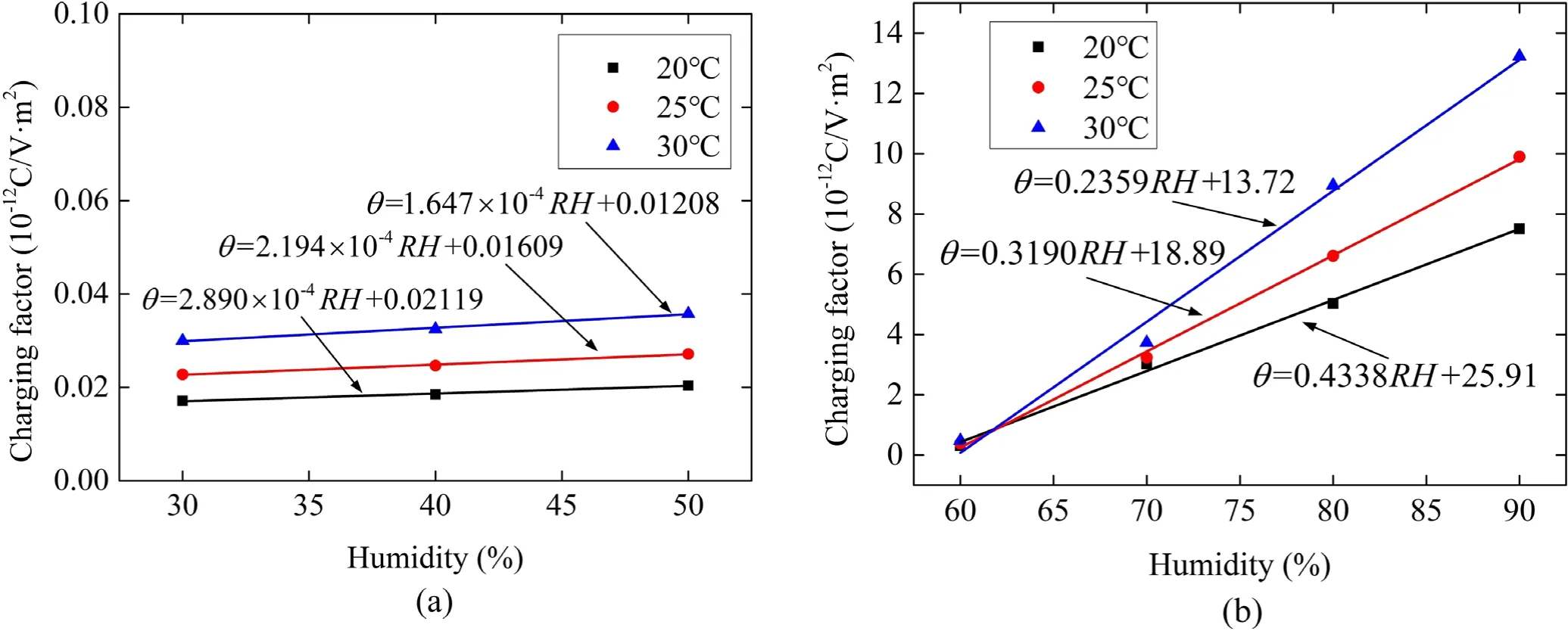
Figure 6.Calculation results and analytic expression of charging factor.(a)The relative humidity is less than 60%,(b)the relative humidity is more than 60%.

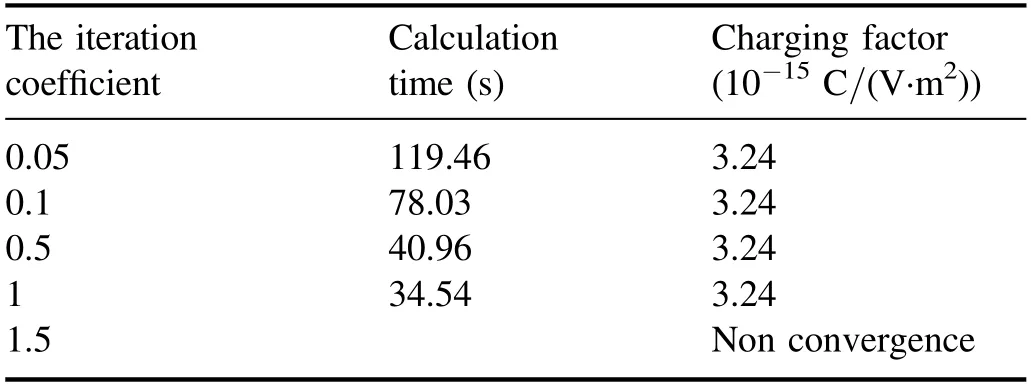
Table 2.The calculation time and charging factor under different iteration coefficients.

The curve of corona inception voltage with temperature and humidity is shown in figure 3.The corona inception voltage increases with the increase of relative humidity and decreases with the increase of temperature.

Some scholars have studied ion mobility under different temperatures and humidity, the calculation models of ion mobility were established.In this work,the calculation model of ion mobility proposed by Zhang Bo et al was used [18].The calculations of positive ion mobility under different temperatures and humidity are shown in figure 4.From figure 4,the positive ion mobility decreases with the increase of relative humidity and temperature.

3.3.Initial value and iterative process of charging factor

In order to ensure the convergence and calculation speed of the calculation results of the charging factor,it is necessary to select a reasonable initial value of the charging factor.The initial value of the charging factor is:

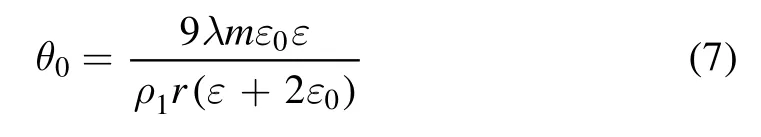
where λ is the correction factor,ρ1is the mass density of water,m is water content in the air, and the value of r is 0.2 μm.

In equation (7), the value range of λ is [0, 1)(When the relative humidity is 0%,λ is 0).The value of λ represents the ratio of the actual charging factor in the space to the possible maximum charging factor.The value of λ obtained through a large number of calculations is shown in figure 5.

From figure 5, it can be seen that the value of λ is small when the relative humidity is less than 60%, and increases with the relative humidity when the relative humidity is more than 60%.In this work,the average is taken as the value of λ.The value of λ is:

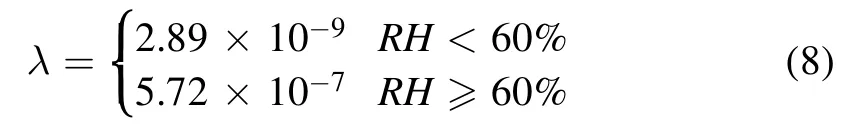
When suspension droplets exist in space, charged suspension droplets will strengthen the ground electric field[10],so the iterative formula of charging factor used in this work is:

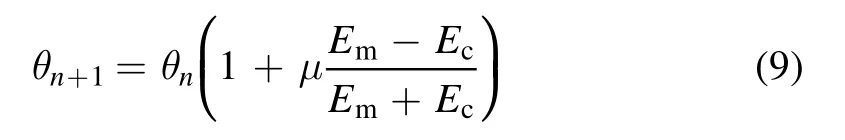
where θn+1and θnare the charging factors of the (n + 1)th and nth iteration, respectively;μ is the iteration coefficient in this paper, and its value is 1.

When T = 25°C and RH = 70%,the charging factor of suspension droplets is calculated under different iteration coefficients.The calculation time and charging factor are shown in table 2.

As shown in table 2,when the iteration coefficient is less than 1, the calculation time is significantly increased, but the calculation accuracy is not significantly improved.When the iteration coefficient is more than 1, the calculation may not converge.Using the value of μ given in this paper can ensure the calculation speed and accuracy.

3.4.Analytic expression of charging factor

A linear function is used to fit the calculated results of the charging factor, and the analytic expression of the fitting curve is shown in figure 6.

The calculated results show that the charging factor is small and increases little with the relative humidity when the relative humidity is less than 60%.When the temperature is 30 °C, the charging factor at RH = 50% is 1.1 times higher than that at RH = 40%.When the relative humidity is more than 60%,the charging factor is large and increases obviously with the increase of relative humidity.When the temperature is 30°C,the charging factor at RH = 70%is 7.8 times higher than that at RH = 60%.

The charging factor increases linearly with the increase of temperature.When the relative humidity is 50%, the charging factor at T = 30°C is 1.32 times higher than that at T = 25°C,and the charging factor at T = 25°C is 1.33 times higher than that at T = 20°C.The increase of saturated water content in space is the main reason for the increase of charging factor with temperature.

The intersection points of analytic expression are calculated.When T = 30 °C, 25°C and 20 °C, the abscissa of intersection points is about 59%.The results show that when the relative humidity is more than 60%,there will be charged suspension droplets in the ion flow field.

The slope and intercept of analytic expression change with temperature.A linear function is used to fit the slope and intercept, and the fitting curve is shown in figure 7.The curve-fitting results are:

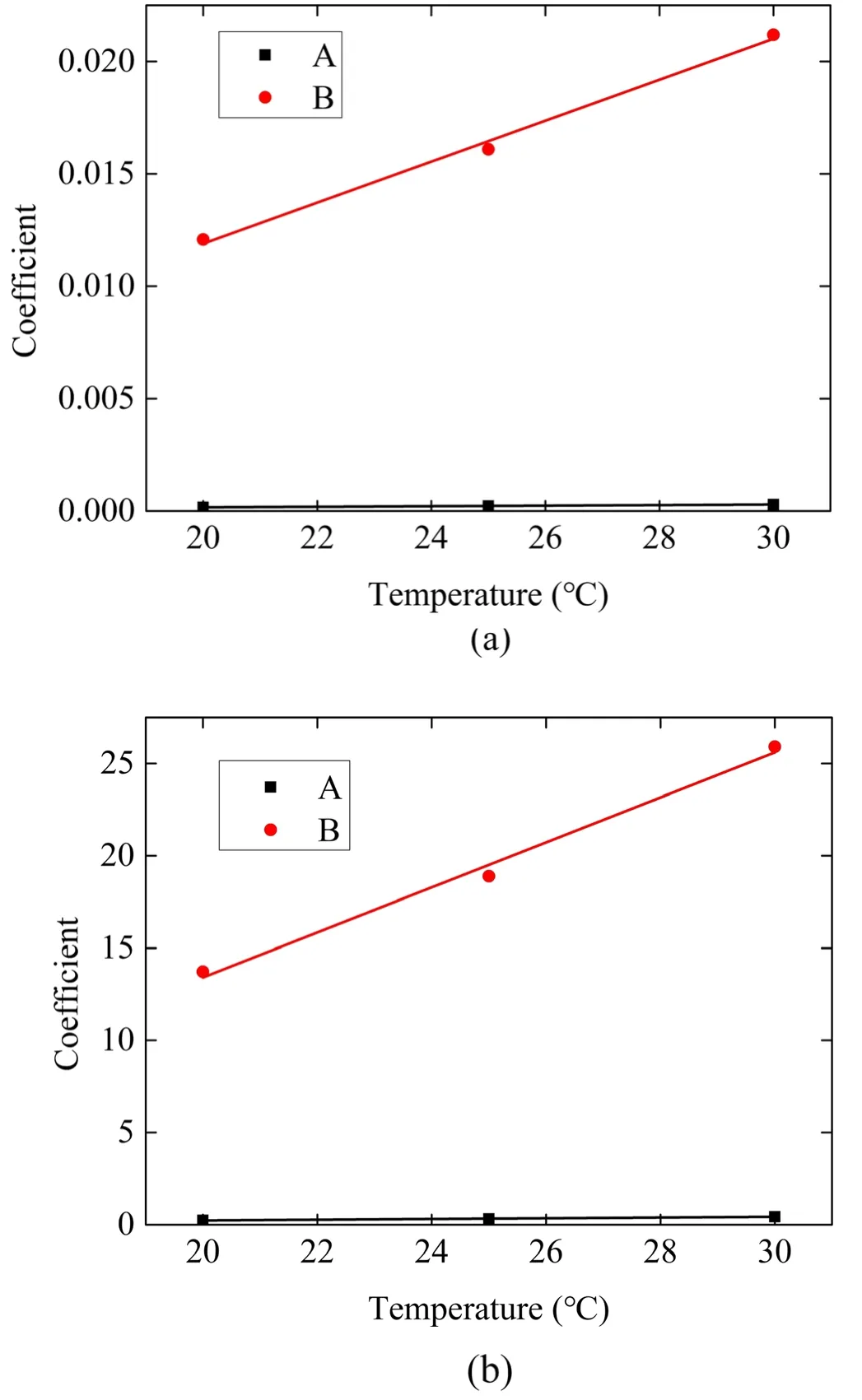
Figure 7.Slope and intercept of analytic expression.(a)The relative humidity is less than 60%, (b)the relative humidity is more than 60%.

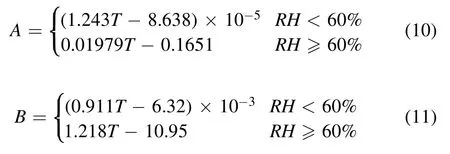
where A and B are the slope and intercept of analytic expression, respectively.

In summary, the charging factor of suspension droplets can be obtained as follows:

3.5.Validation of analytic expression

In order to verify the validity of the analytic expression, the ion flow field was measured at a temperature is 15 °C.The charging factor is calculated based on the measurement of the ion flow field and compared with the calculation results of the analytic expression.The comparison results are shown in figure 8.

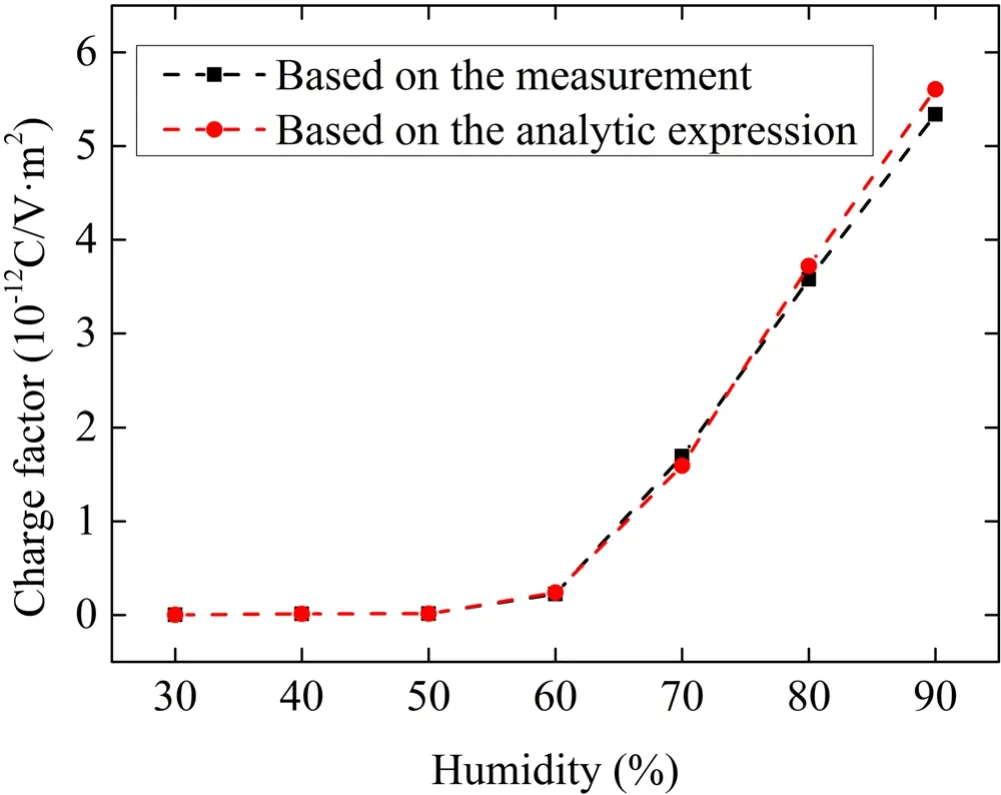
Figure 8.Comparison of calculated values based on the measurement of the ion flow field and analytic expression at T = 15 °C.

The comparison results show that the maximum error is 6.4%,which indicates that the analytic expression can be used to calculate the charging factor and space charge density of suspension droplets and model the ion flow field considering the influence of temperature and humidity.

4.Influence of charged suspension droplets on the ion flow field

The charging factor is introduced into equation (2)to solve the space charge density under different temperatures and humidity.When the applied voltage on the conductor is 50 kV, the percentages of suspension droplets charge density(ρw)and electric field component (Ew)generated by charged suspension droplets at the cage surface are calculated,and the calculation results are shown in figures 9 and 10.

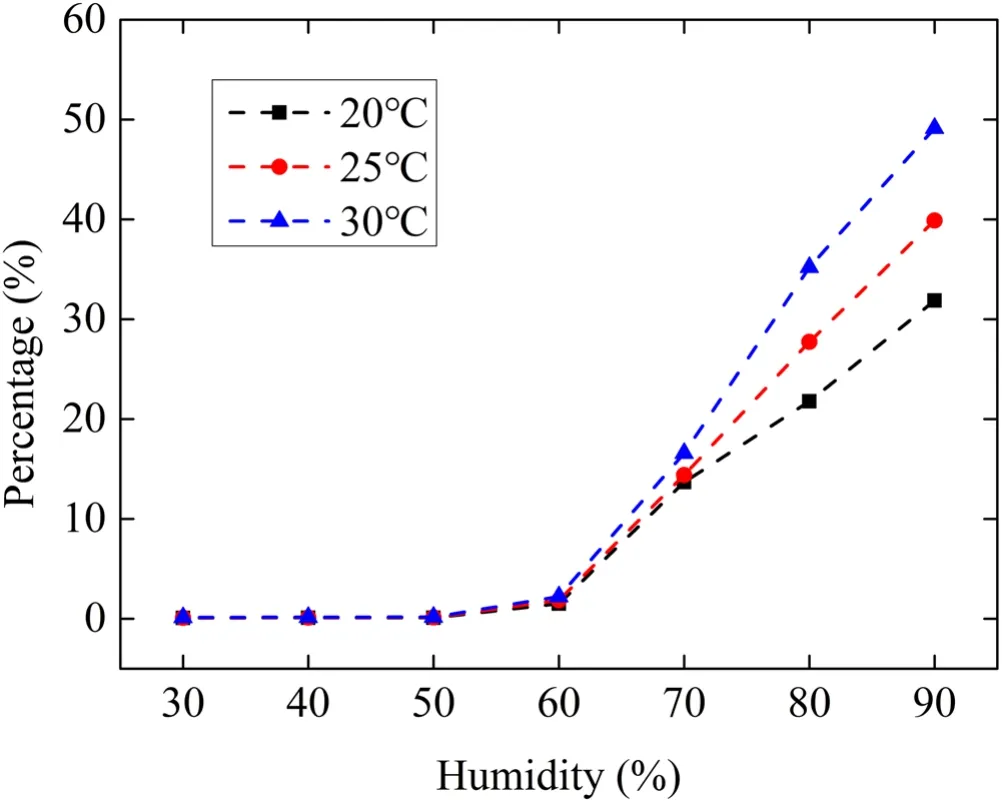
Figure 9.The percentage of suspension droplets charge density.

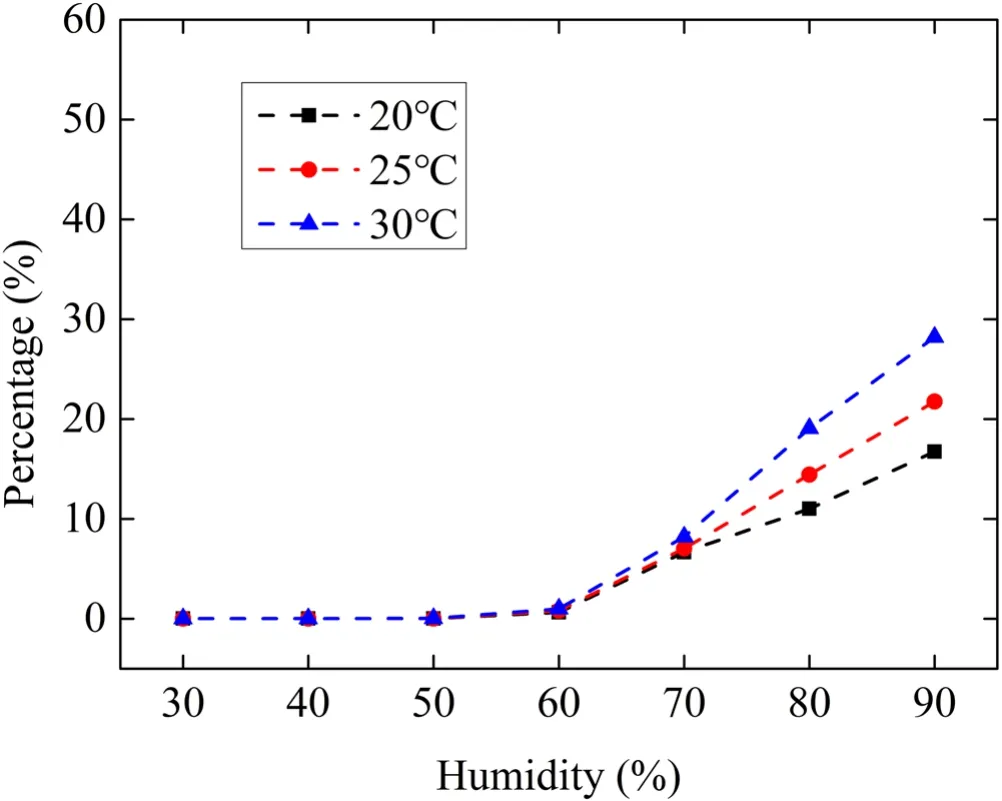
Figure 10.The percentage of electric field component.

As seen in figures 9 and 10,ρwand Ewincrease with the temperature and humidity.When the relative humidity is less than 60%, ρwand Eware small and increase little with the relative humidity and temperature.When the relative humidity is more than 60%,ρwand Eware large and increase obviously with the increase of relative humidity and temperature, which indicates that the electric field distortion caused by charged suspension droplets becomes more serious and charged suspension droplets have an effect on the characteristics of the ion flow field.

Figure 11.Comparison of the electric field at the cage surface between the cases with and without suspension droplets.(a)T = 20°C, (b)T = 25 °C, (c)T = 30 °C.

When the applied voltage on the conductor is 50 kV,the comparison results of the electric field at the cage surface with and without suspension droplets under different temperatures and humidity are shown in figure 11.

The results show that there is little difference between the electric field with and without suspension droplets at the same temperature and relative humidity of less than 60%.When T = 25 °C and RH = 40%, the difference is 0.5%.The results show that the ion flow field is less affected by suspension droplets,and the influence of suspension droplets can be ignored in engineering calculation.

In an environment of the same temperature and relative humidity of more than 60%, the electric field without suspension droplets is less than that with suspension droplets.The results show that the suspension droplets can enhance the total electric field on the ground.With the increase of relative humidity and temperature,the difference between the electric field with and without suspension droplets is larger.When the temperature is 25 °C, the differences are 3.5% and 7.6% at RH = 70% and RH = 90%, respectively.When the relative humidity is 90%, the differences are 5.7% and 11.1% at T = 20 °C and T = 30 °C, respectively.The above analysis shows that the suspension droplets greatly influence the ion flow field of the HVDC conductor under high temperatures and high humidity.

5.Conclusion

In this paper, the charging factor used to characterize the charge characteristics of suspension droplets is introduced.After, the calculation results of the charging factor are fitted by a linear function, and the analytic expression of the charging factor is obtained.Then, the influence of charged suspension droplets in the ion flow field is analyzed.The results are as follows:

(1)The charging factor is small and increases little with the relative humidity when the relative humidity is less than 60%.When the relative humidity is more than 60%,the charging factor of suspension droplets is larger and increases rapidly with the relative humidity, which indicates that there will be obvious charged suspension droplets in the ion flow field.The charging factor of suspension droplets increases linearly with the increase of temperature at the same relative humidity.

(2)When the relative humidity is less than 60%, the difference between the electric field at the cage surface with and without suspension droplets is small, so the influence of suspension droplets can be ignored in engineering calculation.When the relative humidity is more than 60%,the difference between the electric field at the cage surface with and without suspension droplets is 11.1%at RH = 90%and T = 30°C,so the influence of suspension droplets cannot be ignored in the calculation of ion flow field.

Acknowledgments

This work is supported by National Natural Science Foundation of China (No.52077074).